Bhagya

Punjab State Board PSEB 5th Class Hindi Book Solutions Chapter 18 नारियल का बगीचा-केरल प्रदेश Textbook Exercise Questions and Answers.

PSEB Solutions for Class 5 Hindi Chapter 18 नारियल का बगीचा-केरल प्रदेश

Hindi Guide for Class 5 PSEB नारियल का बगीचा-केरल प्रदेश Textbook Questions and Answers

I. बताओ

प्रश्न 1.
केरल के समुद्री किनारे के साथ-साथ किस चीज़ की पट्टी बिछी हुई है ?
उत्तर:
केरल के समुद्री किनारे के साथ-साथ नारियल के वृक्षों की लम्बी पट्टी बिछी हुई है।

प्रश्न 2.
केरल को क्या कहकर बुलाया जाता है ?
उत्तर:
केरल को नारियल का बगीचा कहकर बुलाया जाता है।

प्रश्न 3.
केरल का प्रिय जानवर कौन-सा है?
उत्तर:
केरल का प्रिय जानवर हाथी है।

प्रश्न 4.
केरल के लोगों का मुख्य त्योहार कौनसा है?
उत्तर:
केरल के लोगों का मुख्य त्योहार ओणम है।

प्रश्न 5.
केरल के लोगों का प्रमुख नृत्य कौन| सा है?
उत्तर:
केरल के लोगों का प्रमुख नृत्य कथकली है।

II. निम्नलिखित प्रश्नों के उत्तर चार-पाँच वाक्यों में लिखें

प्रश्न 1.
केरल को नारियल का बगीचा क्यों कहते हैं ?
उत्तर:
केरल के समुद्र के किनारे-किनारे नारियल के वृक्षों की लम्बी पट्टी बिछी हुई है इसी से केरल को नारियल का बगीचा कहते हैं।

प्रश्न 2.
केरल को भारत का नंदनवन क्यों पुकारा जाता है?
उत्तर:
केरल की सुन्दरता और प्राकृतिक दृश्यों की मनोरमता के कारण इसे भारत का नंदनवन कह कर पुकारा जाता है।

प्रश्न 3.
केरल के जंगलों में कौन-कौन से वृक्ष पाए जाते हैं ?
उत्तर:
केरल के जंगलों में रबड़, चन्दन, सागौन और शीशम के वृक्ष पाए जाते हैं।

प्रश्न 4.
केरल के लोगों का पहरावा कैसा है ?
उत्तर:
केरल के लोग अधिकतर ढीले-ढाले कपड़े पहनते हैं। पुरुष लुंगी एवं कुरता पहनते हैं और स्त्रियाँ धोती और ब्लाउज पहनती हैं।

प्रश्न 5.
नारियल के वृक्ष को केरल का कल्पवृक्ष क्यों कहा जाता है ?
उत्तर:
केरल प्रदेश समुद्र के किनारे बसा हुआ प्रदेश है। यहाँ पर नारियल के वृक्षों की भरमार है। चारों ओर नारियल के ऊँचे-ऊँचे वृक्ष लगे दिखाई देते हैं। नारियल के पेड़ इनके लिए बहुपयोगी हैं। इनसे इनकी बहुत-सी ज़रूरतें पूरी होती हैं। इसीलिए नारियल के वृक्ष को केरल का कल्पवृक्ष कहा जाता है।

प्रश्न 6.
हाथी केरल के लोगों का प्रिय जानवर क्यों है ?
उत्तर;
केरल में घने जंगल मिलते हैं। इन जंगलों में हाथी, शेर, चीता आदि जानवर बहुतायत में होते हैं। हाथी यहाँ का विशेष प्रिय जानवर है। इसे लकड़ियाँ ढोने और सवारी करने के प्रयोग में लाया जाता है।

प्रश्न 7.
केरल निवासी ओणम का त्योहार कैसे मनाते हैं?
उत्तर:
केरल के लोग ओणम का त्योहार बड़ी श्रद्धा और उत्साह से मनाते हैं। इस अवसर पर दुकानों और घरों को सजाया जाता है। घरों में भिन्न- भिन्न पकवान और मिठाइयाँ बनती हैं। लक्ष्मी माता
और नौका की पूजा की जाती है। इस अवसर पर कई प्रतियोगिताएँ भी करवाई जाती हैं। हाथियों को सजा कर सवारी निकाली जाती है। कई सांस्कृतिक कार्यक्रम भी इस अवसर पर करवाए जाते हैं। नौका | प्रतियोगिता का विशेष आकर्षण होता है।

III. निम्नलिखित शब्दों को वाक्यों में प्रयोग करें

(i) तुल्य
(ii) विशेष
(iii) मनोरम
(iv) मुख्य
(v) लोकप्रिय
(vi) शिक्षित
(vii) प्रतियोगिता।
उत्तर:
(i) तुल्य-केरल के प्राकृतिक नज़ारे कश्मीर के तुल्य माने जाते हैं।
(i) विशेष-केरल में ओणम के त्योहार में लोग विशेष रुचि लेते हैं।
(iii) मनोरम-केरल के मनोरम दृश्य देखकर मन आनंदित हो गया।
(iv) मुख्य-चावल और मछली केरल के लोगों का मुख्य भोजन है।
(v) लोकप्रिय- (1) ओणम केरल का लोकप्रिय त्योहार है।
(2) कथकली केरल का लोकप्रिय नृत्य है।
(vi) शिक्षित-केरल के प्रायः सभी लोग शिक्षित हैं।
(vii) प्रतियोगिता-ओणम के अवसर पर यहाँ कई प्रतियोगिताएँ होती हैं।

IV. वचन बदलो

मछली, झीलें, नदी, प्रतियोगिता, लड़की, नाटिका, धोती, रस्सी, चटाई, पत्ती।
उत्तर:
मछली = मछलियाँ।
झीलें = झील
नदी = नदियाँ।
प्रतियोगिता = प्रतियोगिताएँ।
लड़की = लड़कियाँ।
नाटिका = नाटिकाएँ।
धोती = धोतियाँ
रस्सी = रस्सियाँ।
चटाई = चटाइयाँ।
पत्ती = पत्तियाँ।

V. समान-अर्थ वाले शब्द लिखो

तट, खूबसूरत, मनोहारी, बहुतायत, भरमार।
उत्तर:
समानार्थक शब्द-
तट = किनारा, कगार।
खूबसूरत = सुन्दर, मनोहर।
मनोहारी = आकर्षक, सुन्दर।
बहुतायत = ज़्यादातर, अधिकतर।
भरमार = बहुत अधिक, ज्यादा।

VI. शुद्ध करके लिखो

परदेशों, परसिध, पशचिम, पूरब, तामीलनाडु, करनाटक, दकशिन, नारीअल, मनौहारी, कलपवृक्ष, गुनकारी, खुबसूरत, पियाले, उदयोग-दन्दे, विशेश, सीदे-सादे।
उत्तर:
परदेशों = प्रदेशों।
परसिध = प्रसिद्ध।
पशचिम = पश्चिम।
पूरब = पूर्व।
तामीलनाडु = तमिलनाडु।
करनाटक = कर्नाटक।
दकशिन = दक्षिण।
नारीअल = नारियल।
मनौहारी = मनोहारी।
कलपवृक्ष = कल्पवृक्ष।
गुनकारी = गुणकारी
खुबसूरत = खूबसूरत।
पियाले = प्याले।।
उदयोग-दन्दे = उद्योग-धंधे
विशेश = विशेष।
सीदे-सादे = सीधे-सादे।

VII. पाठ में से ढूँढ़कर पाँच युग्म शब्द लिखें जैसे-

अलग-अलग
उत्तर:
युग्म शब्द
(i) किनारे – किनारे।
(ii) अलग – अलग।
(iii) सीधे – सादे।
(iv) भिन्न – भिन्न।
(v) ढीले – ढाले।

VIII. ठीक (✓) या (✗) गलत ढूँढकर चिह्न लगाएँ

1. नारियल की लकड़ी का प्रयोग क्रिकेट का बल्ला बनाने में किया जाता है।
2. केरल में मौसम अधिकतर गर्म रहता है।
3. केरल में ज़्यादातर लोग खेतीबाड़ी करते हैं।
4. केरल प्रदेश में नीलगिरी पर्वत फैला हुआ है।
5. केरल के लोग रेलगाड़ी से अधिक सफर करते हैं।
6. केरल का प्रसिद्ध नृत्य कथकली है।
उत्तर:
(1) ✗
(2) ✓
(3) ✓
(4) ✓
(5) ✗
(6) ✓

IX. वाक्य पूरे करो

(चावल, अरब सागर, श्रद्धा, हिन्द महासागर, उत्साह, साबुन, हाथी, कश्मीर)
1. केरल के पूर्व में ………………….. और दक्षिण में…………….है।
2. नारियल तेल ……………. बनाने में भी काम आता है।
3. केरल के प्राकृतिक दृश्य.. ……………. के -ुल्य माने जाते हैं।
4. लकड़ियाँ ढोने और सवारी करने में ……………. का प्रयोग किया जाता है।
5. इडली, डोसा, उत्पम आदि ……………. से बनते हैं।
6. ओणम का त्योहार …………..और ………… से मनाया जाता है।
उत्तर:
1. अरब सागर, हिन्द महासागर।
2. साबुन।
3. कश्मीर।
4. हाथी।
5. चावल।
6. श्रद्धा, उत्साह।

X. सोचिए और लिखिए

(1) केरल प्रदेश से सम्बन्धित चित्र ढूँढ़कर अपनी कापी पर चिपकाएँ।
(2) भारत के अन्य प्रदेशों के बारे में जानकारी मैगज़ीनों, अखबारों, लाइब्रेरी की पुस्तकों से प्राप्त करें और संग्रह करें।
(3) किसी प्रदेश की यात्रा करें तो उसका अनुभव अपनी डायरी में ज़रूर लिखें।
उत्तर:
विद्यार्थी अपने अध्यापकों की सहायता एवं अपने अनुभव द्वारा इन्हें लिखें।

बहुवैकल्पिक प्रश्न

पूछे गए प्रश्नों के सही विकल्प पर (✓) निशान लगाएं

प्रश्न 1.
हमारे देश में कितने प्रदेश हैं?
(क) 28
(ख) 29.
(ग) 30
(घ) 31.
उत्तर:
(क) 28

प्रश्न 2.
केरल कौन-सी दिशा में स्थित है?
(क) पूर्व
(ख) पश्चिम
(ग) उत्तर
(घ) दक्षिण।
उत्तर:
(घ) दक्षिण।

प्रश्न 3.
केरल के पूर्व में कौन-सा सागर है?
(क) अरब सागर
(ख) हिन्द महासागर
(ग) दीप सागर
(घ) अंध सागर।
उत्तर:
(क) अरब सागर

प्रश्न 4.
केरल के दक्षिण में कौन-सा सागर है?
(क) अरब सागर
(ख) हिन्द महासागर
(ग) दीप सागर
(घ) अंध सागर।
उत्तर:
(ख) हिन्द महासागर

प्रश्न 5.
केरल की राजधानी कौन-सी है ?
(क) भूटान
(ख) तिरुवनंतपुरम
(ग) पुरम
(घ) आर. के. पुरम।
उत्तर:
(ख) तिरुवनंतपुरम

प्रश्न 6.
केरल को क्या कह कर बुलाया जाता है?
(क) वृक्षों का वगीचा
(ख) नदियों का बगीचा
(ग) फूलों का बगीचा
(घ) नारियल का बगीचा।
उत्तर:
(घ) नारियल का बगीचा।

प्रश्न 7.
केरल का प्रिय जानवर कौन-सा है?
(क) हाथी
(ख) घोड़ा
(ग) जेबरा
(घ) गैंडा।
उत्तर:
(क) हाथी

प्रश्न 8.
केरल का मुख्य त्योहार कौन-सा है?
(क) दीवाली
(ख) होली
(ग) ओणम
(घ) वैशाखी।
उत्तर:
(ग) ओणम

प्रश्न 9.
केरल के लोगों का प्रमुख नृत्य कौन-सा है ?
(क) कथकली
(ख) कथाकली
(ग) कली
(घ) कथा।
उत्तर:
(क) कथकली

प्रश्न 10.
केरल को भारत का क्या कहा जाता है?
(क) नंद
(ख) नंदनवन
(ग) वन
(घ) नंदी।
उत्तर:
(ख) नंदनवन

नारियल का बगीचा-केरल प्रदेश Summary

नारियल का बगीचा-केरल प्रदेश पाठ का सार

हमारे देश भारत में 28 प्रदेश हैं जिनमें दक्षिण में स्थित केरल प्रदेश समृद्ध और प्रसिद्ध है। इसके पूर्व में अरब सागर और दक्षिण में हिन्द महासागर है। इसकी राजधानी तिरुवनंतपुरम है। इस राज्य के पश्चिम में तमिलनाड़ राज्य है। इस राज्य में नारियल के इतने अधिक पेड़ हैं कि इसे नारियल का बगीचा भी कहा जाता है। यहाँ के लोगों की बहुत अधिक आवश्यकताएँ नारियल के वृक्षों से पूरी होती है। यहाँ अधिक गर्मी या सर्दी नहीं होती। इस राज्य में बहुत अधिक वर्षा होती है। यहाँ के अधिकांश उद्योग-धन्धे जंगलों पर निर्भर करते हैं। यहाँ चावल, गर्म मसाले और चाय बहुत अधिक होती है। नदियों, नहरों और झीलों की अधिकता के कारण इधर-उधर जाने के लिए नावों का प्रयोग किया जाता है। यहाँ सड़कें तो पर्याप्त हैं पर रेलों की संख्या अधिक नहीं है। यहाँ के लोगों का मुख्य भोजन चावल और मछली है। यहाँ के लोग सीधे-सादे और नृत्य संगीत के शौकीन हैं। अधिकतर लोग ढीले-ढाले वस्त्र पहनते हैं। यहाँ का मुख्य त्योहार ओणम है। इस दिन पर्व मनाने के साथ-साथ नौकाओं की दौड आयोजित की जाती है। हाथियों को सजा-संवार कर देवताओं की सवारी निकाली जाती है। यहाँ घूमने के लिए जाने का उचित समय अक्तूबर-नवम्बर महीना है।

कठिन शब्दों के अर्थ:

समृद्ध = सम्पन्न, अमीर। प्रदेश = इलाका। तट = किनारे। चार-चाँद लगाना = शोभा बढ़ाना। मनोहारी = मन को हरने (अच्छा लगने) वाला। दृश्य = नज़ारा। तुल्य = समान, बराबर। बहुतायत = बहुत अधिक। इस्तेमाल = प्रयोम। लोकप्रिय = प्रसिद्ध। शिक्षित = पढ़ेलिखे। शौकीन = शौक रखने वाले। हिस्सा = भाग। प्राकृतिक दृश्य = कुदरती नज़ारे। सांस्कृतिक = संस्कृति से जुड़े हुए। तारीफ = प्रशंसा। पकवान = खाने की चीजें, भोजन। श्रद्धा = विश्वास। अर्चना = प्रार्थना। आधारित = निर्भर। मुख्य = खास। तुल्य = समान। भरमार = अधिकता। आधारित = निर्भर।

Punjab State Board PSEB 5th Class Hindi Book Solutions Chapter 19 रेत और पत्थर Textbook Exercise Questions and Answers.

PSEB Solutions for Class 5 Hindi Chapter 19 रेत और पत्थर

Hindi Guide for Class 5 PSEB रेत और पत्थर Textbook Questions and Answers

I. बताओ

प्रश्न 1.
दोनों दोस्त कहाँ टहल रहे थे?
उत्तर:
दोनों दोस्त समुद्र किनारे टहल रहे थे।

प्रश्न 2.
एक दोस्त ने दूसरे दोस्त को चाँटा क्यों मारा ?
उत्तर:
दोनों दोस्तों में बहस हो गई और बात इतनी बढ़ गई कि एक दोस्त ने दूसरे दोस्त को चाँटा मारा।

प्रश्न 3.
चाँटा लगने पर दोस्त ने क्या किया?
उत्तर:
चाँटा लगने पर दोस्त ने समद्र किनारे रेत पर लिखा-“आज मेरे सबसे प्यारे दोस्त ने मुझे चाँटा मारा है।”

प्रश्न 4.
रेत और पत्थर’ में एक दोस्त बेहोश क्यों हो गया?
उत्तर:
समुद्र में नहाते हुए अचानक उसका पैर पानी के नीचे दलदल में फँस गया और वह डूबने लगा। उसके दोस्त ने उसे बचा लिया, लेकिन वह बेहोश हो गया।

प्रश्न 5.
होश आने पर उसने क्या किया?
उत्तर:
होश आने पर उसने पत्थर पर लिख दिया “आज मेरे सबसे अच्छे दोस्त ने मेरी जान बचायी।”

प्रश्न 6.
इस कहानी से हमें क्या शिक्षा मिलती
उत्तर:
इस कहानी से हमें शिक्षा मिलती है कि अपने दुखों और कष्टों को रेत पर लिखना और अपने प्रति दूसरों के द्वारा किया गया अच्छा कार्य पत्थर या हृदय पर लिखना चाहिए जिसे कोई कभी न मिटा सके।

II. समानार्थक शब्द लिखो

दोस्त = मित्र, सखा
किनारा = ……….. , ………..
चाँटा = ……….. , ………..
पैर = ……….. , ………..
बाँह = ……….. , ………..
पत्थर = ……….. , ………..
लहर = ……….. , ………..
उत्तर:
समानार्थक शब्द
दोस्त = मित्र, सखा।
किनारा = तट, कूल।
चाँटा = चपत, थप्पड़।
पैर = पग, पाँव।
बाँह = हाथ, भुजा।
पत्थर = पाहन, पाथर।
लहर = तरंग, उर्मि।

III. निम्नलिखित वाक्यों को निर्देशानुसार बदलें

1. दो दोस्त समुद्र के किनारे टहल रहे थे। (वर्तमान काल में बदलो)
2.आज मेरे सबसे अच्छे दोस्त ने मेरी जान बचाई (भविष्य काल में बदलो)
3. वह डूबने लगा। (वर्तमान काल में बदलो)
4. हल्की बेहोशी के बाद जब उसे होश आया तो उसने स्वयं को दोस्त की बाँहों में पाया। (भविष्य काल में बदलो)
5. एक ने दूसरे के गाल पर कसकर चाँटा मारा। (वर्तमान काल में बदलो)
उत्तर:
1. दो दोस्त समुद्र के किनारे टहल रहे. हैं।
2. मेरे सबसे अच्छे दोस्त मेरी जान बचाएँगे।
3. वह डूब रहा है।
4. हल्की बेहोशी के बाद जब उसे होश आएगा तो वह स्वयं को दोस्त की बाँहों में पाएगा।
5. एक, दूसरे के गाल पर कसकर चाँटा मारते हैं।

IV. इस पत्थर में सभी शब्द घुल-मिल गए हैं। उनमें से क्रिया शब्द छाँटकर उनसे उचित वाक्य बनाएँ।

उत्तर:
क्रिया शब्द – वाक्य
(1) टहलना – हम शाम को बाग में टहल रहे थे।
(2) मारना – अध्यापक ने मोहन को चाँटा मारा।
(3) बचाना – मैंने अपने दोस्त को बचाया।
(4) सीखना – मैंने कार चलानी सीखी।
(5) लिखना – वह लिखता है।
(6) तराशना – वह पत्थर तराशता है।

V. विलोम शब्द लिखो

(i) दोस्त
(ii) मारना
(iii) नीचे
(iv) होश
(v) दुःख
(vi) अच्छा
उत्तर:
विलोम शब्द
(i) दोस्त = दुश्मन।
(ii) मारना = बचाना।
(iii) नीचे = ऊपर।
(iv) होश = बेहोश।
(v) दुःख = सुख।
(vi) अच्छा = बुरा।

VI. सर्वनाम भरकर वाक्य पूरे करो

1. …………… सबसे प्यारे दोस्त ने चाँटा मारा।
2. इस बार ………….. पत्थर पर लिखा है ऐसा क्यों।
3. ………….. स्वयं को दोस्त की बाँहों में पाया।
4. ……………….. डूबने लगा।
5. ……….. पैर पानी के नीचे दलदल में फंस गया।
उत्तर:
(1) मेरे
(2) तुमने
(3) मैंने
(4) वह
(5). उसका

कुछ करने के लिए-

1. यदि आपने कभी किसी की सहायता की है तो उसका अनुभव लिखें।
2. वीर बालकों की कथा-कहानियाँ पढ़ें।

नोट- विद्यार्थी स्वयं के अनुभव से लिखने का प्रयास करें।

बहुवैकल्पिक प्रश्न

पूछे गए प्रश्नों के सही विकल्प पर (✓) निशान – लगाएं

प्रश्न 1.
दोनों दोस्त कहाँ घूमने गए?
(क) समुद्र के बीच
(ख) समुद्र के किनारे
(ग) नदी के किनारे
(घ) तालाब के किनारे।
उत्तर:
(ख) समुद्र के किनारे

प्रश्न 2.
एक दोस्त ने दूसरे को क्या मारा?
(क) चाँटा
(ख) डंडा
(ग) हाथ
(घ) कांटा।
उत्तर:
(क) चाँटा

प्रश्न 3.
एक दोस्त का पैर कहाँ फंस गया?
(क) दलदल में
(ख) पानी में
(ग) पत्थर में
(घ) समुद्र में।
उत्तर:
(क) दलदल में

प्रश्न 4.
एक दोस्त की जान किसने बचाई ?
(क) तैराक ने
(ख) माँ ने
(ग) पिता ने
(घ) दूसरे दोस्त ने।
उत्तर:
(घ) दूसरे दोस्त ने।

रेत और पत्थर Summary

रेत और पत्थर पाठ का सार

दो दोस्त समुद्र के किनारे घूम रहे थे। किसी बात पर बहस करते हुए एक ने दूसरे की गाल पर चाँटा मार दिया। जिसे चाँटा लगा उसने बिना उससे कुछ कहे रेत पर लिखा-‘आज मेरे सबसे प्यारे दोस्त ने मुझे चाँटा मारा।’ वे दोनों एक साथ घूमते रहे। समुद्र में भाटे के कारण जल स्तर कम होते ही दोनों उसमें नहाने लगे। अचानक वह दोस्त जिसे चाँटा लगा था वह दलदल में धंस कर डूबने लगा पर उसके दोस्त ने उसे बचा लिया। समुद्र से बाहर आकर उसने पत्थर पर लिखा-‘आज मेरे सबसे अच्छे दोस्त ने मेरी जान बचायी है।’ दूसरे दोस्त के द्वारा उसके द्वारा लिखने के लिए रेत और पत्थर के चुनाव के बारे में पूछा तो उसने उत्तर दिया कि जब कोई हमें दुःख या कष्ट देता है तो उसे रेत पर लिख देना चाहिए क्योंकि भुलावे रूपी लहरें और हवाएं उसे मिटा देती। हैं पर जब कोई हमारे लिए अच्छा करता है तो उसे पत्थर पर तराशकर लिख देना चाहिए जहाँ कोई हवा या पानी उसे कभी न मिटा सके।

कठिन शब्दों के अर्थ:

टहल = घूम। नौबत = स्थिति। आघात = चोट। भाटा = समुद्र के पानी का उतार। दलदल = कीचड़ से भरी जगह।

Punjab State Board PSEB 5th Class Hindi Book Solutions Chapter 20 भूल गया है क्यों इंसान Textbook Exercise Questions and Answers.

PSEB Solutions for Class 5 Hindi Chapter 20 भूल गया है क्यों इंसान

Hindi Guide for Class 5 PSEB भूल गया है क्यों इंसान Textbook Questions and Answers

I. बताओ

प्रश्न 1.
इन्सान किस बात को भूल गया है?
उत्तर:
सब इन्सान मिट्टी के पुतले हैं और सबमें एक-जैसी जान है, इस बात को इन्सान भूल गया है।

प्रश्न 2.
अनेक देशों में किस की सन्तान बसी
उत्तर:
अनेक देशों में उसी परमेश्वर की सन्तान बसी हुई है।

प्रश्न 3.
देश और वेश-भूषा अलग होने पर भी मानव में क्या समानता है ?
उत्तर:
अलग-अलग देश और लोगों के पहनावे भिन्न-भिन्न होने पर भी सभी मानव समान हैं, क्योंकि सब में एक जैसे प्राण हैं।

II. सरलार्थ करो

सबकी है मिट्टी की काया
सब पर नभ की निर्मल छाया
यहाँ नहीं कोई आया है, ले विशेष वरदान।
भूल गया है क्यों इन्सान॥
उत्तर:
कवि कहता है कि मनुष्य यह बात क्यों भूल गया है कि शरीर मिट्टी का बना हुआ है। सबके सिर पर आकाश की स्वच्छ छाया है। इस संसार में कोई भी विशेष वरदान लेकर नहीं आया है। भाव यह है कि सब समान हैं।

III. समान तुक वाले शब्द मिलाओ

काया	वेश
उपजाए	छाया
देश	सन्तान
वरदान	बनाए।

उत्तर:
काया – छाया।
उपजाए – बनाए।
देश – वेश।
वरदान – सन्तान।

IV. चित्र को देखो. और पाँच पंक्तियाँ लिखो

उत्तर:
हमारे देश में अलग-अलग जातियों और धर्मों के लोग रहते हैं। उन सबकी वेशभूषा और रहन- सहन अलग-अलग है। हिन्दू लोग धोती और कुर्ता पहनते हैं तो मुस्लिम लोग कुर्ता-पायजामा पहनते हैं। औरतें सूट-सलवार तथा घाघरा चोली पहनती हैं। यहाँ लोग भारतीय वेशभूषा के साथ-साथ पश्चिमी वेशभूषा भी पहनते हैं। फिर भी सब मिलजुल कर रहते हैं इसीलिए मेरा भारत महान् है।

V. समानार्थक शब्द लिखो

(i) इन्सान = मानव, मनुष्य
(ii) काया = …………. , ………….
(iii) धरा = ………….. , …………….
(iv) सन्तान = …………… , …………..
उत्तर:
(i) इन्सान = मानव, मनुष्य।
(ii) काया = तन, शरीर।
(iii) धरा = वसुधा, वसुन्धरा।
(iv) सन्तान = पुत्र, तनय।

VI. करिए

कविता को हाव-भाव के साथ गाएँ।

VII. नए शब्द बनाएँ

(i) मिट्टी = ट् + ट = ……………
(ii) इन्सान = + स = …………….
(iii) निर्मल = र् + म = ……………
उत्तर:
(i) खट्टा
(ii) इन्सानियत।
(iii) निर्मम।

बहुवैकल्पिक प्रश्न

पूछे गए प्रश्नों के सही विकल्प पर (✓) निशान लगाएं

प्रश्न 1.
इन्सान की काया किसकी है?
(क) मिट्टी की
(ख) सोने की
(ग) चाँदी की
(घ) खून की।
उत्तर:
(क) मिट्टी की

प्रश्न 2.
मानव किसने उपजाए?
(क) पिता ने
(ख) माँ ने
(ग) धरती ने
(घ) नभ ने।
उत्तर:
(ग) धरती ने

प्रश्न 3.
सभी देशों में किसकी संतान बसी है?
(क) परमेश्वर की
(ख) पिता की
(ग) माँ की
(घ) दोनों की।
उत्तर:
(क) परमेश्वर की

प्रश्न 4.
सभी मनुष्यों में एक जैसा क्या है ?
(क) प्राण
(ख) धन
(ग) सम्मान
(घ) वैभव।
उत्तर:
(क) प्राण

भूल गया है क्यों इंसान Summary

भूल गया है क्यों इंसान पाठ का सार

कवि मनुष्य को याद कराना चाहता है कि वह क्यों भूल गया है कि सबका शरीर मिट्टी से बना है। वह नाशवान है और सब पर आकांश की निर्मल छाया है। सदा के लिए यहाँ रहने का वरदान लेकर कोई नहीं आया है। चाहे इस संसार में लोगों के बनाए अनेक देश हैं पर सभी मनुष्यों का जीवन धरती के कारण ही है। चाहे देश भिन्न हों, उनका पहनावा अलग हो पर सभी मनुष्यों में प्राण तो एक-से ही हैं।

पद्यांशों के सरलार्थ

1. भूल गया है क्यों इन्सान।
सबकी है मिट्टी की काया,
सब पर नभ की निर्मल छाया,
यहाँ नहीं कोई आया है,
ले विशेष वरदान।

कठिन शब्दों के अर्थ:
इन्सान = मनुष्य। काया = शरीर। नभ = आकाश। निर्मल = स्वच्छ। छाया = छांव।

प्रसंग:
प्रस्तुत पद्यांश हमारी पाठ्य-पुस्तक में संकलित ‘बच्चन’ जी द्वारा लिखित कविता ‘भूल गया है क्यों इन्सान’ में से लिया गया है।

सरलार्थ:
कवि कहता है कि मनुष्य यह बात क्यों भूल गया है कि शरीर मिट्टी का बना हुआ है। सबके सिर पर आकाश की स्वच्छ छाया है। इस संसार में कोई भी विशेष वरदान लेकर नहीं आया है। भाव यह है कि सब समान हैं।

भावार्थ:
मनुष्य मरणधर्मा है। उसे निश्चित रूप से संसार छोड़कर मृत्यु को प्राप्त करना ही है।

2. भूल गया है क्यों इन्सान।
धरती ने मानव उपजाए,
मानव ने ही देश बनाए,
बहु देशों में बसी हुई है,
एक धरा-संतान।

कठिन शब्दों के अर्थ:
मानव = मनुष्य। उपजाए = पैदा किए। बहु = बहुत। धरा = धरती।

सरलार्थ:
कवि कहता है कि-मनुष्य इस बात को क्यों भूल गया है कि इस धरती ने मनुष्य को पैदा किया है। फिर मनुष्य ने देशों को बनाया है, बहुत-से देशों में यह धरती की सन्तान (मनुष्य) बसी हुई है।

भावार्थ:
इन्सान ने धरती पर रहते हुए भेदभाव के कारण देशों में भूमि को बाँट दिया है।

3. भूल गया है क्यों इन्सान।
देश अलग हैं, देश अलग हों,
वेश अलग हैं, वेश अलग हों,
मानव का मानव से लेकिन,
अलग न अन्तर-प्राण
भूल गया है क्यों इन्सान।

कठिन शब्दों के अर्थ:
वेश = पहनावा। अन्तर-प्राण = जीवात्मा।

सरलार्थ:
कवि कहता है-मनुष्य यह क्यों भूल गया है कि देश अलग-अलग हैं और भले ही ये अलग-अलग हों। मनुष्य के पहनावे अलग हैं और चाहे वे अलग-अलग हों, परन्तु मनुष्य में मौजूद जीवात्मा सब में समान है।

भावार्थ:
इन्सानों में चाहे भेदभाव है पर उनमें विद्यमान जीवात्मा एक ही होती है।

Punjab State Board PSEB 5th Class Hindi Book Solutions Chapter 21 तेंदुए से मुठभेड़ Textbook Exercise Questions and Answers.

PSEB Solutions for Class 5 Hindi Chapter 21 तेंदुए से मुठभेड़

Hindi Guide for Class 5 PSEB तेंदुए से मुठभेड़ Textbook Questions and Answers

I. बताओ

प्रश्न 1.
छवि के गाँव का नाम बताएँ।
उत्तर:
छवि के गाँव का नाम है-खटूखाल।

प्रश्न 2.
छवि पर किसने हमला किया ?
उत्तर:
छवि पर नरभक्षी तेंदुए ने हमला किया।

प्रश्न 3.
छवि ने तेंदुए पर कहाँ वार किया ?
उत्तर:
छवि ने तेंदुए के पेट पर वार किया।

प्रश्न 4.
तेंदुआ अपने शिकार को छोड़कर क्यों भाग गया ?
उत्तर:
छवि द्वारा अचानक किए गए वार से तेंदुआ घबरा गया और वह अपने शिकार को छोड़कर भाग गया।

प्रश्न 5.
गाँव वाले उसे सबसे पहले किस अस्पताल में लेकर गए?
उत्तर:
गाँव वाले उसे सबसे पहले गाँव के प्राथमिक अस्पताल में लेकर गए।

प्रश्न 6.
किस अस्पताल के डॉक्टरों ने छवि की जान बचायी और कैसे ?
उत्तर:
पी० जी० आई० चण्डीगढ़ के अस्पताल के डॉक्टरों ने आप्रेशन करके छवि की जान बचाई।

II. निम्नलिखित मुहावरों के वाक्य बनाएँ

मुहावरा – अर्थ – वाक्य
1. मन में लड्डू फूटना = खुश होना ……………..
2. फूला न समाना = खुश होना – ……………
3. पैरों तले जमीन खिसक जाना = होश हवास न रहना – ………….
4. मौत सिर पर खेलना = मौत करीब होना – ………………
उत्तर:
(1) मन में लड्डू फूटना = खुश होना – शिकार को अपने सामने पाकर तेंदुए के मन में लड्डू फूटने लगे।
(2) फूला न समाना = खुश होना-कक्षा में प्रथम आने का समाचार पाकर सुरेश तो फूला नहीं समा रहा था।
(3) पैरों तले जमीन खिसक जाना = होशहवास न रहना-अचानक हुए प्रहार से तेंदुआ घबरा गया उसके तो जैसे पैरों तले से ज़मीन खिसक गई।
(4) मौत सिर पर खेलना = मौत करीब होनाछवि को अहसास हो गया कि मौत उसके सिर पर खेल रही है।

III. पढ़ो, समझो और लिखो

1. वर्तमानकाल: उसका शिकार उसके सामने है।
भूतकाल: उसका शिकार उसके सामने था।
भविष्यकाल: उसका शिकार उसके सामने होगा।

2. वर्तमान काल : ……………
भूतकाल : छवि बुरी तरह से घबरा गई थी।
भविष्यकाल : ………….
उत्तर:
वर्तमान काल : छवि बुरी तरह से घबरा रही है।
भूतकाल : छवि बुरी तरह से घबरा गई थी।
भविष्यत् काल : छवि बुरी तरह से घबरा जाएगी।

3. वर्तमान काल: …………..
भूतकाल: ………….
भविष्यकाल: तेंदुआ जानवरों और लोगों को अपना शिकार बनाएगा।
उत्तर:
वर्तमान काल : तेंदुआ जानवरों और लोगों को अपना शिकार बनाता है।
भूतकाल : तेंदुए ने जानवरों और लोगों को अपना शिकार बनाया।
भविष्यत् काल : तेंदुआ जानवरों और लोगों को अपना शिकार बनाएगा।

IV. नीचे दिए गए शब्दों के बहुवचन रूप लिखें

(i) झोंपड़ी = झोंपड़ियाँ।
(ii) बात = बातें।
(iii) बेटी = ………….
(iv) औरत = ……………
(v) कहानी = …………….
(vi) भैंस = …………..
(vii) छुट्टी = …………..
(vii) गर्दन = …………..
उत्तर:
बहुवचन
(i) झोंपड़ी = झोंपड़ियाँ।
(ii) बात = बातें।
(iii) बेटी = बेटियाँ।
(iv) औरत = औरतें।
(v) कहानी = कहानियाँ।
(vi) भैंस = भैंसें।
(vii) छुट्टी = छुट्टियाँ
(vii) गर्दन = गर्दनें।

V. शुद्ध शब्द पर गोला लगाएँ-

(1) परिचय/परीचय/परीचै
(2) कब्जे/कबजे/कब्जे
(3) हिमत/हीमत/हिम्मत।
(4) झोंपड़ी/झोंपड़ी/झोंपड़ी
(5) पराथमिक/प्राथमिक/प्राथमीक
(6) आप्रेशन/ओपरेशन/आपरेशन।
उत्तर:
(1) परिचय।
(2) कब्जे।
(3) हिम्मत।
(4) झोंपड़ी।
(5) प्राथमिक।
(6) आप्रेशन।

VI. निम्नलिखित प्रत्येक शब्द समूह में से सबसे जो अलग हो, उस पर गोला लगाएँ।

(1) हिम्मत, बहादुरी, ताकत, घबराहट
(2) पेट, खेल, मुँह, गर्दन
(3) वार, चोट, बात, प्रहार
(4) भागना, छुट्टी, दौड़ना, रुकना।
(5) चण्डीगढ़, गाँव, देहरादून, खटूखाल
(6) अस्पताल, डॉक्टर, कल्पना, आप्रेशन।
उत्तर:
(1) घबराहट
(2) खेल
(3) बात
(4) छुट्टी
(5) गाँव
(6) कल्पना।

VII. पाठ में आए निम्नलिखित अव्यवस्थित शब्दों को व्यवस्थित करके लिखें

चा क अ न, बो क द र च, ड़ क फा र,
अचानक, …………. , …………..
……….., …………., …………
ता अ स प ल, जू बा द व, याँ क नि हा .
…………. , …………….. , ………..
…………. , …………….. , ………..
…………. , …………….. , ………..
उत्तर:
(1) अचानक।
(2) दबोचकर।
(3) फाड़कर।
(4) अस्पताल।
(5) बावजूद।
(6) कहानियाँ।

VIII. निम्नलिखित में से संज्ञा, सर्वनाम, क्रिया शब्द छाँटें-

प्रश्न 1.
उसकी गर्दन से खून बह रहा था
उत्तर:
संज्ञा – गर्दन, खून
सर्वनाम – उसकी
क्रिया – बह रहा था

प्रश्न 2.
बहादुर छवि के आगे उसको भागना पड़ा …………. , …………..
उत्तर:
संज्ञा – छवि।
सर्वनाम – उसको
क्रिया – भागना।

प्रश्न 3.
उसने तेंदुए के पेट पर लगातार लात मारनी शुरू कर दी …………. , …………….
उत्तर:
संज्ञा – तेंदुए, पेट, लात।
सर्वनाम – उसने
क्रिया – मारनी।

प्रश्न 4.
छवि ने उसके जबड़े को चीर दिया। ……………. , ……………..
उत्तर:
संज्ञा – छवि, जबड़े।
सर्वनाम – उसके
क्रिया – चीर दिया।

प्रश्न 5.
वह नरभक्षी तेंदुए के चंगुल से बच गयी। …………. , …………..
उत्तर:
संज्ञा – तेंदुए।
सर्वनाम – वह
क्रिया – बच गई।

बहुवैकल्पिक प्रश्न

पूछे गए प्रश्नों के सही विकल्प पर (✓) निशान लगाएं

प्रश्न 1.
छवि के गांव का नाम क्या है?
(क) खटूखाल
(ख) देशवाल
(ग) नैनीताल
(घ) देहरादून।
उत्तर:
(क) खटूखाल

प्रश्न 2.
छवि पर किसने हमला किया ?
(क) शेर
(ख) चीता
(ग) तेंदुआ
(घ) बाघ
उत्तर:
(ग) तेंदुआ

प्रश्न 3.
छवि ने तेंदुए को क्या मारा.?
(क) लात
(ख) मुक्के
(ग) लाठी
(घ) तलवार।
उत्तर:
(क) लात

प्रश्न 4.
छवि ने तेंदुए का क्या किया ?
(क) भगा दिया
(ख) मार दिया
(ग) जला दिया
(घ) छोड़ दिया।
उत्तर:
(क) भगा दिया

तेंदुए से मुठभेड़ Summary

तेंदुए से मुठभेड़ पाठ का सार

उत्तराखण्ड के उत्तरकाशी में खटूखाल नाम के एक छोटे से गाँव की यह एक सच्ची घटना है। 18 जुलाई, सन् 2010 की घटना है। छवि नामक एक औरत अपनी बेटी के साथ सो रही थी। अचानक रात के समय एक नरभक्षी तेंदुआ वहाँ आ गया। उसने भैंसों को बंधे हुए देखा तो दूसरी ओर सोई हुई छवि और उसकी बेटी को देखा। इतने सारे शिकार एक साथ देखकर तेंदुआ बहुत खुश हुआ। वह बिना किसी आवाज़ के धीरे-धीरे छवि के पास पहुँचा। उसने छवि की गर्दन पर वार किया और उसे अपने मुँह में दबाकर वहाँ से दौड़ पड़ा। छवि बुरी तरह घबरा गई थी। उसे इस बात का पता चल गया कि अब मृत्यु उसके समीप ही है। अचानक उसमें इतनी ताकत आ गई कि उसने अपने आप को छुड़ाने के लिए तेंदुए के पेट पर लात मारनी शुरू कर दी। तेंदुए ने इसकी कल्पना भी नहीं की थी। वह अप्रत्याशित मार से घबरा गया और एक पल के लिए रुका।

उसी पल छवि ने उससे छूटने की कोशिश करते हुए उसके जबड़े को अपने दोनों हाथों से पकड़ कर चीर दिया। तेंदुआ इस प्रहार से घबरा गया और छवि को छोड़कर भाग खड़ा हुआ। छवि तेंदुए के चंगुल से बच गई थी। उसकी गर्दन से खून बह रहा था। उसने अपने पहने हुए कपड़े का एक हिस्सा फाड़कर गर्दन पर बाँधा और घायल हालत में घर पहँची। उसकी बेटी ने गाँव वालों को सारी बात बताई तो गाँव वाले उसे लेकर गाँव के प्राथमिक चिकित्सा केन्द्र ले गए, परन्तु वहाँ इसका इलाज सम्भव न था। गाँव वाले उसे लेकर देहरादून के जिला अस्पताल पहुंचे लेकिन वहाँ के डॉक्टरों ने उसे चण्डीगढ़ पी० जी० आई० ले जाने के लिए कह दिया। अब तक छवि की हालत काफ़ी बिगड़ गई थी। पी० जी० आई० तक पहुँचते-पहुँचते घटना को बीस घण्टे हो चुके थे और छवि की हालत बहुत गम्भीर हो गई थी। पी० जी० आई० के डॉक्टरों ने हिम्मत जुटाते हुए आप्रेशन करके छवि को बचा लिया। तीन हफ्तों के बाद उसे अस्पताल से छुट्टी मिल गई।

छवि अपनी हिम्मत और साहस के बल पर तेंदुए से बच गई। लोगों को जब इस घटना का पता चला तो वे इससे मिलने आने लगे। छवि के मुँह से उसकी वीरता की कहानी सुनकर सभी हैरान हो जाते। सारे गाँव में छवि की बहादुरी का किस्सा सभी की जुबान पर था।

कठिन शब्दों के अर्थ:

कारनामा = काम। नरभक्षी = नर (मनुष्य) को खाने वाला। लड्डू फूटना = बहुत खुश होना। व्याकुल = परेशान। आहट = आवाज़, शोर। वार = हमला। दबोचकर = दबाकर। फूला नहीं समा रहा था = बहुत प्रसन्न हो रहा था। अहसास = मालूम, आभास। कल्पना = सोच। पैरों तले जमीन खिसकना = घबरा जाना। चंगुल = पकड़। अचंभित = हैरान। दिक्कत = परेशानी, कठिनाई। नाजुक = पतली। किस्सा = कहानी, कथा।

Punjab State Board PSEB 5th Class Hindi Book Solutions Hindi Grammar Vyakaran Exercise Questions and Answers, Notes.

PSEB 5th Class Hindi Grammar Vyakaran

प्रश्न 1.
व्याकरण किसे कहते हैं ?
उत्तर:
जिस शास्त्र के द्वारा हमें भाषा के शुद्ध या अशुद्ध होने का ज्ञान प्राप्त हो, उसे व्याकरण कहते हैं।

प्रश्न 2.
हिन्दी व्याकरण के कितने भाग हैं ?
उत्तर:
हिन्दी व्याकरण के तीन भाग हैं-

1. वर्ण विचार।
2. शब्द विचार।
3. वाक्य विचार।

प्रश्न 3.
भाषा किसे कहते हैं? इसके कितने प्रकार हैं ?
उत्तर:
वह साधन जिसके माध्यम से हम अपने मन के विचार दूसरों पर प्रकट करते हैं तथा दूसरों के विचार समझते हैं भाषा कहलाती है। जैसेहिन्दी, पंजाबी, मराठी, बंगला आदि। भाषा के दो प्रकार हैं-
(क) मौखिक
(ख) लिखित।

प्रश्न 4.
लिपि किसे कहते हैं ? हिन्दी की लिपि का नाम लिखो।
उत्तर:
जिन वर्ण चिह्नों के द्वारा भाषा लिखी जाती है, उसे लिपि कहते हैं। हिन्दी भाषा की लिपि का नाम देवनागरी है।

प्रश्न 5.
वर्ण या अक्षर किसे कहते हैं ?
उत्तर:
उस छोटी-से-छोटी ध्वनि को जिसके टुकड़े न हो सकें, वर्ण या अक्षर कहा जाता है।
जैसे-अ, इ, उ, ऋ, क्, च्, ट्, त्, प् आदि।

प्रश्न 6.
हिन्दी वर्णमाला में कितने वर्ण (अक्षर) हैं ?
उत्तर:
हिन्दी वर्णमाला में कुल 44 वर्ण हैं।। इनमें से 11 स्वर और 33 व्यंजन हैं।

प्रश्न 7.
शब्द किसे कहते हैं ? इसके कितने प्रकार हैं ?
उत्तर:
दो या दो से अधिक वर्षों के मेल से बनी सार्थक ध्वनि को शब्द कहते हैं। जैसे-र् + आ, म् + अ = राम।
शब्द दो प्रकार के होते हैं-सार्थक और निरर्थक।

प्रश्न 8.
संज्ञा की परिभाषा लिखो और उसके भेद बताओ।
उत्तर:
किसी विशेष व्यक्ति, वस्तु, स्थान, नगर आदि के नाम को संज्ञा कहते हैं। जैसे-रमेश, सोहन, मेज, कुर्सी, दिल्ली, जालन्धर आदि। संज्ञा के प्रायः तीन भेद माने जाते हैं-

1. व्यक्तिवाचक संज्ञा
2. जातिवाचक संज्ञा
3. भाववाचक संज्ञा।

प्रश्न 9.
सर्वनाम की परिभाषा लिखो। उसके कितने भेद हैं ?
उत्तर:
वाक्य में संज्ञा के स्थान पर प्रयुक्त होने वाले विकारी शब्दों को सर्वनाम कहते हैं। जैसे सोहन, मोहन के साथ उसके घर गया। इस वाक्य में ‘उसके’ सर्वनाम मोहन के स्थान पर प्रयुक्त हुआ है। सर्वनाम के छ: भेद हैं-पुरुषवाचक, निश्चय-वाचक, अनिश्चयवाचक, प्रश्नवाचक, सम्बन्धवाचक और निजवाचक।

प्रश्न 10.
विशेषण किसे कहते हैं और उसके भेद बताओ।
उत्तर:
जो शब्द संज्ञा या सर्वनाम की विशेषता प्रकट करते हैं, उन्हें विशेषण कहा जाता है। जैसेवीर पुरुष। इसमें ‘वीर’ शब्द पुरुष की विशेषता प्रकट करता है। इसलिए यह विशेषण है। विशेषण के भेद

1. गुणवाचक विशेषण
2. परिमाण वाचक विशेषण
3. संख्यावाचक विशेषण
4. सार्वनामिक विशेषण।

प्रश्न 11.
क्रिया किसे कहते हैं? क्रिया के कितने भेद हैं?
उत्तर:
जिन शब्दों के द्वारा किसी काम का करना, होना, सहना आदि पाया जाए, उसे क्रिया कहते हैं। जैसे-मोहन पढ़ता है। कमला लिखती है। क्रिया के भेद-क्रिया के दो भेद माने जाते हैं-

1. अकर्मक क्रिया
2. सकर्मक क्रिया।

प्रश्न 12.
वचन किसे कहते हैं और वचन कितने प्रकार के होते हैं ?
उत्तर:
शब्दों के जिस रूप में किसी वस्तु के एक अथवा अनेक होने का बोध हो, उसे वचन कहते हैं। हिन्दी में दो वचन हैं-

1. एकवचन
2. बहुवचन।

1. एकवचन-संज्ञा का जो रूप एक ही वस्तु का बोध कराए, उसे एकवचन कहते हैं। जैसेलड़की, बहन आदि।
2. बहुवचन-संज्ञा का जो रूप एक से अधिक वस्तुओं का बोध कराए, उसे बहुवचन कहते हैं। जैसे-लड़कियाँ, घोड़े, बहनें आदि।

प्रश्न 13.
कारक किसे कहते हैं ?
उत्तर:
संज्ञा या सर्वनाम के जिस रूप में उस वाक्य का दूसरे शब्दों से सम्बन्ध जाना जाए, उस रूप को कारक कहते हैं। जैसे–मोहन ने पुस्तक को मेज़ पर रख दिया।

प्रश्न 14.
काल किसे कहते हैं और काल के भेद लिखो।
उत्तर:
क्रिया के जिस रूप से उसके होने या करने के समय का बोध हो, उसे काल कहते हैं। काल के मुख्य भेद हैं-

1. भूतकाल-जिससे क्रिया के बीते हुए समय में होने का बोध हो, उसे भूतकाल कहते हैं।
2. वर्तमान काल-जिससे क्रिया के चल रहे अथवा वर्तमान समय में होने का बोध हो, उसे वर्तमान काल कहते हैं।
3. भविष्यत् काल-जिससे क्रिया के आने वाले समय में होने का बोध हो, उसे भविष्यत् काल कहते हैं।

विराम चिह्न

प्रश्न 1.
विराम चिह्न से क्या अभिप्राय है? हिन्दी में प्रचलित विराम चिह्नों को स्पष्ट करें।
उत्तर:
बातचीत करते समय हम अपने भावों को स्पष्ट करने के लिए कहीं-कहीं ठहरते हैं। लिखने में भी ठहराव प्रकट करते हैं। ठहराव को प्रकट करने के लिए जो चिह्न लगाए जाते हैं, वे विराम चिह्न कहलाते हैं।

निर्धारित विराम चिह्न

(क) पूर्ण विराम (।)- वाक्य की पूर्ति की सूचना देने वाले चिह्न को पूर्ण विराम कहते हैं। जैसे-अनुशासनहीनता देश के लिए घातक है।

(ख) अर्द्ध विराम (:)- वाक्य की पूर्ण समाप्ति न होने पर भी जहां बीच में समाप्ति सी लगे। अगले वाक्य से जोड़ने वाले अव्यय का अभाव हो, तब इसका प्रयोग होता है। जैसे-आजकल शिक्षा का उद्देश्य नौकरी है; इसलिए उसका वास्तविक महत्त्व जाता रहा है।

(ग) प्रश्न सूचक (?)- वाक्य को प्रश्नवाचक सूचित करने के लिए इसका प्रयोग होता है। जैसे-क्या मूर्ख को समझाना सरल है?

(घ) विस्मयादि बोधक (!)- मानसिक आवेगों के लिए इसका प्रयोग होता है। जैसे-हाय ! मैं मारा गया। उफ़ ! इतनी पीड़ा।

प्रश्न 2.
निम्नलिखित वाक्यों में विराम चिह्न लगाइए

1. राम पढ़ कर आ गया है
2. सुधा अब तुम उठ जाओ मोहन आने वाला
3. क्या आज वर्षा होगी
4. ओह अब मैं थक गया हूँ
5. जीत ही मेरा लक्ष्य है इसलिए दिन-रात एक कर दिया है
6. मारिया ने कहा अहा कैसा अद्भुत प्रकाश है अन्त में हमने इसे पा ही लिया

उत्तर:

1. राम पढ़ कर आ गया है। (पूर्ण विराम)
2. सुधा अब तुम उठ जाओ; मोहन आने वाला है। (अर्द्ध विराम, पूर्ण विराम)
3. क्या आज वर्षा होगी ? (प्रश्नवाचक)
4. ओह ! अब मैं थक गया हूँ। (विस्मयादि बोधक, पूर्ण विराम)
5. जीत ही मेरा लक्ष्य है; इसलिए दिन-रात एक कर दिया है। (अर्द्ध विराम, पूर्ण विराम)
6. मारिया ने कहा-“अहा ! कैसा अद्भुत प्रकाश है। अन्त में हमने इसे पा ही लिया।”.
   (विस्मयादि बोधक, पूर्ण विराम)

व्यावहारिक व्याकरण चार्ट

चार्ट नं० 1
अशुद्ध-शुद्ध

अशुद्ध – शुद्ध
अनीवार्य – अनिवार्य
सनान – स्नान
अधिआपक – अध्यापक
इश्वर – ईश्वर
आग्या – आज्ञा
दुख – दुःख
बुद्धीमान – बुद्धिमान्
परार्थना – प्रार्थना
दुश्ट – दुश्ट
शरत – शर्त
उदयोग – उद्योग
सबजी – सब्जी
सर्मपित – समर्पित
असमरथ – असमर्थ
चौकनना – चौकन्ना
चुनोती – चुनौती
चकव्यूह – चक्रव्यूह
कृश्ण – कृष्ण
युधिष्ठर – युधिष्ठिर

महातमा – महात्मा
परिक्षा – परीक्षा
प्रतीख्शा – प्रतीक्षा
पंडित – पण्डित
मीठाई – मिठाई
मन्दर – मन्दिर
प्रन्तु – परन्तु
हिरदे – हृदय
शरन – शरण
मिठाईयां – मिठाइयाँ
सुरेन्दर – सुरेन्द्र
अनन्द – आनन्द
जित – जीत
कवि – कवी
पेंसिल – पैंसल
तैयारी – त्यारी
गनेष – गणेश
लक्षमी – लक्ष्मी
प्रतिग्या – प्रतिज्ञा
विग्यान – विज्ञान
रितु – ऋतु
|रिण – ऋण
रिषि – ऋषि
किरपा – कृपा
ऐक – एक
एसा – ऐसा.
म्रित्यु – मृत्यु
पृथ्वी – प्रिथ्वी
इस्री – स्त्री
स्वामि – स्वामी
हिन्दु – हिन्दू
आँसू – आंसु
भुमि – भूमि
बूढ़ा – बूड़ा
प्रशन – प्रश्न
पुन्य – पुण्य
किरन – किरण
पियार – प्यार
दुरदशा – दुर्दशा
धरम – धर्म
हिमांचल – हिमाचल
दृष्टी – दृष्टि
सेनापती – सेनापति
निरभय – निर्भय
अभीवादन – अभिवादन
रूचि – रुचि
लिपी – लिपि
उपस्थति – उपस्थित
गतवय – गंतव्य
आविश्कार – आविष्कार
मनुश्य – मनुष्य
समून्द्र – समुद्र
समीत – समर्पित
सवरगीय – स्वर्गीय
राजिसथान – राजस्थान
वयायाम – व्यायाम
मधया अवकाश – मध्यावकाश
तीतलीयाँ – तितलियाँ
पराचीनकाल – प्राचीनकाल
परतयेक – प्रत्येक
हसपताल – अस्पताल
समजदार – समझदार
मसतिषक – मस्तिष्क
सवासथिय – स्वास्थ्य
पौशटिक – पौष्टिक
परियाप्त – पर्याप्त
परिकर्मा – परिक्रमा
चर्कव्यूह – चक्रव्यूह
मरुसथल – मरुस्थल
उतेजित – उत्तेजित
मनुश्य – मनुष्य
दूरदरशन – दूरदर्शन
श्रीमति – श्रीमती
कलपना – कल्पना
ग्रहकार्य – गृहकार्य
दृष्टीहीन – दृष्टिहीन
परभावी – प्रभावी
विगयापन – विज्ञापन
विशेष – विषेश
उदयोग – उद्योग
गरव – गर्व
द्रिष्टि – दृष्टि
प्रसिद – प्रसिद्ध
रिषि – ऋषि
परबत – पर्वत
निरमल – निर्मल
दुरगम – दुर्गम
विषेश – विशेष
अभिलाशा – अभिलाषा
अर्पन – अर्पण
साइकल – साइकिल
परदूषण – प्रदूषण

चार्ट नं० 2
लिंग परिवर्तन

पुंल्लिग – स्त्रीलिंग
आदमी – औरत
भाई – बहन
पिता – माता
वर – वधू
सेवक – सेविका
ससुर – सास
बुद्धिमान् – बुद्धिमती
साधु – साध्वी
कवि – कवयित्री
चिड़ा – चिड़िया
बैल – गाय
शिष्य – शिष्या.
विधुर – विधवा
नायक – नायिका
बूढ़ा – बुढ़िया
बालक – बालिका
पाठक – पाठिका
अध्यापक – अध्यापिका
बन्दर – बन्दरिया
अभिनेता – अभिनेत्री
चूहा – चुहिया
बछड़ा – बछिया
कुत्ता – कुतिया
भैंसा – भैंस
युवा – युवती
मित्र – मित्रा, सहेली
महाराजा – महारानी
मामा – मामी
लोटा – लुटिया
दादा – दादी
चाचा – चाची
वीर – वीरांगना
सखा – सखी
स्त्री – पुरुष
हाथी – हथिनी
बकरा – बकरी
घोड़ा – घोड़ी
मोर – मोरनी
देव – देवी
नर – नारी
नाना – नानी
विद्वान् – विदुषी
लड़का – लड़की
पुत्र – पुत्री
पुजारी – पुजारिन
शेर – शेरनी
सुनार – सुनारिन
बेटा – बेटी
देवर – देवरानी
जेठ – जेठानी
नौकर – नौकरानी
राजपूत – राजपूतानी
छात्र – छात्रा
श्रीमान् – श्रीमती
दास – दासी
सयाना – सयानी
हिरन – हिरनी
लेखक – लेखिका
बिलाव – बिल्ली
माली – मालिन
राजा – रानी
गायक – गायिका
मछुआरा – मछुआरिन
पति – पत्नी
नौकर – नौकरानी
मुर्गी – मुर्गा
राजकुमार – राजकुमारी

चार्ट नं० 3
वचन बदलो

एकवचन – बहुवचन
बच्चा – बच्चे
लड़का – लड़के
रात – रातें
घोड़ा – घोड़े
कुत्ता – कुत्ते
रस्सा – रस्से
खिलौना – खिलौने
तिनका – तिनके
आँख – आँखें
भैंस – भैंसें
अंगूठा – अंगूठे
कपड़ा – कपड़े
पुस्तक – पुस्तकें
दवात – दवातें
पेंसिल – पेंसिलें
कलम – कलमें
रानी – रानियाँ
मछली – मछलियां
चिड़िया – चिड़ियाँ
डिबिया – डिबियाँ
गुड़िया – गुड़ियाँ
बुढ़िया – बुढ़ियाँ
तिथि – तिथियाँ
नदी – नदियाँ
टोपी – टोपियाँ
लड़की – लड़कियाँ
सखी – सखियाँ
कापी – कांपियाँ
कन्या – कन्याएँ
रानी – रानियाँ
नदी – नदियाँ
बच्चा – बच्चे
कन्या – कन्याएँ
लड़का – लड़के
वह – वे
घर – घरों
स्त्री – स्त्रियाँ
दासी – दासियाँ
औरत – औरतें
सब्जी – सब्ज़ियाँ
माला – मालाएँ
चिड़िया – चिड़ियाँ
वह – वे
दासी – दासियाँ
रस्सी – रस्सियाँ
जिसने – जिन्होंने
कुआँ – कुएँ
दवाई – दवाइयाँ
रचना – रचनाएँ

चार्ट नं० 4
भाववाचक संज्ञाएँ

शब्द – भाववाचक संज्ञा
अच्छा – अच्छाई
अधिक – अधिकता
अनुकूल – अनुकूलता
अपना – अपनत्व
अज्ञान – अज्ञानता
आलसी – आलस्य
आवश्यक – आवश्यकता
ईश्वर – ऐश्वर्य
ऊँचा – ऊँचाई
उदार – उदारता
उचित – औचित्य, उचितता।
उड़ना – उड़ान
एक – एकता
कठिन – कठिनता
कंजूस – कंजूसी
कायर – कायरता
कुशल – कुशलता
क्रूर – क्रूरता
खट्टा – खटाई
खेलना – खेल
खोदना – खुदाई
गहरा – गहराई
गिरना – गिरावट
गरम – गरमी
गुलाम – गुलामी
घबराना – घबराहट
चतुर – चतुराई
चालाक – चालाकी
चौड़ा – चौड़ाई
चढ़ना – चढ़ाई
चलना – चाल
चुनना – चुनाव
झुकना – झुकाव
ठण्डा – ठण्डक
ठगना – ठगी
तेज़ – तेजी
खेलना – खेल
चालाक – चालाकी
पीसना – पिसाई
बूढ़ा – बुढ़ापा
बहना – बहाव
भूखा – भूख
मित्र – मित्रता
मनुष्य – मनुष्यता.
मीठा – मिठास
वीर – वीरता
लड़ना – लड़ाई
स्वतन्त्र – स्वतन्त्रता
सरल – सरलता
बच्चा – बचपन
शीघ्र – शीघ्रता
मोटा – मोटापा
प्यासा – प्यास
दुर्बल – दुर्बलता
लम्बा – लम्बाई
हँसना – हँसी
पशु – पशुता
बुद्धिमान् – बुद्धिमत्ता

चार्ट नं० 5
विशेषण रचना

शब्द – भाववाचक संज्ञा
अर्थ – आर्थिक
अपमान – अपमानित
अभिमान – अभिमानी
अवश्य – आवश्यक
आदर – आदरणीय
आकर्षण – आकर्षक
इच्छा – इच्छुक
इतिहास – ऐतिहासिक
ईर्ष्या – ईर्ष्यालु
ईश्वर – ईश्वरीय
कपट – कपटी
कल्पना – काल्पनिक
केन्द्र – केन्द्रीय
कांटा – कंटीला
कुल – कुलीन
क्रोध – क्रोधी
कृपा – कृपालु
उद्योग – औद्योगिक
खोज – खोजी
खेलना – खिलाड़ी
ग्राम – ग्रामीण
गुण – गुणी, गुणवान्
गाना – गवैया
घर – घरेलू
घृणा – घृणित
चमक – चमकीला
जंगल – जंगली
जोश – जोशीला
झूठ – झूठी
झगड़ा – झगड़ालू
तेज – तेजस्वी
तप – तपस्वी
दया – दयालु
डर – डरावना
दान – दानी
दिन – दैनिक
दुध – दुधारू
देश – देशीय
धन – धनी
नमक – नमकीन
नशा – नशीला
बर्फ – बर्फीला
भार – भारी
रंग – रंगीन
मुख – मौखिक
विज्ञान – वैज्ञानिक
साहस – साहसिक
नगर – नागरिक
मास – मासिक
वर्ष – वार्षिक
मन – मनस्वी
तप – तपस्वी
विदेश – विदेशी
शरीर – शारीरिक
समय – सामयिक
स्वदेश – स्वदेशी
समाज – सामाजिक
हृदय – हार्दिक
पाप – पापी
नगर – नागरिक

चार्ट नं० 6
विपरीतार्थक या विलोम शब्द

शब्द – विपरीतार्थक
अर्थ – अनर्थ
अन्त – आरम्भ
अपना – पराया
अच्छा – बुरा
अधिक – कम
पूर्ण – अपूर्ण
अनुकूल – प्रतिकूल
अमृत – विष
असाध्य – साध्य
आदर – अनादर
आदि – अन्त
आय – व्यय
आदान – प्रदान
आकाश – पाताल
आना – जाना
आस्तिक – नास्तिक
आज्ञा – अवज्ञा
आलसी – उद्यमी
आशा – निराशा
इच्छा – अनिच्छा
ईश – अनीश
उदार – कृपण
उदय – अस्त
उधार – नकद
उन्नति – अवनति
उत्तर – प्रश्न
उठना – बैठना
उपकार – अपकार
उत्थान – पतन
उतार – चढ़ाव
उचित – अनुचित
उपस्थित – अनुपस्थित
उपयोगी – अनुपयोगी
एक – अनेक
कठिन – सरल
काला – सफेद
कायर – वीर
कोमल – कठोर
क्रोध – शान्ति
क्रय – विक्रय
कुरूप – सुरूप
खुशबू – बदबू
खूबसूरत – बदसूरत
गर्म – ठण्डा
गर्मी – सर्दी
गीला – सूखा
घर – बाहर
चालाक – सीधा
चतुर – मूर्ख
चढ़ना – उतरना
हार – जीत
जय – पराजय
जागरण – सुप्त
जागना – सोना
दृश्य – अदृश्य
दूषित – शुद्ध
दयालु – निर्दयी
दुश्मन – दोस्त
दुःख – सुख
दूर – पास
धर्म – अधर्म
पवित्र – अपवित्र
अंधेरा – उजाला
पतित – पवित्र
भयभीत – निर्भय
मित्र – शत्रु
मृत्यु – जीवन
मरण – जन्म
मूक – वाचाल
मूर्ख – बुद्धिमान
मूल्य – अमूल्य
मूल्यवान् – अमोल
मान – अपमान
मीठा – कड़वा
योग्य – अयोग्य
रात – दिन
राजा – रंक
राग – विराग
लाभ – हानि
लाभकारी – हानिकारक
लोभ – निर्लोभ
विजय – पराजय
विपरीत – समान
विशाल – लघु
व्यवस्था – अव्यवस्था
शीतल – गर्म
शान्त – अशान्त
समर्थ – असमर्थ
सजीव – निर्जीव
सरल – कठिन
सफल – असफल
समान – असमान
सुपूत – कपूत
सन्तुलित – असन्तुलित
साधारण – असाधारण
साक्षर – निरक्षर
सुर – असुर
सुविधा – असुविधा
सुस्त – चुस्त
सुगन्धि – दुर्गन्ध
श्याम – शुक्ल
श्याम – श्वेत
सुन्दर – कुरूप
हिंसक – अहिंसक

चार्ट नं० 7
पर्यायवाची या समानार्थक शब्द

अमृत – सोम, सुधा, पीयूष।
असुर – राक्षस, दैत्य, दानव, दनुज।
अग्नि – आग, अनल, पावक, दहन।
अन्धकार – अन्धेरा, तम, तिमिर।
आँख – नेत्र, चक्षु, नयन, लोचन।
आनन्द – हर्ष, खुशी, प्रसन्नता, मोद।
आकाश – नभ, अम्बर, आसमान, गगन।
इच्छा – अभिलाषा, कामना, लालसा।
ईश्वर – प्रभु, भगवान्, परमात्मा, जगदीश।
कपड़ा – वस्त्र, पट, वसन।
कमल – पंकज, सरोज, अरविन्द।
किनारा – तट, कूल, कगार, तीर।
गो – गाय, सुरभि, धेनु।
घर – गृह, सदन, भवन।
घोड़ा – अश्व, बाजी, घोटक, तुरंग।
चन्द्रमा – चाँद, इन्द, राकेश, शशि, चन्द्र।
जल – वारि, पानी, नीर, पय।
तलवार – खड्ग, कृपाण, असि।
तीर – बाण, शर, सायक।
दिन – दिवस, वार, अहन।
देवता – सुर, देव, अमर।
नदी – सरिता, तरंगिणी, नद, तटिनी।
नमस्कार – प्रणाम, नमस्ते, अभिवादन।
पृथ्वी – ज़मीन, धरती, भूमि।
पुत्र – बेटा, सुत, तनय।
पर्वत – गिरि, पहाड़, अचल, शैल।
पक्षी – खग, नभचर, विहग।
बादल – मेघ, घन, जलद, नीरद।
बिजली – विद्युत्, तड़ित, दामिनी।
फूल – सुमन, कुसुम, पुष्प।
माता – जननी, माँ, मैया।
राजा – नृप, नरेश, भूपति, भूप।
वायु – हवा, मारुत, पवन, अनिल।
रात – रात्रि, निशा, रजनी।
संसार – जग, जगत, दुनिया।
सूर्य – रवि, भानु, सूरज, दिनकर।
सरोवर – तालाब, तड़ाग, सर।
समुद्र – सिन्धु, सागर, उद्धि, जलधि।
शत्रु – वैरी, रिपु, अरि, दुश्मन।
डाली – शाखा, लता, टहनी।

चार्ट नं० 8
अनेक शब्दों के लिए एक शब्द

जो पढ़ा लिखा न हो – अशिक्षित, अनपढ़
जिसका आदि न हो – अनादि
जिसमें धैर्य न हो – अधीर
जो कुछ भी न जानता हो – अज्ञ
जो गिना न जा सके – अगणित, अगण्य
जहां कोई न जा सके – अगम्य, अगम
जहां अनाथ रहते हों – अनाथालय
जिसका कोई शत्रु पैदा न हुआ हो – अजातशत्रु
जो परीक्षा में पास न हो – अनुत्तीर्ण
जो थोड़ा जानता हो – अल्पज्ञ
जो बिना वेतन के काम करे – अवैतनिक
जिसका अन्त न हो – अनन्त
जो कभी बूढ़ा न हो – अजर
जो कभी न मरे – अमर
जिसे भेदा न जा सके – अभेध
जिसको कोई जीत न सके – अजेय
जिस पर विश्वास न किया जा सके। – अविश्वसनीय
जिसका कोई इलाज न हो सके – असाध्य
जिसका कोई मूल्य न हो – अमूल्य
जिसका कोई पार न हो – अपार
जो ईश्वर को मानता हो – आस्तिक
आलोचना करने वाला – आलोचक
अत्याचार करने वाला – अत्याचारी
जिसकी तुलना न हो – अतुलनीय
अन्याय करने वाला – अन्यायी
गाँव में रहने वाला – ग्रामीण
शहर में रहने वाला – शहरी
जिसका आकार न हो – निराकार
जिसका आकार हो – साकार
जिसमें बल न हो – निर्बल
दूसरों पर दया करने वाला – दयालु
दूसरों का उपकार करने वाला – परोपकारी
सदा सच बोलने वाला – सत्यवादी
साथ-साथ पढ़ने वाला – सहपाठी
साथ-साथ चलने वाला – सहचर
किए हुए उपकार को मानने वाला – कृतज्ञ
किए हुए उपकार को न मानने वाला – कृतघ्न
ईश्वर को न मानने वाला – नास्तिक
जो सब कुछ जानता हो – सर्वज्ञ
जो बहुत कम जानता हो – अल्पज्ञ
जो दिखाई न दे – अदृश्य

जो पत्र-पत्रिकाओं का सम्पादन करता हो – सम्पादक
जहाँ पोस्ट कार्ड लिफाफे आदि मिलते हैं – डाकघर
जो पाठशाला में पढ़ाए – अध्यापक
जो नई-नई बातों की खोज करे – शोधकर्ता
खेती सम्बन्धी पढ़ाई – कृषि शिक्षा
रेलगाड़ियों के रुकने का स्थान – रेलवे-स्टेशन
घोड़ों के बाँधने का स्थान – अस्तबल
जिस लिपि द्वारा अंधे पढ़ते-लिखते हैं – ब्रेल-लिपि
संगीत को जानने वाला – संगीतज्ञ
जहाँ बीमारों का इलाज होता है – अस्पताल
जिसका कोई पार न हो – अपार
जहाँ प्रयोग किए जाते हैं – प्रयोगशाला
जिसको दिखाई न देता हो – अन्धा
जहाँ पत्र-पत्रिकाएँ तथा पुस्तकें पढ़ने को मिलें – पुस्तकालय
जिसमें दया न हो – निर्दय
नीचे लिखा हुआ – निम्नलिखित
जहाँ विद्यार्थी पढ़ते हैं – विद्यालय
समाचार इकट्ठा करने वाला – संवाददाता
हाथी हाँकने वाला – महावत
टाइप पर प्राप्त समाचार – फैक्स
रिपोर्ट तैयार करने वाला – रिपोर्टर
हिंसा करने वाला – हिंसक
पहाड़ के आसपास का स्थान जहाँ नमी हो – तलहटी
सम्पादक की ओर से – सम्पादकीय
लम्बी आयु वाला – दीर्घायु
जिसकी आत्मा महान हो – महात्मा
दूर की बात सोचने वाला – दूरदर्शी
तेज़ गति से चलने वाला – तीव्रगामी
संदेश ले जाने वाला – संदेशवाहक
साथ पढ़ने वाला – सहपाठी

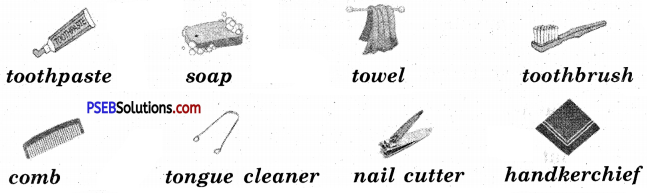
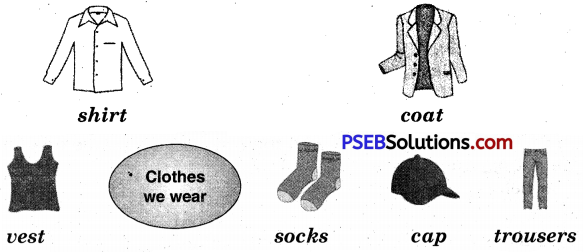
Punjab State Board PSEB 5th Class Hindi Book Solutions Hindi Rachana Nibandh Lekhan निबंध-लेखन Exercise Questions and Answers, Notes.

PSEB 5th Class Hindi Rachana निबंध-लेखन

1. मेरा मित्र

राम मेरा पक्का मित्र है। वह पाँचवीं कक्षा में पढ़ता है। उसकी आयु 12 वर्ष है। वह बड़ा स्वस्थ और सुन्दर है। उसका कद लम्बा और बाल काले घने हैं। यह सदा साफ़-सुथरे वस्त्र पहनता है। वह बड़ा परिश्रमी है। उसके पिता जी एक वकील हैं। उसकी माता जी एक स्कूल में पढ़ाती हैं। राम बहुत होनहार लड़का है। वह परीक्षा में सदा प्रथम रहता है। वह कमज़ोर लड़कों की सहायता करता है। वह सदा समय पर स्कूल जाता है। वह सदा सच बोलता है। सभी अध्यापक उससे प्यार करते हैं। भगवान् करे, उसकी आयु लम्बी हो।

2. मेरा प्रिय अध्यापक

मेरे स्कूल में दस अध्यापक हैं। मैं श्री राम लाल को सबसे अधिक पसन्द करता हूँ। उनकी आयु 30 वर्ष की है। वे सुन्दर और स्वस्थ हैं। वे हमें हिन्दी पढ़ाते हैं। उनके पढ़ाने का ढंग बहुत अच्छा है। वे बड़े दयालु हैं। वे कमज़ोर लड़कों की हमेशा सहायता करते हैं। वे सदा साफ़ कपड़े पहनते हैं। वे एक अच्छे कवि हैं। वे सदा समय पर स्कूल आते हैं। वे अपने से बड़ों का सम्मान करते हैं। स्कूल के सभी बच्चे उन्हें बहुत प्यार करते हैं। हमें उन पर बड़ा गर्व है।

3. मेरी मुख्याध्यापिका

श्रीमती अजीत कौर मेरी मुख्याध्यापिका है। उनकी आयु 40 वर्ष की है। वे स्वस्थ और सुन्दर हैं। उनका कद लम्बा और बाल काले और लम्बे हैं। वे पाँचवीं श्रेणी को पढ़ाती हैं। वे बड़े सरल ढंग से पढाती हैं।। हमें उनकी हर एक बात समझ में आती है। वे | प्रातःकाल समय पर स्कूल में आती हैं और स्कूल | का चक्कर लगाती हैं। वे अध्यापिकाओं से बड़ा अच्छा व्यवहार करती हैं। वे बच्चों से बड़ा प्यार करती हैं। वे बच्चों के माता-पिता को भी समयसमय पर स्कूल बुलाती हैं। हमें उन पर बड़ा गर्व है।

4. मेरा स्कूल
अथवा
मेरा विद्यालय

मेरे स्कूल (विद्यालय) का नाम ‘सरकारी हाई स्कूल’ है। यह रेलवे स्टेशन के समीप ही स्थित है। इसमें दस कमरे हैं। हर कमरे में बिजली का पंखा लगा हुआ है। सभी कमरे बहुत अच्छे सजे हुए हैं। | मेरे स्कूल में छोटा-सा बाग है। इसमें सुन्दर फूल | खिले हुए हैं। स्कूल में एक खेल का मैदान भी है। हम आधी छुट्टी के समय यहाँ खेलते हैं। स्कूल में सभी अध्यापक अच्छे हैं। उनका पढ़ाने का ढंग बड़ा सरल है। इसलिए हमारे स्कूल के परिणाम सदा अच्छे रहते हैं। सच पूछो तो हमारा स्कूल हमारे नगर का सबसे अच्छा स्कूल है। मुझे अपने स्कूल पर गर्व है।

5. अच्छा विद्यार्थी

विद्या प्राप्त करने वाला विद्यार्थी कहलाता है। अच्छे विद्यार्थी को अपने गुरुओं का आदर करना चाहिए। उसको स्वस्थ होना चाहिए। उसे खूब ज्ञान प्राप्त करना चाहिए। उसे सादा जीवन बिताना चाहिए। उसे सदा अपने माता-पिता की आज्ञा माननी चाहिए। उसे सदा सच बोलना चाहिए। उसे सदा समय पर स्कूल जाना चाहिए। उसे मन लगाकर पढ़ना चाहिए। उसे खेलों में भी भाग लेना चाहिए। उसे सदा अच्छी बातें सोचनी चाहिएं। उसे बुरे लोगों से दूर रहना चाहिए।

6. गाय

गाय एक पालतू पशु है। उसकी चार टांगें, दो आँखें, दो कान और एक लम्बी पूँछ होती है। इसके दो सींग भी होते हैं। गाय हरी घास और भूसा खाती है। यह हमें दूध देती है। इसका दूध मीठा और स्वास्थ्य को बढ़ाने वाला होता है। इसके दूध से दही, लस्सी, मक्खन और घी बनता है। इसका दूध बच्चों, बूढ़ों और बीमार लोगों के लिए बहुत लाभदायक है। इसके गोबर से खाद तथा उपले बनते हैं। भारत के लोग गाय को गौ माता कहते हैं। हिन्दू इसकी पूजा करते हैं।

7. दशहरा

दशहरा भारत का प्रसिद्ध त्योहार है। यह दीवाली से 20 दिन पहले मनाया जाता है। 9 दिन तक रामलीला होती है और दसवें दिन दशहरे का त्योहार मनाया जाता है। इस दिन श्री रामचन्द्र जी ने रावण पर विजय पाई थी। इसलिए दशहरे को ‘विजयदशमी’ भी कहते हैं। दशहरे के दिन दुकानदार अपनी दुकानें सजाते हैं। इस दिन बाजारों में बड़ी भीड़ होती है। शाम के समय नगर के बाहर भारी मेला लगता है। सूर्य डूबने से कुछ समय पहले रावण, कुम्भकर्ण और मेघनाद के पुतलों को जलाया जाता है। लोग मेले की दुकानों से मिठाइयां तथा खिलौने खरीदते हैं और खुशी-खुशी घरों को लौट आते हैं।

8. दिवाली

दिवाली भारत का प्रसिद्ध त्योहार है। यह त्योहार दशहरे के बीस दिन बाद आता है। यह त्योहार श्री रामचन्द्र जी के अयोध्या लौटने की खुशी में मनाया जाता है। इसी दिन सिखों के छठे गुरु श्री गुरु हरिगोबिंद जी जहांगीर की नज़रबन्दी से आज़ाद होकर आए थे। पंजाब में अमृतसर की दिवाली देखने योग्य होती है। दिवाली के कुछ दिन पहले दुकानों तथा घरों में सफ़ेदी की जाती है। दिवाली के दिन दुकानदार दुकानें सजाते हैं। बाजारों में बड़ी में जाते हैं। वे मिठाइयां, पटाखे और खिलौने खरीदते हैं। रात के समय लक्ष्मी की पूजा की जाती है। सभी लोग अपने-अपने घरों में दीये जलाते हैं। बच्चे रात को पटाखे चलाते हैं।

9. मेरा प्रिय त्योहार

लोहड़ी पंजाब का एक प्रसिद्ध त्योहार है। यह जनवरी के महीने में आता है। त्योहार के दिन बच्चे प्रात:काल ही टोलियां बनाकर निकल पड़ते हैं। वे घर-घर जाकर लोहड़ी मांगते हैं और लोहडी के। गीत गाते हैं। लोग उन्हें रेवड़ियां, गुड़ तथा मक्की के भुने हुए दाने देते हैं। जिस घर में कोई विवाह हुआ हो या किसी बच्चे का जन्म हुआ हो, वहाँ से उन्हें और भी अधिक चीजें मिलती हैं। कुछ लोग उन्हें लकड़ियां और उपले भी देते हैं। रात के समय इनका एक ढेर लगाकर आग लगाई जाती है। लोग आग में चिड़वे और रेवड़ियों की. आहुति डालते हैं। काफ़ी 1 देर तक लोग आपस में बैठकर बातें करते हैं। इस प्रकार यह त्योहार खुशी के साथ-साथ भाईचारे का सन्देश भी देता है।

10. वैशाखी का त्योहार

वैशाखी पंजाब का एक प्रसिद्ध त्योहार है। पंजाब में यह त्योहार बड़ी धूमधाम से मनाया जाता है। यह कई कारणों से मनाया जाता है। इस समय तक किसानों की फसल पक जाती है और वे खुशी से
झूम उठते हैं। इसी दिन सन् 1699 ई० में गुरु गोबिन्द सिंह जी ने खालसा पंथ की नींव भी रखी थी। वैशाखी के दिन कई स्थानों पर मेले लगते हैं। इनमें खेलों के मुकाबले होते हैं।

11. व्यायाम
साधारण शब्दों में व्यायाम कसरत करने का नाम है। व्यायाम हमारे शरीर के लिए बहुत ज़रूरी है। शरीर में फुर्ती आती है। व्यायाम करने से हम स्वस्थ रहते हैं। व्यायाम करने वालों को बुढ़ापा जल्दी नहीं आता। शरीर सुन्दर और मजबूत बनता है। व्यायाम से खून का संचार बढ़ता है। स्त्रियों और पुरुषों के लिए अलग-अलग प्रकार के व्यायाम हैं। व्यायाम करने से काम में रुचि बढ़ती है और मन प्रसन्न रहता है। इसलिए शरीर को स्वस्थ रखने के लिए प्रतिदिन व्यायाम करना चाहिए क्योंकि अंग्रेजी की एक कहावत है कि स्वस्थ शरीर में ही स्वस्थ मन रहता है।

12. मेरा गाँव

भारत गाँवों का देश है। इसकी 80% जनता गाँवों में रहती है। मेरे गाँव का नाम ……… है। इसमें दो सौ के लगभग मकान हैं। अधिकतर मकान पक्के हैं, परन्तु कुछ मकान कच्चे भी हैं। गाँव की गलियां कच्ची हैं परन्तु गलियों में पक्की नालियां बनी हुई हैं। गाँव में एक छोटा-सा बाजार है। गाँव के बाहर एक डाकखाना है। इसमें एक डिस्पैंसरी भी है। यहाँ रोगियों को मुफ्त दवा दी जाती है। गाँव में एक पंचायत घर भी है। इसके चारों ओर हरे-भरे खेत हैं। मेरे गाँव के निवासी बहुत अच्छे हैं। वे कभी एक दूसरे से नहीं लड़ते। मुझे अपने गाँव पर गर्व है। मेरा गाँव एक आदर्श गाँव है।

13. मेरा देश

भारत मेरा प्यारा देश,
सब देशों से न्यारा देश।

मेरे देश का नाम भारत है। यह एक विशाल देश है। इसकी आबादी 125 करोड़ के लगभग है। यह 28 राज्यों पर आधारित है। यह तीनों ओर से समुद्र से घिरा हुआ है। इसके उत्तर में हिमालय पर्वत है। मेरे देश की धरती बड़ी उपजाऊ है। इस देश में गंगा-यमुना जैसी महान् नदियां बहती हैं। यहाँ की धरती में खनिज पदार्थों के भण्डार भी छिपे पड़े हैं। इस देश में लगभग सभी धर्मों के लोग रहते हैं। अब भारत प्रगति के मार्ग पर अग्रसर है। यहाँ अनेक उद्योग-धन्धे पनप रहे हैं। मुझे अपने देश पर गर्व है क्योंकि “सारे जहाँ से अच्छा हिन्दोस्तां हमारा।”

14. स्वतन्त्रता दिवस

हमारा देश 15 अगस्त, 1947 को स्वतन्त्र हुआ था। इसलिए हम हर साल 15 अगस्त को स्वतन्त्रता दिवस मनाते हैं। यह दिन भारत में बड़ी धूम-धाम से मनाया जाता है। इस दिन लाल किले पर तिरंगा झण्डा फहराया जाता है। इसके बाद प्रधानमन्त्री भाषण देते हैं। इस दिन स्थान-स्थान पर खेल, नाटक, कवि दरबार, नाच-गाने आदि होते हैं। रात को दीपमाला होती है। यह दिन हमें याद दिलाता है कि हमें | आज़ादी की हर तरह से रक्षा करनी चाहिए।

15. छब्बीस जनवरी

26 जनवरी हमारा गणतन्त्र दिवस है। हम हर वर्ष इसे बड़ी धूमधाम से मनाते हैं। गणतन्त्र दिवस का मुख्य समारोह दिल्ली में होता है। इस वर्ष 26 जनवरी को हमारे स्कूल में गणतन्त्र दिवस मनाया गया। स्कूल को अच्छी तरह सजाया गया। समारोह में मुख्य अतिथि के लिए एस० डी० एम० को बुलावा दिया गया। वे प्रातः नौ बजे स्कूल पहुंचे। द्वार पर अध्यापकगण ने उनका स्वागत किया। स्कूल के बैंड ने स्वागती धुन बजाई। वे मंच पर पहुँचे और उन्होंने राष्ट्रीय झण्डे को फहराया। सभी ने मिलकर राष्ट्रीय गान गाया। तब समारोह आरम्भ हुआ। कई प्रकार के खेल और नाटक प्रस्तुत किए गए। कुछ विद्यार्थियों ने देश प्यार के गीत और कविताएं गाईं। विजेताओं को इनाम दिए गए। बारह बजे समारोह समाप्त हो गया। हम सभी खुशी-खुशी घर लौट आए।

16. श्री गुरु नानक देव जी

श्री गुरु नानक देव जी सिक्खों के पहले गुरु थे। उनका जन्म तलवण्डी गाँव में हुआ। इस स्थान को आजकल ननकाना साहिब कहते हैं। अब यह पाकिस्तान में है। उनके पिता जी का नाम मेहता कालू राम जी और माता जी का नाम तृप्ता जी था। बचपन से उनका ध्यान ईश्वर की भक्ति करने की ओर था। उन्होंने अपना सारा जीवन लोगों की भलाई में लगा दिया। उन्होंने स्थान-स्थान पर घूम कर लोगों को अपना उपदेश दिया। उन्होंने लोगों को बताया कि ईश्वर एक है और हम सब भाई-भाई हैं। उन्होंने लोगों को नेक कमाई करने और सत्यनाम का जाप करने की शिक्षा दी।

17. अमृतसर की सैर

अमृतसर एक सुन्दर नगर है। यह हमारा पवित्र तीर्थ स्थान है। इसे गुरु की नगरी भी कहते हैं। गत रविवार मैं अपने पिता जी के साथ अमृतसर गया। सबसे पहले हम दरबार साहिब देखने गए। हमने बाबा अटल का ऊँचा बुर्ज तथा कई अन्य भवन देखे। इसके बाद हमने जलियांवाला बाग देखा। यहां सन् 1919 ई० में अनेक लोग शहीदी को प्राप्त हुए थे। वहां से हम दुर्गियाना मन्दिर देखने गए। यह मन्दिर दुर्गा देवी के नाम पर बना है। हमने कम्पनी बाग भी देखा। अमृतसर नगर शिक्षा तथा व्यापार का केन्द्र है। हमने अनेक बाज़ार तथा गलियां देखीं। शाम को हम माल रोड की सैर को निकले। सच तो यह है कि अमृतसर की सैर से मन मुग्ध हो गया।

18. श्री गुरु गोबिन्द सिंह जी

श्री गरु गोबिन्द सिंह जी सिक्खों के दसवें गुरु थे। उनका जन्म पटना में सन् 1666 ई० में हुआ। उनको अच्छी शिक्षा दी गई। उन्हें घुड़सवारी, तीर तथा तलवार चलाना भी सिखाया गया। उनकी आयु अभी 9 वर्ष की थी कि उनके पिता जी को शहीद कर दिया गया। पिता जी की शहीदी के बाद वह सिक्खों के गुरु बने। उन्होंने सन् 1699 ई० में खालसा पंथ की स्थापना की और सिक्खों को नाम के आगे ‘सिंह’ शब्द लगाने का आदेश दिया। गुरु जी ने देश की जनता की रक्षा की। वह ग़रीबों का सहारा बने। उन्होंने ऊँच-नीच का भेदभाव मिटाया। उन्होंने धर्म की रक्षा के लिए अपने चारों पुत्रों का बलिदान दे दिया। वे जीवन भर लड़ते रहे। उन्होंने अन्याय के सामने कभी शीश नहीं झुकाया। इतिहास में उनका नाम सदा अमर रहेगा।

19. समाचार-पत्र

समाचार-पत्र के द्वारा हम घर पर बैठे ही देशविदेश के समाचारों को जान लेते हैं। संसार के किसी कोने में घटने वाली घटना तार, टेलीफोन अथवा टेलीप्रिंटर के द्वारा समाचार-पत्रों के कार्यालयों में पहुँच जाती है।

समाचार-पत्रों से हमें अनेक लाभ हैं। नगर, प्रान्त, देश तथा विदेश आदि के समाचारों को हम समाचारपत्र द्वारा घर बैठे जान लेते हैं। इससे हमारे ज्ञान में भी वृद्धि होती है। समय-समय पर इनमें अनेक प्रकार के चित्र भी छपते रहते हैं। इन चित्रों के द्वारा जहाँ हमारा मनोरंजन होता है, वहाँ इनसे अनेक प्रकार के ऐतिहासिक, धार्मिक, प्राकृतिक स्थानों की भी जानकारी होती है।

समाचार-पत्रों में कहानियां, कविताएँ, जीवनियां तथा हास्य की सामग्री भी छपती रहती है। इन्हें पढ़कर हमारा मनोरंजन होता है। समाचार-पत्र सब के लिए उपयोगी हैं।

20. हॉकी का मैच

रविवार का दिन था। मैं अपने भाई के स्कूल में गया। वहाँ हॉकी का मैच हो रहा था। एक ओर जैन स्कूल की तथा दूसरी ओर हीरो स्कूल की टीम थी। जैन स्कूल के खिलाड़ी बड़ा अच्छा खेल रहे थे। उनका कप्तान बाल लेकर दौड़ा और उसने गोल कर दिया। शीघ्र ही मध्यान्तर (आधा समय) हो गया।

मध्यान्तर के बाद मैच फिर शुरू हुआ। हीरो स्कूल के लड़के जोश में आ गए। वे एक होकर खेलने लगे। शीघ्र ही उन्होंने गोल कर दिया। मुकाबला अन्त तक होता रहा। कोई भी टीम और गोल न कर सकी। अन्त में मैच बराबर रहा।

21. मेरा प्रिय नेता
अथवा
महात्मा गांधी

महात्मा गांधी भारत के महान् नेताओं में से थे। उन्होंने अहिंसा और सत्याग्रह के प्रयोग से अंग्रेज़ों को भारत छोड़ने पर विवश कर दिया। दुनिया के इतिहास में उनका नाम हमेशा अमर रहेगा। गाँधी जी मेरे प्रिय नेता थे।

इस महापुरुष का जन्म 2 अक्तूबर सन् 1869 को गुजरात काठियावाड़ के पोरबन्दर स्थान पर हुआ। आप मोहनदास कर्मचन्द गाँधी के नाम से प्रख्यात हुए। आपके पिता राजकोट राज्य में दीवान थे। माता पुतलीबाई बहत धार्मिक प्रवृत्ति की महिला थी। गाँधी जी को उच्च आदर्शों और संस्कारों की शिक्षा इनकी माता जी से ही मिली।

महात्मा गांधी ने उच्च शिक्षा प्राप्त कर भारत की आज़ादी के लिए संघर्ष शुरू कर दिया। उन्होंने अहिंसा के शस्त्र से भारत में अंग्रेज़ शासन का अन्त कर दिया। जिसके परिणामस्वरूप 15 अगस्त, सन् 1947 को भारत आज़ाद हुआ।

स्वतन्त्रता का पुजारी बापू गाँधी 30 जनवरी, सन् 1948 को एक मनचले नौजवान नाथूराम गोडसे की गोली का शिकार हुआ। अहिंसा का सबसे बड़ा उपासक हिंसा की भेंट चढ़ गया। देशवासी गांधी जी को सदा स्मरण रखेंगे।

22. प्रातःकाल की सैर

प्रात:काल का समय बड़ा सुहावना होता है। इस समय ताज़गी छाई हुई होती है। प्रात:काल का वातावरण मन को अतीव प्रसन्नता देने वाला तथा स्फूर्तिदायक होता है। प्रकृति इस समय अपना अनुपम सौन्दर्य प्रदर्शित करती है। सुबह सवेरे नर्म-नर्म बिस्तर को छोड़कर जो व्यक्ति भ्रमण का आनन्द लूटता है, प्रकृति उसे धनवान, बुद्धिमान् और स्वस्थ होने का वरदान देती है।

गर्मियों में साढ़े चार या पाँच तथा सर्दियों में साढ़े पाँच-छ: बजे का समय भ्रमण के लिए उपयुक्त समय है। बिस्तर छोड़ने में थोड़ा-सा कष्ट अवश्य अनुभव होता है लेकिन जब हम उठ बैठते हैं तो सारा आलस्य भी समाप्त हो जाता है। प्रात:काल की सैर स्वास्थ्य के लिए बहुत ही श्रेष्ठ है। इससे व्यक्ति नीरोग रहता है। चेहरा खिला रहता है। पढ़ने में दिल लगता है। प्रात: की सैर सबके लिए अच्छा व्यायाम है।

23. बसन्त ऋतु बसन्त ऋतु का आगमन चैत्र तथा वैशाख के महीनों में होता है। इन्हीं महीनों में न ठिठुराने वाला जाड़ा होता है, न भयंकर झुलसाने वाली लू चलती हैं, न वर्षा होती है। खुले दिन होते हैं। सभी बेलों तथा वृक्षों पर फूल-पत्ते आ जाते हैं। बागों में नई बहार आ जाती है। आम, अनार, अमरूद आदि वृक्षों पर बौर आ जाता है। कोयल पंचम स्वर में गाने लगती है।

खेतों में वसन्ती रंग के सरसों के फूल तथा नीले रंग के अलसी के फूल सबके मन को मोह लेते हैं। अत: लोग बसन्त की बहार को देखने के लिए घरों से निकल पड़ते हैं तथा इसका सुहावना आनन्द लेते हैं। शीत ऋतु का जमा हुआ रक्त पिघल कर शरीर के प्रत्येक अंग में पहुँचता है।

इस ऋतु का बड़ा महत्त्व यह भी है कि इस ऋत के आरम्भ में अपने धर्म पर बलिदान होने वाले हकीकत राय को धर्मान्ध काज़ियों ने मरवा दिया था। वीर हकीकत की याद में यह त्योहार धूमधाम से मनाया जाता है। वसन्त ऋतु मुझे बहुत प्रिय लगती है।

24. रंगों का पर्व : होली

भारत त्योहारों का देश है। इस देश में प्रायः प्रत्येक महीने में कोई न कोई त्योहार अवश्य आता है। रंगों का त्योहार होली का आगमन मार्च के महीने में होता है। इस पर्व में चारों ओर रंगों की बहार आ जाती है। कहीं अबीर उड़े तो कहीं गुलाल चले, कहीं पिचकारी है तो कहीं रंगों में रंगे लोग मस्ती में गा रहे हैं। चारों ओर प्रसन्नता और उल्लासता छा जाती है।

होली का आरम्भ कब हुआ इसके सम्बन्ध में कथा प्रचलित है कि नास्तिक हिरण्यकश्यपु राजा ने अपने पुत्र प्रह्लाद को जला देने की सोची। हिरण्यकश्यपु की बहन होलिका जिसे यह वरदान मिला था कि आग में जल नहीं सकती, प्रह्लाद को गोद में लेकर आग में जा बैठी। प्रह्लाद तो बच गया पर होलिका जल गई। तभी से यह उत्सव मनाया जाता है। इस दिन लोग सुबह से ही मित्रों की टोलियां बनाकर अपने मित्रों को रंगने के लिए घरों से निकल पड़ते हैं। चारों ओर होली है-होली है का शोर सुनाई देता है। ब्रज और मथुरा की होली का तो रूप ही और होता है। रंगों से रंगे हुए, गाते बजाते, नाचते हुए मस्ती से भरे लोग सब तरफ दिखाई पड़ते हैं। यह त्योहार परस्पर द्वेष, वैर भाव भुलाकर प्रेमपूर्वक मिलकर रहने का सन्देश देता है।

25. मेरा घर

मेरा घर शहर के एक साफ़-सुथरे इलाके में स्थित है। यह शहर के बीचों-बीच स्थित है। मेरे घर में मेरे साथ-साथ मेरी माता जी, पिता जी तथा मेरा छोटा भाई भी रहते हैं। मेरे घर में छ: कमरे हैं। सभी सदस्यों के लिए अलग-अलग कमरे हैं। मेरा भी अपना एक अलग कमरा है। मेरा घर दो मंजिला है। मेरा कमरा दूसरी मंजिल पर है। यहाँ पर मेरी सभी वस्तुएँ रखी हुई हैं। मेरा एक अलग से पढ़ने का कमरा भी है। यहाँ पर बैठ कर मैं अपनी पढ़ाईलिखाई करता हूँ। सभी कमरे खुले और हवादार हैं। सभी कमरों पर संगमरमर का फर्श है। कमरों की दीवारों पर रंग किया हुआ है। सभी कमरे सुन्दर ढंग से सजे हुए हैं। मेरे घर में एक सुन्दर बागीचा भी है। यहां पर रंग-बिरंगे तथा सुन्दर-सुन्दर फूल लगे हुए हैं। फूलों की सुगन्ध मन को मोह लेती है। शाम के समय हम बागीचे में बैठते तथा खेलते हैं। पक्की ईंटों और संगमरमर से बना मेरा घर सचमुच बहुत सुन्दर है। मुझे अपने घर पर गर्व है।

26. किसान

भारत देश एक कृषि प्रधान देश है। यहाँ के अधिकतर लोग खेती-बाड़ी का कार्य करते हैं। किसान सारे लोगों के लिए भोजन देने का कार्य करते हैं, इसीलिए इन्हें ‘अन्नदाता’ कहा जाता है। किसान दिन-रात अपने खेतों में मेहनत करके अन्न उत्पन्न करता है। गर्मी, सर्दी, वर्षा, आँधी-तूफ़ान चाहे कैसी भी ऋतु हो वह तो अपने खेतों में डटा रहता है। उसके लिए कोई रविवार नहीं, कोई छुट्टी नहीं, उसका तो बस काम ही पूजा है। अपनी मेहनत से बोई हुई फसलों को पकते हुए देखकर वह फूला नहीं समाता। पकी हुई फसलों को देखकर वह खुशी से झूम उठता है।

देश में क्या हो रहा है, इन सब बातों से उसे जैसे कोई सरोकार नहीं उसे यदि ध्यान रहता है तो अपने खेतों का, चिन्ता यदि कोई है तो बस यही कि कहीं फसल खराब न हो जाए। किसान बहुत ही सरल हृदय तथा निष्कपट स्वभाव के होते हैं। उसके लिए तो बस काम व परिश्रम ही ईश्वर की सच्ची पूजा है। पहले किसान खेतों में हल चलाने के लिए बैलों का सहारा लेते थे पर आजकल ट्रेक्टरों आदि का सहारा लिया जाता है। गेहूँ, धान आदि फसलों को बोना, उगाना, संभालना और समय पर मण्डी पहुँचाना तथा फिर नई फसलों को बोना ही उसका जीवन-चक्र है।

27. हमारा देश

हमारे देश का नाम भारत है। यह हमारी मातृभूमि है। दुष्यन्त और शकुन्तला के पुत्र भरत के नाम पर इसका भारत नाम पड़ा। यह एक विशाल देश है। जनसंख्या की दृष्टि से यह संसार में दूसरे स्थान पर है। इसकी जनसंख्या 125 करोड़ से अधिक है। यहाँ पर अलग-अलग जातियों के लोग रहते हैं।

भारत के उत्तर में हिमालय है और शेष तीनों ओर समुद्र है। स्थान-स्थान पर हरे-भरे वन इसकी शोभा हैं। यह एक कृषि प्रधान देश है। यहाँ की 80% जनता गाँवों में रहती है। यहाँ गेहूँ, मक्का -बाजरा, ज्वार, चना, धान, गन्ना आदि फसलें होती हैं। यहाँ की धरती बहुत उपजाऊ है। यहाँ गंगा-यमुना जैसी पवित्र नदियाँ बहती हैं। इसकी भूमि से लोहा, कोयला, सोना आदि कई प्रकार के खनिज पदार्थ निकलते हैं।

यहाँ पर कई धर्मों के लोग निवास करते हैं। सभी प्रेम से रहते हैं। यहाँ पर अनेक तीर्थस्थल हैं। ताजमहल, लालकिला, सारनाथ, शिमला, मंसूरी, श्रीनगर आदि पर्यटन स्थल हैं जो देखने योग्य हैं। यहाँ पर कई महापुरुषों ने जन्म लिया। राम-कृष्ण, गुरुनानक, दयानन्द, रामतीर्थ तिलक, गांधी आदि इस देश की शोभा थे। यहाँ के राष्ट्रपति श्री प्रणब मुखर्जी हैं। यह देश दिन दुगुनी रात चौगुनी उन्नति कर रहा है।

28. खेलों के लाभ

विद्यार्थी जीवन में खेलों का बड़ा महत्त्व है। पुस्तकों में उलझ कर थका-मांदा विद्यार्थी जब खेल 1 के मैदान में जाता है तो उसकी थकावट तुरन्त गायब हो जाती है। विद्यार्थी अपने आप में चुस्ती और
ताज़गी अनुभव करता है। मानव जीवन में सफलता के लिए मानसिक, शारीरिक और आत्मिक शक्तियों के विकास से जीवन सम्पूर्ण बनता है।

स्वस्थ, प्रसन्न, चुस्त और फुर्तीला रहने के लिए शारीरिक शक्ति का विकास ज़रूरी है। शरीर का विकास खेल-कूद पर निर्भर करता है। यदि हम सारा दिन कार्य करते हैं तो शरीर में घबराहट, चिड़चिड़ापन या सुस्ती छा जाती है। ज़रा खेल के मैदान में जाइये, फिर देखिए घबराहट, चिड़चिड़ापन या सुस्ती केसे दूर भागती है। शरीर हल्का-फुल्का और साहसी बन जाता है। मन में और अधिक कार्य करने की लगन पैदा होती है।

खेलों में भाग लेने से विद्यार्थी खेल के मैदान में से अनेक शिक्षाएं ग्रहण करता है। खेलें संघर्ष द्वारा विजय प्राप्त करने की भावना पैदा करती हैं। खेलें हँसते-हँसते अनेक कठिनाइयों पर विजय प्राप्त करना सिखा देती हैं। खेल के मैदान में विद्यार्थी के अन्दर अनुशासन में रहने की भावना पैदा होती है। सहयोग करने तथा भ्रातृभाव की आदत बनती है।

29. मेरा मनपसंद खेल

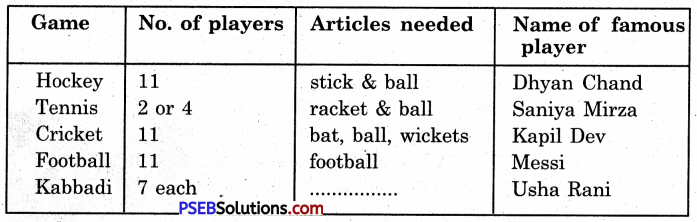
आज हमारे भारत देश में अनेक खेल खेले जाते हैं। उन सब खेलों में कबड्डी का खेल मेरा प्रिय खेल है। यह खेल सब खेलों में सस्ता खेल है। आज यह खेल भी राष्ट्रीय खेल में गिना जाता है। यह दो प्रकार का है-पंजाबी कबड्डी और नेशनल कबड्डी।

यह खेल भी दो पक्षों में खेला जाता है। इस खेल की प्रत्येक टीम में सात खिलाड़ी होते हैं। यह खेल केन्द्र में रेखा डालकर खेला जाता है। यह खेल किसी मैदान में खेला जाता है। इस खेल में से एक खिलाड़ी का सांस यदि टूट जाता है और विपक्ष के खिलाड़ी उसे छू लेते हैं या पकड़ लेते हैं तो रैफरी उसे मरा हुआ घोषित कर देता है। इस प्रकार विपक्ष एक अंक प्राप्त कर लेता है।

यदि वह खिलाड़ी दूसरे पक्ष के जितने खिलाड़ियों को छूकर अपने पक्ष में लौट आता है तो रैफरी विपक्ष के उतने ही खिलाड़ियों को मरा हुआ घोषित कर देता है। इस प्रकार यह बीस मिनट तक चलता है। बीस मिनट बाद रैफरी अर्धावकाश की सीटी मार देता है। अर्धावकाश पांच मिनट का होता है। इस समय में खिलाड़ी जलपान करते हैं। इसके अतिरिक्त दोनों टीमों के प्रशिक्षक अपने खिलाड़ियों की गलतियों को बताते हैं।

पाँच मिनट बाद रैफरी की सीटी के बाद फिर खेल उसी क्रम से शुरू होता है और बीस मिनट के बाद फिर समाप्त हो जाता है। पहले तथा दूसरे समय में जो टीम ज्यादा नम्बर लेती है वही विजयी होती है।

30. ग्रीष्म ऋतु

बसन्त की समाप्ति पर ग्रीष्म का आगमन होता है। ग्रीष्म ऋतु ज्येष्ठ और आषाढ़ महीनों में पड़ती है। बसन्त में चलने वाली मन्द-मन्द हवा ग्रीष्म के आते ही गर्म हवा में बदल जाती है जिसे दूसरे शब्दों में ‘लू’ कहते हैं।

ग्रीष्म के प्रारम्भ होते ही दिन भी मानों गर्मी से फैलने लगते हैं। सूर्य का उदय शीघ्र ही हो जाता है और फिर अस्ताचल की ओर उसका गमन भी देर से होता है। दोपहर के समय तो मानो आकाश से अंगारे बरसते हैं। एक ओर जलाती हुई लू तो दूसरी ओर जलाती हुई भूमि। इस समय बाहर निकलना भी एक समस्या बन जाता है। पशु-पक्षी भी इस समय पेड़ों के नीचे विश्राम करते हैं।

Punjab State Board PSEB 5th Class Hindi Book Solutions Hindi Rachana Patra Lekhan पत्र-लेखन Exercise Questions and Answers, Notes.

PSEB 5th Class Hindi Rachana पत्र-लेखन

1. अपने स्कूल के मुख्याध्यापक को बीमारी के कारण छुट्टी के लिए प्रार्थना-पत्र लिखो।

सेवा में
मुख्याध्यापक महोदय,
डी० ए० वी० प्राइमरी स्कूल,
अमृतसर।
श्रीमान् जी,

निवेदन यह है कि मुझे कल स्कूल से घर पहुँचते ही बुखार आ गया था। इसलिए मैं स्कूल में उपस्थित नहीं हो सकता। कृपा करके मुझे तीन दिन का अवकाश प्रदान करें। मैं आपका बहुत धन्यवादी होऊंगा।

आपका आज्ञाकारी शिष्य,
राजीव कुमार
कक्षा पाँचवीं,
रोल नं० 15

दिनांक : 10 फरवरी, 20…..

2. अपनी मुख्याध्यापिका को घर में आवश्यक काम होने के कारण छुट्टी के लिए प्रार्थना-पत्र लिखो।
अथवा
अपने स्कूल के मुख्याध्यापक जी को तीन दिन की छुट्टी के लिए प्रार्थना-पत्र लिखो।

सेवा में
मुख्याध्यापिका महोदया,
महात्मा गांधी प्राथमिक विद्यालय,
लुधियाना।
श्रीमती जी,

निवेदन यह है कि मेरी माता जी कल से सख्त बीमार हैं। मेरे पिता जी बाहर गए हुए हैं। माता जी की देखभाल के लिए मेरा घर में रहना बहुत आवश्यक है। इसलिए मैं स्कूल नहीं आ सकती। कृपा करके मुझे तीन दिन की छुट्टी दे दीजिए।

आपकी आज्ञाकारी शिष्या,
मीना शर्मा
कक्षा पाँचवीं,
रोल नं0 18

दिनांक : 12 जुलाई, 20 ……

3. कल्पना कीजिए कि आपका नाम मोहित है तथा आप पाँचवीं कक्षा के विद्यार्थी हैं। आपकी बड़ी बहन का विवाह 8 मार्च को होना निश्चित हुआ है, आप अपने मुख्याध्यापक को 3 दिन की छुट्टी के लिए प्रार्थना पत्र लिखें।

सेवा में
मुख्याध्यापक महोदय,
आर्य प्राथमिक स्कूल,
होशियारपुर।
श्रीमान् जी,

सविनय निवेदन यह है कि मेरी बड़ी बहन का विवाह 8 मार्च को होना निश्चित हुआ है। मेरा इस विवाह में शामिल होना बहुत ज़रूरी है। इसलिए मैं स्कूल में उपस्थित होने में असमर्थ हूँ। कृपा करके मुझे 7 मार्च से 9 मार्च तक तीन दिन का अवकाश प्रदान करें। मैं आपका अति आभारी होऊंगा।

आपका आज्ञाकारी शिष्य,
मोहित
कक्षा पाँचवीं,
रोल नं0 22

दिनांक : 6 मार्च, 20……

4. अपने स्कूल के मुख्याध्यापक को फीस माफी के लिए प्रार्थना-पत्र लिखो।

सेवा में
मुख्याध्यापक महोदय,
ए० एस० प्राथमिक स्कूल,
जालन्धर।
श्रीमान जी,

निवेदन यह है कि मैं आपके स्कूल की कक्षा पाँचवीं ‘ए’ का विद्यार्थी हूँ। मेरे पिता जी बहुत ग़रीब हैं। वे एक मामूली-सी दुकान पर काम करते हैं। इससे घर का खर्च भी अच्छी प्रकार से नहीं चलता। इसलिए वे मेरी फीस नहीं दे सकते, परन्तु | मैं अपनी पढ़ाई छोड़ना नहीं चाहता। कृपा करके आप मेरी फीस माफ कर दें। मैं आपका बहुत धन्यवादी होऊंगा।

आपका आज्ञाकारी शिष्य,
भारत भूषण
पाँचवीं ‘ए’
रोल नं० 15

दिनांक : 24 अप्रैल, 20 ……

5. मान लीजिए कि आप हिमांशी हैं। आपको कक्षा अध्यापक ने कक्षा में देरी से आने पर 50 रुपये जुर्माना लगाया है। आप अपने स्कूल के मुख्याध्यापक को जुर्माना माफी के लिए पत्र लिखें। . (From

सेवा में
मुख्याध्यापक महोदय,
नेहरू प्राथमिक विद्यालय,
गुरदासपुर।
श्रीमान् जी,

निवेदन यह है कि मैं कल प्रातः काल अपने गाँव से साइकिल से स्कूल आ रही थी। रास्ते में मेरी साइकिल में पंचर हो गया। मुझे स्कूल पहुँचने के लिए दो मील तक पैदल चलना पड़ा। इसलिए मैं एक घण्टा देर से पहुँची। अध्यापक महोदय ने मुझे 50 रुपये जुर्माना कर दिया है। मैं इससे पहले कभी देर से नहीं आयी। आगे भी देर से नहीं आऊंगी। कृपा करके आप मेरा जुर्माना माफ कर दें।

मैं आपकी बहुत धन्यवादी होऊंगी।।

आपकी आज्ञाकारी शिष्या,
कक्षा पाँचवीं,
रोल नं० 4

दिनांक : 16 नवम्बर, 20……

6. स्कूल छोड़ने का प्रमाण-पत्र (सर्टिफिकेट) लेने के लिए मुख्याध्यापक को प्रार्थना-पत्र लिखो।

सेवा में
मुख्याध्यापक महोदय,
नगरपालिका प्राथमिक पाठशाला,
नवांशहर।
श्रीमान् जी,

निवेदन यह है कि मेरे पिता जी का स्थानांतरण (तबादला) हो गया है। वे कल यहाँ से कानपुर जा रहे हैं और साथ में परिवार भी जा रहा है। इस अवस्था में मेरा यहाँ अकेला रहना कठिन है। कृपा करके मुझे स्कूल छोड़ने का प्रमाण-पत्र दीजिए ताकि मैं वहां जाकर स्कूल में प्रवेश पा सकूँ।

धन्यवाद सहित।

आपका आज्ञाकारी शिष्य,
सुनील कक्षा पाँचवीं,
रोल नं0 32

दिनांक : 11 जनवरी, 20 ……..

7. मित्र को गर्मियों की छुट्टियां एक साथ बिताने के लिए निमन्त्रण-पत्र लिखो।

330, मॉडल टाऊन,
लुधियाना।
15 जून, 20…

प्रिय मित्र राकेश,

अगले सप्ताह से गर्मी की छुट्टियां आरम्भ हो रही हैं। स्कूल दो मास तक बन्द रहेगा। इस बार मैंने छुट्टियों के दिन अपने चाचा जी के यहां शिमला में बिताने का निश्चय किया है। मित्र, यदि तुम भी मेरे साथ चलो तो मज़ा आ जाएगा। वहाँ हम मिलकर पढ़ेंगे और शाम के समय वहां के प्राकृतिक दृश्यों का आनन्द लेंगे। शिमला में मेरे चाचा जी का एक बहुत बड़ा मकान है। अतः हमें वहाँ रहने में कोई
तुम छुट्टी आरम्भ होते ही मेरे पास पहुँच जाओगे। यहाँ से अगले ही दिन हम शिमला के लिए रवाना हो जाएंगे।

तुम्हारा मित्र,
सुमीत।

8. चाचा जी (मामा जी) को उपहार के लिए धन्यवाद-पत्र लिखो।

21, माल रोड,
बठिण्डा।
28 सितम्बर, 20 …..

पूज्य चाचा जी,
नमस्ते !

मेरे जन्म दिन पर. आपके द्वारा भेजा गया उपहार मुझे मिल गया है। यह एक सुन्दर घड़ी है। मुझे यह घड़ी बहुत पसन्द आई है। वैसे भी मुझे घड़ी की ज़रूरत थी। मुझे स्कूल पहुँचने में सदा देरी हो जाती थी, परन्तु अब मैं समय से पहले ही स्कूल पहँच जाया करूँगा। मेरे पेपर भी शुरू होने वाले हैं। इन दिनों में मैं इस घड़ी से पूरा-पूरा लाभ उठा सकूँगा। इस सुन्दर उपहार के लिए मैं आपका बहुतबहुत धन्यवाद करता हूँ। चाची जी को प्रणाम। बिटू को प्यार।

आपका भतीजा,
अश्विनी।

9. अपने छोटे भाई को लेख सुधारने के लिए , एक पत्र लिखो।

परीक्षा भवन,
…….. नगर।
25 अगस्त, 20…

प्रिय दिनेश,

आज तुम्हारा पत्र मिला। तुम्हारा लेख देखकर बड़ा दुःख हुआ। मैं बड़ी कठिनाई से तुम्हारा पत्र पढ़ सका हूँ। सुलेख के बिना तुम परीक्षा में अच्छे अंक | न पा सकोगे। इसलिए अपना लेख सुधारने की कोशिश | करो। क्योंकि लेख का सुलेख होना आवश्यक है।

तुम्हारा बड़ा भाई,
मोहन लाल।

10. राखी प्राप्ति पर अपनी बड़ी बहन को एक पत्र लिखो।

परीक्षा भवन,
…….. नगर।
25 अगस्त, 20 …….

पूज्य बहन जी,

चरण वन्दना। आज आपकी भेजी हुई राखी मिली। बड़ी सुन्दर राखी है। इस पर लगा फूल मनमोहक है। यह राखी आपके स्नेह की निशानी है। मैं इस स्नेह के बन्धन को अच्छी तरह निभाऊंगा। जीजा जी को प्रणाम । पूनम को प्यार।

आपका प्रिय भाई,
पवन।

11. नगरपालिका के प्रधान को गली की सफ़ाई के लिए पत्र लिखो।’

परीक्षा भवन,
……… नगर।
12 अप्रैल, 20 ……..

सेवा में
श्रीमान् प्रधान जी,
नगरपालिका,
………. नगर।

आपसे निवेदन है कि हमारी गली की दशा बहुत खराब है। सारी गली में कूड़े और गन्दगी के ढेर लगे हुए हैं। गन्दगी के कारण बीमारी फैलने का बहुत डर है। इसलिए कृपा करके आप हमारी गली की सफ़ाई की ओर पूरा ध्यान दें।

आपके आज्ञाकारी
हम हैं निवासी,
…………
राम गली,
………. पुर।

12. अपने मित्र को अपने बड़े भाई के विवाह पर निमन्त्रण पत्र लिखो।

75/बी पीतमपुरा,
दिल्ली।
8 दिसम्बर, 20 ……..

प्रिय राजेश,
सप्रेम नमस्ते।

तुम्हारी कोई जानकारी कई दिनों से नहीं मिली। तुम्हें यह जानकर प्रसन्नता होगी कि मेरे बड़े भाई का शुभ-विवाह 16 दिसम्बर को निश्चित हुआ है। बारात दिल्ली से मथुरा जा रही है। तुम्हें बारात में अवश्य चलना होगा। मैंने रमेश, अरुण तथा जगदीश को भी बुलाया है। तुम साथ रहोगे तो और भी मजा आएगा। इसलिए तुम 15 दिसम्बर तक यहाँ अवश्य पहुँच जाना। छोटे भाई राजेन्द्र को भी लाना मत भूलना।

प्रतीक्षा में.
तुम्हारा मित्र,
विश्वास वर्मा।

13. अपने मित्र को पत्र लिखो कि वह आपकी बहन की शादी में शामिल हो।

16/A जनकपुरी,
लुधियाना।
4 दिसम्बर, 20…….

प्रिय सुरेन्द्र,
सप्रेम नमस्ते,

तुम्हें यह जानकर बड़ी प्रसन्नता होगी कि मेरी बहन का विवाह 18-12-20…. को निश्चित हुआ है। इस खुशी के अवसर पर तुम्हारा यहाँ उपस्थित रहना बहुत ही ज़रूरी है। तुम पत्र मिलते ही यहाँ आने की तैयारी कर लो। मुझे तुम्हारा इन्तज़ार रहेगा। साथ में अंकल और आंटी को लाना मत भूलना। बाकी बातें मिलने पर होंगी।

प्रतीक्षा में,
तुम्हारा अभिन्न मित्र,
राजेन्द्र।

14. पत्रों के सही वितरण न होने पर स्थानीय पोस्ट मास्टर को शिकायत भरा पत्र लिखो।

सेवा में
पोस्टमास्टर महोदय,
मुख्य डाकघर,
लुधियाना।
श्रीमान् जी,

निवेदन है कि हमारे मुहल्ले का डाकिया बहुत आलसी और लापरवाह है। वह ठीक समय पर पत्र नहीं पहुँचाता। कभी-कभी तो वह बच्चों के हाथ पत्र देकर चला जाता है। वे पत्र इधर-उधर फेंक देते हैं। कल ही शर्मा जी का पत्र नाली में गिरा हुआ पाया गया। हमने उसे कई बार समझाया है, पर वह हमारी बात मानता ही नहीं।

इसलिए आपसे प्रार्थना है कि या तो आप उसे समझा दें ताकि वह आगे से ऐसा न करे या फिर आप उसे बदल दीजिए। आशा है कि आप हमारी प्रार्थना पर अवश्य ध्यान देंगे।

प्रार्थी
रवि, मोहन, दीपक और अन्य।

15. रुपए मंगवाने के लिए पिता जी को पत्र लिखो।

………….. स्कूल,
……… शहर।
22 अगस्त, 20…

पूज्य पिता जी,
सादर प्रणाम।

आपको यह जानकर प्रसन्नता होगी कि मैं चौथी कक्षा में अच्छे अंक प्राप्त करके प्रथम श्रेणी में उत्तीर्ण हुआ हूँ। अब मुझे पाँचवीं श्रेणी में दाखिला लेना है तथा नई पुस्तकें और कापियाँ खरीदनी हैं। इसलिए कृपा करके मुझे 500 रुपए मनीआर्डर द्वारा तुरन्त भेज दें ताकि ठीक समय पर मैं दाखिला ले सकू।

माता जी को प्रणाम और टिंकू को प्यार।
आपका आज्ञाकारी पुत्र,
संजय।

16. अपने जन्म दिन पर अपने चाचा जी को निमन्त्रण (बुलावा) पत्र लिखो।

205, मॉडल टाऊन।
अमृतसर।
20 अप्रैल, 20 ……..

पूज्य पिता जी,
सादर, प्रणाम।

आपको यह जानकर प्रसन्नता होगी कि 23 अप्रैल को मेरा जन्म दिन है। इसलिए मैं अपने मित्रों को शाम की चाय पार्टी दे रहा हूँ, आप भी चाची जी, रिंकू और नीता को लेकर इस छोटी-सी चाय पार्टी पर आएं।

चाची जी को प्रणाम। रिंकू और नीतू को प्यार।

आपका भतीजा,
नरेश कुमार।

17. मित्र की सफलता पर बधाई पत्र लिखो।

208, प्रेम नगर,
पटियाला।
11 अप्रैल, 20 …….

प्रिय मित्र सुरेश,

कल ही तुम्हारा पत्र मिला। यह पढ़कर बहुत ही खुशी हुई कि तुम पाँचवीं कक्षा में प्रथम श्रेणी में उत्तीर्ण (पास) हो गए हो। मेरी ओर से अपनी इस शानदार सफलता पर हार्दिक बधाई स्वीकार करो। मैं कामना (इच्छा) करता हूँ कि तुम अगली परीक्षा में भी इसी प्रकार सफलता प्राप्त करोगे। मैं एक बार फिर तुम्हें बहुत-बहुत बधाई देता हूँ।

अपने माता-पिता को मेरा प्रणाम कहना।

तुम्हारा मित्र,
राजबीर।

18. अपनी माता जी को अपनी कुशलता के बारे में पत्र लिखो।

सरकारी स्कूल छात्रावास,
जालन्धर।
1 अगस्त, 20 …….

पूज्य माता जी,
सादर प्रणाम।

मैं पिछले रविवार को यहाँ पहुँच गया था। सोमवार से हमारी पढ़ाई ठीक प्रकार से शुरू हो गई है। मैं पूर्णतया कुशल से हूँ। बुखार के कारण जो कमज़ोरी आ गई थी, वह अब नहीं रही। छात्रावास में भोजन का अच्छा प्रबन्ध है। किसी प्रकार की चिन्ता न करें। अब मैं समय सारिणी के अनुसार चल रहा हूँ। प्रात 5 बजे उठ जाता हूँ। शौचादि से निवृत्त होकर कुछ व्यायाम करता हूँ, फिर नहा-धोकर अल्पाहार लेकर पढ़ने बैठ जाता हूँ। 9 बजे स्कूल का समय है। वहां से लौटकर सायं 5 बजे सब छात्र क्रीड़ा-क्षेत्र में चले जाते हैं। मैं कबड्डी टीम में हूँ। रोज़ की कसरत से मेरे अन्दर नई ताज़गी का आभास होने लगता है। रात को समय पर सो जाता हूँ।

आपका पुत्र,
अमित कुमार,
पाँचवीं ‘ए’।

19. मुख्याध्यापक को विद्यालय से मुफ्त पुस्तकें एवं वर्दी प्राप्त करने के लिए प्रार्थना पत्र।

सेवा में
मुख्याध्यापक जी,
राजकीय प्राथमिक विद्यालय,
सोनीपत।
महोदय,

निवेदन है कि मैं आपके विद्यालय की पाँचवीं कक्षा का छात्र हूँ। मेरे पिताजी सरकारी कार्यालय में लिपिक हैं। हम तीन भाई-बहन हैं। पिताजी का वेतन इतना कम है कि वे हमारी पढ़ाई का भार सम्भालने में कठिनाई का अनुभव कर रहे हैं। मैंने पाँचवीं कक्षा की वार्षिक परीक्षा में 96% अंक प्राप्त किये हैं। खेलों में भी मेरी रुचि है।

आपसे सानुरोध प्रार्थना है कि हमारी आर्थिक स्थिति को ध्यान में रखते हुए मुझे विद्यालय की ओर से छठी श्रेणी की पुस्तकें तथा वर्दी देने की कृपा करें। मैं आपका हृदय से आभारी रहूँगा।

आपका आज्ञाकारी शिष्य,
रमेश,
पाँचवीं ‘ग’

दिनांक …………..

20. सैक्शन बदलने के लिए प्रार्थना-पत्र।

सेवा में
श्रीमान् प्रधानाध्यापक जी,
हिन्दू प्राथमिक स्कूल,
जगरांव।
आदरणीय महोदय,

विनम्र निवेदन है कि मैं आपके स्कूल की पाँचवीं कक्षा सैक्शन ‘बी’ में पढ़ता हूँ। मैं इस विद्यालय का पुराना विद्यार्थी हूँ। एक मास तक इस सैक्शन में पढ़ने के बाद मुझे यह अनुभव हुआ कि इस सैक्शन में विद्यार्थी पढ़ने वाले कम और शरारती अधिक हैं। साथ ही इस सैक्शन के अंग्रेज़ी के अध्यापक हमें व्याकरण नहीं पढ़ाते। हिन्दी के अध्यापक भी कक्षा में गप्पें मारते रहते हैं और वे विशेष उत्साह नहीं दिखाते। विद्यार्थी घर से जो काम करके लाते हैं उसे कोई देखता नहीं। साथ ही पिछले वर्ष इस सैक्शन का परिणाम भी उतना अच्छा नहीं रहा।

कृपा करके आप मुझे सैक्शन ‘ए’ मैं बैठने की आज्ञा दें। आपका अति आभारी रहूँगा।

आपका आज्ञाकारी शिष्य,
राजीव,
पाँचवीं-बी।

तिथि ………

21. अपने छोटे भाई को पत्र लिखो जिसमें प्रातः भ्रमण (सुबह की सैर) के लाभ बताए गए हों।

208 कृष्ण नगर,
बहादुरगढ़।
11 जुलाई, 20….

प्रिय भाई नरेश,
चिरंजीव रहो।

कल माता जी का पत्र मिला। पढ़ कर पता चला कि तुम बीमार रहने के कारण बहुत कमज़ोर हो गए हो। तुम सुबह देर तक सोए रहते हो। प्यारे भाई! प्रातः उठ कर सैर करनी चाहिए। सुबह की सैर से स्वास्थ्य उत्तम होता है। प्रातः भ्रमण से शरीर चुस्त रहता है। कोई बीमारी पास नहीं फटकती। मांसपेशियों में नए रक्त का संचार होता है। फेफड़ों को साफ़ वायु मिलती है। ओस पड़ी घास पर नंगे पांव चलने से बल, बुद्धि और आँखों की रोशनी बढ़ती है। दिमाग को शक्ति मिलती है। इसलिए प्रातः घूमने अवश्य जाया करो।

आशा है तुम मेरे आदेश का पालन करोगे। पूज्य माताजी को प्रणाम और अनु को प्यार।

तुम्हारा बड़ा भाई,
प्रदीप कुमार।

Punjab State Board PSEB 5th Class Hindi Book Solutions Hindi Rachana Kahani Lekhan कहानी-लेखन Exercise Questions and Answers, Notes.

PSEB 5th Class Hindi Rachana कहानी-लेखन

1. कुसंगति का फल

किसी वन में एक हंस और कौआ रहते थे। हंस स्वभाव से ही भोला-भाला था और कौआ बड़ा दुष्ट था। वे दोनों रात को एक पेड़ पर रहा करते थे।

एक दिन गर्मी के मौसम में वे दोनों दोपहर के समय पेड़ पर इकट्ठे बैठे हुए थे। इतने में एक यात्री गर्मी से व्याकुल हुआ वृक्ष की छाया में आराम करने के लिए आ लेटा। वह ठण्डी छाया में शीघ्र ही गहरी नींद सो गया। आराम से सोए हुए उसने अपना मुँह खोल दिया। इतने में दूसरे के सुख को न सहन करने वाले कौए ने उसके मुँह पर बीठ कर दी। यात्री जाग पड़ा। उसने क्रोध से ऊपर की ओर देखा। दुष्ट कौआ चालाक था। वह तो शीघ्र उड़ गया। परन्तु स्वभाव से सुशील हंस अभी वहीं पंख फैलाए बैठा हुआ था। यात्री ने उसकी शरारत समझी। उसने उसी समय अपने बाण से उसे मार दिया।

शिक्षा- (1) दुर्जन के साथ कहीं नहीं जाना चाहिए और न कहीं बैठना चाहिए।
(2) दुष्ट की संगति से मनुष्य अकेला भला।

2. एकता में बल है

किसी गाँव में एक बूढ़ा किसान रहता था। उसके चार पुत्र थे। वे चारों बहुत आलसी थे। वे आपस में लड़ते-झगड़ते रहते थे। किसान ने उन्हें बहुत समझाया लेकिन व्यर्थ। एक दिन उसने अपने चारों पुत्रों को अपने पास बुलाया तथा लकड़ियों का एक गट्ठर लाने को कहा। वे लकड़ियों का एक गट्ठर ले आए। उसने हर एक लड़के को वह गट्ठर तोड़ने के लिए दिया। लेकिन कोई भी उसे न तोड़ सका। तब उसने एक-एक लकड़ी सभी को दी। अब सभी ने आसानी से तोड दी। तब उसने उन्हें समझाया कि, “अगर तुम अकेले-अकेले रहोगे तो लोग तुम्हें नष्ट कर देंगे। अतः तुम सब इकट्ठे रहो। लड़ना-झगड़ना अच्छा नहीं। “एकता में ही बल है।” यह सुन कर वे सब मिल-जुल कर रहने लगे।

शिक्षा- मिल-जुल कर रहना चाहिए। एकता में ही बल है।

3. अंगूर खट्टे हैं

एक बार एक लोमड़ी थी। एक दिन वह बहुत भूखी थी। वह भोजन को ढूंढते-ढूंढते एक बाग में गई। वहाँ उसने वृक्ष की बेल पर कुछ अंगूरों के अच्छे गुच्छे देखे। वह उन्हें देखकर बहुत प्रसन्न हुई। वह अंगूरों को खाना चाहती थी। अंगूर ऊँचे थे।। वह ऊँची-ऊँची छलांगें लगाने लगी, किन्तु वह उन तक पहँच न सकी। बार-बार उछलने से वह बहुत थक गई। आखिर वह बाग से बाहर जाती हुई कहने लगी कि ये अंगूर तो खट्टे हैं। यदि मैं इन्हें खाऊंगी तो बीमार हो जाऊंगी।

शिक्षा- “हाथ न पहुँचे, थू कौड़ी।”

4. प्यासा कौआ

गर्मियों के दिन थे। दोपहर के समय बहुत सख्त गर्मी पड़ रही थी। एक कौआ पानी की तलाश में भटकता हुआ एक बाग में पहुँचा। अचानक उसकी नज़र वृक्ष के नीचे पड़े एक घड़े पर गई। वह उड़ कर वहां जा कर घड़े पर बैठा। उसने चोंच पानी में डालने की कोशिश की लेकिन उसकी चोंच पानी तक न पहुँच सकी क्योंकि घड़े में पानी बहुत कम था।

तभी उसे एक उपाय सूझा। उसने घड़े के आसपास बिखरे हुए कंकर उठा कर घड़े में डालने शुरू कर दिए। पानी ऊपर आ गया। उसने जी भर कर पानी पीया और उड़ गया।

शिक्षा- जहाँ चाह वहाँ राह।
अथवा
युक्ति से मुक्ति मिलती है।

5. चालाक लोमड़ी

एक लोमड़ी थी। वह बहुत भूखी थी। वह भोजन की खोज में इधर-उधर घूमने लगी। जब सारे जंगल में भटकने के बाद भी कुछ न मिला तो वह गर्मी और भूख से परेशान होकर एक पेड़ के नीचे बैठ गई। अचानक उसकी नज़र ऊपर की ओर गई। वृक्ष पर एक कौआ बैठा हुआ था। उसके मुँह में रोटी का टुकड़ा था। रोटी देखकर लोमड़ी के मुँह में पानी भर आया। वह कौए से रोटी छीनने का उपाय सोचने लगी।

तभी उसने कौए को कहा, “क्यों कौआ भैया। सुना है तुम गीत बहुत अच्छा गाते हो। क्या मुझे गीत नहीं सुनाओगे ?” कौआ अपनी झूठी प्रशंसा को सुन कर बहुत खुश हुआ। उसने ज्यों ही गाने के लिए मुँह खोला त्यों ही रोटी का टुकड़ा नीचे गिर गया। लोमड़ी ने रोटी का टुकड़ा उठाया और नौ-दो ग्यारह हो गई। कौआ पछताने लगा।

शिक्षा- किसी की झूठी प्रशंसा में नहीं आना चाहिए।

6. टोपी वाला और बन्दर

एक सौदागर था। वह टोपियां बेचकर अपना पेट पाला करता था। एक दिन वह टोपियां बेचने दूसरे गाँव में गया। गर्मी से व्याकुल होकर वह वृक्ष के नीचे बैठ गया। बैठते ही उसे नींद आने लगी। वह टोपियों से भरा सन्दूक पास रखकर सो गया।

उसी वृक्ष पर कुछ बन्दर बैठे थे। वे झट नीचे उतरे, सौदागर के सन्दुक से टोपियां निकाल कर उन्होंने अपने सिर पर पहन ली और छलांगें लगाते हुए वृक्ष पर चढ़ गए। नींद खुलने पर सौदागर ने सन्दूक खुला देखा और टोपियां उसमें से गुम पाईं। क्या मेरी टोपियां कोई चुरा ले गया है ? वह यह सोच ही रहा था कि तभी उसकी नज़र वृक्ष के ऊपर टोपियां पहने बन्दरों पर पड़ी।

सौदागर ने बन्दरों को बहुत डराया लेकिन टोपियां प्राप्त करने में उसे सफलता न मिली। अन्त में उसने एक उपाय सोचा और सोचते ही अपने सिर से टोपी उतार कर नीचे फेंक दी। इस पर बन्दरों ने भी अपनी-अपनी टोपियां सिर से उतार कर ज़मीन पर फेंक दीं। सौदागर ने टोपियां इकट्ठी की और अपने घर की ओर चल पड़ा।

शिक्षा- जो काम बुद्धि से हो सकता है, वह ताकत से नहीं।

7. दो बिल्लियाँ और बन्दर

किसी नगर में दो बिल्लियाँ रहती थीं। एक दिन उन्हें कहीं से रोटी का एक टुकड़ा मिला। वे आपस में लड़ने लगीं। वे उसे आपस में समान भागों में बांटना चाहती थीं, लेकिन उन्हें कोई उपाय न सूझा।

उसी समय एक बन्दर उधर आ निकला। वह बहुत चालाक था। उसने बिल्लियों से लड़ने का कारण पूछा। बिल्लियों ने उसे सारी बात सुना दी। वह कहीं से तराजू लाया और बोला, “लाओ, मैं तुम्हारी रोटी को बराबर-बराबर बांट देता हूँ।” उसने रोटी के दो टुकड़े लेकर एक-एक पलड़े में रख दिए। जिस पलड़े में रोटी अधिक होती बन्दर उससे थोड़ी-सी तोड़ कर खा लेता। इस प्रकार थोड़ी-सी रोटी रह गई। बिल्लियों ने अपनी थोड़ी-सी रोटी वापस मांगी। बन्दर ने कहा, “यह तो मेरी मजदूरी है।” यह कह कर उसने बाकी बची हुई रोटी भी मुँह में डाल ली। बिल्लियाँ मुँह ताकती रह गईं।

शिक्षा- आपस में लड़ना-झगड़ना अच्छा नहीं होता।

8. ईमानदार लकड़हारा

एक गाँव में एक लकड़हारा रहता था। वह बहुत ग़रीब था। वह निकट के जंगल से लकड़ी काट कर लाता और बेचकर अपना निर्वाह करता था।

एक दिन वह नदी के किनारे वृक्ष काट रहा था। अचानक उसके हाथ से कुल्हाड़ा छूट गया और नदी में गिर पड़ा। लकड़हारा बेचारा रोने लगा। उसने सोचा कि मैं अब बच्चों का पालन कैसे करूँगा। वह रो रहा था कि जल देवता उपस्थित हुआ। उसने पूछा, “तुम क्यों रो रहे हो? लकड़हारे ने सारी बात बताई। जल देवता पानी में कूद पड़ा और सोने का कुल्हाड़ा निकाल लाया। उसने लकड़हारे से पूछाक्यों यही तुम्हारा कुल्हाड़ा है? लकड़हारे ने उसे लेने से इन्कार कर दिया और कहा यह मेरा कुल्हाड़ा नहीं है। जल के देवता ने फिर पानी में डुबकी लगाई। और इस बार चाँदी का कुल्हाड़ा लाया। परन्तु लकड़हारे ने फिर वह कुल्हाड़ा लेने से इन्कार कर दिया। अब तीसरी बार जल का देवता फिर नदी में कूदा और वही लोहे का कुल्हाड़ा निकाल लाया।

उस कुल्हाड़े को देखते ही लकड़हारा खुशी से नाच उठा-हां, हां यही मेरा कुल्हाड़ा है। जल का देवता उसकी ईमानदारी से बहुत खुश हुआ। उसने लकड़हारे को शेष दोनों कुल्हाड़े भी इनाम के तौर पर दे दिए।

शिक्षा- (1) ईमानदारी सबसे उत्तम नीति है।
(2) सदा सच बोलना चाहिए।

9. शेर और चूहा

एक दिन गर्मी बहुत पड़ रही थी। एक शेर शिकार ढंढते-ढंढते थक गया। उसने एक छायादार वृक्ष देखा और उसके नीचे सो गया। पास ही एक चूहे का बिल था। थोड़ी देर बाद चूहा अपने बिल से बाहर निकला। वह शेर के शरीर पर चढ कर कूदने लगा। शेर जाग पड़ा और उसने चूहे को अपने पंजे में जकड़ लिया। चूहा बहुत चालाक था। वह घबराया नहीं। उसने शेर से कहा-“महाराज आप जंगल के राजा हैं। मैं छोटा-सा जीव हूँ। मुझे मारना आपको शोभा नहीं देता। आप दया करके मुझे छोड़ दें। कभी | मैं भी आपके काम आ सकता हूँ।” यह सुनकर शेर हँस पड़ा और उसने चूहे को छोड़ दिया।

कुछ दिनों के बाद उस जंगल में एक शिकारी आया। वह शेर शिकारी के जाल में फंस गया। उसने अपने आप को जाल से छुड़ाने की बहुत कोशिश की, परन्तु सफल न हो सका। अन्त में वह ज़ोर| ज़ोर से दहाड़ने लगा। उसी चूहे ने शेर की आवाज़ को पहचान लिया। वह झट से जाल के पास गया। उसने कुछ ही देर में अपने तेज़ दाँतों से जाल को काट दिया और शेर की जान बचाई। शेर ने उसका धन्यवाद किया।

शिक्षा- (1) किसी को छोटा नहीं समझना चाहिए।
(2) कर भला हो भला।

10. हाथी और दर्जी

किसी राजा के पास एक हाथी था। हाथी प्रतिदिन स्नान करने के लिए नदी पर जाया करता था। रास्ते में एक दर्जी की दुकान पड़ती थी। दर्जी बहुत दयालु तथा उदार था। उसकी हाथी से मित्रता हो गई। वह हाथी को प्रतिदिन कुछ-न-कुछ खाने के लिए देता था।

एक दिन दर्जी किसी कारण से क्रोध में बैठा हुआ था। हाथी आया और कुछ प्राप्त करने के लिए अपनी सूंड आगे की। दर्जी ने उसे कुछ खाने के लिए नहीं दिया, परन्तु उसकी सूंड में सूई चुभो दी। हाथी को क्रोध आ गया। वह नदी पर गया। उसने स्नान किया और लौटते समय अपनी सूंड में गन्दा पानी भर लिया। दर्जी की दुकान पर पहुँच कर उसने सारा गन्दा पानी उसके कपड़ों पर फेंक दिया। दर्जी के सारे कपड़े खराब हो गए। इस तरह हाथी ने दर्जी से बदला लिया।

शिक्षा- जैसे को तैसा।

11. लालची कुत्ता

एक कुत्ता था। एक बार वह भोजन की तलाश में इधर-उधर घूम रहा था। अन्त में उसे एक रोटी का टुकडा मिला। वह इसे अकेले में बैठकर खाना चाहता था । इसलिए वह एकान्त की खोज में निकल पड़ा। वह एक नदी के किनारे पहुँचा। उसने पानी में झाँक कर देखा। उसे पानी में अपनी ही परछाईं दिखाई दी। उसने सोचा कि वह कोई दूसरा कुत्ता है जिसके मुँह में भी रोटी का टुकड़ा है। उसके मन में लालच आ गया। उसने सोचा कि क्यों न मैं इससे भी रोटी का टुकड़ा छीन लूं इसलिए वह ज़ोर से भौंका। भौंकने से उसका अपना रोटी का टुकड़ा भी पानी में गिर गया। वह अपना रोटी का टुकड़ा भी खो बैठा। अब वह पछताने लगा लेकिन अब क्या हो सकता था। वह चुपचाप वापिस लौट आया।

शिक्षा- लालच बुरी बला है।

12. दो मित्र और रीछ

मोहन और सोहन दो मित्र थे। दोनों एक ही गाँव में रहते थे। एक बार वे दोनों किसी काम से शहर जा रहे थे। रास्ते में एक जंगल पडता था। दोनों जंगल से गुजर रहे थे कि सामने से उन्हें एक रीछ (भालू) आता दिखाई दिया। रीछ को देखकर मोहन तो झट से एक पेड़ पर चढ़ गया और पत्तों के बीच छिप गया। सोहन को पेड़ पर चढ़ना नहीं आता था। उसने सुन रखा था कि रीछ मरे हुए जीव को नहीं खाता। वह झट से अपनी साँस रोक कर धरती पर लेट गया। रीछ ने उसे सँघा और मरा हुआ समझ कर | छोड़कर चला गया। रीछ के चले जाने पर मोहन पेड़ पर से नीचे उतरा और सोहन से कहने लगा कि बताओ रीछ तुम्हारे कान में क्या कह रहा था। सोहन ने कहा कि उसने मुझे समझाया कि स्वार्थी मित्रों पर कभी विश्वास नहीं करना चाहिए। ऐसे मित्रों से दूर रहना चाहिए।

शिक्षा- धोखेबाज़ मित्रों से सावधान रहना चाहिए।

Punjab State Board PSEB 5th Class Hindi Book Solutions Hindi Grammar muhavare मुहावरे Exercise Questions and Answers, Notes.

PSEB 5th Class Hindi Grammar मुहावरे

1. अन्धे की लकड़ी – एकमात्र सहारा।
प्रयोग – रमेश ही बुढ़ापे में मुझ अन्धे की लकड़ी है।

2. अंगूठा दिखाना – साफ़ इन्कार करना।
प्रयोग – जब मैंने सुरेश से दस रुपये मांगे तो उसने मुझे अंगूठा दिखा दिया।

3. अपना उल्लू सीधा करना – अपना मतलब निकालना।
प्रयोग – आजकल हर कोई अपना उल्लू सीधा करना चाहता है।

4. अगर – मगर करना टाल मटोल करना।
प्रयोग – जब मैंने राजेश से पचास रुपए मांगे तो वह अगर मगर करने लगा।

5. अंग – अंग ढीला होना थक जाना।
प्रयोग – दिन भर मेहनत करने से मजदूरों का अंग अंग ढीला हो जाता है।

6. आँखों का तारा – बहुत प्यारा।
प्रयोग – सुनील तो अपने माता पिता की आँखों का तारा है।

7. ईंट से ईंट बजाना – नष्ट करना।
प्रयोग – बन्दा बहादुर ने सरहिन्द की ईंट से ईंट बजा दी।

8. ईद का चाँद होना – बहुत दिनों बाद दिखाई देना।
प्रयोग – अरे सुरेश ! तुम तो ईद का चाँद हो गए हो, कहाँ रहते हो?

9. कान पर जूं न रेंगना – कोई असर न होना।
प्रयोग – मेरे कहने पर तो उसके कान पर जूं तक नहीं रेंगी।

10. कमर कसना – तैयार होना।
प्रयोग – आज से मैंने पढ़ने के लिए कमर कस ली है।

11. काम तमाम करना – मार डालना।
प्रयोग – तलवार के एक ही वार में महाराणा प्रताप ने अपने शत्रु का काम तमाम कर दिया।

12. खाक छानना – मारे मारे फिरना।
प्रयोग – बचपन में बलदेव ने पढ़ाई नहीं की और अब खाक छानता फिरता है।

13. गाढ़े पसीने की कमाई को तीली लगानामेहनत से कमाई दौलत को बुरी तरह उजाड़ देना।
प्रयोग – मूर्ख बेटे ने बाप की गाढ़े पसीने की कमाई को तीली लगा दी।

14. खदेड़ देना – भगा देना।
प्रयोग – शीघ्र ही भारतीय सैनिकों ने शत्रु को खदेड़ दिया।

15. खून खौलना – बहुत जोश आना।
प्रयोग – सुरेश की जली कटी बातें सुनकर मेरा तो खून खौलने लगा।

16. घी के दीये जलाना – बहुत खुशी मनाना।
प्रयोग – जब अयोध्या में श्री रामचन्द्र जी वापस पहुँचे तो लोगों ने घी के दीये जलाये।

17. घोड़े बेच कर सोना-बे – फिक्र होकर सोना।
प्रयोग – परीक्षा के बाद सब विद्यार्थी घोड़े बेच कर सोते हैं।

18. चकमा देना – धोखा देना।
प्रयोग – डाकू पुलिस को चकमा देकर भाग गया।

19. छक्के छुड़ाना – बुरी तरह हराना।
प्रयोग – युद्ध में भारतीय सेना ने पाकिस्तान की सेना के छक्के छुड़ा दिये।

20. छीना झपटी करना – लेने के लिये भिड़ना।
प्रयोग – छीना झपटी मत करो, सबको प्रसाद मिलेगा।

21. दाँत खट्टे करना – बुरी तरह हराना।
प्रयोग – हमने हर बार दुश्मन के दाँत खट्टे किए हैं।

22. डींग मारना – शेखी बघारना।
प्रयोग – डींग मारने वालों पर विश्वास मत कीजिए।

23. नमक – मिर्च लगाना छोटी सी बात को बढ़ाचढ़ा कर कहना।
प्रयोग – हरीश के स्कूल से भागने पर सुरेश ने मुख्याध्यापक से खूब नमक-मिर्च लगाकर उसकी शिकायत की।

24. ताक में रहना – टोह में रहना।
प्रयोग – रमेश हमेशा इसी ताक में रहता था कि कब चोरी करने का मौका मिले।

25. लोहे के चने चबाना – भारी कठिनाई में डालना।
प्रयोग – भारतीय सैनिकों ने शत्रु को लोहे के चने चबवा दिये।

26. धमा-चौकड़ी – ऊधम मचाना।
प्रयोग – अध्यापक की अनुपस्थिति में छात्रों ने खूब धमा चौकड़ी मचाई।

27. मुँह की खाना – बुरी तरह हारना।
प्रयोग – हर बार पाकिस्तान ने मुँह की खाई है।

28. तलवार की भेंट होना – लड़ते-लड़ते मरना।
प्रयोग – युद्ध में पंजाब के बहुत-से जवान तलवार की भेंट चढ़ गए।

29. नाकों चने चबाना – खूब तंग करना।
प्रयोग – सुभाष चन्द्र बोस जैसे वीरों ने अंग्रेज़ी सेना से टक्कर लेकर उनको नाकों चने चबवा दिए थे।

30. नानी याद आना – संकट में पड़ना, घबराना।
प्रयोग – जब भीम की गदा की चोटें दुर्योधन को सहनी पड़ी तो उसे नानी याद आ गई।

31. नौ-दो ग्यारह होना – भाग जाना।
प्रयोग – सिपाही को देखते ही चोर नौ-दो ग्यारह हो गया।

32. पानी-पानी होना – बहुत लज्जित होना।
प्रयोग – सच्चाई सामने आने पर बलदेव पानीपानी हो गया।

33. लाल-पीला होना – क्रुद्ध होना, गुस्से में आना।
प्रयोग – पहले बात तो सुन लो, व्यर्थ में क्यों लाल-पीले हो रहे हो ?

34. लोहा-लेना – युद्ध करना।
प्रयोग – अधिकतर मुग़ल सम्राट राजपूतों से लोहा नहीं लेना चाहते थे।

35. टूट पड़ना – एकदम हमला करना।
प्रयोग – शत्रु-सेना को देखते ही भारतीय सैनिक उन पर टूट पड़े।

36. हवा से बातें करना – बहुत तेज़ दौड़ना।
प्रयोग – महाराणा प्रताप का घोड़ा हवा से बातें करता था।

37. सुख-दुःख का साथी – हमेशा साथ देने वाला।।
प्रयोग – अच्छा मित्र सुख-दुःख का साथी होता

38. शोक के बादल छाना – गहरा दुःख आना।
प्रयोग – इकलौते पुत्र की मृत्यु पर सारे परिवार में शोक के बादल छा गए।

39. प्रसन्नता की लहर दौड़ना – सब ओर खुशी छाना।
प्रयोग – मैच में जीत पर सारे शहर में प्रसन्नता की लहर दौड़ गई।

40. जान हथेली पर लेना – भारी मुसीबत सहना।
प्रयोग – सैनिक जान हथेली पर लेकर सीमा की रक्षा करते हैं।

41. चार चाँद लगाना – भारतीय सैनिकों ने कारगिल में शत्रु को खदेड़ कर वीरता को चार चाँद लगा दिए।
मन उचाट होना – विद्यार्थी को पढ़ाई से मन उचाट नहीं करना चाहिए।

42. मुँह दिखाना – रमेश ने चोरी क्या की, अब उसे मित्रों को भी मुँह दिखाना कठिन हो गया।
वीरगति को प्राप्त होना – कारगिल युद्ध में सैंकड़ों जवान वीरगति को प्राप्त हो गए।

Punjab State Board PSEB 5th Class English Book Solutions Chapter 9 Safe Circle Textbook Exercise Questions and Answers.

PSEB Solutions for Class 5 English Chapter 9 Safe Circle

I. Let’s Answer

(A) Answer the following questions:

Question 1.
How old was Sonia ?
(ਸੋਨੀਆ ਕਿੰਨੇ ਸਾਲ ਦੀ ਸੀ ?)
Answer:
Sonia was six years old.

Question 2.
Why was Sonia sad ?
(ਸੋਨੀਆ ਉਦਾਸ ਕਿਉਂ ਸੀ ?)
Answer:
Sonia was sad because she could not catch the butterfly.

Question 3.
Why did the butterfly fly away ?
(ਤਿਤਲੀ, ਕਿਉਂ ਉੱਡ ਗਈ ?)
Answer:
Sonia made the butterfly uncomfortable.

Question 4.
What is a bad touch ?
(ਬੁਰਾ ਛੂਹਣਾ ਕੀ ਹੁੰਦਾ ਹੈ ?)
Answer:
The touch that makes us feel uncomfortable is a bad touch.

Question 5.
Which is a safe circle ?
(ਸੁਰੱਖਿਅਤ ਦਾਇਰਾ ਕਿਹੜਾ ਹੁੰਦਾ ਹੈ ?)
Answer:
A safe circle is a group of people who care for us and with whom we feel comfortable.

(B) Make sentences with the following words:

1. butterfly : …………..
2. daughter : …………….
3. body : …………..
4. teacher : …………..
5. happy : ……………
Answer:
1. butterfly : I saw a butterfly in the garden.
2. daughter : She has one daughter.
3. body : Name any five body parts.
4. teacher : Sh. Mohan Lal is our science teacher.
5. happy : I am very happy today.

(C) Choose the correct option and write the answer in the space given below:

Question 1.
Sonia tried to catch:
(a) a dog.
(b) a butterfly.
(C) a bird.
Answer:
(b) a butterfly.

Question 2.
If someone touches us and we feel bad:
(a) that is a normal touch.
(b) that is a good touch.
(c) that is a bad touch.
Answer:
(c) that is a bad touch.

Question 3.
When mother hugs Sonia:
(a) she feels comfortable and good.
(b) she hates it.
(c) she feels bad.
Answer:
(a) she feels comfortable and good.

Question 4.
If somebody makes me uncomfortable:
(a) I would go with him.
(b) I would run to my safe circle.
(c) I would talk with him.
Answer:
(b) I would run to my safe circle.

II. Vocabulary

(A) Read the following words. Underline the words which come under the title Public Places and write them in the space given:

money, driver, computer, hospital, students, bank, zoo, pilot, park, player, airport, market, office, teacher, college, marriage, school, bus-stand, animals, garden
Answer:
Public Places:
bus-stand, hospital, office, zoo, market, school, airport, garden, bank, college.

(B) Correct the spellings:

1. sefe
2. houes
3. betterfly
4. Sonea
5. circal
6. daughtar
7. mather
8. touche
9. gud
10. teecher
Answer:
1. safe
2. house
3. butterfly
4. Sonia
5. circle
6. daughter
7. mother
8. touch
9. good
10. teacher

(C) Contraction:

Read the following (short forms):

I will – I’ll
I am – I’m
Does not – Doesn’t
You are – You’re
Let us – Let’s
We will – We’ll
Have not – Haven’t
Could not – Couldn’t
Should not – Shouldn’t
Is not – isn’t
She will – She’ll
I have – I’ve

ਨੋਟ – ਇਨ੍ਹਾਂ ਨੂੰ ਧਿਆਨ ਨਾਲ ਪੜ੍ਹੋ ਅਤੇ ਇਨ੍ਹਾਂ ਦਾ ਪ੍ਰਯੋਗ ਕਰਨਾ ਸਿੱਖੋ ।

नोट : इन्हें ध्यान से पढ़ें और इनका प्रयोग करना सीखें।

III. Language Corner

(A) Write the plural form of the noun in the space and complete the sentence:

1. I brush my …………… ………….. everyday. (tooth)
2. The ……………………… are running (mouse)
3. …………….. are dancing. (child)
4. A few ……………………. went inside the room. (man)
5. Many …………………………… were injured. (person)
6. The …………………….. are shining in the sky. (star)
Answer:
1. teeth
2. mice
3. Children
4. men
5. persons
6. stars.

(B) Find out the adjectives from the story. Write comparative and superlative degree:

Adjective	Comparative degree	Superlative degree
1. beautiful	more beautiful	most beautiful
2. comfortable	————	———–
3. good	————	————
4. bad	———-	———–
5. wise	———-	———–
6. intelligent	———-	———–
7. happy	———–	————

Answer:

Adjective	Comparative degree	Superlative degree
1. beautiful	more beautiful	most beautiful
2. comfortable	more comfortable	most comfortable
3. good	better	best
4. bad	worse	worst
5. wise	wiser	wisest
6. intelligent	more intelligent	most intelligent
7. happy	happier	happiest

(C) Write two adjectives to describe each of the following nouns:

1. ice cream – ………….. , …………….
2. dress – ……………… , …………….
3. book – …………… , …………….
4. stone – ………….. , …………….
5. hair – ……………. , …………….
6. building – ……………. , …………….
Answer:
1. ice cream – sweet, tasty
2. dress – beautiful, nice
3. book – story, new
4. stone – white, polished
5. hair – black, grey
6. building – big, tall

(D) Substitution table:

Make sentences using correct form of verb and write in your notebook.

Answer:
I play football.
I read newspaper daily.
I go for a morning walk.

They play football.
They read newspaper daily.
They go for a morning walk.

She plays football.”
She reads newspaper daily.
She goes for a morning walk.

We play football.
We read newspaper daily.
We go for a morning walk.

You play football.
You read newspaper daily.
You go for a morning walk.

(E) Conjunctions:

The words which are used to join two words, groups of words, sentences or phrases are called conjunctions.

ਦੋ ਸ਼ਬਦਾਂ, ਸ਼ਬਦ ਸਮੂਹਾਂ, ਵਾਕਾਂ ਜਾਂ ਵਾਕਾਂਸ਼ਾਂ ਨੂੰ ਆਪਸ ਵਿਚ ਜੋੜਨ ਵਾਲੇ ਸ਼ਬਦ Conjunctions ਕਹਾਉਂਦੇ ਹਨ । ਇਨ੍ਹਾਂ ਨੂੰ Joining Words ਵੀ ਕਿਹਾ ਜਾਂਦਾ ਹੈ ।

दो शब्दों, शब्द समूहों, वाक्यों या वाक्यांशों को आपस में जोड़ने वाले शब्द Conjunctions कहलाते हैं। इन्हें joining words भी कहा जाता हैं।
e.g. Raj and Manjit are friends.
Wait till he comes.
I am tall but he is short.

(F) Underline the joining words in the following sentences:

1. You are wise but he is an idiot.
2. Ravi is fat as well as lazy.
3. Minu and Aman are friends.
4. Sudesh is rich and nice.
5. Montu can’t go to school because he is ill.
6. Man proposes but God disposes.
7. Run fast or you will miss the train.
8. He is weak still he is doing well.
9. We eat so that we can stay healthy.
10. They are playing and shouting.

(G) Fill in the blanks with the conjunctions given in the box:

as well as, but, because, or, unless, for, and, so, still, until

1. I have two cows ………….. a goat.
2. I want to thank her …………….. the beautiful gift.
3. Renu is tall …………….. her sister is short.
4. He went to the doctor ………………. he was sick.
5. Wait here ……………….. I get back.
6. He is rich …………. honest
7. The girl has lost her book, …………. she is crying.
8. You will not score good marks …………….. you work hard.
9. He is poor …………. he is happy.
10. Will you take tea …………………… coffee?
Answer:
1. and
2. for
3. but
4. because
5. until
6. as well as
7. so
8. unless
9. still
10. or.

(H) Read the underlined words and put them in their respective column:

I have a beautiful doll. I call it fairy. It has blue eyes. Vipan, my brother has bought it for me from Chandigarh. My family also likes it very much. I play with it. I comb its hair very softly.

IV. Let’s Read

Read aloud the following words:

chain
peach
chart
reach
check
beach
chest
teach
chair:
touch
chill
branch
cheat
march
cheap
much
chalk
lunch
chief

ਨੋਟ – ਆਪਣੇ ਅਧਿਆਪਕ ਦੀ ਸਹਾਇਤਾ ਨਾਲ ਵਿਦਿਆਰਥੀ ਆਪਣੇ ਆਪ ਕਰਨ ।

नोट-अपने teacher की सहायता से विद्यार्थी स्वयं करें।

V. Apostrophe(‘s)

ਨੋਟ – ਕਿਸੇ Noun ਦੇ ਨਾਲ ’s ਦਾ ਪ੍ਰਯੋਗ ਉਸਦੇ ਅਧਿਕਾਰ ਨੂੰ ਪ੍ਰਗਟ ਕਰਦਾ ਹੈ ।

VI. Let’s Write

Write a few lines on ‘My Teacher’:

MY TEACHER

Sh./Smt. ………….. is my teacher. He/She is an M.A., B.Ed. He/She is 40 years old. He/She is very tall and handsome. He/She gets up early. Hel She goes for a walk. He/She comes to school in time. He/She works very hard. He/She teaches us English. He/She is a good player. He/She is a fine singer. He/She is good to all. He/She helps the poor students. I am proud of him/her.

VII. Value I Learnt (ਮੁੱਲ ਬੋਧ)

If any stranger is making you uncomfortable what will you do ? Tick (✓) all the correct options:
1. run away from that place
2. shout loudly
3. talk to the stranger
4. will not react
5. talk to your parents
6. talk to your teacher
7. will not disclose to anyone
Answer:
1. (✓)
2. (✓)
3. (✗)
4. (✗)
5. (✓)
6. (✓)
7. (✗)

VIII. Activity

Write names and paste pictures of your safe circle.

Answer:

ਨੋਟ – ਬੱਚੇ ਇੱਥੇ ਆਪਣੇ ਮਾਤਾ-ਪਿਤਾ, ਅਧਿਆਪਕ/ਅਧਿਆਪਕਾ, ਦਾਦਾ-ਦਾਦੀ ਅਤੇ ਨਾਨਾ-ਨਾਨੀ ਦੇ ਚਿੱਤਰ ਲਗਾਉਣ ।

Note : बच्चे यहाँ अपने माता-पिता, अध्यापक/अध्यापिका, दादा-दादी तथा नाना-नानी के चित्र लगाएं।

Pre-Reading

Question 1.
Do you feel safe in the company of your parents or alone?
Answer:
I feel safe in the company of my parents.

Question 2.
When do you feel unsafe ?
Answer:
I feel unsafe when I am alone at home.

Question 3.
Do you know what is good or bad touch ?
Answer:
Yes, my mother has told me everything about this.

Word-Meanings

Word/Phrase	Meaning in English	Meaning in Punjabi
Disappointed	very sad/upset	ਉਦਾਸ ਨਿਰਾਸ਼
Explain	give details	ਸਮਝਾਉਣਾ/ਵਿਆਖਿਆ ਕਰਨਾ
Backyard	open area at the back of the house	ਘਰ ਦੇ ਪਿੱਛੇ ਦਾ ਵਿਹੜਾ
Uncomfortable	not feeling easy	ਤਕਲੀਫ਼ ਪਹੁੰਚਾਉਣਾ/ਪਰੇਸ਼ਾਨ ਕਰਨਾ
Consists	is made of	ਬਣਿਆ ਹੋਇਆ
Touch	contact/feel	ਛੁਹਣਾ/ਸਪਰਸ਼ ਕਰਨਾ
Belongs	is attached to	ਨਾਲ ਸੰਬੰਧਿਤ
Similarly	in the same way	ਇਸ ਪ੍ਰਕਾਰ
Remember	bear in mind	ਯਾਦ ਰੱਖਣਾ
Of course	certainly	ਬਿਨਾਂ-ਸ਼ੱਕ
Intelligent	understanding	ਸਮਝਦਾਰ

Word/Phrase	Meaning in English	Meaning in Hindi
Disappointed	very sad/upset	उदास/निराश
Explain	give details	समझाना
Backyard	open area at the back of the house	घर के पीछे का आंगन
Uncomfortable	not feeling easy	बेचैन/परेशान
Consists	is made of	बना होना
Touch	contact/feel	छूना/स्पर्श
Belongs	is attached to	से संबंधित
Similarly	in the same way	इसी प्रकार
Remember	bear in mind	याद रखना
Of course	certainly	नि:संदेह
Intelligent	understanding	समझदार

Safe Circle Summary in Punjabi

ਛੇ ਸਾਲ ਦੀ ਇਕ ਲੜਕੀ ਸੋਨੀਆ ਘਰ ਦੇ ਪਿੱਛੇ ਵਿਹੜੇ ਵਿਚ ਖੇਡ ਰਹੀ ਸੀ । ਉਸਨੇ ਇਕ ਸੁੰਦਰ ਤਿੱਤਲੀ ਦੇਖੀ । ਉਸਨੇ ਉਸਨੂੰ ਪਕੜਣ ਦੀ ਕੋਸ਼ਿਸ਼ ਕੀਤੀ, ਪਰੰਤੂ ਪਕੜ ਨਹੀਂ ਸਕੀ ਅਤੇ ਉਦਾਸ ਹੋ ਗਈ । ਉਹ ਆਪਣੀ ਮੰਮੀ ਦੇ ਕੋਲ ਆ ਗਈ ।

Safe Circle Summary in Hindi

छ: वर्ष की एक लड़की सोनिया घर के पीछे आंगन में खेल रही थी। उसने एक सुन्दर तितली देखी। उसने उसे पकड़ने की कोशिश की परन्तु पकड़ नहीं सकी और निराश हो गई। वह अपनी मम्मी के पास आ गई।