Bhagya

Punjab State Board PSEB 6th Class Maths Book Solutions Chapter 5 Fractions Ex 5.5 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 6 Maths Chapter 5 Fractions Ex 5.5

1. Add the following:

Question (i)

Solution:

2. Subtract the following:

Question (i)

Solution:

3. Simplify the following:

Question (i)

Solution:

4. An iron pipe of length \(6 \frac{2}{3}\) metres long was cut into two pieces. One piece is \(4 \frac{3}{7}\) metre long. What is the length of other pieces?
Solution:
Total length of an iron pipe
= \(6 \frac{2}{3}\) m = \(\frac{20}{3}\) metre
Length of one piece
= \(4 \frac{3}{7}\) metre = \(\frac{31}{7}\) metre
∴ Length of other piece

5. Ashok bought \(\frac{7}{10}\)kg of mangoes and Taran \(\frac{11}{15}\)kg of apples. How much fruit did he buy in all?
Solution:

6. Avi did \(\frac{3}{5}\) of his homework on Saturday and \(\frac{1}{10}\) of the same homework on Sunday. How much of the homework did he do over the weekend?
Solution:
Homework he did weekend
\(\frac{3}{5}+\frac{1}{10}=\frac{3 \times 2}{5 \times 2}+\frac{1}{10}=\frac{6}{10}+\frac{1}{10}\)
∴ Home work done in weekend
\(\frac{6+1}{10}=\frac{7}{10}\)

7. Charan spent \(\frac {1}{4}\) of his pocket money on a movie and \(\frac {3}{8}\) on a new pen and \(\frac {1}{8}\) on a pencil. What fraction of his pocket money did he spend?
Solution:
Total money he spent
= \(\frac{1}{4}+\frac{3}{8}+\frac{1}{8}=\frac{1}{4} \times \frac{2}{2}+\frac{3}{8}+\frac{1}{8}\)
= \(\frac{2}{8}+\frac{3}{8}+\frac{1}{8}=\frac{2+3+1}{8}\)
= \(\frac{6}{8}=\frac{3}{4}\)
So, pocket money spent = \(\frac {3}{4}\)

8. Simar lives at a distance of 4 km from the school. Prabhjot lives at a distance of \(\frac {2}{3}\) km less than Simar’s distance from the school. How far does Prabhjot live from the school?
Solution:
Distance of Simar from school = 4 km Distance of Prabhjot from school

Punjab State Board PSEB 7th Class Maths Book Solutions Chapter 11 Perimeter and Area Ex 11.3 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 7 Maths Chapter 11 Perimeter and Area Ex 11.3

1. Find the circumference of circle whose
(i) Radius (r) = 21 cm
(ii) Radius (r) = 3.5 cm
(iii) Diameter = 84 cm
Solution:
(i) Given radius (r) = 21 cm
circumference of circle = 2πr
= 2 × \(\frac {22}{7}\) ×21
= 132 cm

(ii) Given radius (r) = 3.5 cm
Circumference = 2πl
= 2 × \(\frac {22}{7}\) × 3.5
= 22 cm

(iii) Given Diameter (d) = 84 cm
radius (r) = \(\frac{d}{2}=\frac{84}{2}\)
= 42 cm
Circumference = 2πr
= 2 × \(\frac {22}{7}\) × 42
= 264 cm

2. If the circumference of a circular sheet is 176 m, find its radius.
Solution:
Given circumference of circular sheet = 176 m
Let radius = r
So 2πr = 176
r = \(\frac{176}{2 \pi}\)
\(\frac{176}{2 \times \frac{22}{7}}\)
= 28 m

3. A circular disc of diameter 8.4 cm is divided into two parts what is the perimeter of each semicircular part ?
Solution:
Given diameter of a circular disc = 8.4 cm
radius (r) = \(\frac{8.4}{2}\) = 4.2 cm
Perimeter of semicircular part = πr + 2r
= \(\frac {22}{7}\) × 4.2 + 2 × 4.2
= 22 × 0.6 + 8.4
= 21.6 cm

4. Find the area of the circle having
(i) Radius r = 49 cm
(ii) Radius r = 2.8 cm
(iii) Diameter = 4.2 cm
Solution:
(i) Given radius (r) = 49 cm
Area of circle = πr²
= \(\frac {22}{7}\) × 49 × 49
= 7546 cm²

(ii) Given radius (r) = 2.8 cm
Area of circle = πr²
= \(\frac {22}{7}\) × 2.8 × 2.8
= 24.64 cm²

(iii) Given diameter (d) = 4.2 cm
radius (r) = \(\frac{d}{2}=\frac{4.2}{2}\)
Area of circle = πr²
= \(\frac {22}{7}\) × 2.1 × 2.1
= 13.86 cm²

5. A gardener wants to fence a circular garden of radius 15 m. Find the length of wire, if he makes three rounds offense. Also, find the cost of wire if it costs ₹ 5 per meter (Take π = 3.14).
Solution:
Given radius of circular garden (r) = 15 m
Circumference of the circular garden = 2πr
= 2 × 3.14 × 15
= 94.2 m
So, length of the wire to make three rounds offense
= 3 × 94.2
= 282.6 cm
Cost of wire= ₹ 5 × 282.6
= ₹ 1413

6. Which of the following has larger area and by how much ?
(a) Rectangle with length 15 cm and breadth 5.4 cm
(b) Circle of diameter 5.6 cm.
Solution:
(a) Given length of rectangle = 15 cm
breadth = 5.4 cm
Area of rectangle = length × breadth
= 15 × 5.4
= 81 cm2

(b) Given diameter of circle (d) = 5.6 cm
radius (r) = \(\frac{d}{2}=\frac{5.6}{2}\)
= 2.8 cm
Area of the circle = πr²
= \(\frac {22}{7}\) × (2.8)²
= 24.64 cm²
Hence, Rectangle has more area = 81 – 24.64
= 56.36 cm²

7. From a rectangular sheet of length 15 cm and breadth 12 cm a circle of radius 3.5 cm is removed. Find the area of remaining sheet.
Solution:
Given length of rectangular sheet = 15 cm
Breadth of rectangle sheet = 12 cm
Area of rectangular sheet = length × breadth
= 15 × 12
= 180 cm²
Given radius of circle (r) = 3.5 cm
Area of circle = πr²
= \(\frac {22}{7}\) × (3.5)²
= 38.5 cm²
Since circle is removed from rectangular sheet.
So, area of remaining sheet = Area of rectangular Sheet – Area of circle
= 180 – 38.5
= 141.5 cm2

8. From a circular sheet of radius 7 cm, a circle of radius 2.1 cm is removed, find the area of remaining sheet.
Solution:
Radius of the circular sheet = 7 cm
Area of the circular sheet = 1 cm
= πr2 = \(\frac {22}{7}\) × 7 × 7 cm²
=154 cm²
Radius of the circle = 2.1 cm
Area of the circle
\(\frac {22}{7}\) × 2.1 × 1.1 = \(\frac{22}{7} \times \frac{21}{10} \times \frac{21}{10}\)
= \(\frac {1386}{100}\)
= 13.86 cm²
Area of the remaining sheet = 154 cm² – 13.86 cm²
= 140.14 cm²

9. Smeep took a wire of length 88 cm and bent it into the shape of a circle, find the radius and area of the circle. If the same wire is bent into a square, what will be the side of the square ? Which figure encloses more area ?
Solution:
Given length of wire = 88 cm
The wire is bent into the shape of circle.
Circumference of circle = length of the
2πr = 88
r = \(\frac{88}{2 \pi}=\frac{44}{\pi} \mathrm{cm}\)
= 14 cm
Area of the circle = πr²
= π × (14)²
= \(\frac {22}{7}\) × 14 × 14
= 616 cm²
If the same wire is bent into the square
Let side of the square = a
Perimeter of square = length of the wire
4 × a = 88
a = \(\frac {88}{4}\)
= 22 cm
Area of the square = (side)²
= (22)²
= 484 cm²
Hence circle enclosed more area.

10. A garden is 120 m long and 85 m broad. Inside the garden, there is a circular pit of diameter 14 m. Find the cost of planting the remaining part of the garden at the rate of ₹ 5.50 per square meter.
Solution:
Given length of garden = 120 m
Breadth of garden = 85 m
Area of garden = length × breadth
= 120 × 85
= 10200 m²
Given diameter of circular pit (d) = 14 m
radius (r) = \(\frac{d}{2}=\frac{14}{2}\)
= 7 m
Area of circular pit = πr²
= \(\frac {22}{7}\) × 7 × 7
= 154 m²
Remaining part of garden = Area of garden for planting – Area of circular pit
= 10200 – 154
= 10046 m²
Cost of planting the remaining part of the garden
= ₹ 5.50 × 10046
= ₹ 55243

11. In the figure PQ = QR and PR = 56 cm. The radius of inscribed circle is 7 cm. Q is centre of semicircle. What is the area of shaded region ?

Solution:
Given PQ = QR
PR = 56 cm
Radius of inscribed circle = 7 cm
So PR = PQ + QR
= PQ + PQ = 2PQ
Hence, PQ = \(\frac{\mathrm{PR}}{2}=\frac{56}{2}\)
= 28 cm
So QR = PQ = 28 cm
Area of shaded region = Area of semicircle of diameter PR – Area of semicircle of diameter PQ – Area of semicircle of diameter QR – Area of inscribe circle

12. The minute hand of a circular clock is 18 cm long. How far does the tip of minute hand move in one hour ?
Solution:
Given minute hand of a circular clock = 18 cm
Distance covered by minute hand in 1 hour = 2πr
= 2 × 3.14 × 18
= 2 × \(\frac {314}{100}\) × 18
= \(\frac {11304}{100}\)
= 113.04 cm.

13. Multiple choice questions :

Question (i).
The circumference of a circle of diameter 10 cm is :
(a) 31.4 cm
(b) 3.14 cm
(c) 314 cm
(d) 35.4 cm
Answer:
(a) 31.4 cm

Question (ii).
The circumference of a circle with radius 14 cm is :
(a) 88 cm
(b) 44 cm
(c) 22 cm
(d) 85 cm
Answer:
(a) 88 cm

Question (iii).
What is the area of the circle of radius 7 cm ?
(a) 49 cm
(b) 22 cm²
(c) 154 cm²
(d) 308 cm²
Answer:
(c) 154 cm²

Question (iv).
Find the diameter of a circle whose area is 154 cm² ?
(a) 4 cm
(b) 6 cm
(c) 14 cm
(d) 12 cm
Answer:
(c) 14 cm

Question (v).
A circle has area 100 times the area of another circle. What is the ratio of their circumferences ?
(a) 10 : 1
(b) 1 : 10
(c) 1 : 1
(d) 2 : 1
Answer:
(a) 10 : 1

Question (vi).
Diameter of a circular garden is 9.8 cm. Which of the following is its area ?
(a) 75.46 cm²
(b) 76.46 cm²
(c) 74.4 cm²
(d) 76.4 cm²
Answer:
(a) 75.46 cm²

Punjab State Board PSEB 6th Class Maths Book Solutions Chapter 5 Fractions Ex 5.3 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 6 Maths Chapter 5 Fractions Ex 5.3

1. Write the fraction for the shaded part and check whether these fractions are equivalent or not?

Question (i)

Solution:

2. Find four equivalent fractions of the followings:

Question (i)
(i) \(\frac {1}{4}\)
(ii) \(\frac {3}{5}\)
(iii) \(\frac {7}{9}\)
(iv) \(\frac {5}{11}\)
(v) \(\frac {2}{3}\)
Solution:

3. Write the lowest equivalent fraction (simplest form) of :

Question (i)
(i) \(\frac {10}{25}\)
(ii) \(\frac {27}{54}\)
(iii) \(\frac {48}{72}\)
(iv) \(\frac {150}{60}\)
(v) \(\frac {162}{90}\)
Solution:

4. Are the following fractions equivalent or not?

Question (i)
\(\frac{5}{12}, \frac{25}{60}\)
Solution:
We have

By cross product,
5 × 60 = 300 and 12 × 25 = 300
Since two cross products are same
So, the given fractions are equivalent.

Question (ii)
\(\frac{6}{7}, \frac{36}{42}\)
Solution:
We have

By cross product,
6 × 42 = 252 and 7 × 36 = 252
Since two cross products are same
So, the given fractions are equivalent.

Question (iii)
\(\frac{7}{9}, \frac{56}{72}\)
Solution:
We have

By cross product,
7 × 72 = 504 and 9 × 56 = 504
Since two cross products are same
So, the given fractions are equivalent.

5. Replace [ ] 1 in each of the following by the correct number.

Question (i)
\(\frac{2}{7}\) = 12 / [ ]
Solution:
Observe the numerators we have 12 ÷ 2 = 6
So, we multiply both numerator and denominator of \(\frac {2}{7}\) by 6
We get \(\frac{2}{7}=\frac{2 \times 6}{7 \times 6}=\frac{12}{42}\)
Hence, the correct number in [ ] 1 is 42

Question (ii)
\(\frac{5}{8}\) = 35 / [ ]
Solution:
Observe the numerators we have 35 ÷ 5 = 7
So, we multiply both numerator and denominator of \(\frac {5}{8}\) by 7
We get \(\frac{5}{8}=\frac{5 \times 7}{8 \times 7}=\frac{35}{56}\)
Hence, the correct number in [ ] is 56.

Question (iii)
\(\frac{24}{36}\) = 6 / [ ]
Solution:
Observe the numerators we have 24 ÷ 6 = 4
So, we divide both numerator and denominator of \(\frac {24}{36}\) by 4
We get \(\frac{24}{36}=\frac{24 \div 4}{36 \div 4}=\frac{6}{9}\)
Hence, the correct number in [ ] is 9

Question (iv)
\(\frac{30}{48}\) = 8 / [ ]
Solution:
Observe the denominators we have 48 ÷ 8 = 6
So, we divide both numerator and denominator of \(\frac {30}{48}\) by 6
We get \(\frac{30}{48}=\frac{30 \div 6}{48 \div 6}=\frac{5}{8}\)
Hence, the correct number in ⊇ is 5

Question (v)
\(\frac{7}{4}\) = 42 / [ ]
Solution:
Observe the numerators we have 42 ÷ 7 = 6
So, we multiply both numerator and denominator of \(\frac {7}{4}\) by 6
We get \(\frac{7}{4}=\frac{7 \times 6}{7 \times 6}=\frac{42}{24}\)
Hence, the correct number in [ ] is 24

6. Find the equivalent fraction of \(\frac {3}{5}\), having

Question (i)
numerator 18
Solution:
(i) Equivalent fraction of \(\frac {3}{5}\), having numerator 18 is
\(\frac{3}{5}\) = 18 / [ ]
Observe the numerators, we have 18 ÷ 3=6
So, we multiply both numerator and denominator of \(\frac {3}{5}\) by 6
∴ \(\frac{3}{5}=\frac{3 \times 6}{5 \times 6}=\frac{18}{30}\)
Thus, required equivalent fraction of \(\frac{3}{5}=\frac{18}{30}\)

Question (ii)
denominator 20
Solution:
Equivalent fraction of \(\frac {3}{5}\), having denominator 20 is \(\frac{3}{5}\) = [ ] / 20
Observe the denominators, we have 20 ÷ 5 = 4
So, we multiply both numerator and denominator of \(\frac {3}{5}\) by 4
Thus, required equivalent fraction of \(\frac{3}{5}=\frac{12}{20}\)
∴ \(\frac{3}{5}=\frac{3 \times 4}{5 \times 4}=\frac{12}{20}\)
Thus, required equivalent fraction of
\(\frac{3}{5}=\frac{12}{20}\)

Question (iii)
numerator 24.
Solution:
Equivalent fraction of \(\frac {3}{5}\) , having numerator 24 is
\(\frac{3}{5}\) = 24 / [ ]
Observe the numerators, we have 24 ÷ 3 = 8
So, we multiply both numerator and denominator of \(\frac {3}{5}\) by 8
∴ \(\frac{3}{5}=\frac{3 \times 8}{5 \times 8}=\frac{24}{40}\)
Thus, required equivalent fraction of
\(\frac{3}{5}=\frac{24}{40}\)

7. Find the equivalent fraction of \(\frac {24}{40}\), having

Question (i)
(i) numerator 6
(ii) numerator 48
(iii) denominator 20
Solution:
(i) Equivalent fraction of \(\frac {24}{40}\), numerator 6 is
\(\frac{24}{40}\) = 6 / [ ]
Observe the numerators, we have 24 ÷ 6 = 4
So, we divide both numerator and denominator of \(\frac {24}{40}\) by 4
∴ \(\frac{24}{40}=\frac{24 \div 4}{40 \div 4}=\frac{6}{10}\)
Thus, required equivalent fraction of
\(\frac{24}{40}=\frac{6}{10}\)

Question (ii)
numerator 48
Solution:
Equivalent fraction of \(\frac {24}{40}\), having numerator 48 is
\(\frac{24}{40}\) = 48 / [ ]
Observe the numerators, we have 48 ÷ 24 = 2
So, we multiply both numerator and denominator of \(\frac {24}{40}\) by 2
∴ \(\frac{24}{40}=\frac{24 \times 2}{40 \times 2}=\frac{48}{80}\)
Thus, required equivalent fraction of
\(\frac{24}{40}=\frac{48}{80}\)

Question (iii)
denominator 20
Solution:
Equivalent fraction of \(\frac {24}{40}\), having denominator 20 is
\(\frac{24}{40}\) = [ ] / 20
Observe the denominators, we have 40 ÷ 20 = 2
So, we divide both numerator and denominator of \(\frac {24}{40}\) by 2
\(\frac{24}{40}=\frac{24 \div 2}{40 \div 2}=\frac{12}{20}\)
∴ Thus, required equivalent fraction of
\(\frac{24}{40}=\frac{12}{20}\)

Punjab State Board PSEB 9th Class Maths Book Solutions Chapter 9 Areas of Parallelograms and Triangles Ex 9.1 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 9 Maths Chapter 9 Areas of Parallelograms and Triangles Ex 9.1

Question 1.
Which of the following figures lie on the same base and between the same parallels. In such a case, write the common base and the two parallels.

(i)

Answer:
In figure (i), trapezium ABCD and ∆ PDC lie on the same base and between the same parallels.
Here, DC is the common base and DC and AB are two parallels.

(ii)

Answer:
In figure (ii), no two figures lie on the same base and between the same parallels.

(iii)

Answer:
In figure (iii), parallelogram PQRS and ∆ TQR lie on the same base and between the same parallels.
Here, QR is the common base and QR and PS are two parallels.

(iv)

Answer:
In figure (iv), no two figures lie on the same base and between the same parallels.

(v)

Answer:
In figure (v), parallelograms ABCD and APQD as well as trapeziums ABQD and APCD, all the four figures, lie on the same base and between the same parallels.
Here, AD is the common base and AD and BQ are two parallels.

(vi)

Answer:
In figure (vi), no two figure lie on the same base and between the same parallels.

Punjab State Board PSEB 12th Class Hindi Book Solutions Hindi Grammar alankar अलंकार Exercise Questions and Answers, Notes.

PSEB 12th Class Hindi Grammar अलंकार

प्रश्न-पत्र में अलंकारों के लक्षण तथा उदाहरण भी पूछे जाएँगे। अलंकारों के भेदोपभेद अथवा उन पर तुलनात्मक प्रश्न पूछे जाएँगे। यहाँ प्रत्येक अलंकार का लक्षण, उदाहरण तथा स्पष्टीकरण दिया गया है।

‘अन्य उदाहरण’ शीर्षक के अन्तर्गत कुछ और उदाहरण भी दिए गए हैं। विद्यार्थी अपनी सुविधा एवं रुचि के अनुसार कोई भी उदाहरण कंठस्थ कर सकता है।

आरम्भ में अलंकार का महत्त्व, लक्षण तथा उसके भेदों का भी उल्लेख कर दिया गया है। अलंकार का महत्व-अलंकार का साधारण अर्थ है-अलंकृत करना, शोभा बढ़ाना। लोक-भाषा में अलंकार का अर्थ गहना अथवा आभूषण है। जिस प्रकार स्त्रियाँ अपने सौन्दर्यवर्द्धन के लिए भिन्न-भिन्न प्रकार के गहनों का प्रयोग करती हैं उसी प्रकार कवि भिन्न-भिन्न प्रकार के अलंकारों के प्रयोग द्वारा कविता-कामिनी की शोभा बढ़ाते हैं। कंगन से हाथ की, कुण्डल से कान की और हार से गले की शोभा बढ़ती है और ये मिलकर कामिनी के शरीर को सौन्दर्य और आकर्षण प्रदान करते हैं। ठीक इसी प्रकार अनुप्रास, उपमा आदि अलंकारों का प्रयोग कविता को आकर्षक और प्रभावशाली बना देता है। कभी शब्द-विशेष का प्रयोग कविता को चमत्कार प्रदान करता है तो कभी अर्थ का प्रयोग कविता के भाव में उत्कर्ष ला देता है।

उपर्युक्त संक्षिप्त विवेचन के उपरान्त अलंकार की परिभाषा इस प्रकार दी जा सकती है-
परिभाषा-“शब्द और अर्थ में चमत्कार उत्पन्न कर कविता की शोभा बढ़ाने वाले तत्त्व को अलंकार कहते है।

अलंकार के भेद-अलंकार के मुख्य रूप में दो भेद हैं-
1. शब्दालंकार
2. अर्थालंकार

1. शब्दालंकार-जहाँ शब्दों के कारण किसी रचना में चमत्कार उत्पन्न हो, वहाँ शब्दालंकार होता है। अनुप्रास, यमक, श्लेष, वक्रोक्ति आदि शब्दालंकार हैं।

2. अर्थालंकार-जहाँ अर्थों के कारण किसी रचना में चमत्कार उत्पन्न हो वहाँ अर्थालंकार होता है। उपमा, रूपक आदि प्रसिद्ध अर्थालंकार हैं।

शब्दालंकार और अर्थालंकार में अन्तर

स्पष्ट किया जा चुका है कि शब्द के कारण चमत्कार उत्पन्न होने पर शब्दालंकार और अर्थ के कारण चमत्कार उत्पन्न होने पर अर्थालंकार होता है।

शब्दालंकार में शब्द-विशेष के निकाल देने पर चमत्कार समाप्त हो जाता है अर्थात् शब्द-विशेष के स्थान पर समानार्थी शब्द भी चमत्कार को बनाए रखने में असमर्थ हैं।

जैसे–’पानी के बिना मोती का कोई महत्त्व नहीं’ यहाँ मोती के पक्ष में ‘पानी’ का अर्थ ‘चमक’ है। अब इसके स्थान पर समानार्थी शब्द जल, नीर, अम्बु, तोय आदि शब्दों को रख दें तो अलंकार समाप्त हो जाएगा, क्योंकि केवल ‘पानी’ का अर्थ ही चमक है। शेष कोई भी शब्द इस अर्थ को प्रकट नहीं करता।

उसका मुख ‘चन्द्रमा’ के समान सुंदर है। इस कथन में चमत्कार का कारण अर्थ है- ‘चाँद’ भी सुंदर है और उसका मुख भी सुंदर है। यहाँ चाँद का कोई भी समानार्थी शब्द राकेश, इन्दु, शशि आदि रख देने से अर्थ का चमत्कार बना रहता है।

(क) शब्दालंकार

1. अनुप्रास

अनुप्रास अलंकार की परिभाषा और उदाहरण लिखें।
लक्षण-जहाँ व्यंजनों की बार-बार आवृति के कारण चमत्कार उत्पन्न हो, वहाँ अनुप्रास अलंकार होता है।
उदाहरण- चारू चंद्र की चंचल किरणें
खेल रही थीं जल थल में।

यहाँ ‘च’ की आवृति के कारण चमत्कार है।
अतः यहाँ अनुप्रास अलंकार है। विशेष- व्यंजनों की आवृति के साथ स्वर कोई भी आ सकता है अर्थात् जहाँ स्वरों की विषमता होने पर भी व्यंजनों की एक क्रम में आवृति हो, वहाँ ‘अनुप्रास’ अलंकार होता है। जैसे-
क्या आर्यवीर विपक्ष वैभव देखकर डरते कहीं?
उपरोक्त पंक्ति में ‘व’ की आवृति है।
इस प्रकार
1. तरनि-तनूजा तट तमाल तरुवर बहु छाय। इस पंक्ति में ‘त’ की आवृति है।
2. तजकर तरल तरंगों को, इन्द्रधनुष के रंगों को॥ (‘त’ की आवृति)
3. सत्य स्नेह सील सुख सागर उपरोक्त पंक्ति में (स) की आवृति है।
4. कल-कल कोमल कुसुम कुंज पर मधु बरसाने वाला कौन?
उपरोक्त पंक्ति में ‘क’ की आवृति है।

2. यमक

प्रश्न-यमक अलंकार की परिभाषा उदाहरण सहित लिखें।
उत्तर:
परिभाषा-जहाँ एक शब्द अथवा शब्द-समूह का एक से अधिक बार प्रयोग हो परन्तु प्रत्येक बार उसका अर्थ भिन्न-भिन्न हो, वहाँ यमक अलंकार होता है।
उदाहरण- कनक कनक ते सौगुनी मादकता अधिकाय।
या खाए बौराय जग, वा पाए बौराय॥

कनक का अर्थ यहाँ पर सोना तथा धतूरा है। यहाँ कनक शब्द का प्रयोग दो बार हुआ है और दोनों ही बार उनके अर्थ भिन्न-भिन्न हैं अतः यहाँ यमक अलंकार है।
उदाहरण- तों पर बारों उरबसी, सुनो राधिके सुजान।
तू मोहन के उरबसी, है उरबसी समान॥

‘उरबसी’ शब्द का प्रयोग तीन बार हुआ है। (i) उर्वशी नामक अप्सरा। (ii) उर में बसी हुई। (iii) गले में पहना जाने वाला आभूषण।
(2) ऊँचे घोर मन्दर के अन्दर रहन वारी,
ऊँचे घोर मन्दर के अन्दर रहाती है।
(i) मन्दर = महल (ii) मन्दर = गुफा।

(3) आँखें ये निगोड़ी खूब ऊधम मचाती आली,
आप कलपाती नहीं, हमें कलपाती हैं।
(i) कल्पाती = चैन पाती (ii) कलपाती = बेचैन करती, व्याकुल करती।

(4) रहिमन उतरे पार भार झोंकि सब भार में।
(i) भार = बोझा (ii) भार = भाड़।

(ख) अर्थालंकार

1. उपमा

उपमा अलंकार के चार अंग हैं-
1. उपमेय 2. उपमान 3. वाचक शब्द 4. साधारण धर्म।

1. उपमेय-जिसकी समता की जाती है, उसे उपमेय कहते हैं।
2. उपमान-जिससे ममता की जाती है, उसे उपमान कहते हैं।
3. वाचक शब्द-उपमेय और उपमान की समता प्रकट करने वाले शब्द को वाचक शब्द कहते हैं।

साधारण धर्म-उपमेय और उपमान में गुण (रूप, गुण आदि) की समानता को साधारण धर्म कहते हैं।
लक्षण-जहाँ रूप, रंग या गुण के कारण उपमेय की उपमान से तुलना की जाए, वहाँ उपमा अलंकार होता है।
उदाहरण-
पीपर पात सरिस मन डोला।
(पीपल के पत्ते के समान मन डोल उठा)
उपमेय-मन
उपमान–पीपर-पात
वाचक शब्द-सरिस (समान)
साधारण धर्म-डोला

जिस उपमा के चारों अंग होते हैं, उसे पूर्णोपमा कहते हैं। इनमें से किसी एक अथवा अधिक के लुप्त होने से लुप्तोपमा अलंकार होता है। अतः उपमा दो प्रकार की होती है
1. पूर्णोपमा 2. लुप्तोपमा।

अन्य उदाहरण-
1. हो क्रुद्ध उसने शक्ति छोड़ी एक निष्ठुर नाग-सी।
(उसने क्रोध से भरकर एक शक्ति (बाण) छोड़ी जो साँप के समान भयंकर थी।
(यहाँ ‘शक्ति’ उपमेय की ‘नाग’ उपमान से तुलना होने के कारण उपमा अलंकार है।)

2. वह किसलय के से अंग वाला कहाँ ?
(यहाँ अंग’ उपमेय की ‘किसलय’ उपमान से तुलना है) .

3. राम कीर्ति चाँदनी-सी, गंगाजी की धारा-सी,
सुपचपला की चमक से सुशोभित अपार है।
(धर्म लुप्त है, अतः यहाँ लुप्तोमा अलंकार है।)

4. भारत के सम भारत है।

5. सीमा-रहित अनंत गगन-सा विस्तृत उसका प्रेम हुआ।

6. हँसने लगे तब हरि अहा ! पूणेन्दु-सा मुख खिल गया।

7. कर लो नभ-सा शुचि जीवन को,
नर हो, न निराश करो मन को।

8. मांगन मरन समान है मत कोई मांगो भीख।

2. रूपक

लक्षण-उपमेरु उपमान जहँ एकै रूप कहायें।
अर्थात्-जहाँ उपमान पर उपमेय का आरोप किया जाए वहाँ रूपक अलंकार होता है। इस अलंकार में लक्षणा से चमत्कार उत्पन्न होता है।
‘रूपक’ का अर्थ है-‘रूप धारण करना।’ इस अलंकार में उपमेय उपमान का रूप धारण करता है।
उदाहरण-
उदित उदय-गिरि मंच पर रघुवर बाल पतंग।
विकसे संत-सरोज सब हरषे लोचन भंग॥

स्पष्टीकरण-
मंच पर श्रीराम के आने पर संतजनों को हर्ष हुआ। प्रस्तुत चौपाई में इतना ही कहा गया है किन्तु उदयगिरी (पर्वत यहाँ से सूर्योदय होता है) और मंच, रघुवर और बाल पतंग (सूर्य) संत और सरोज (कमल) लोचन और भृग (भंवरा) में एकरूपता दिखाकर सुन्दर रूपक बाँधा गया है।

दूसरा उदाहरण-
अम्बर-पनघट में डुबो रही
ताराघट उषा-नगरी : -जय शंकर प्रसाद

स्पष्टीकरण-
यहाँ अम्बर में पन घट, तारा में घट (घड़ा) और उषा में नागरी (सुन्दर स्त्री) का आरोप किया गया है। अत: यहाँ रूपक अलंकार है।

3. श्लेष

लक्षण-जहाँ पर एक ही शब्द के एक से अधिक अर्थ निकलें वहाँ श्लेष अलंकार होता है।
उदाहरण-
रहिमन पानी राखिये, बिन पानी सब सून।
पानी गये न ऊबरै, मोती, मानुस, चून॥

(रहीम कवि कहते हैं कि मनुष्य को पानी अर्थात् अपनी इज्जत अथवा मान-मर्यादा की रक्षा करनी चाहिए। बिना पानी के सब सूना है, व्यर्थ है। पानी के चले जाने से मोती का, मनुष्य का और चूने का कोई महत्त्व नहीं।)
यहाँ ‘पानी’ शब्द के तीन अर्थ हैं-
1. मोती के पक्ष में ‘पानी’ का अर्थ है ‘चमक’
2. मनुष्य के पक्ष में ‘पानी’ का अर्थ ‘इज्जत’.
3. चूने के पक्ष में ‘पानी’ का अर्थ है पानी (जल)
क्योंकि पानी के बिना चूने की सफेदी नहीं उभरती।
यहाँ ‘पानी’ शब्द के एक से अधिक अर्थ निकलते हैं। अतः यहाँ श्लेष अलंकार है।

अन्य उदाहरण-
(क) विपुल धन अनेकों रत्न हो साथ लाये।
प्रियतम बतला दो लाल मेरा कहाँ है॥
लाल-1. पुत्र 2. मणि।

(ख) को घटि ये वृषभानुजा।
वे हलधर के वीर।
वृषभानुजा-
1. वृषभानु की पुत्री अर्थात् राधिका।
2. वृष की अनुजा अर्थात् बैल की बहन।
हलधर-1. बलराम 2. हल को धारण करने वाला।

(ग) जो रहीम गति दीप की, कुल कपूत गति तोई।
बारे उजियारो करै, बढ़े अंधेरो होई॥

(दीपक की और कपूत की एक-सी गति होती है। दीपक के जलने पर प्रकाश होता है और उसके बुझने पर अन्धेरा हो जाता है। इसी प्रकार कपूत बचपन में कुल को उज्ज्वल करता है, परन्तु बड़ा होने पर अन्धेरा करता है, कुल को बदनाम करता है।)

‘बारे’ और ‘बढ़े’ के दो अर्थ हैं और दोनों अभीष्ट होने से श्लेष अलंकार होता है।
श्लेष अलंकार दो प्रकार का होता है-
(क) शब्द-श्लेष (ख) अर्थ-श्लेष।

ऊपर के उदाहरण में चमत्कार ‘बारे’ और ‘बढ़े’ के दो अर्थ होने के कारण हैं। यदि इनके बदले इनके पर्यायवाची शब्द रख दिए जाएं तो चमत्कार न रहेगा। यह चमत्कार शब्द-विशेष के प्रयोग के कारण है, अतः यहाँ शब्द-श्लेष है। अर्थ-श्लेष में श्लिष्ट शब्द को उसके पर्याय से बदल देने पर भी चमत्कार बना रहता है। अर्थ-श्लेष में शब्द का अर्थ तो प्राय: एक ही होता है, परन्तु वह दो पक्षों में उसी अर्थ के द्वारा भिन्न तात्पर्य देता है।

बोर्ड परीक्षा में पूछे गए प्रश्न

प्रश्न 1.
निम्नलिखित कथन की तुलना किसी दूसरी वस्तु के साथ की जाए, वह ……. अलंकार होता है।
उत्तर:
उपमा अलंकार।

प्रश्न 2.
जहां उपमान पर उपमेय का आरोप किया जाए, वहां …….. अलंकार होता है।
उत्तर:
रूपक अलंकार।

प्रश्न 3.
जिस रचना में व्यंजनों की आवृत्ति के कारण चमत्कार उत्पन्न हो, वहाँ ……… अलंकार होता है ।
अथवा
जिस रचना में वर्गों की बार-बार आवृत्ति के कारण चमत्कार उत्पन्न हो, वहाँ ……… अलंकार होता है।
उत्तर:
अनुप्रास।

प्रश्न 4.
जहाँ एक वस्तु की तुलना किसी दूसरी प्रसिद्ध वस्तु के साथ की जाये, वहाँ ……. अलंकार होता
उत्तर:
उपमा।

बहुविकल्पीय प्रश्नोत्तर

प्रश्न 1.
अलंकार का प्रमुख कार्य होता है?
(क) शोभा बढ़ाना
(ख) शोभा खोना
(ग) शोभा लेना
(घ) नष्ट करना।
उत्तर:
(क) शोभा बढ़ाना

प्रश्न 2.
अलंकार के प्रमुख भेद होते हैं?
(क) एक
(ख) दो
(ग) तीन
(घ) चार।
उत्तर:
(ख) दो

प्रश्न 3.
‘काली घटा का घमंड घटा’ में निहित अलंकार है
(क) अनुप्रास
(ख) यमक
(ग) श्लेष
(घ) रूपक।
उत्तर:
(ख) यमक

प्रश्न 4.
‘रघुपति राघव राजा राम’ पंक्ति में निहित अलंकार है
(क) यमक
(ख) श्लेष
(ग) अनुप्रास
(घ) रूपक।
उत्तर:
(ग) अनुप्रास,

प्रश्न 5.
‘आए महंत बसंत’ में निहित अलंकार का नाम लिखिए
(क) रूपक
(ख) उपमा
(ग) अनुप्रास
(घ) यमक।
उत्तर:
(क) रूपक

प्रश्न 6.
‘पीपर पात सरिस.मन डोला’ पंक्ति में उपमेय क्या है?
(क) मन
(ख) पीपर
(ग) पात
(घ) डोला।
उत्तर:
(क) मन

प्रश्न 7.
जहां एक ही शब्द के अनेक अर्थ प्रकट हों वहां कौन-सा अलंकार होता है?
(क) अनुप्रास
(ख) रूपक
(ग) यमक
(घ) श्लेष।
उत्तर:
(घ) श्लेष।

Punjab State Board PSEB 9th Class Maths Book Solutions Chapter 8 Quadrilaterals MCQ Questions with Answers.

PSEB 9th Class Maths Chapter 8 Quadrilaterals MCQ Questions

Multiple Choice Questions and Answer

Answer each question by selecting the proper alternative from those given below each question to make the statement true:

Question 1.
The ratio of four angles in order of a quadrilateral is 2 : 4 : 5 : 4. Then, the measure of the smallest angle of the quadrilateral is
A. 120°
B. 96°
C. 48°
D. 60°
Answer:
C. 48°

Question 2.
In quadrilateral PQRS, ∠P = 5x, ∠Q = 3x, ∠R = 4x and ∠S = 6x. Then, the measure of the greatest angle of quadrilateral PQRS is …………… .
A. 100°
B. 60°
C. 80°
D. 120°
Answer:
D. 120°

Question 3.
In quadrilateral ABCD, ∠A + ∠B = 150°.
Then ∠C + ∠D =
A. 105°
B. 210°
C. 150°
D. 300°
Answer:
B. 210°

Question 4.
In trapezium PQRS, PQ || RS. If ∠P = 150°, then ∠S = …………. .
A. 75°
B. 150°
C. 60°
D. 30°
Answer:
D. 30°

Question 5.
The perimeter of parallelogram ABCD is 22 cm.
If AB = 4 cm, then BC = ……………. cm.
A. 7
B. 6
C. 5.5
D. 4
Answer:
A. 7

Question 6.
In parallelogram ABCD, ∠A – ∠B = 30°. Then, ∠C = ……………… .
A. 105°
B. 75°
C. 150°
D. 60°
Answer:
A. 105°

Question 7.
In parallelogram ABCD, the bisectors of ∠A and ∠B intersect at M. If ∠A = 80°, then ∠AMB = ……………. .
A. 40°
B. 50°
C. 80°
D. 90°
Answer:
D. 90°

Question 8.
In parallelogram ABCD, the ratio ∠A : ∠B : ∠C : ∠D can be
A. 3 : 4 : 5 : 6
B. 2 : 3 : 3 : 2
C. 2 : 3 : 2 : 3
D. 2 : 3 : 5 : 8
Answer:
C. 2 : 3 : 2 : 3

Question 9.
In parallelogram ABCD, 3 ∠ A = 2 ∠ B. Then, ∠ D = ………………. .
A. 120°
B. 108°
C. 72°
D. 60°
Answer:
B. 108°

Question 10.
In ∆ ABC, E and F are the midpoints of AB and AC respectively. If EF = 4 cm, then BC = …………… cm.
A. 8
B. 2
C. 4
D. 12
Answer:
A. 8

Question 11.
In ∆ ABC, P is the midpoint of AB and Q is the midpoint of AC. Then, PQCB is a ………….. .
A. trapezium
B. parallelogram
C. rectangle
D. rhombus
Answer:
A. trapezium

Question 12.
In ∆ ABC, D, E and F are the midpoints of AB, BC and CA respectively. If the perimeter of ∆ DEF is 30 cm, then the perimeter of ∆ ABC is ……………. cm.
A. 15
B. 30
C. 45
D. 60
Answer:
D. 60

Question 13.
∆ ABC is an equilateral triangle. D, E and F are the midpoints of AB, BC and CA respectively. If AB = 8 cm, the perimeter of ∆ DEF is …………… cm.
A. 24
B. 12
C. 6
D. 48
Answer:
B. 12

Question 14.
ABCD is a rectangle. If AB = 5 cm and BC = 12
cm, then BD = ………………. cm
A. 17
B. 13
C. 8.5
D. 1
Answer:
B. 13

Question 15.
ABCD is a rhombus. If AC = 10 cm and BD = 24 cm, the perimeter of ABCD is …………………. cm.
A. 13
B. 26
C. 52
D. 48
Answer:
C. 52

Punjab State Board PSEB 12th Class Hindi Book Solutions Hindi Grammar samas समास Exercise Questions and Answers, Notes.

PSEB 12th Class Hindi Grammar समास

प्रश्न 1.
समास किसे कहते हैं ? उदाहरण सहित लिखें।
उत्तर:
परस्पर सम्बन्ध रखने वाले दो या दो से अधिक शब्दों को मिलकर बनने वाले एक स्वतन्त्र सार्थक शब्द को समास कहते हैं। जैसे:
राजा और कुमार से मिलकर-राजकुमार
राजा और पुरुष से मिलकर-राजपुरुष

प्रश्न 2.
समास परस्पर सम्बन्ध रखने वाले कौन से शब्दों के मेल से बनता है?
उत्तर:
संज्ञा के साथ संज्ञा का, संज्ञा के साथ विशेषण का, विशेषण के साथ विशेषय का तथा अव्यय के साथ संज्ञा का परस्पर मेल होने से समास बनता है।

प्रश्न 3.
‘समास’ शब्द का अर्थ सोदाहरण स्पष्ट करें।
उत्तर:
‘समास’ शब्द संस्कृत भाषा का है जिसका अर्थ है संक्षेपीकरण अर्थात् संक्षिप्त करना। जैसे ‘कपड़े से छना हुआ’ शब्द समूह का संक्षिप्त रूप होगा कपड़छन।
याद रखें : समास की विशेषता यह है कि यह जिस शब्द समूह का संक्षिप्त रूप होता है उसके अर्थ में किसी प्रकार का परिवर्तन नहीं होता जैसे कि ऊपर दिए गए उदाहरण ‘कपड़छन’ से स्पष्ट होता है।

प्रश्न 4.
समस्तपद या सामासिक शब्द किसे कहते हैं? सोदाहरण लिखें।
उत्तर:
समास करते समय परस्पर मेल होने वाले शब्दों के बीच की विभक्तियों या योजक शब्दों का लोप होकर जो शब्द बनते हैं, उन्हें ‘समस्त पद’ या ‘सामासिक शब्द’ कहते हैं। जैसे-
राम और लक्ष्मण = राम लक्ष्मण
चक्र है पाणि (हाथ) में जिसके = चक्रपाणि
यहाँ ‘राम लक्ष्मण’ तथा ‘चक्रपाणि’ समस्त पद या सामासिक शब्द हैं।

प्रश्न 5.
विग्रह किसे कहते हैं? सोदाहरण स्पष्ट करें।
उत्तर:
किसी शब्द में समास का पता करने के लिए समस्त पद के खण्डों को अलग-अलग करना पड़ता है, उसे विग्रह कहते हैं। अर्थात् समस्त पदों का विग्रह करके ही किसी शब्द का समास जाना जा सकता है। अतः विग्रह की परिभाषा हम इस तरह कर सकते हैं-
समस्त पदों के खण्ड करके विभक्तियाँ आदि लगाकर परस्पर सम्बन्ध दिखलाने की रीति को ‘विग्रह’ कहते हैं। जैसे-
राह-खर्च का विग्रह होगा-रास्ते के लिए खर्च
नीलकमल का विग्रह होगा-नीला है जो कमल

याद रखें: कभी विग्रह के आधार पर एक ही शब्द कई समासों का उदाहरण हो जाता है। जैसे ‘नीलकंठ’ का विग्रह यदि नीला है जो कंठ किया जाएगा तो यह ‘कर्मधारय’ समास होगा। किन्तु यदि इसी शब्द का विग्रह नीला है कंठ जिसका अर्थात् शंकर भगवान् किया जाएगा तो यह ‘बहुब्रीहि’ समास होगा।

समास के सम्बन्ध में कुछ याद रखने वाली बातें

1. हिन्दी में समास प्रायः दो शब्दों से ही बनते हैं जबकि संस्कृत में अनेक शब्दों से बनते हैं। हिन्दी में ‘सुत-वितनारी-भवन-परिवारा’ ही सबसे लम्बा समास है। इसके अतिरिक्त तन-मन-धन, जन-मन-गण, धूप-दीप-नैवैध आदि बहुत थोड़े शब्द हैं जो दो से अधिक शब्दों के मेल से बने हैं।

2. सामासिक शब्द बनते समय परस्पर मिलने वाले दोनों शब्दों की विभक्तियों या योजक शब्दों का लोप हो जाता है। जैसे राम और कृष्ण का समास राम-कृष्ण होने पर ‘और’ योजक शब्द का लोप हो गया है।

3. समास कुछ अपवादों को छोड़कर प्रायः दो सजातीय शब्दों में ही होता है। जैसे रसोई-घर का रसोईशाला नहीं बनेगा अथवा पाठशाला का पाठ घर शब्द नहीं बनेगा।
रेल-गाड़ी, जिला-धीश, धन-दौलत, मनमौजी, दुःख-सुख आदि इनके अपवाद हैं।

4. हिन्दी में मुख्यतः तीन ही प्रकार के शब्दों के सामासिक-शब्द प्रयोग में आते हैं। जैसे-
संस्कृत के-यथा-शक्ति, मनसिज, पुरुषोत्तम, युधिष्ठिर आदि।
हिन्दी के-भरपेट, अनबन, नीलकमल, दही-बड़ा, बैलगाड़ी, अलोना-सलोना आदि।
उर्दू-फ़ारसी के-नालायक, खुशबू, सौदागर, बेशक, चारदीवारी आदि।
इसके अतिरिक्त हिन्दी में कुछ अंग्रेजी शब्दों के मेल से अथवा हिन्दी अंग्रेजी शब्दों के मेल से भी समास बनते हैं। जैसे-
रेलवे स्टेशन, बुकिंग-ऑफिस, टिकट-चैकर, टाइम-टेबल तथा बस-अड्डा, पुलिस-चौकी, दल-बन्दी, पार्टी-बाज़ी आदि।

समास के भेद

प्रश्न 1.
समास के भेद किस आधार पर किये जाते हैं ?
उत्तर:
समास के भेद उसके पदों की प्रधानता-अप्रधानता के आधार पर किये जाते हैं। अर्थात् समास में कभी पहला पद प्रधान होता है तो कभी दूसरा और कभी-कभी दोनों ही पद प्रधान होते हैं अथवा कोई भी पद प्रधान नहीं होता। जैसे
रमेश गान्धी-भक्त है। यहां गान्धी-भक्त में भक्त प्रधान है क्योंकि रमेश भक्त है गान्धी नहीं।

प्रश्न 2.
समास के कितने भेद हैं?
उत्तर:
समास के मुख्यतः चार भेद माने जाते हैं। जो निम्नलिखित हैं-
1. अव्ययीभाव-इसमें पहला पद प्रधान होता है।
2. तत्पुरुष-इसमें दूसरा पद प्रधान होता है।
3. द्वन्द्व-इसमें दोनों पद प्रधान होते हैं।
4. बहुब्रीहि-इसमें कोई भी पद प्रधान नहीं होता।
कुछ विद्वान् समास के दो अन्य भेद भी मानते हैं-
1. कर्मधारय
2. द्विगु

किन्तु अनेक विद्वान् इन्हें तत्पुरुष समास का ही एक भेद मानते हैं।
इस तरह समास के कुल छः भेद माने जा सकते हैं-
1. अव्ययीभाव
2. तत्पुरुष
3. कर्मधारय
4. द्विगु
5. द्वन्द्व तथा
6. बहुब्रीहि

अव्ययी भाव

प्रश्न 1.
अव्ययीभाव समास की परिभाषा उदाहरण सहित लिखें।
उत्तर:
जिस समास में पहला पद प्रधान हो और समस्त पद अव्यय (क्रिया विशेषण) का काम करे, उसे अव्ययी भाव समास कहते हैं। जैसे-
संस्कृत शब्दों से-यथा शक्ति, प्रतिदिन, यावज्जीवन, व्यर्थ
हिन्दी शब्दों से-भरपेट, हाथों-हाथ, दिनों-दिन, हर घड़ी आदि।

याद रखें-1. अव्ययीभाव समास में समस्त शब्द अव्यय होता है। अतः उसके साथ विभक्ति चिह्न नहीं लगता। जैसे-
यह पुस्तक हाथों हाथ बिक गयी।
वे रातों रात शहर छोड़ कर चले गए।

2. यथा, प्रति, भर तथा आ जिस शब्द के पहले पद होते हैं, वे सब अव्ययीभाव समास कहलाते हैं। जैसा प्रत्येक, प्रतिवर्ष, भरसक, आमरण आदि।

3. द्विरुक्त शब्द बहुधा अव्ययीभाव होते हैं। जैसे-घड़ी-घड़ी, पल-पल, रोज़-रोज़, घर-घर, दर-दर, वन-वन आदि।

4. द्विरुक्त शब्दों के बीच में ‘ही’ अथवा ‘आ’ लगने पर भी अव्ययीभाव ही होता है। जैसे-दिल ही दिल, मन ही मन, साथ ही साथ, एकाएक, मुँहा-मुँह, धड़ाधड़, सरासर आदि।

2. तत्पुरुष

प्रश्न 1.
तत्पुरुष समास की परिभाषा उदाहरण सहित लिखें।
उत्तर:
जिस समास का दूसरा पद प्रधान होता है और दोनों पदों के बीच कर्ता तथा सम्बोधन कारक के अतिरिक्त शेष किसी भी कारक की विभक्ति का लोप हो जाता है। जैसे-
ग्रन्थकार = ग्रन्थ के करने वाला
तुलसीकृत = तुलसी से कृत।
देश-भक्ति = देश के लिए भक्ति
भयभीत = भय से भीत
हिमालय = हिम (बर्फ) का घर
शोकमग्न = शोक में मग्न

ऊपर के उदाहरणों में क्रमशः कर्म, करण, सम्प्रदान, अपादान, सम्बन्ध तथा अधिकरण कारक चिह्नों का लोप हुआ है। इन उदाहरणों में दूसरे पद ही प्रधान हैं। जैसे राज पुरुष में पुरुष प्रधान है क्योंकि यदि हम कहें राज पुरुष पधार रहे हैं तो इसका अर्थ होगा ऐसा पुरुष पधार रहा है जिसका सम्बन्ध राजा से है वह राजा नहीं है।

प्रश्न 2.
तत्पुरुष समास के कितने भेद हैं ? उदाहरण सहित लिखें।
उत्तर:
तत्पुरुष समास में प्रथम पद के साथ जिस कारक की विभक्ति आती है तथा जो समास करते समय लुप्त हो जाती है, उसी कारक के अनुसार तत्पुरुष का नाम भी होता है। जैसे-

1. कर्म तत्पुरुष-यशप्राप्त (यश को प्राप्त)
2. करण तत्पुरुष-हस्तलिखित (हस्त (हाथ) से लिखित) .
3. सम्प्रदान तत्पुरुष-गुरुदक्षिणा (गुरु के लिए दक्षिणा)
4. अपादान तत्पुरुष-ऋण मुक्त (ऋण से मुक्त)
5. सम्बन्ध तत्पुरुष-पवन पुत्र (पवन का पुत्र)
6. अधिकरण तत्पुरुष-घुड़सवार (घोड़े पर सवार)

कुछ अन्य प्रश्न
सामासिक शब्द सूची

परीक्षा में प्रायः सामासिक शब्द देकर उनके समास का नाम लिखने को भी कहा जाता है अथवा कभी-कभी कुछ शब्द देकर यह पूछा जाता है कि इस शब्द में समास कौन-सा है। इसी बात को ध्यान में रखते हुए एक विस्तृत सूची यहाँ दी जा रही है।

1. अव्ययीभाव समास

अजानु-जानुओं (घुटनों) तक
अनुगमन-पीछे चलना
अतिकष्ट-बहुत कष्ट
आजीवन-जीवन पर्यन्त
आमरण-मरण पर्यन्त
उपकण्ठ-कण्ठ के समीप
उपकुल-कुल के समीप
उपकृष्ण-कृष्ण के समीप
उपनगर-नगर के समीप
प्रतिदिन-दिन-दिन
अनजाने-जाने बिना
घड़ी-घड़ी-हर घड़ी
घर-घर-हर घर
ज्ञानपूर्वक-ज्ञान के अनुसार
निडर-बिना डर
गली-गली-प्रत्येक गली
प्रत्येक-एक-एक
यथामति–मति के अनुसार
यथा विधि-विधि के अनुसार
यथा शक्ति-शक्ति के अनुसार
यथा शीघ्र-जितना शीघ्र हो सके उतना शीघ्र
यथा संख्य-संख्या के अनुसार
यथा सम्भव-जैसा सम्भव हो
यथा साध्य-जो हो सके
यथा सामर्थ्य-सामर्थ्य के अनुसार
यथोचित -जितना उचित हो
निस्संदेह-संदेह के बिना
बीचों-बीच-ठीक बीच में
भर पेट-पेट भर कर
भरसक-पूरी शक्ति से
हाथों हाथ-हाथ ही हाथ
साफ-साफ-बिलकुल साफ

2. तत्पुरुष

परलोकगमन-परलोक को गमन
यशप्राप्त-यश को प्राप्त
विदेशगत-विदेश को गया हुआ
शरणागत-शरण को आगत (आया हुआ)
स्वर्गगत-स्वर्ग को गत (गया हुआ)
मोक्षप्राप्त-मोक्ष को प्राप्त

कर्म तत्पुरुष-
गंगा प्राप्त-गंगा को प्राप्त
ग्रन्थकार-ग्रन्थ को करने (रचने) वाला
ग्रामगत-ग्राम को गया हुआ
जलपिपासु-जल पीने की इच्छा रखने वाला
जेबकतरा-जेब को कतरने (काटने) वाला
देशगत-देश को गया हुआ

करण तत्पुरुष-
अकाल-पीड़ित-अकाल से पीड़ित
अनुभव-जन्य-अनुभव से जन्य (उत्पन्न)
आचार-हीन-आचार से रहित
ईश्वर-प्रदत्त-ईश्वर से प्रदत्त प्रदत्त (दिया हुआ)
कपड़छन-कपड़े से छना हुआ
कलंकयुक्त-कलंक से युक्त
कष्टसाध्य-कष्ट से साध्य

धनहीन-धन से रहित
प्रेमातुर-प्रेम से आतुर
बाढ़-पीड़ित-बाढ़ से पीड़ित
बाणबिद्ध-बाण से बिद्ध
बिहारी रचित-बिहारी द्वारा रचित
भुखमरा-भूख से मरा हुआ
मदमाता-मद से माता (मस्त)
कीर्तियुक्त-कीर्ति से युक्त
मदाँध-मद से अंधा
गुणयुक्त-गुण से युक्त
मदोन्मत्त-मद से उन्मत्त
गुण हीन-गुण से रहित
मनगढन्त-मन से गढ़ी हुई
गुन भरा-गुण से भरा हुआ
मन चाहा-मन से चाहा
गुरुकृत-गुरु से कृत (किया हुआ)
मन माना-मन से माना हुआ
गुरुदत्त-गुरु से दत्त (दिया हुआ)
मुँह माँगा-मुँह से माँगा हुआ
जन्मरोगी-जन्म से रोगी
रेखांकित-रेखा से अंकित
ज्ञान मुक्त–ज्ञान से मुक्त
रेलयात्रा-रेल से यात्रा
ज्ञान युक्त–ज्ञान से युक्त
वाग्दत्ता-वाक् से दत्ता
तुलसीकृत-तुलसी से कृत
शोकाकुल-शोक से आकुल
दयार्द्र-दया से आर्द्र
श्रीयुक्त-श्री (लक्ष्मी) से युक्त
दर्द भरा-दर्द से भरा हुआ
श्रीहीन–श्री (लक्ष्मी) से हीन
दुःर्खात–दुःख से आर्त (व्याकुल)
हस्तलिखित-हाथ से लिखित
दोषपूर्ण-दोष से पूर्ण

सम्प्रदान तत्पुरुष

आराम कुर्सी-आराम के लिए कुर्सी
प्रयोगशाला-प्रयोग के लिए शाला
क्रीड़ा क्षेत्र-क्रीड़ा के लिए क्षेत्र
बलिपशु-बलि के लिए पशु
कृष्णापर्ण-कृष्ण के लिए अर्पण
मार्गव्यय-मार्ग के लिए व्यय
गुरुदक्षिणा-गुरु के लिए दक्षिणा
यज्ञशाला-यज्ञ के लिए शाला
गौशाला-गौओं के लिए शाला
युद्धक्षेत्र-युद्ध के लिए क्षेत्र
जेब खर्च-जेब के लिए खर्च
युद्धभूमि-युद्ध के लिए भूमि
डाकगाड़ी-डाक के लिए गाड़ी
रसोई घर-रसोई के लिए घर
देवबलि-देवता के लिए बलि
राज्यलिप्सा-राज्य के लिए लिप्सा
देशभक्ति-देश के लिए भक्ति
राहखर्च-राह के लिए खर्च
देशापर्ण देश के लिए अपर्ण
रेलभाड़ा-रेल के लिए भाड़ा
परोपकार-पर (दूसरे) के लिए उपकार
सत्याग्रह-सत्य के लिए आग्रह
पाठशाला-पाठ के लिए शाला
हथकड़ी-हाथ के लिए घड़ी
पुत्रशोक-पुत्र के लिए शोक
हवनसामग्री-हवन के लिए सामग्री
पुत्रहित-पुत्र के लिए हित

अपादान तत्पुरुष

आकाशवाणी-आकाश से आने वाली वाणी
देशनिर्वासन-देश से निर्वासन
आशातीत-आशा से अधिक
धनहीन-धन से हीन
ईश्वरविमुख-ईश्वर से विमुख
धर्मभ्रष्ट-धर्म से भ्रष्ट
ऋणमुक्त-ऋण से मुक्त
पथभ्रष्ट-पथ से भ्रष्ट
कामचोर-काम से जी चुराने वाला
पदच्युत-पद से च्युत
गुरुभाई-गुरु से पढ़कर भाई
बन्धनमुक्त-बन्धन से मुक्त
जन्मांध-जन्म से अन्धा
भयभीत-भय से भीत
जन्मपूर्व-जन्म से पूर्व
रोगमुक्त-रोग से मुक्त
जलजात-जल से जात (उत्पन्न)
लक्ष्यभ्रष्ट-लक्ष्य से भ्रष्ट
देशनिकाला-देश से निकालना
सर्वोत्तम-सर्व से उत्तम
भवसागर-भव का सागर
भारतरत्न-भारत का रत्न
भारतवासी-भारत के वासी
भ्रातृस्नेह-भ्राता का स्नेह
मृगशावक-मृग का शावक (बच्चा)
यमलोक-यम का लोक
यमुनातट-यमुना का तट
रघुकुलमणि-रघुकुल की मणि
राजकुमार-राजा का कुमार
राजनीतिज्ञ-राजनीति का ज्ञाता
राजपुरुष-राजा का पुरुष
राजवंश-राजा का वंश
रामकहानी-राम की कहानी
लखपति–एक लाख का पति
वनमाली-वन का माली

सम्बन्ध तत्पुरुष

अछूतोद्धार-अछूतों का उद्धार
अमचूर-आम का चूरा
अमृतरस-अमृत का रस
आत्महत्या-आत्मा (अपनी) की हत्या
कनकघट-कनक (सोने) का घट (घड़ा)
कालिदास-काली का दास
कुलदीप-कुल का दीपक
गंगातट-गंगा का तट
गजराज-गजों का राजा
गुरुसेवा-गुरु की सेवा
गौरीपुत्र-गौरी (पार्वती) का पुत्र
घुड़दौड़-घोड़ों की दौड़
जलधारा-जल की धारा
जीवनसाथी-जीवन का साथी
तरणितनूजा-तरणि (सूर्य) की तनूजा (पुत्री)
दिनचर्या-दिन की चर्या
दिनमान-दिन का मान
दीनानाथ-दिनों का नाथ
देवकन्या-देवता की कन्या
देवराज-देवताओं का राजा
देवालय-देव का आलय
देशसेवक-देश का सेवक
परनिन्दा-पर (दूसरे) की निन्दा
पराधीन-पर (दूसरे) के अधीन
पशुपति-पशुओं का पति
प्रजापति-प्रजाओं का पति
प्रेमसागर-प्रेम का सागर
बैलगाड़ी-बैलों की गाड़ी
वायुसेना-वायु की सेना
विचाराधीन-विचार के अधीन
विद्यार्थी-विद्या का अर्थी (इच्छुक)
विद्यालय-विद्या का आलय
विश्वविद्यालय–विश्व की विद्यालय
सचिवालय-सचिवों का आलय
सिरदर्द-सिर का दर्द
सुखसागर-सुख का सागर
सूर्यपुत्र-सूर्य का पुत्र
सेनापति-सेना का पति
हिन्दुस्थान-हिन्दुओं का स्थान
हिमालय-हिम (बर्फ) का घर

अधिकरण तत्पुरुष

आत्मविश्वास-आत्म (अपने) पर विश्वास
आनन्दमग्न-आनन्द में मग्न
आपबीती-अपने पर बीती।
कानाफूसी-कानों में फुसफुसाहट
कलाप्रवीण-कला में प्रवीण
गृहप्रवेश-गृह में प्रवेश
घुड़सवार-घोड़े पर सवार
जनप्रिय-जनता में प्रिय
दानवीर-दान (देने) में वीर
देशाटन-देश में अटन (भ्रमण)
धर्मवीर-धर्म में वीर
रणकौशल-रण में कौशल
लोकप्रिय-लोक में प्रिय
वनवास-वन में वास
शोकमग्न-शोक में मग्न

(क) नञ् तत्पुरुष

निषेध या अभाव के अर्थ में किसी शब्द से पूर्व ‘अ’ या ‘अन्’ लगाने से जो समास बनता है, उसे नञ् तत्पुरुष समास कहते हैं। जैसे-
अहित = न हित
अपूर्ण = न पूर्ण
अधर्म = न धर्म
असंभव = न संभव
अब्राह्मण = न ब्राह्मण
अन्याय = न न्याय
अनुदार = न उदार
अनाश्रित =न आश्रित
अनिष्ट =न इष्ट
अनाचार = न आचार

विशेष-(क) प्रायः संस्कृत शब्दों में जिस शब्द के आदि में व्यंजन होता है, तो ‘नञ्’ समास में उस शब्द से पूर्व ‘अ’ जुड़ता है और यदि शब्द के आदि में स्वर होता है, तो उससे पूर्व ‘अन्’ जुड़ता है, जैसे-
अन् + अन्य = अनन्य
अन् + उत्तीर्ण = अनुत्तीर्ण
अ + वांछित = अवांछित
अ + स्थिर = अस्थिर।

(ख) किंतु उक्त नियम प्रायः तत्सम शब्दों पर ही लागू होता है, हिंदी शब्दों पर नहीं। हिंदी शब्दों में सर्वत्र ऐसा नहीं होता, जैसे-
अन् + चाहा = अनचाहा
अ + काज = अकाज
अन + होनी = अनहोनी है
अन + बन = अनबन
अ + न्याय = अन्याय
अन + देखा = अनदेखा
अ + टूट = अटूट
अ + सुंदर = असुंदर।

(ग) हिंदी और संस्कृत शब्दों के अतिरिक्त ‘गैर’ और ‘ना’ वाले शब्द भी ‘न’ तत्पुरुष के अंतर्गत आ जाते हैं, जैसे-
नागवार नापसंद
गैर हाज़िर नाबालिग
नालायक गैरवाज़िब।

(ख) अलुक् तत्पुरुष

जिस तत्पुरुष समास में पहले पद की विभक्ति का लोप नहीं होता, उसे ‘अलुक्’ तत्पुरुष समास कहते हैं, जैसे-
मनसिज = मन में उत्पन्न
वाचस्पति = वाणी का पति
विश्वंभर = विश्व को भरने वाला
युधिष्ठिर = युद्ध में स्थिर
धनंजय = धन को जय करने वाला
खेचर = आकाश में विचरने वाला।

(ग) उपपद तत्पुरुष

जिस तत्पुरुष समास का स्वतंत्र रूप में प्रयोग नहीं किया जा सकता, ऐसे सामासिक शब्दों को ‘उपपद’ तत्पुरुष समास कहते हैं, जैसे-
जलज = जल + ज (‘ज’ का अर्थ उत्पन्न अर्थात् पैदा होने वाला है, पर इस शब्द को अलग से प्रयोग नहीं किया जा सकता है।)
इसी प्रकार
तटस्थ = तट + स्थ
गृहस्थ = गृह + स्थ
पंकज = पंक + ज
जलद = जल + द
कृतघ्न = कृत + न
उरग = उर + ग
तिलचट्टा = तिल + चट्टा
लकड़फोड़ = लकड़ + फोड़
बटमार = बट + मार
घरघुसा = घर + घुसा
पनडुब्बी = पन + डुब्बी
घुड़चढ़ी = घुड़ + चढ़ी
कलमतराश = कलम + तराश
सौदागर = सौदा + गर
ग़रीबनिवाज़ = ग़रीब + निवाज़
चोबदार = चोब + दार।

3. कर्मधारय

जिस समास के दोनों पदों के बीच विशेष्य-विशेषण अथवा उपमेय-उपमान का संबंध हो और दोनों पदों में एक ही कारक (कर्ता कारक) की विभक्ति आए, उसे कर्मधारय समास कहते हैं, जैसे-
नीलकमल = नीला है जो कमल
लाल-मिर्च = लाल है जो मिर्च
पुरुषोत्तम = पुरुषों में है जो उत्तम
महाराजा = महान् है जो राजा
चंद्रमुख = चंद्र के समान है जो मुख
पुरुषसिंह = सिंह के समान है जो पुरुष
नील-कंठ = नीला है जो कंठ
महाजन = महान् है जो जन
पीतांबर = पीत है जो अंबर
सज्जन = सत् (अच्छा) है जो जन
भलामानस = भला है जो मानस (मनुष्य)
सद्गुण = सद् (अच्छे) हैं जो गुण
शुभागमन = शुभ है जो आगमन
नीलांबर = नीला है जो अंबर
महाविद्यालय = महान् है जो विद्यालय
काला-पानी = काला है जो पानी
चरण-कमल = कमल रूपी चरण
प्राण-प्रिय = प्राणों के समान प्रिय
वज्र-देह = वज्र के समान देह
विद्या धन = विद्या रूपी धन
देहलता = देह रूपी लता
घनश्याम = घन के समान श्याम
काली-मिर्च = काली है जो मिर्च
महारानी = महान् है जो रानी
नील-गाय = नीली है जो गाय
कर-कमल = कमल के समान
कर मुखचंद्र = मुख रूपी चंद्र
नरसिंह = सिंह के समान है जो नर
भव-सागर = भव रूपी सागर
बुद्धिबल = बुदधि रूपी बल
गुरुदेव = गुरु रूपी देव
कर-पल्लव = पल्लव रूपी कर
कमल-नयन = कमल के समान नयन
कनक-लता = कनक की सी लता
चंद्रमुख = चंद्र के समान मुख
मृगनयन = मृग के नयन के समान नयन
कुसुम-कोमल = कुसुम के समान कोमल
सिंह-नाद = सिंह के नाद के समान नाद
जन्मांतर = अंतर (अन्य) जन्म
नराधम = अधम है जो नर
दीनदयालु = दीनों पर है जो दयालु
मुनिवर = मुनियों में है जो श्रेष्ठ
मानवोचित = मानवों के लिए है जो उचित
पुरुष-रत्न = पुरुषों में है जो रत्न
घृतांत = घृत में मिला हुआ अन्न
पर्णशाला = पर्ण (पत्तों से) निर्मित शाला
छाया-तरु = छाया-प्रधान तरु
वन-मानुष = वन में निवास करने वाला मानुष
गुरु-भाई = गुरु के संबंध से भाई
बैलगाड़ी = बैलों से खींची जाने वाली गाड़ी
माल-गाड़ी = माल ले जाने वाली गाड़ी
गुडंबा = गुड से पकाया हुआ आम
दही-बड़ा = दही में डूबा हुआ बड़ा
जेब-घड़ी = जेब में रखी जाने वाली घड़ी
पन-चक्की = पानी से चलने वाली चक्की

4. द्विगु

जिस समास में पहला पद संख्यावाचक हो और समस्त समूह या समाहार का ज्ञान कराए, उसे द्विगु समास कहते हैं, जैसे-
शताब्दी = शत (सौ) अब्दों (वर्षों) का समूह
सतसई = सात सौ दोहों का समूह
चौराहा = चार राहों (रास्तों) का समाहार
चौमासा = चार मासों का समाहार
अठन्नी = आठ आनों का समूह
पंसेरी = पाँच सेरों का समाहार
दोपहर = दो पहरों का समाहार
त्रिफला = तीन फलों का समूह
चौपाई = चार पदों का समूह
नव-रत्न = नौ रत्नों का समूह
त्रिवेणी = तीन वेणियों (नदियों) का समाहार
सप्ताह = सप्त (सात) अह (दिनों) का समूह
सप्तर्षि = सात ऋषियों का समूह
अष्टाध्यायी = अष्ट (आठ) अध्यायों का समूह
त्रिभुवन = तीन भुवनों (लोकों) का समूह
पंचवटी = पाँच वट (वृक्षों) का समाहार
नवग्रह = नौ ग्रहों का समाहार
चतुर्वर्ण = चार वर्णों का समूह
चतुष्पदी = चार पदों का समाहार
पंचतत्व = पाँच तत्वों का समूह।

बहब्रीहि समास

बहुब्रीहि समास की परिभाषा उदाहरण सहित लिखें।
उत्तर:
जिस समास का कोई भी पद प्रधान नहीं होता और दोनों पद किसी अन्य शब्द (संज्ञा) के विशेषण होते हैं, उसे बहुब्रीहि समास कहते हैं। जैसे-
नीलकण्ठ-नीला है कण्ठ जिसका अर्थात् शिव
दिगम्बर-दिशाएं ही हैं वस्त्र जिसके अर्थात् नग्न
चन्द्रमुखी-चन्द्र के समान है मुख है जिसका (कोई स्त्री)
मनचला-मन रहता हो चंचल जिसका
दशानन-दश है आनन (मुख) जिसके अर्थात् रावण

द्वन्द्व समास

द्वन्द्व समास की परिभाषा उदाहरण सहित लिखें।
उत्तर:
जिस समस्त पद के दोनों पद प्रधान हों तथा विग्रह (अलग-अलग) करने पर दोनों पदों के बीच ‘और’, ‘तथा’, ‘अथवा’, ‘या’ आदि योजक शब्द लगें, उन्हें द्वन्द्व समास कहते हैं। जैसे-
पाप-पुण्य-पाप अथवा पुण्य।
पति-पत्नी-पति और पत्नी।
अन्न-जल-अन्न और जल
भीम-अर्जुन-भीम और अर्जुन।
राधा-कृष्ण-राधा और कृष्ण
सीता-राम-सीता और (राम)
निशि-वासर-निशि और वासर
दालभात-दाल और भात
देश-विदेश-देश और विदेश
जल-थल-जल और थल
दीन-ईमान-दीन और ईमान
पूर्वपश्चिम-पूर्व और पश्चिम।

सामासिक शब्द सूची

बहब्रीहि समास

अंशुमाली–अंशु (किरणें) है माला जिसकी-सूर्य
अजातशत्रु-अजात (नहीं पैदा हुआ हो) है शत्रु जिसका
अजानुबाहु-अजानु (घुटनों तक लम्बी) है भुजाएं जिसकी-अवतारी पुरुष
अनहोनी-न होने वाली घटना
उदारहृदय-उदार हृदय है जिसका
कनकटा-कान कटा हुआ है जिसका
कनफटा-कान फटे हुए हैं जिसके
कुसुमाकर-कुसमों का खजाना है जो-वसंत ऋतु।
गजानन-गज का मुख है जिसका गणेश
विषधर-विष को धारण करने वाला सर्प।
कुसुमाकर-कुसुमों का आकार (समूह) है जो-बसन्त ऋतु
गिरिधर-गिरि (पर्वत) को धारण करने वाला-श्रीकृष्ण
घनश्याम-घन के समान श्याम (काला) है जो-श्रीकृष्ण
चन्द्रमुखी-चन्द्रमा के समान मुख है जिसका।
चन्द्रवदनि-चन्द्रमा के समान बदन (मुख) है जिसका
चन्द्रशेखर-शेखर (मस्तक) पर है चन्द्र जिसके-शिवजी
चक्रपाणि-चक्र है पाणि (हाथ) में जिसके-विष्णु
चतुर्भुज-चार भुजाएं हैं जिसकी-विष्णु
चारपाई-चार हैं पैर जिसके-खाट
तिमंजिला-तीन हैं मंजिल जिसकी
त्रिनेत्र-तीन हैं नेत्र जिसके अर्थात् शिव।
दशानन–दश हैं आनन (मुख) जिसके-रावण
दिगम्बर-दिशाएं हैं वस्त्र जिसके-शिवजी
दुरात्मा-दुष्ट (बुरी) आत्मा वाला
धर्मात्मा-धर्म में आत्मा वाला
नीलकण्ठ-नीला है कण्ठ जिसका-शिवजी
पंकज-पंक (कीचड़) में पैदा हुआ है जो-कमल
पंचानन–पाँच हैं मुख जिसके-ब्रह्मा जी
पंचवटी-पाँच हैं वट (वृक्ष) जहाँ
पद्मासना-पद्म (कमल) है आसन जिसका-सरस्वती
पीताम्बर-पीले हैं अम्बर (कपड़े) जिसके-श्रीकृष्ण, विष्णु
प्रधानमन्त्री–मन्त्रियों में प्रधान है जो
बड़बोला-बड़े बोल बोलने वाला
मनचला-मन है चलायमान (चंचल) जिसका
मयूरवाहन-मयूर (मोर) है वाहन जिसका-शिवजी पुत्र कार्तिकेय
महावीर-महान् है वीर जो-हनुमान जी
मीनाक्षी-मीन (मछली) जैसी आँखें हैं जिसकी
मृगाक्षी/मृगनयनी-मृग की आँखों जैसी आँखें हैं जिसकी स्त्री विशेष।
मृगेन्द्र-मृगों का इन्द्र (राजा) है जो-सिंह
मृत्युञ्जय-मृत्यु को जीतने वाला है जो-शिवजी
मेघनाद-मेघ के समान नाद है जिसका-रावण पुत्र इन्द्रजीत
लम्बोदर-लम्बा है उदर जिसका-गणेश
महादेव-महान् है जो देव-शिव।
त्रिलोचन-तीन हैं नेत्र जिसके-शिव
बारहसिंगा-बारह है सींग जिसके (वह हिरन)
वीणापाणि-वीणा है पाणि (हाथ) में जिसके-सरस्वती
चक्रधर-चक्र को धारण करने वाला-विष्णु
सहस्रबाहु-सहस्र (हज़ार) भुजाओं वाला-एक रक्षक का नाम
सिरकटा-सिर है कटा हआ जिसका।
सुलोचना-सुन्दर है लोचन जिस (स्त्री) के
षटकोण-षट (छ:) है जिसके कोण
षडानन-छ: मुख हैं जिसके
पतझड़-झड़ते हैं पत्ते जिसमें वह ऋतु
अष्टाध्यायी-आठ अध्यायों वाला (पणिनी व्याकरण)
महात्मा-महान् है आत्मा जिसकी
गुरुद्वारा-गुरु का द्वारा है जो (सिक्ख धर्म का परम-पवित्र धार्मिक स्थल)

द्वन्द्व समास

अन्न-जल-अन्न और जल
नमक-मिर्च-नमक और मिर्च
अमीर-गरीब-अमीर और ग़रीब
नर-नारी-नर और नारी
आचार-व्यवहार-आचार और व्यवहार
नाच-रंग-नाच और रंग
आब-हवा-आब (पानी) और हवा
नाम-निशान-नाम और निशान
ऊँचा-नीचा-ऊँचा और नीचा
निशि-वासर-निशि (रात) और वासर (दिन)
खरा-खोटा-खरा और खोटा
रुपया-पैसा-रुपया और पैसा
गुण-दोष-गुण और दोष
नोन-तेल-नोन और तेल
चाल-चलन-चाल और चलन
पाप-पुण्य-पाप और पुण्य
जन्म-मरण-जन्म और मरण
पास-पड़ोस-पास और पड़ोस
जञान-विज्ञान-ज्ञान और विज्ञान
बीस-पच्चीस-बीस और पच्चीस
तिल-चावल-तिल और चावल
भूखा-प्यासा-भूखा और प्यासा
थोड़ा बहुत-थोड़ा और बहुत
माँ-बाप-माँ और बाप
दस-बीस-दस और बीस
राजा-रंक-राजा और रंक
दाल-रोटी-दाल और रोटी
रात-दिन-रात और दिन
दीन-ईमान-दीन और ईमान
राम-कृष्ण-राम और कृष्ण
आचार-व्यवहार-आचार और व्यवहार
राम-लक्ष्मण–राम और लक्ष्मण
दो-चार-दो और चार
लूट-मार-लूट और मार
धनी-निर्धन-धनी और निर्धन
वेद-पुराण-वेद और पुराण
धर्म-अधर्म-धर्म और अधर्म
सुख-दुःख-सुख और दुःख
नदी-नाले-नदी और नाले
राजा-रानी-राजा और रानी।
गंगा-यमुना-गंगा और यमुना
माता-पिता-माता और पिता,
धूप-दीप-धूप और दीप।।
लोभ-मोह-लोभ और मोह।

बोर्ड परीक्षा में पूछे गए प्रश्न

किन्हीं पाँच के समास/समास विग्रह कीजिए।
1. यथा शक्ति, आजीवन, देश निकाला, राह के लिए खर्च, राजा का कुमार।
2. विधि के अनुसार, हाथ ही हाथ में, सेनापति, सिरदर्द, चतुर्भुज।
3. जन्म से लेकर, प्रत्येक गली, रोजगार के बिना, मधुमक्खी, पदच्युत।
4. पति-पत्नी, मेघनाद महात्मा, पूर्व-पश्चिम, लम्बा है उदर जिसका, आचार और व्यवहार, दश हैं आनन जिसके, गंगा और यमुना।
5. महान है आत्मा जिसकी, अन्न और जल, झड़ते हैं पत्ते जिसमें, नर और नारी, गणेश, मृत्युंजय, सीता-राम, ‘ भीम-अर्जुन।
6. रात और दिन, पीत है अम्बर जिसका, सुख और दुःख, कुसुमों का खजाना है, जो धूप-दीप, पंकज, दालभात, चक्रधर।
7. धनहीन, महात्मा, त्रिलोकी, नवरत्न, सत्य के लिए आग्रह, पथ से भ्रष्ट, राजा की नीति, दूध और दही।
8. कुरूप, घनश्याम, पंचवटी, पञ्चानन, महान् है जो देव, न होने वाली घटना, तीन हैं मंज़िल जिसकी, गिरि को धारण करने वाला।
9. चौमासा, अनन्त, दोपहर, विद्यासागर, आठ अध्यायों का समाहार, राह के लिए खर्चे, गणों का पति, पीत हैं अम्बर जिसके।
10. आचार और व्यवहार, मेघ के समान है नाद, सात दिनों का समूह, मालगाड़ी, हस्तलिखित, विद्यालय।
11. जल और थल, महान् है आत्मा जिसकी, तीन रंगों का समूह आजीवन, धनहीन, घुड़सवार।
12. सीता और गीता, झड़ते हैं पत्ते जिसमें, चार भुजाओं का समूह, धर्मवीर, बेखटके, पीताम्बर।
13. पूर्व और पश्चिम, पीला है जो अम्बर, चार भुजाओं वाला, दशानन, यथाशक्ति, अमीर-ग़रीब।
14. देश और विदेश, माल ढोने वाली गाड़ी, नौ ग्रहों का समूह, राजकमार, यथानियम, अन्न-जल।
15. गंगा और यमुना, रेल पर चलने वाली गाड़ी, जन्म से लेकर, सत्याग्रह, गुरुदक्षिणा, पति-पत्नी।
16. शक्ति के अनुसार, महान है आत्मा जिसकी, सिर में दर्द, धनहीन, अन्न-जल, चतुर्भुज।
17. तीन फलों का समूह, रात और दिन, झड़ते हैं पले जिसमें, यथानियम, गौशाला, राष्ट्रपति।
18. रुचि के अनुसार, राजा और रानी, नौ ग्रहों का समूह, गिरिधर , देशवासी नीलाम्बर।
19. नर और नारी, महान है जो देव, सात दिनों का समूह, पतझड़, यथानियम, पाप-पुण्य।
20. भीम और अर्जुन, लाल है जो रूमाल, दो पहरों का समूह, रामभक्ति, यथाकाल, माता-पिता।
21. दाल और भात, महान है जो जन, जन्म से लेकर, बाढ़ पीड़ित, राह खर्च, धूप-दीप।

बोर्ड परीक्षा में पूछे गए प्रश्न

Set-A
निम्नलिखित पदों का समास करें
I. माता और पिता का समास करें।
II. ‘रसोई घर’ के निम्नलिखित विकल्पों में से सही समास-विग्रह विकल्प को चुनें:
(क) रसोई का घर (ख) रसोई के लिए घर (ग) रसोई में घर (घ) रसोई से घर।

III. निम्नलिखित कथने में सही अथवा ग़लत लिखें:
‘तिरंगा’ शब्द का समास विग्रह होगा-‘तीन रंगों का समूह’।

Set-B
I. ‘उत्तर और दक्षिण’ का समास करें।
II. ‘राह खर्च’ पद के निम्नलिखित विकल्पों में से सही समास विकल्पों में से सही समास-विग्रह को चुनें
(क) राह में खर्च (ख) राह को खर्च (ग) राह से खर्च (घ) राह के लिए खर्च।
III. ‘त्रिनेत्र’ शब्द का समास विग्रह होगा-तीन हैं नेत्र जिसके अर्थात् शिव

Set-C
I. निम्नलिखित पदों का समास करें। राजा का कुमार।
II. ‘यथानियम’ पद के निम्नलिखित विकल्पों में से सही समास-विग्रह विकल्प को चुनें:
(क) यथा का नियम (ख) नियम के अनुसार (ग) यथा और नियम (घ) यथा के नियम। .
III. निम्नलिखित कथन में सही अथवा ग़लत लिखें
‘पीताम्बर’ शब्द का समास विग्रह होगा-पी लिया है अम्बर जिसने।

Set-A, B, C
(i) निम्नलिखित पदों का समास करें: लोभ और मोह।
(ii) ‘घनश्याम’ पद के निम्नलिखित में से सही समास-विग्रह विकल्प को चुनें :
(अ) घन के लिए श्याम (ब) घन के समान श्याम (स) घन से श्याम (द) घन से श्याम।
(iii) निम्नलिखित कथन में सही अथवा ग़लत लिखें :
‘त्रिफला’ शब्द का समास विग्रह होगा-‘तीन फलों का समूह’।

Set-A, B, C
(i) ‘गुरु दक्षिणा’ के लिए विग्रह का सही विकल्प चुनकर लिखें:
(क) गुरु और दक्षिणा (ख) गुरु के लिए दक्षिणा (ग) गुरु की दक्षिणा (घ) गुरु द्वारा दक्षिणा।
(ii) ‘जन्माध’ का विग्रह होगा-‘जन्म से अंधा’, सही या गलत लिखकर उत्तर दें।

Set-A, B, C
I. निम्नलिखित पदों का समास करें
देश का वासी।
II. ‘रसोई घर’ पद के निम्नलिखित विकल्पों में से सही समास-विग्रह विकल्प को चुनें :
(क) रसोई घर (ख) रसोई के लिए घर (ग) रसोई में घर (घ) रसोई से घर।
III. ‘मालगाड़ी’ शब्द का समास विग्रह होगा-‘माल की गाड़ी’।

बहुविकल्पीय प्रश्नोत्तर

प्रश्न 1.
जिस समास में पूर्व और उत्तर दोनों पद प्रधान होते हैं उसे कहते हैं?
(क) द्विगु
(ख) द्वन्द्व
(ग) कर्मधारय
(घ) अव्ययीभाव।
उत्तर:
(ख) द्वन्द्व

प्रश्न 2.
जिसमें अन्य पद प्रधान हो उसे कहते हैं?
(क) अव्ययीभाव
(ख) बहुव्रीहि
(ग) तत्पुरुष
(घ) द्विगु।
उत्तर:
(ख) बहुब्रीहि

प्रश्न 3.
‘आजीवन’ में कौन-सा समास है?
(क) तत्पुरुष
(ख) कर्मधारय
(ग) बहुव्रीहि
(घ) अव्ययीभाव।
उत्तर:
(घ) अव्ययीभाव

प्रश्न 4.
‘आबोहवा’ में निहित समास है
(क) द्विगु
(ख) कर्मधारय
(ग) द्वन्द्व
(घ) तत्पुरुष।
उत्तर:
(ग) द्वन्द्व

प्रश्न 5.
‘सत् जो जन’ में कौन-सा समास निहित है?
(क) कर्मधारय
(ख) बहुब्रीहि
(ग) अव्ययीभाव
(घ) द्वन्द्व।
उत्तर:
(क) कर्मधारय।

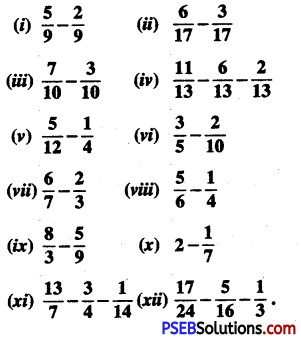
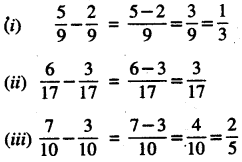
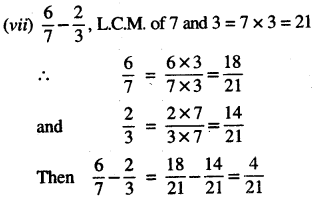
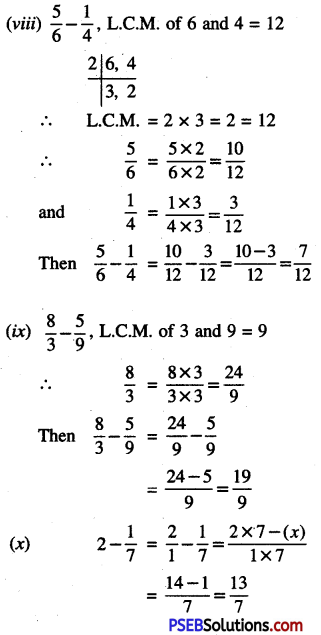
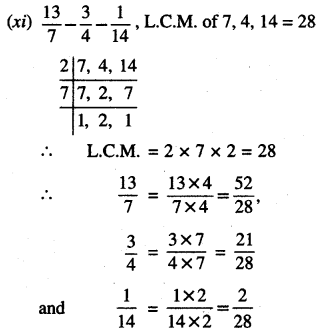
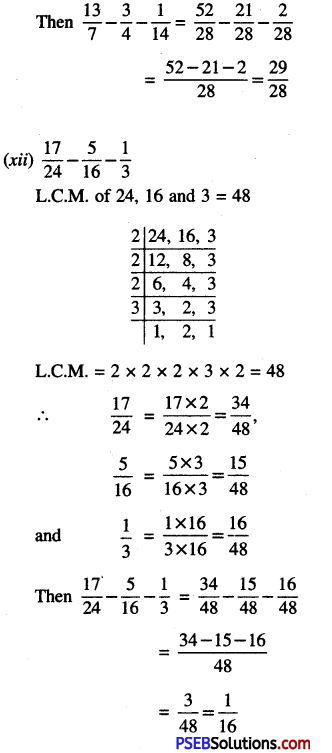
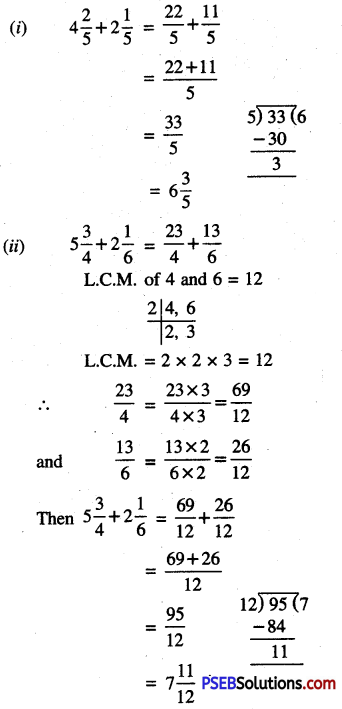
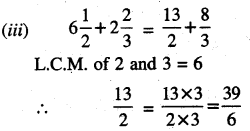
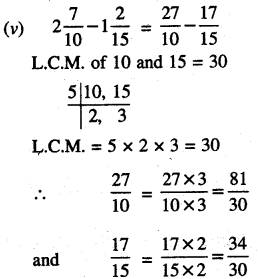
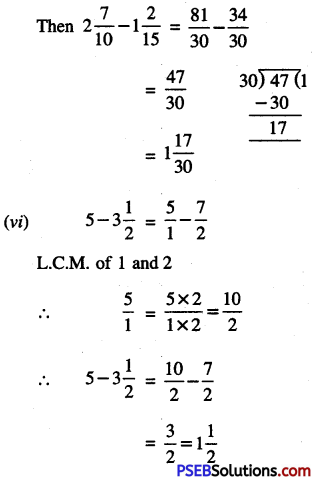
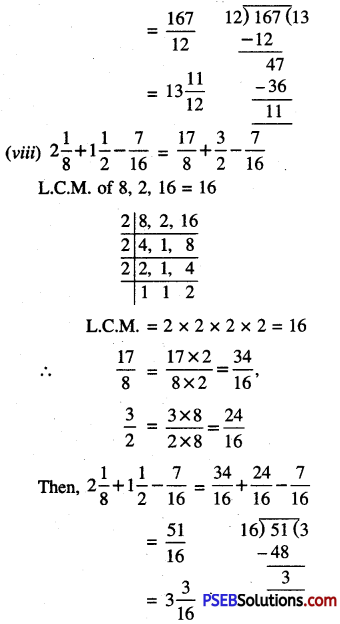
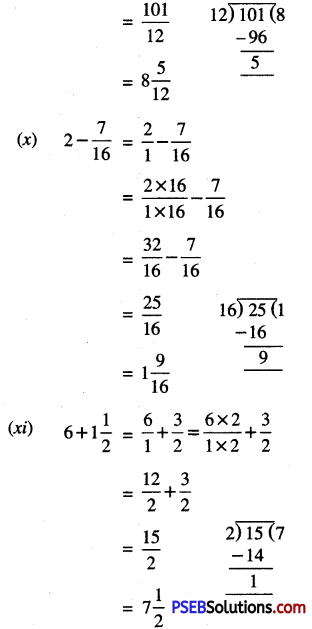
Punjab State Board PSEB 10th Class Maths Book Solutions Chapter 5 Arithmetic Progressions Ex 5.2 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 10 Maths Chapter 5 Arithmetic Progressions Ex 5.2

Question 1
Fill in the blanks in the following table, given that a is the first term, d the common difference and a the nth term of the
AP:

Solution:
(i) Here a = 7, d = 3, n = 8
∵ a_n = a + (n – 1)d
∴ a₈ = 7 + (8 – 1)3
= 7 + 21 = 28.

(ii) Here a = – 18, n = 10, a_n = 0
∵ a_n = a + (n – 1)d
∴ a₁₀ = – 18 + (10 – 1)d
or 0 = – 18 + 9d .
or 9d = 18
d = \(\frac{18}{2}\) = 2.

(iii) Here d = – 3, n = 18, a_n = – 5
∵ a_n = a + (n – 1)d
∴ a₁₈ = a + (18 – 1)(-3)
or -5 = a – 51
or a = – 5 + 51 = 46.

(iv) Here a = – 18.9, d = 2.5 a_n = 3.6
∵ a_n = a + (n – 1)d
∴ 3.6 = – 18.9 + (n – 1) 2.5
or 3.6 + 18.9 = (n – 1) 2.5
or (n – 1) 2.5 = 22.5
or n – 1 = \(\frac{22.5}{2.5}\)
or n = 9 + 1 = 10.

(v) Here a = 3.5, d = 0, n = 105
∵ a_n = a + (n – 1) d
∴ a_n = 3.5 + (105 – 1) 0
a_n = 3.5 + 0 = 3.5.

Question 2.
Choose the correct choice in the following and justify:
(i) 30th term of the AP: 10, 7, 4, …………….. is
(A) 97 (B) 77 (C) – 77 (D) – 87

(ii) 11th term of the AP: – 3, – \(\frac{1}{2}\), 2, ………. is
(A) 28 (B) 22 (C) – 38 (D) – 48\(\frac{1}{2}\)

Solution:
(i) Given A.P. is 10, 7, 4 ……………
T₁ = 10, T₂ = 7, T₃ = 4
T₂ – T₁ = 7 – 10 = – 3
T₃ – T₂ = 4 – 7 = – 3
∵ T₂ – T₁ = T₃ – T₂ = – 3 = d(say)
∵ T_n = a + (n – 1) d
Now, T₃₀ = 10 + (30 – 1)(-3)
= 10 – 87 = – 77
∴ Correct choice is (C).

(ii) Given A.P. is – 3, –\(\frac{1}{2}\), 2, ……….
T₁ = – 3 T₂ = –\(\frac{1}{2}\), T₃ = 2, …………..
T₂ – T₁ = –\(\frac{1}{2}\) + 3 = \(\frac{-1+6}{2}=\frac{5}{2}\)
T₃ – T₂ = 2 + \(\frac{1}{2}\) = \(\frac{4+1}{2}=\frac{5}{2}\)
∵ T₂ – T₁ = T₃ – T₂ = \(\frac{5}{2}\) = d(say)
∵ T_n = a + (n – 1) d
Now, T₁₁ = -3 + (11 – 1) \(\frac{5}{2}\)
= -3 + 10 × \(\frac{5}{2}\) = – 3 + 25 = 22
∴ Correct choice is (B).

Question 3.
In the following APs, find the missing terms in the boxes:
(i) 2, , 26
(ii), 13, , 3
(iii) 5,, , 9
(iv) – 4, , , , , 6
(v) , 38, , , , – 22
Solution:
Let a be the first term and ‘d’ be the common difference of given A.P.
(i) Here T₁ = a = 2
and T₃ = a + 2d = 26
or 2 + 2d = 26
or 2d = 26 – 2 = 24
or d = 12
∴ Missing term = T₂ = a + d = 2 + 12 = 14.

(ii) Here, T₂ = a + d = 13 ……………(1)
and T₄ = a + 3d = 3 …………….(3)
Now, (2) – (1) gives

Substitute this value of d in (1), we get
a – 5 = 13
a = 13 + 5 = 18.
∴ T₁ = a = 18
T₃ = a + 2d = 18 + 2(-5)
= 18 – 10 = 8.

(iii)Here T₁ = a = 5
and T₄ = a + 3d = 9
or a + 3d = \(\frac{19}{2}\)
or 5 + 3d = \(\frac{19}{2}\)
or 3d = \(\frac{19}{2}\) – 5
or 3d = \(\frac{19-10}{2}=\frac{9}{2}\)
or d = \(\frac{9}{2} \times \frac{1}{3}=\frac{3}{2}\)
T₂ = a + d = 5 + \(\frac{3}{2}\)
= \(\frac{10+3}{2}=\frac{13}{2}\)
T₃ = a + 2d = 5 + 2(\(\frac{3}{2}\)) = 5 + 3 = 8.

(iv) Here T₁ = a = —
T₆ = a + 5d = 6
or -4 + 5d = 6
or 5d = 6 + 4
or 5d = 10
or d = \(\frac{10}{2}\) = 2
Now, T₂ = a + d = -4 + 2 = -2
T₃ = a + 2d = – 4 + 2(2)
= – 4 + 4 = 0
T₄ = a + 3d = – 4 + 3(2)
= – 4 + 6 = 2
T₅ = a + 4d = – 4 + 4(2)
= – 4 + 8 = 4

(v) Here T₂ = a + d = 38 ………….(1)
and T₆ = a + 5d = -22 ……………(2)
Now, (2) – (1) gives

Substitute this value of d in (1), we get
a + (-15) = 38
a = 38 + 15 = 53
∴ T₁ = a = 53
T₃ = a + 2d = 53 + 2(-15) = 53 – 30 = 23.
T₄ = a + 3d = 53 + 3(-15) = 53 – 45 = 8
T₅ = a + 4d = 53 + 4(-15) = 53 – 60 = – 7.

Question 4
Which term of the A.P. 3, 8, 13, 18, …………… is 78?
Solution:
Given A.P. is 3, 8, 13, 18, ………….
T₁ = 3, T₂ = 8, T₃ = 13, T₄ = 18
T₂ – T₁ =8 – 3=5
T₃ – T₂= 13 – 8=5
T₂ – T₁ = T₃ – T,= 5 = d (say)
Using, T_n = a + (n – I) d
or 78 = 3 + (n – 1) 5
or 5(n – 1) = 78 – 3 = 75
or n – 1 = 15
or n = 15 + 1 = 16
Hence, 16th term of given AP. is 78.

Question 5.
Find the number of terms in each of the following APs:
(i) 7, 13, 19,…, 205
(ii) 18, 15\(\frac{1}{2}\), 13, ………….., – 47
Solution:
(i) Given A.P. is 7, 13, 19, …………..
T₁ = 7, T₂ = 13, T₃ = 19
T₂ – T₁ = 13 – 7 = 6
T₃ – T₂ = 19 – 13 = 6
T₂ – T₁ = T₃ – T₂ = 6 = d(say)
Using formula, T_n = a + (n – 1) d
205 = 7 + (n – 1) 6
or (n – 1) 6 = 205 – 7 = 198
or (n – 1) = \(\frac{198}{6}\)
or n – 1 = 33
n = 33 + 1 = 34
Hence, 34th term of an AP. is 205.

(ii) Given A P. is 18, 15\(\frac{1}{2}\), 13, …………..
T₁ = 18, T₂ = 15\(\frac{1}{2}\) = \(\frac{31}{2}\), T₃ = 13
T₂ – T₁ = \(\frac{31}{2}\) – 18 = \(\frac{31-36}{2}=-\frac{5}{2}\)
T₃ – T₂ = 13 – \(\frac{31}{2}\) = \(\frac{26-31}{2}=-\frac{5}{2}\)
∵ T₂ – T₁ = T₃ – T₂ = \(\frac{-5}{2}\) = d (say)
Using formula. T_n = a + (n – 1) d
– 47 = 18 + (n – 1) \(\frac{-5}{2}\)
or (n – 1) (\(\frac{-5}{2}\)) = – 47 – 18
or (n – 1) (\(\frac{-5}{2}\)) = – 65
or n – 1 = – 65 × – \(\frac{2}{5}\)
or n – 1 = 26
or n = 26 + 1 = 27
Hence, 27th term of an A.P. is – 47.

Question 6.
Is – 150 a term of 11, 8, 5, 2….? why?
Solution:
Given sequence is 11, 8, 5, 2, ………..
T₁ = 11, T₂ = 8, T₃ = 5, T₄ = 2
T₂ – T₁ = 8 – 11 = – 3
T₃ – T₂ = 5 – 8 = – 3
T₄ – T₃ = 2 – 5 = – 3
T₂ – T₁ = T₃ – T₂ = T₄ – T₃ = – 3 = d (say).
Let – 150 be any term of given A.P.
then T_n = – 150
a+(n – 1)d = – 150
or 11 +(n – 1)(- 3) = – 150
or (n – 1)( – 3) = – 150 – 11 = – 161
or n – 1 = \(\frac{161}{3}\)
or n = \(\frac{161}{3}\) + 1 = \(\frac{161+3}{3}\)
n = \(\frac{164}{3}\) = 54\(\frac{2}{3}\),
which is not a natural number.
Hence, – 150 cannot be a term of given A.P.

Question 7.
Find the 31st term of an AP whose 11th term is 38 and 16th term is 73.
Solution:
Let ‘a’ and 4d’ be the first term and common difference of given A.P.
Given that T₁₁ = 38
a +(11 – 1) d = 38
[∵ T_n = a + (n – 1) d]
a + 10 d = 38
and T₁₆ = 73
a + (16 – 1) d = 73
[∵ T_n = a + (n – 1) d]
a + 15 d = 73
Now, (2) – (1) gives

Substitute this value of d in (1), we get
a + 10 (7) = 38
or a + 70 = 38
or a = 38 – 70 = – 32
Now, T₃₁ = a + (31 – 1) d
= – 32 + 30 (7) = – 32 + 210 = 178.

Question 8.
An AP consists of 50 terms of which 3rd term is 12 and the last term is 106. Find the 291h term.
Solution:
Let ‘a’ and ‘d’ be the first term and common difference of given A.P.
Given that, T₃ = 12
a + (3 – 1) d = 12
∵ T_n = a + (n – 1) d
or a + 2d = 12 ………………(1)
and Last term = T₅₀ = 106
a + (50 – 1) d = 106
∵ T_n = a + (n – 1) d
a + 49 d = 106 ……………(2)
Now, (2) – (1) gives

Substitute this value of d in (1), we get
a + 2(2) = 12
or a + 4 = 12
or a + 12 – 4 = 8
Now, T₂₉ = a + (29 – 1) d
= 8 + 28 (2) = 8 + 56 = 64.

Question 9.
If the 3rd and 9th tenus of an A.P. are 4 and – 8 respectively, which term of this A.P. is zero.
Solution:
Let ‘a’ and ‘d’ be the first term and common difference of given AP.
Given that: T₃ = 4
a + (3 – 1) d = 4
∵ T_n = a + (n – 1) d
a + 2d = 4 …………..(1)
and T₉ = – 8
a + (9 – 1) d = 8
and T₉ = – 8
a + (9 – 1)d = 8
∵ T_n = a + (n – 1) d
or a + 8d = – 8
Now, (2) – (1) gives

Substitute this value of d in (1), we get
a + 2(- 2) = 4
or a – 4 = 4
or a = 4 + 4 = 8
Now, T_n = 0 (Given)
a + (n – 1) d = 0
or 8 + (n – 1)(- 2)=0
or -2 (n – 1) = – 8
or n – 1 = 4
or n = 4 + 1 = 5
Hence, 5th term of an AP. is zero.

Question 10.
The 17th term of an A.P. exceeds its 10th term by 7. Find the common difference.
Solution:
Let ‘a’ and ‘d’ be the first term and common difference of given A.P.
Now, T₁₇ = a + (17 – 1) d = a + 16 d
and T₁₀ = a + (10 – 1) d = a + 9 d
According to question
T₁₇ – T₁₀ = 7
(a + 16 d) – (a + 9 d) = 7
or a + 16 d – a – 9 d = 7
7 d = 7
or d = \(\frac{7}{7}\) = 1
Hence, common difference is 1.

Question 11.
Which term of the A.P. 3, 15, 27, 39, …………. will be 132 more than its 54th term?
Solution:
Let ‘a’ and ‘d’ be the first term and common difference of given A.P.
Given A.P. is 3, 15, 27, 39, …
T₁ = 3, T₂ = 15, T₃ = 27, T₄ = 39
T₂ – T₁ = 15 – 3 = 12
T₃ – T₂ = 27 – 15 = 12
:. d=T₂ – T₁ = T₃ – T₂ =12
Now, T₅₄ = a + (54 – 1) d
= 3 + 53 (12) = 3 + 636 = 639
According to question
T = T₅₄ + 132
a + (n – 1)d = 639 + 132
3 + (n – 1)(12) = 771
(n – 1) 12 = 771 – 3 = 768
or n – 1 = \(\frac{768}{12}\) = 64
or n = 64 + 1 = 65
Hence, 65th term of A.P. is 132 more than its 54th term.

Question 12.
Two APs have the same common difference. The difference between their 100th terms is 100, what is the difference between their 1000th terms?
Solution:
Let ‘a’ and ‘d’ be the first term and common difference of first AP.
Also, ‘A’ and ‘d’ be the first term and common difference of second A.P.
According to question
[T₁₀₀ of second A.P.] – [T₁₀₀ of first A.P.] = 100
or[A +(100 – 1)d] – [a +(100 – 1)d] = 100
or A + 99d – a – 99d = 100
or A – a = 100
Now, [T₁₀₀₀ of second A.P.] – [T₁₀₀₀ of first A.P.]
= [A + (1000 – 1) d) – (a + (1000 – 1) d]
= A + 999 d – a – 999 d
= A – a = 100 [Using (I)].

Question 13.
How many three-digits numbers are divisible by 7?
Solution:
Three digits numbers which divisible by 7 are 105, 112, 119 , 994
Here a = T₁ = 105, T₂ = 112, T₃ = 119 and T_n = 994
T₂ – T₁ = 112 – 105=7
T₃ – T₂ = 119 – 112=7
∴ d = T₂ – T₁ = T₃ – T₂ = 7
Given that, T_n = 994
a + (n – 1) d = 994
or 105 + (n – 1) 7 = 994
or (n – 1) 7 = 994 – 105
or (n – 1) 7 = 889
or n – 1 = \(\frac{889}{7}\) = 127
or n = 127 + 1 = 128.
Hence, 128 terms of three digit number are divisible by 7.

Question 14.
How many multiples of 411e between 10 and 250?
Solution:
Multiples of 4 lie between 10 and 250 are 12, 16, 20, 24, … 248
Here a = T₁ = 12, T₂ = 16, T₃ = 20 and T_n = 248
T₂ – T₁ = 16 – 12 = 4
T₃ – T₂ = 20 – 16 = 4
∴ d = T₂ – T₁ = T₃ – T₂ = 4
Given that, T_n = 248
a + (n – 1) d = 248
or 12 + (n – 1)4 = 248
or 4(n – 1) = 248 – 12 = 236
or n – 1 = \(\frac{236}{4}\) = 59
or n = 59 + 1 = 60
Hence, there are 60 terms which are multiples of 4 lies between 10 and 250.

Question 15.
For what value of n, are the n terms of two A.P.s 63, 65, 67, …………. and 3, 10, 17, …………….. equal?
Solution:
Given A.P. is 63, 65, 67, ……………..
Here a = T₁ = 63, T₂ = 65, T₃ = 67
T₂ – T1 = 65 – 63 = 2
T₃ – T₂ = 67 – 65 = 2
∴ d = T₂ – T₁ = T₃ – T₂ = 2
and second A.P. is 3, 10, 17, …
Here a = T₁ = 3, T₂ = 10, T₃ = 17
T₂ – T₁ = 10 – 3 = 7
T₃ – T₂ = 17 – 10 = 7
According to question.
[nth term of first A.P.] = [nth term of second A.P.]
63 + (n – 1)2 = 3 + (n – 1) 7
or 63 + 2n – 2 = 3 + 7n – 7
or 61 + 2n = 7n – 4
or 2n – 7n = – 4 – 61
– 5n = – 65
n = \(\frac{65}{5}\) = 13.

Question 16.
Determine the AP. whose third term is 16 and 7 term exceeds the by 12.
Solution:
Let ‘a’ and ‘d’ be the first term and common difference of given A.P.
Given that T₃ = 16
a + (3 – 1) d = 16
a + 2d = 16
According to question
T₇ – T₅ = 12
[a + (7 – 1) d] – [a + (5 -1) d] = 12
a + 6 d – a – 44 = 12
2d = 12
d = \(\frac{12}{2}\) = 6
Substitute this value of d in (1), we get
a + 2(6) = 16
a = 16 – 12 = 4 .
Hence, given A.P. are 4, 10, 16, 22, 28, ………….

Question 17.
Find the 20th term from the last term of the AP: 3, 8, 13, ………., 253.
Solution:
Given A.P. is 3, 8, 13, …………., 253
Here, a = T₁ = 3, T₂ = 8, T₃ =13 and T_n = 253
T₂ – T₁ = 8 – 3 = 5
T₃ – T2 = 13 – 8 = 5
∴ d = T₂ – T₁ = T₃ – T₁ = 5
Now, T_n = 253
3 + (n – 1)5 = 253
∵ T_n = a + (n – 1) d
(n – 1) 5 = 250
n-1 = \(\frac{250}{5}\) = 50
n = 50 + 1 = 51
20th term from the end of AP = (Total number of terms) – 20 + 1
= 51 – 20 + 1 = 32nd term
∴ 20th term from the end of AP
= 32nd term from the starting
= 3 + (32 – 1)5
∵ Tn = a + (n – 1)d
= 3 + 31 × 5
= 3 + 155 = 158.

Question 18.
The sum of the 4th and 8th term of an AP is 24 and the sum of the 6’ and 10th terms is 44. FInd the first three terms of the A.P.
Solution:
Let ‘a’ and ‘d’ be the first term and common difference of given A.P.
According to 1st condition
T₄ + T₈ = 24
a + (4 – 1) d + a + (8 – 1) d = 24
∵ T_n = a + (n – 1) d
or 2a + 3d + 7d = 24
2a + 10d = 24
a + 5d = 12 …………(1)
According to 2nd condition
T₆ + T₁₀ = 44
a + (6 – 1) d + a +(10 – 1) d = 44
∵ T_n = a + (n – 1) d
2a + 5d + 9d = 44
2a + 14d = 44
a + 7d = 22
Now (2) – (1) gives

Substitute this value of d in (I). we get
a + 5(5) = 12
a + 25 = 12
a = 12 – 25 = -13
T₁ = a = -13
T₂ = a + d = 13 + 5 = -8
T₂ = a + 2d = – 13 + 2(5) = – 13 + 10 = -3
Hence, given A.P. is -13, -8, -3, ……………

Question 19.
Subba Rao started work in 1995 at an annual salary of ₹ 5000 and received an increment of ₹ 200 each year. In which year did his income reach ₹ 7000?
Solution:
Subba Rao’s starting salary = ₹ 5000
Annual increment = ₹ 200
Let ‘n’ denotes number of years.
∴ first term = a = ₹ 5000
Common diflerence = d = ₹ 200
and T_n = ₹ 7000
5000 + (n – 1) 200 = 7000
∵ T_n = a + (n – 1) d
(n – 1) 200 = 7000 – 5000
or (n – 1) 200 = 2000
or n – 1 = \(\frac{2000}{200}\) = 10
or n = 10 + 1 = 11
Now, in case of year the sequence are 1995. 1996, 1997, 1998, ……………
Here a = 1995, d = 1 and n = 11
Let T_n denotes the required year.
∴ T_n = 1995 + (11 – 1) 1
= 1995 + 10 = 2005
Hence, in 2005, Subba Rao’s salary becomes 7000.

Question 20.
Ramkali saved ₹ 5 in the first week of a year and then increased her weekly saving by ₹ 1.75. If in the nth week, her weekly saving becomes ₹ 20.75, find n.
Solution:
Amount saved in first week = ₹ 5
Increment in saving every week = ₹ 1.75
It is clear that, it form an A.P. whose terms are
T₁ = 5, d = 1.75
∴ T₂ = 5 + 1.75 = 6.75
T₃ = 6.75 + 1.75 = 8.50
Also. T_n = 20. 75 (Given)
5 + (n – 1) 1.75 = 20.75
∵ T_n = a + (n – 1) d
or (n – 1) 1.75 = 20.75 – 5
or (n – 1) 1.75 = 15.75
or (n – 1) = \(\frac{1575}{100} \times \frac{100}{175}\)
or n – 1 = 9
or n = 9 + 1 = 10
Hence, in 10th week, Ramkali’s saving becomes ₹ 20.75.

Punjab State Board PSEB 9th Class Maths Book Solutions Chapter 8 Quadrilaterals Ex 8.2 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 9 Maths Chapter 8 Quadrilaterals Ex 8.2

Question 1.
ABCD is a quadrilateral in which F Q, R and S are midpoints of the sides AB, BC, CD and DA respectively (see the given figure 1). AC is a diagonal. Show that:
(i) SR || AC and SR = \(\frac{1}{2}\) AC
(ii) PQ = SR
(iii) PQRS is a parallelogram.

Answer:
In ∆ DAC, S and R are the midpoints of DA and DC respectively.
Through C draw a line parallel to AD which intersects line SR at T.
In ∆ DRS and ∆ CRT
∠ DRS = ∠ CRT (Vertically opposite angles)
∠ RSD = ∠ RTC (Alternate angles formed by transversal ST of DS || TC)
DR = CR (R is the midpoint of DC.)
∴ ∆ DRS ≅ ∆ CRT (AAS rule)
∴ DS = CT and SR = RT (CPCT)
As S is the midpoint of DA, we have DS = SA.
∴ SA = CT
And, by construction, SA || CT.
∴ Quadrilateral SACT is a parallelogram.
∴ ST || AC
∴ SR || AC ………… (1)
Now, SR = RT gives SR = \(\frac{1}{2}\)ST
In parallelogram SACT, ST = AC.
∴ SR = \(\frac{1}{2}\)AC ……………. (2)
Taking (1) and (2) together,
SR || AC and SR = \(\frac{1}{2}\)AC ….. Result (1)
Similarly, in ∆ ABC, P and Q are the midpoints of AB and BC respectively. ,
∴ PQ || AC and PQ = \(\frac{1}{2}\)AC
Now, SR = \(\frac{1}{2}\)AC and PQ = \(\frac{1}{2}\)AC
∴ PQ = SR …… Result (ii)
Similarly, SR || AC and PQ || AC.
∴ PQ || SR
Thus, in quadrilateral PQRS, PQ = SR and PQ || SR.
Hence, by theorem 8.8, PQRS is a parallelogram. … Result (iii)

Question 2.
ABCD is a rhombus and F Q, R and S are the midpoints of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rectangle.
Answer:

ABCD is a rhombus and F Q, R and S are the midpoints of sides AB, BC, CD and DA respectively.
∴ In ∆ ABC, PQ || AC and PQ = \(\frac{1}{2}\)AC.
∴ In ∆ ADC, SR || AC and SR = \(\frac{1}{2}\)AC.
Hence, in quadrilateral PQRS, PQ || SR and PQ = SR.
∴ Quadrilateral PQRS is a parallelogram.
Now, since ABCD is a rhombus, AC and BD bisect each other at right angles at M.
∴ ∠ AMB = 90°
Now, AC || PQ and MN is their transversal.
∴ ∠ AMN + ∠ MNP = 180° (Interior angles on the same side of transversal)
∴ ∠ AMB + ∠MNP = 180°
∴ 90° + ∠ MNP = 180°
∴ ∠ MNP = 90°
In ∆ ABD, P and S are the midpoints of AB and AD respectively.
∴ PS || BD and NP is their transversal.
∴ ∠ DNP + ∠ NPS = 180°
∴ ∠ MNP + ∠ NPS =180°
∴ 90° + ∠ NPS = 180°
∴ ∠ NPS = 90°
∴ ∠ SPQ = 90°
Thus, in parallelogram PQRS, one angle ∠P is a right angle.
Hence, quadrilateral PQRS is a rectangle.

Question 3.
ABCD is a rectangle and P, Q, R and S are midpoints of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rhombus.
Answer:

Since ABCD is a rectangle, its diagonals are equal.
∴ AC = BD
∴ \(\frac{1}{2}\)AC = \(\frac{1}{2}\)BD
In ∆ ABC, P and Q are the midpoints of AB and BC respectively.
∴ PQ = \(\frac{1}{2}\)AC
Similarly, in ∆ ADC, SR = \(\frac{1}{2}\)AC; in ∆ ABD, SP = \(\frac{1}{2}\) BD and in ∆ BCD, QR = \(\frac{1}{2}\) BD.
Now, PQ = SR = \(\frac{1}{2}\)AC, SP = QR = \(\frac{1}{2}\)BD and \(\frac{1}{2}\)AC = \(\frac{1}{2}\)BD
Hence, in quadrilateral PQRS,
PQ = QR = RS = SP
Thus, all the sides of quadrilateral PQRS are equal.
Hence, quadrilateral PQRS is a rhombus.

Question 4.
ABCD is a trapezium in which AB || DC, BD is a diagonal and E is the midpoint of AD. A line is drawn through E parallel to AB intersecting BC at F (see the given figure). Show that F is the midpoint of BC.

Answer:
Suppose line EF drawn through E and parallel to AB intersects BD at M.
EF || AB and AB || DC
∴ EF || DC
Trapezium ABCD is divided into two triangles, ∆ ABD and ∆ BCD, by diagonal BD.
In ∆ ABD, E is the midpoint of AD and a line through E and parallel to AB intersects BD at M.
Hence, by theorem 8.10, M is the midpoint of BD.
Now, in ∆ BCD, M is the midpoint of BD and a line through M and parallel to CD intersects BC at F.
Hence, by theorem 8.10, F is the midpoint of BC.
Note: The following result about the length of EF can also be derived:
EF = \(\frac{1}{2}\)(AB + CD)
Moreover, if X and Y are the midpoints of the diagonals of above trapezium ABCD, then XY = \(\frac{1}{2}\)|AB – CD|.

Question 5.
In a parallelogram ABCD, E and F are the midpoints of sides AB and CD respectively (see the given figure). Show that the line segments AF and EC trisect the diagonal BD.

Answer:
E and F are the midpoints of AB and CD respectively.
∴ AE = \(\frac{1}{2}\)AB and CF = \(\frac{1}{2}\)CD
In parallelogram ABCD, AB = CD and AB || CD.
∴ AE = CF and AE || CF
Hence, quadrilateral AECF is a parallelogram.
∴ AF || EC
∴ AP || EQ
In ∆ ABP E is the midpoint of AB and EQ || AR
∴ Q is the midpoint of PB. (Theorem 8.10)
∴PQ = QB …………… (1)
Similarly, in ∆ DQC, F is the midpoint of DC and FP || CQ.
∴ P is the midpoint of DQ. (Theorem 8.10)
∴ DP = PQ …………….. (2)
From (1) and (2), DP = PQ = QB.
Moreover, DP + PQ + QB = BD.
Thus, AF and EC trisect the diagonal BD.

Question 6.
Show that the line segments joining the midpoints of the opposite sides of a quadrilateral bisect each other.
Answer:

In quadrilateral ABCD, P Q, R and S are the midpoints of sides AB, BC, CD and DA respectively.
In ∆ ABC, P and Q are the midpoints of AB and BC respectively.
∴ PQ || AC and PQ = \(\frac{1}{2}\)AC …………….. (1)
In ∆ ADC, S and R are the midpoints of DA and DC respectively.
∴ SR || AC and SR = \(\frac{1}{2}\)AC ……………… (2)
From (1) and (2),
PQ = SR and PQ || SR.
Thus, in quadrilateral PQRS, sides in one pair of opposite sides are equal and parallel. Hence, quadrilateral PQRS is a parallelogram. The diagonals of a parallelogram bisect each other. [Theorem 8.6]
∴ PR and SQ bisect each other.
Thus, the line segments joining the midpoints of the opposite sides of a quadrilateral bisect each other.

Question 7.
ABC is a triangle right angled at C. A line through the midpoint M of hypotenuse AB and parallel to BC intersects AC at D. Show that:
(i) D is the midpoint of AC.
(ii) MD ⊥ AC
(iii) CM = MA = \(\frac{1}{2}\)AB
Answer:

In ∆ ABC, ∠ C is a right angle and M is the midpoint of hypotenuse AB. A line through M and parallel to BC intersects AC at D.
Hence, by theorem 8.10, DM bisects AC.
∴ D is the midpoint of AC. ….. Result (i)
In ∆ ABC, ∠ C is a right angle.
∴ ∠ C = 90°
Now, BC || DM and DC is their transversal.
∴ ∠ MDC + ∠ DCB = 180° (Interior angles on the same side of transversal)
∴ ∠ MDC + 90° = 180°
∴ ∠ MDC = 90°
Thus, MD is perpendicular to AC.
∴ MD ⊥ AC …… Result (ii)
Now, in ∆ ADM and ∆ CDM,
AD = CD (D is the midpoint of AC)
∠ ADM = ∠ CDM (Right angles)
DM = DM (Common)
∴ ∆ ADM ≅ ∆ CDM (SAS rule)
∴ AM = CM (CPCT) ……………. (1)
Now, M is the midpoint of AB.
∴ AM = \(\frac{1}{2}\)AB …… (2)
< Prom (1) and (2),
CM = MA = \(\frac{1}{2}\)AB …… Result (iii)

Punjab State Board PSEB 9th Class Maths Book Solutions Chapter 8 Quadrilaterals Ex 8.1 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 9 Maths Chapter 8 Quadrilaterals Ex 8.1

Question 1.
The angles of a quadrilateral are in the ratio 3: 5 : 9: 13. Find all the angles of the quadrilateral.
Answer:
Let, ABCD be a given quadrilateral.
∴ ∠A : ∠B : ∠C : ∠D = 3 : 5 : 9 : 13
Sum of ratios = 3 + 5 + 9 + 13 = 30
In quadrilateral ABCD, ∠A + ∠B + ∠C + ∠D = 360°
∴ ∠A = \(\frac{3}{30}\) × 360° = 3 × 12 = 36°
∴ ∠B = \(\frac{5}{30}\) × 360° = 5 × 12 = 60°
∴ ∠C = \(\frac{9}{30}\) × 360° = 9 × 12 = 108°
∴ ∠D = \(\frac{13}{30}\) × 360° = 13 × 12 = 156°
Thus the angles of the given quadrilateral are 36°, 60°, 108° and 156°.

Question 2.
If the diagonals of a parallelogram are equal, then show that it is a rectangle.

Answer:
In parallelogram ABCD, diagonals are equal.
∴ AC = BD.
In ∆ DAB and ∆ CBA.
DA = CB (Theorem 8.2)
AB = BA (Common)
DB = CA (Given)
∴ ∆ DAB ≅ ∆ CBA sss rule)
∴ ∠ DAB = ∠CBA (CPCT)
In parallelogram ABCD, AD || BC and AB is their transversal.
∴ ∠ DAB + ∠ CBA = 180°
(Interior angles on the same side of transversal)
Thus, in parallelogram ABCD, two angles ∠A and∠B are right angles. Hence, all the angles are right angle.
Hence, the parallelogram ABCD having equal diagonals is a rectangle.

Question 3.
Show that if the diagonals of a quadrilateral bisect each other at right angles, then it is a rhombus.

Answer:
In quadrilater ABCD. diagonals AC and BD bisect each other at M at right angles.
∴ AM = CM, BM = DM and
∠AMB = ∠CMB = ∠CMD = ∠AMD = 90°.
In ∆ AMB and ∆ CMB,
AM = CM
∠ AMB = ∠CMB
BM = BM (Common)
∴ ∆ AMB ≅ ∆ CMB (SAS rule)
∴ AB = CB (CPCT)
Similarly, proving ∆ BMC ≅ ∆ DMC and ∆ DMA ≅ ∆ BMA, we get BC = DC and DA = BA.
Thus, in quadrilateral. ABCD.
AB = BC CD = DA.
Therefore, quadrilateral ABCD is a rhombus.
Thus, if the diagonals of a quadrilateral bisect each other at right angles, then it is a rhombus.

Question 4.
Show that the diagonals of a square are equal and bisect each other at right angles.

Answer:
ABCD is a square in which diagonals AC and BD intersect at M.
Every square is a parallelogram.
∴ AC and BD bisect each other. …………… (1)
In ∆ DAB and ∆ CBA,
DA = CB (Sides of a square)
∠ DAB = ∠ CBA (Right angles in a square)
AB = BA (Common)
∴ ∆ DAB ≅ ∆ CBA (SAS rule)
∴ BD = AC (CPCT) ……………….. (2)
Now, in ∆ AMB and ∆ CMB,
AM = CM (BD bisects AC at M).
BM = BM (Common)
AB = CB (Sides of a square)
∴ ∆ AMB ≅ ∆ CMB (SSS rule)
∴ ∠ AMB = ∠CMB (CPCT)
But, ∠ AMB and ∠ CMB form a linear pair.
∴ ∠ AMB + ∠ CMB = 180°
Hence, ∠AMB = ∠ CMB = 90° (3)
(1), (2) and (3) taken together proves that the diagonals of a square are equal and bisect each other at right angles.

Question 5.
Show that if the diagonals of a quadrilateral are equal and bisect each other at right angles, then it is a square.

Answer:
In quadrilateral ABCD, diagonals AC and BD are equal and bisect each other at right angles.
∴ AC = BD,
MA = MC = MB = MD = \(\frac{1}{2}\)AC = \(\frac{1}{2}\)BD and
∠AMB = ∠CMB= ∠DMC = ∠DMA= 90°.
In ∆ AMB and ∆ CMB,
AM = CM
∠ AMB = ∠ CMB (Right angles)
BM = BM (Common)
∴ ∆ AMB ≅ ∆ CMB (SAS rule)
∴ AB = CB (CPCT)
Similarly, we can prove that BC = DC and
DA = BA.
Thus, in quadrilateral ABCD,
AB = BC = CD = DA …………… (1)
Now, in ∆ DAB and ∆ CBA,
DA = C B
BD = AC (Given)
AB = BA (Common)
∴ ∆ DAB ≅ ∆ CBA (SSS rule)
∴ ∠DAB = ∠CBA (CPCT)
Thus, in quadrilateral ABCD, ∠A = ∠B.
Similarly, we can prove that ∠B = ∠C and ∠C = ∠D.
Thus, in quadrilateral ABCD,
∠A = ∠B = ∠C = ∠D.
Moreover. In quadrilateral ABCD,
∠A + ∠B + ∠C + ∠D = 360°
∴ ∠A = ∠B = ∠C = ∠D = \(\frac{360^{\circ}}{4}\) = 90° ……………… (2)
Thus, (1) and (2) taken together proves that in quadrilateral ABCD, all the sides are equal and all the angles are equal.
Therefore, quadrilateral ABCD is a square.
Thus, if the diagonals of a quadrilateral are equal and bisect each other at right angles, then it is a square.

Question 6.
Diagonal AC of a parallelogram ABCD bisects ∠A (see the given figure). Show that (i) it bisects ∠C also, (ii) ABCD is a rhombus.

Answer:
Diagonal AC of parallelogram ABCD bisects ∠A.
∴ ∠DAC = ∠BAC …………… (1)
Now, ∠BAC and ∠DCA are alternate angles formed by transversal AC of AB || CD.
∴ ∠BAC = ∠DCA …………… (2)
Similarly, ∠DAC and ∠BCA are alternate angles formed by transversal AC of AD || BC.
∴ ∠DAC = ∠BCA ……………… (3)
From (1), (2) and (3),
∠DCA = ∠BCA.
But, ∠DCA + ∠BCA = ∠BCD (Adjacent angles)
∴ AC bisects ∠C also.
In parallelogram ABCD,
∠A = ∠C (Theorem 8.4)
∴ \(\frac{1}{2}\)∠A = \(\frac{1}{2}\)∠C
∴ ∠ DAC = ∠ DCA
∴ In ∆ DAC, DA = DC (Sides opposite to equal angles)
Moreover, in parallelogram ABCD,
AB = CD and BC = DA (Theorem 8.2)
∴ AB = BC = CD = DA
Thus. In parallelogram ABCD, all the sides are equal.
Hence, ABCD is a rhombus.

Question 7.
ABCD is a rhombus. Show that diagonal AC bisects ∠A as well as ∠C and diagonal BD bisects ∠B as well as ∠D.
Answer:

ABCD is a rhombus
∴ AB || DC, BC || AD and AB = BC = CD = DA.
AB || DC and AC is their transversal.
∴ ∠CAB = ∠ACD (Alternate angles)
In, ∆ DAC, CD = DA
∴ ∠ACD = ∠CAD
Then, ∠CAB = ∠CAD
But, ∠CAB + ∠CAD = ∠ DAB (Adjacent angles)
∴ ∠ CAB = ∠CAD = \(\frac{1}{2}\) ∠DAB
This shows that AC bisects ∠A.
Again, BC || AD and AC is their transversal.
∴ ∠ BCA = ∠ DAC (Alternate angles)
In, ∆ DAC, DA = DC
∴ ∠ DAC = ∠ DCA
Then, ∠BCA = ∠DCA
But, ∠ BCA + ∠ DCA = ∠ DCB (Adjacent angles)
∴ ∠ BCA = ∠ DCA = \(\frac{1}{2}\)∠ DCB
This shows that AC bisects ∠C.
Thus, AC bisects ∠A as well as ∠C.
Similarly, taking BD as transversal of AB || DC, and BC || AD, it can be proved that BD bisects ∠B as well as ∠D.

Question 8.
ABCD is a rectangle in which diagonal AC bisects ∠A as well as ∠C. Show that: (i) ABCD is a square. (ii) Diagonal BD bisects ∠B as well as ∠D.
Answer:

In rectangle ABCD, AB = CD, BC = AD, AB || CD and BC || AD.
AC bisects ∠A as well as ∠C.
∴ ∠DAC = ∠BAC = \(\frac{1}{2}\)∠A and
∠ DCA = ∠ BCA = \(\frac{1}{2}\)∠C
Now, AB || CD and AC is their transversal.
∴ ∠ BAC = ∠ DCA (Alternate angles)
∴ ∠ DAC = ∠ DCA
Thus, in ∆ DAC, ∠DAC = ∠DCA
∴ AD = CD (Sides opposite to equal angles)
From this, we get AB = BC = CD = DA.
Also, in rectangle ABCD,
∠A = ∠B = ∠C = ∠D = 90°
Hence, ABCD is a square. …..Result (i)
In ∆ BCD, BC = CD
∴ ∠ CBD = ∠ CDB
Moreover, AB || CD and BD is their transversal.
∴ ∠ CDB = ∠ ABD (Alternate angles)
∴ ∠ CBD = ∠ ABD
Now, ∠ CBD + ∠ ABD = ∠ ABC
∴ ∠ CBD = ∠ ABD = \(\frac{1}{2}\) ∠ ABC
Thus, BD bisects ∠B.
Similarly, diagonal BD bisects ∠ D.
Hence, diagonal BD bisects ∠B as well as ∠D …….. Result (ii)

Question 9.
In parallelogram ABCD, two points P and Q are taken on diagonal BD such that DP = BQ (see the given figure). Show that:
(i) ∆ APD ≅ ∆ CQB
(ii) AP = CQ
(iii) ∆ AQB ≅ ∆ CPD
(iv) AQ = CP
(v) APCQ is a parallelogram.
Answer:

ABCD is a parallelogram.
∴ AD || BC and BD is their transversal.
∴ ∠ADB = ∠CBD (Alternate angles)
∴ ∠ADP = ∠CBQ …………… (1)
Similarly, CD || BA and BD is their transversal.
∴ ∠ ABD = ∠ CDB (Alternate angles)
∴ ∠ABQ = ∠CDP ……………… (2)
In ∆ APD and ∆ CQB,
AD = CB (Opposite sides of a parallelogram)
∠ ADP = ∠ CBQ [by (1)]
DP = BQ (Given)
∴ ∆ APD ≅ ∆ CQB (SAS rule) ……. Result (i)
∴ AP = CQ (CPCT) …… Result (ii)
In ∆ AQB and ∆ CPD,
AB = CD (Opposite sides of a parallelogram)
∠ ABQ = ∠ CDP [by (2)]
BQ = DP (Given)
∴ ∆ AQB ≅ ∆ CPD (SAS rule) …….. Result (iii)
∴ AQ = CP (CPCT) ………….. Result (iv)
Now, in quadrilateral APCQ, AP = CQ and AQ = CP
Hence, by theorem 8.3, APCQ is a parallelogram. ………. Result (v)

Question 10.
ABCD is a parallelogram and AP and Cg are perpendiculars from vertices A and C on diagonal BD (see the given figure). Show that
(i) ∆ APB ≅ ∆ CQD
(ii) AP = CQ

Answer:
In parallelogram ABCD, AB || CD and BD is their transversal.
∴ ∠ ABD = ∠ CDB (Alternate angles)
∴ ∠ABP = ∠CDQ ……………. (1)
Now, in ∆ APB and ∆ CQD,
AB = CD (Opposite sides of a parallelogram)
∠ ABP = ∠ CDQ [by (1)]
∠ APB = ∠ CQD (Right angles)
∆ APB ≅ ∆ CQD (AAS rule) ………… Result (i)
∴ AP = CQ (CPCT) ……….. Result (ii)

Question 11.
In ∆ ABC and ∆ DBF, AB = DE, AB || DE, j BC = EF and BC || EF. Vertices A, B and C are joined to vertices D, E and F respectively (see the given figure). Show that:
(i) Quadrilateral ABED is a parallelogram
(ii) Quadrilateral BEFC is a parallelogram
(iii) AD || CF and AD = CF
(iv) Quadrilateral ACFD is a parallelogram
(v ) AC = DF
(vi) ∆ ABC ≅ ∆ DEF.

Answer:
In quadrilateral ∆ BED, AB = DE and AB || DE. Thus, in quadrilateral ABED, sides in one s pair of opposite sides are equal and parallel. Hence, by theorem 8.8, quadrilateral ABED is a parallelogram. …… Result (i)
Similarly, in quadrilateral BEFC, BC = EF and BC || EF.
Hence, by theorem 8.8, quadrilateral BEFC is a parallelogram. …………. Result (ii)
In parallelogram ABED, AD || BE and in parallelogram BEFC, BE || CE Thus, AD and CF both are parallel to BE.
∴ AD || CF ……….(1)
In parallelogram ABED, AD = BE and in parallelogram BEFC, BE = CF.
∴ AD = CF ……… (2)
Taking (1) and (2) together, we get
AD || CF and AD = CF ………. Result (iii)
In quadrilateral ACFD, AD || CF and AD = CF. Hence, by theorem 8.8, quadrilateral ACFD is a parallelogram. ………. Result (iv)
AC and DF are opposite sides of parallelogram ACFD.
∴ AC = DF ………….. Result (v)
Now, in ∆ ABC and ∆ DEF,
AB = DE (Given)
BC = EF (Given)
AC = DF [by result (v)l
∴ ∆ ABC ≅ ∆ DEF (SSS rule) …… Result (vi)

Question 12.
ABCD is a trapezium in which AB || CD and AD = BC (see the given figure). Show that:
(i) ∠A = ∠B
(ii) ∠C = ∠D
(iii) ∆ ABC ≅ ∆ BAD
(iv) diagonal AC = diagonal BD
[Hint: Extend AB and draw a line through C parallel to DA intersecting AB produced at E.)

Answer:
AB is extended to E, and AB || CD.
∴ AE || CD
In quadrilateral ADCE, AE || CD and by consturction CE || DA.
∴ Quadrilateral ADCE is a parallelogram.
∴ AD = CE
Moreover, AD = BC (Given)
∴ BC = CE
In ∆ BCE, BC = CE
∴ ∠CBE = ∠CEB
∴ ∠CBE = ∠CEA ………….. (1)
In parallelogram ADCE, AD || CE and AE is their transversal.
∴ ∠ DAE + ∠ CEA = 180° (Interior angles on the same side of transversal)
∴ ∠ DAE + ∠ CBE = 180° [by (1)]
∴ ∠ DAE = 180° – ∠ CBE …………… (2)
Moreover, ∠ ABC + ∠ CBE = 180° (Linear pair)
∴ ∠ ABC = 180° – ∠ CBE …………. (3)
From (2) and (3),
∠ DAE = ∠ ABC
∴ ∠A = ∠B ……… Result (i)
AB || CD and AD is their transversal.
∴ ∠A + ∠D = 180°
∴ ∠D = 180°- ∠A ………….. (4)
AB || CD and BC is their transversal.
∴ ∠B + ∠C = 180°
∴ ∠C = 180°- ∠B
∴ ∠C = 180° – ∠ A [by result (i)] ……… (5)
From (4) and (5),
∠C = ∠D …….. Result (ii)
Draw diagonals AC and BD.
In ∆ ABC and ∆ BAD,
BC = AD (Given)
∠ ABC = ∠ BAD [by result (i)]
AB = BA (Common)
∴ ∆ ABC ≅ ∆ BAD (SAS rule) ………. Result (iii)
∴ AC = BD (CPCT)
Thus, diagonal AC = diagonal BD … Result (iv)
Note: A trapezium in which non-parallel sides are equal is called an isosceles trapezium. As proved above, in an isosceles trapezium, the diagonals are equal and the angles on each parallel side are equal.