Prasanna

Punjab State Board PSEB 12th Class Physics Book Solutions Chapter 10 Wave Optics Textbook Exercise Questions and Answers.

PSEB Solutions for Class 12 Physics Chapter 10 Wave Optics

PSEB 12th Class Physics Guide Wave Optics Textbook Questions and Answers

Question 1.
Monochromatic light of wavelength 589 nm is incident from air on a water surface. What are the wavelength, frequency and speed of (a) reflected, and (b) refracted light? The Refractive index of water is 1.33.
Answer:
Wavelength of incident monochromatic light, λ = 589 nm = 589 x 10^-9 m
Speed of light in air, c = 3 x 10⁸ m/s
Refractive index of water, µ = 1.33

(a) The ray will reflect back in the same medium as that of the incident ray. Hence, the wavelength, speed and frequency of the reflected ray will be the same as that of the incident ray.
Frequency of light is given by the relation,
v = \(\frac{c}{\lambda}=\frac{3 \times 10^{8}}{589 \times 10^{-9}}\)
= 5.09 x 10¹⁴ Hz
Hence, the speed, frequency, and wavelength of the reflected light are 3 x 10⁸ m/s, 5.09 x 10¹⁴ Hz, and 589 nm respectively.

(b) Frequency of light does not depend on the property of the medium in which it is travelling. Hence, the frequency of the refracted ray in water will be equal to the frequency of the incident or reflected light in air.
Refracted frequency, v = 5.09 x 10¹⁴ Hz
Speed of light in water is related to the refractive index of water as
v_w = \(\frac{c}{\mu}\)
v_w = \(\frac{3 \times 10^{8}}{1.33} \) = 2.26 x 10⁸ m/s
Wavelength of light in water is given by the relation,
λ = \(\frac{v_{w}}{v}=\frac{2.26 \times 10^{8}}{5.09 \times 10^{14}}\)
= 444.007 x 10^-9 m
= 444.01 nm
Hence, the speed, frequency and wavelength of refracted light are 2.26 x 10⁸ m/s, 5.09 x 10¹⁴ Hz
and 444.01 nm respectively.

Question 2.
What is the shape of the wavefront in each of the following cases:
(a) Light diverging from a point source. ;
(b) Light emerging out of a convex lens when a point source is placed at its focus.
(c) The portion of the wavefront of light from a distant star intercepted hy the Earth.
Answer:
(a) The shape of the wavefront in case of a light diverging from a point source is spherical. The wavefront emanating from a point source is shown in the given figure

(b) The shape of the wavefront in case of a light emerging out of a convex lens when a point source is placed at its focus is a plane or a parallel grid. This is shown in the given figure

(c) The portion of the wavefront of light from a distant star intercepted by the Earth is a plane.

Question 3.
(a) The refractive index of glass is 1.5. What is the speed of light in glass? (Speed of light in vacuum is 3.0x 10⁸ ms^-1). Is the speed of light in glass independent of the colour of light? If not, which of the two colours red and violet travels slower in a glass prism?
Answer:
(a) Refractive index of glass, µ = 1.5
Speed of light, c = 3 x 10⁸ m/s
Speed of light in glass is given by the relation,
v = \(\frac{c}{\mu}=\frac{3 \times 10^{8}}{1.5} \) = 2 x 10⁸ m/s
Hence, the speed of light in glass is 2 x 10⁸ m/s.

(b) The speed of light in glass is not independent of the colour of light.
The refractive index of a violet component of white light is greater than the refractive index of a red component. Hence, the speed of violet light is less than the speed of red light in glass. Hence, violet light travels slower than red light in a glass prism.

Question 4.
In a Young’s double-slit experiment, the slits are separated by 0.28 mm and the screen is placed 1.4 m away. The distance between the central bright fringe and the fourth bright fringe is measured to be 1.2 cm. Determine the wavelength of light used in the experiment.
Answer:
Distance between the slits, d = 0.28 mm = 0.28 x 10^-3 m
Distance between the slits and the screen, D = 1.4m
Distance between the central fringe and the fourth (n = 4) fringe, u = 1.2 cm = 1.2 x 10^-2 m
In case of a constructive interference, we have the ‘relation for the distance between the two fringes as
u = \(n \lambda \frac{D}{d}\)

where, n = order of fringes = 4 = 4λ= wavelength of light used
∴ λ = \(\frac{u d}{n D}\)
= \(\frac{1.2 \times 10^{-2} \times 0.28 \times 10^{-3}}{4 \times 1.4}\)
= 6 x 10^-7 = 600 nm
Hence, the wavelength of the light is 600 nm.

Question 5.
In Young’s double-slit experiment using monochromatic light of wavelength λ, the intensity of light at a point on the screen where path difference is λ is K units. What is the intensity of light at a point where path difference is λ / 3?
Answer:
Here, I =K when path difference = λ
I’ = ? when path difference = \(\frac{\lambda}{3}\)
We know that the intensity I is given by
I = 2I₀(1 + cosΦ) ………………………….. (1)
When Φ = phase difference

When path difference is λ, let Φ be the phase difference.
∴ From relation,
Φ’ = \(\frac{2 \pi}{\lambda}\) x, we get
Φ’ = \(\frac{2 \pi}{\lambda} \cdot \lambda\) = 2π
∴From eqn.(1),
K = 2I₀ (1+ cos 2π) (∵ cos 2π =1)
= 2I₀(1+1)
or K = 4I₀
or I₀ = \(\frac{K}{4}\) ……………………………… (2)
Let Φ, be the phase difference for a path difference \(\frac{\lambda}{3}\)
∴ Φ₁ = \(\frac{2 \pi}{\lambda} \times \frac{\lambda}{3}\)
= \(\frac{2 \pi}{3}\)
∴ I’ = 2I₀(1+cosΦ₁)

Question 6.
A beam of light consisting of two wavelengths, 650 mn and 520 nm, is used to obtain interference fringes in a Young’s double-slit experiment.
(a) Find the distance of the third bright fringe on the screen from the central maximum for wavelength 650 nm.
(b) What is the least distance from the central maximum where the bright fringes due to both the wavelengths coincide?
Answer:
First wavelength of the light beam, λ₁ = 650 nm
Second wavelength of the light beam, λ₂ = 520 nm
Distance of the slits from the screen = D
Distance between the two slits = d
(a) Distance of the n^th bright fringe on the screen from the central maximum is given by the relation,
x = nλ₁\(\left(\frac{D}{d}\right)\)
For third bright fringe, n = 3
∴ x = 3x 650\(\left(\frac{D}{d}\right)\) = 1950\(\left(\frac{D}{d}\right)\) nm

(b) Let the n^th bright fringe due to wavelength λ₂ and (n – 1)^th bright fringe due to wavelength λ₁ coincide on the screen. We can equate the conditions for bright fringes as nλ₂ = (n-1)λ
520 n = 650 n -650
650 = 130 n
∴ n = 5
Hence, the least distance from the central maximum can be obtained by the relation
x = nλ₂\(\left(\frac{D}{d}\right)\) = 5 x 520\(\left(\frac{D}{d}\right)\) = 2600\(\left(\frac{D}{d}\right)\) nm
Note : The value of d and D are not given in the question.

Question 7.
In a double-slit experiment, the angular width of a fringe is found to be 0.2° on a screen placed 1 m away. The wavelength of light used is 600 nm. What will be the angular width of the fringe if the entire experimental apparatus is immersed in water? Take refractive index of water to be 4/ 3.
Answer:
Distance of the screen from the slits, D = 1 m
The wavelength of light used, λ₁ = 600 nm
Angular width of the fringe in air, θ₁=0.2°
Angular width of the fringe in water = θ₂
Refractive index of water, µ = \(\frac{4}{3}\)
Refractive index is related to angular width as

Therefore, the angular width of the fringe in water will reduce to 0.15°.

Question 8.
What is the Brewster angle for air to glass transition? (Refractive index of glass = 1.5)
Answer:
Refractive index of glass, µ = 1.5
Brewster angle = θ
Brewster angle is related to refractive index as
tanθ = µ
θ= tan^-1 (1.5)=56.31°
Therefore, the Brewster angle for air to glass transition is 56.3 1°.

Question 9.
Light of wavelength 5000 A falls on a plane reflecting surface. What are the wavelength and frequency of the reflected light? For what angle of incidence is the reflected ray normal to the incident ray?
Answer:
Wavelength of incident light, λ = 5000 Å = 5000 x 10^-10 m
Speed of light, c =3 x 10⁸ m
Frequency of incident light is given by the relation,
v = \(\frac{c}{\lambda}=\frac{3 \times 10^{8}}{5000 \times 10^{-10}}\) = 6 x 10¹⁰ Hz

The wavelength and frequency of incident light is the same as that of reflected ray. Hence, the wavelength of reflected light is 5000 Å and its frequency is 6 x 10¹⁴ Hz. When reflected ray is normal to incident ray, the sum of the angle of incidence, ∠i and angle of reflection, ∠r is 90°.

According to the law of reflection, the angle of incidence is always equal to the angle of reflection. Hence, we can write the sum as
∠i + ∠r =90
∠i + ∠i=90
∠i = \( \frac{90}{2}\) = 45°
Therefore, the angle of incidence for the given condition is 45°.

Question 10.
Estimate the distance for which ray optics is a good approximation for an aperture of 4 mm and wavelength 400 nm.
Answer:
Fresnel’s distance (Z_F) is the distance for which the ray optics is a good approximation. It is given by the relation,
Z_F = \(\frac{a^{2}}{\lambda}\)
where,
aperture width, a = 4 mm = 4 x 10^-3m
wavelength of light, λ = 400 nm = 400 x 10^-9 m
Z_F = \(\frac{\left(4 \times 10^{-3}\right)^{2}}{400 \times 10^{-9}}\) = 40 m
Therefore, the distance for which the ray optics is a good approximation is 40 m.

Additional Exercises

Question 11.
The 6563 Å H_α line emitted by hydrogen in a star is found to be red-shifted by 15 Å. Estimate the speed with which the star is receding from the Earth.
Answer:
Wavelength of H_α line emitted by hydrogen, λ = 6563 Å
= 6563 x 10^-10 m.
Star’s red-shift, (λ’ – λ) = 15 Å = 15 x 10^-10 m
Speed of light, c = 3 x 10⁸ m/s
Let the velocity of the star receding away from the Earth be v.
The redshift is related with velocity as

Therefore, the speed with which the star is receding away from the Earth is 6.87 x10⁵ m/s.

Question 12.
Explain how corpuscular theory predicts the speed of light in a medium, say, water, to be greater than the speed of light in vacuum. Is the prediction confirmed by experimental determination of the speed of light in water? If not, which alternative picture of light is consistent with experiment?
Answer:
According to Newton’s corpuscular theory of light, when light corpuscles strike the interface of two media from a rarer (air) to a denser (water) medium, the particles experience forces of attraction normal to the surface. Hence, the normal component of velocity increases while the component along the surface remains unchanged.
Hence, we can write the expression
c sin i = v sin r …………………………… (1)
where i = Angle of incidence
r = Angle of reflection
c = Velocity of light in air
v = Velocity of light in water

We have the relation for a relative refractive index of water with respect to air as
μ = \(\frac{v}{c}\)
Hence, equation (1) reduces to
\(\frac{v}{c}=\frac{\sin i}{\sin r}\) = μ
But, μ > 1
Hence, it can.be inferred from equation (2) that v > c. This is not possible since this prediction is opposite to the experimental results of c > v. The wave picture of light is consistent with the experimental results.

Question 13.
You have learnt in the text how Huygen’s principle leads to the laws of reflection and refraction. Use the same principle to deduce directly that a point object placed in front of a plane mirror produces a virtual image whose distance from the mirror is equal to the object’s distance from the mirror.
Answer:
Let an object at 0 be placed in front of a plane mirror MO’ at a distance r (as shown in the given figure).

A circle is drawn from the centre (0) such that it just touches the plane mirror at point 0′. According to Huygen’s principle, XY is the wavefront of incident light. If the mirror is absent, then a similar wavefront X’ Y’ (as XT) would form behind 0′ at distance r (as shown in the given figure).

X’ Y’ can be considered as a virtual reflected ray for the plane mirror. Hence, a point object placed in front of the plane mirror produces a virtual image whose distance from the mirror is equal to the object distance (r).

Question 14.
Let us list some of the factors, which could possibly influence the speed of wave propagation :
(i) nature of the source.
(ii) direction of propagation.
(iii) motion of the source and/or observer.
(iv) wavelength.
(v) intensity of the wave.
On which of these factors, if any, does
(a) the speed of light in vacuum,
(b) the speed of light in a medium (say, glass Or water), depend?
Answer:
(a) The speed of light in a vacuum i. e., 3 x 10⁸ m/s (approximately) is a universal constant. It is not affected by the motion of the source, the observer, or both. Hence, the given factor does not affect, the speed of light in a vacuum.
(b) Out of the listed factors, the speed of light in a medium depends on the wavelength of light in that medium.

Question 15.
For sound waves, the Doppler formula for frequency shift differs slightly between the two situations : (i) source at rest; observer moving, and (ii) source moving; observer at rest. The exact Doppler formulas for the case of light waves in a vacuum are, however, strictly identical for these situations. Explain why this should be so. Would you expect the formulas to be strictly identical for the two situations in the case of light travelling in a medium?
Answer:
No, sound waves can propagate only through a medium. The two given situations are not scientifically identical because the motion of an observer relative to a medium is different in the two situations. Hence, the Doppler formulas for the two situations cannot be the same.

In the case of light waves, sound can travel in a vacuum. In a vacuum, the above two cases are identical because the speed of light is independent of the motion of the observer and the motion of the source. When light travels in a medium, the above two cases are not identical because the speed of light depends on the wavelength of the medium.

Question 16.
In a double-slit experiment using light of wavelength 600 nm, the angular width of a fringe formed on a distant screen is 0.1°. What is the spacing between the two slits?
Answer:
Wavelength of light used, λ = 600 nm = 600 x 10^-9 m
Angular width of fringe, θ = 0.1° = 0.1 x \(\frac{\pi}{180}=\frac{3.14}{1800}\)rad
Angular width of a fringe is related to slit spacing (d) as
θ = \(\frac{\lambda}{d}\)

Therefore, the spacing between the two slits is 3.44 x 10^-4 m.

Question 17.
Answer the following questions:
(a) In a single slit diffraction experiment, the width of the slit is made double the original width. How does this affect the size and intensity of the central diffraction band?
(b) In what way is diffraction from each slit related to the interference pattern in a double-slit experiment?
(c) When a tiny circular obstacle is placed in the path of light from a distant source, a bright spot is seen at the centre of the shadow of the obstacle. Explain why?
(d) Two students are separated by a 7 m partition wall in a room 10 m high. If both light and sound waves can bend around obstacles, how is it that the? students are unable to see each other even though they can converse easily.
(e) Ray optics is based on the assumption that light travels in a straight line. Diffraction effects (observed when light propagates through small apertures/slits or around small obstacles) disprove this assumption. Yet the ray optics assumption is so commonly used in an understanding of location and several other properties of images in optic instruments. What is the justification?
Answer:
(a) In a single slit diffraction experiment, if the width of the slit is made double the original width, then the size of the central diffraction band reduces to half and the intensity of the central diffraction band increase up to four times.

(b) The interference pattern in a double-slit experiment is modulated by diffraction from each slit. The pattern is the result of the interference of the diffracted wave from each slit.

(c) When a tiny circular obstacle is placed in the path of light from a distant source, a bright spot is seen at the centre of the shadow of the obstacle. This is because light waves are diffracted from the edge of the circular obstacle, which interferes constructively at the centre of the shadow. This constructive interference produces a bright spot.

(d) Bending of waves by obstacles by a large angle is possible when the size of the obstacle is comparable to the wavelength of the waves. On the one hand, the wavelength of the light waves is too small in comparison to the size of the obstacle. Thus, the diffraction angle will be very small. Hence, the students are unable to see each other. On the other hand, the size of the wall is comparable to the wavelength of the sound waves. Thus, the bending of the waves takes place at a large angle. Hence, the students are able to hear each other.

(e) The justification is that in ordinary optical instruments, the size of the aperture involved is much larger than the wavelength of the light used.

Question 18.
Two towers on top of two hills are 40 km apart. The line joining them passes 50 m above a hill halfway between the towers. What is the longest wavelength of radio waves, which can be sent between the towers without appreciable diffraction effects?
Answer:
Distance between the towers, d = 40 km
Height of the line joining the hills, d = 50 m
Thus, the radial spread of the radio waves should not exceed 50 km.
Since the hill is located halfway between the towers, Fresnel’s distance can be obtained as
Z_P = 20 km = 20 x 10³m
Aperture can be taken as
a = d= 50 m

Fresnel’s distance is given by the relation,
Z_p = \(\frac{a^{2}}{\lambda}\)
where, λ = wavelength of radio waves
∴ λ = \(\frac{a^{2}}{Z_{P}}\)
= \(\frac{(50)^{2}}{20 \times 10^{3}}\) = 1250 x 10^-4 = 0.1250 m
= 12.5 cm
Therefore, the wavelength of the radio waves is 12.5 cm.

Question 19.
A parallel beam of light of wavelength 500 nm falls on a narrow slit and the resulting diffraction pattern is observed on a screen 1 m away. It is observed that the first minimum is at a distance of 2.5 mm from the centre of the screen. Find the width of the slit.
Answer:
Wavelength of light beam, λ = 500 nm = 500 x 10^-9 m
Distance of the screen from the slit, D=1m
For first minima, n = 1
Distance between the slits = d
Distance of the first minimum from the centre of the screen can be obtained as
x = 2.5mm = 2.5 x 10^-3 m
It is related to the order of minima as

Therefore, the width of the slits is 0.2 mm.

Question 20.
Answer the following questions :
(a) When a low flying aircraft passes overhead, we sometimes notice a slight shaking of the picture on our TV screen. Suggest a possible explanation.
(b) As you have learnt in the text, the principle of linear superposition of wave displacement is basic to understanding intensity distributions in diffraction and interference patterns. What is the justification of this principle?
Answer:
(a) Weak radar signals sent by a low flying aircraft can interfere with the TV signals received by the antenna. As a result, the TV signals may get distorted. Hence, when a low flying aircraft passes overhead, we sometimes notice a slight shaking of the picture on our TV screen.

(b) The principle of linear superposition of wave displacement is essential to our understanding of intensity distributions and interference patterns. This is because superposition follows from the linear character of a differential equation that governs wave motion. If y₁ and y₂ are the solutions of the second-order wave equation, then any linear combination of y± and y2 will also be the solution of the wave equation.

Question 21.
In deriving the single slit diffraction pattern, it was stated that the intensity is zero at angles of n λ/a. Justify this by suitably dividing the slit to bring out the cancellation.
Answer:
Consider that a single slit of width d is divided into n smaller slits.
∴ Width of each slit, d’ = \(\frac{d}{n}\)
Angle of diffraction is given by the relation,
θ = \(\frac{\frac{d}{d^{\prime}} \lambda}{d}=\frac{\lambda}{d^{\prime}} \)
Now, each of these infinitesimally small slit sends zero intensity in direction θ. Hence, the combination of these slits will give zero intensity.

Punjab State Board PSEB 12th Class Physics Book Solutions Chapter 5 Magnetism and Matter Textbook Exercise Questions and Answers.

PSEB Solutions for Class 12 Physics Chapter 5 Magnetism and Matter

PSEB 12th Class Physics Guide Magnetism and Matter Textbook Questions and Answers

Question 1.
Answer the following questions regarding earth’s magnetism:
(a) A vector needs three quantities for its specification. Name the three independent quantities conventionally used to specify the earth’s magnetic field.

(b) The angle of dip at a location in southern India is about 18°
Would you expect a greater or smaller dip angle in Britain?

(c) If you made a map of magnetic field lines at Melbourne in Australia, would the lines seem to go into the ground or come out of the ground?

(d) In which direction would a compass free to move in the vertical plane point to, if located right on the geomagnetic north or south pole?

(e) The earth’s field, it is claimed, roughly approximates the field due to a dipole of magnetic moment 8 × 10²² JT^-1 located at its centre. Check the order of magnitude of this number in some way.

(f) Geologists claim that besides the main magnetic N-S poles, there are several local poles on the earth’s surface oriented in different directions. How is such a thing possible at all?
Answer:
(a) The three independent quantities conventionally used for specifying earth’s magnetic field are magnetic declination, angle of dip and horizontal component of earth’s magnetic field.

(b) The angle of dip at a point depends on how far the point is located with respect to the North Pole or the South Pole. The angle of dip would be greater in Britain (it is about 70°) than in southern India because the location of Britain on the globe is closer to the magnetic North Pole.

(c) It is hypothetically considered that a huge bar magnet is dipped inside earth with its North Pole near the geographic South Pole and its South Pole near the geographic North Pole.

Magnetic field lines emanate from a magnetic North Pole and terminate at a magnetic South Pole. Hence, in a map depicting earth’s magnetic field lines, the field lines at Melbourne, Australia would seem to come out of the ground.

(d) If a compass is located on the geomagnetic North Pole or South Pole, then the compass will be free to move in the horizontal plane while earth’s field is exactly vertical to the magnetic poles. In such a case, the compass can point in any direction.

(e) Magnetic moment, M = 8 × 10²² JT^-1
Radius of earth, r = 6.4 × 10⁶ m
Magnetic field strength, B = \(\frac{\mu_{0} M}{4 \pi r^{3}}\)
∴ B = \(\frac{4 \pi \times 10^{-7} \times 8 \times 10^{22}}{4 \pi \times\left(6.4 \times 10^{6}\right)^{3}}\) = 0.3G
This quantity is of the order of magnitude of the observed field on earth.

(f) Yes, there are several local poles on earth’s surface oriented in different directions. A magnetised mineral deposit is an example of a local N-S pole.

Question 2.
Answer the following questions :
(a) The earth’s magnetic field varies from point to point in space. Does it also change with time? If so, on what time scale does it change appreciably?

(b) The earth’s core is known to contain iron. Yet geologists do not regard this as a source of the earth’s magnetism. Why?

(c) The charged currents in the outer conducting regions of the earth’s core are thought to be responsible for earth’s magnetism. What might be the ’battery’ (i.e., the source of energy) to sustain these currents?

(d) The earth may have even reversed the direction of its field several times during its history of 4 to 5 billion years. How can geologists know about the earth’s field in such distant past?

(e) The earth’s field departs from its dipole shape substantially at large distances (greater than about 30,000 km). What agencies may be responsible for this distortion?

(f) Interstellar space has an extremely weak magnetic field of the order of 10^-12 T. Can such a weak field he of any significant consequence? Explain.
[Note: Exercise 5.2 is meant mainly to arouse your curiosity. Answers to some questions above are tentative or unknown. Brief answers wherever possible are given at the end. For details, you should consult a good text on geomagnetism.]
Answer:
(a) Earth’s magnetic field changes with time. It takes a few hundred years to change by an appreciable amount. The variation in earth’s magnetic field with the time cannot be neglected.

(b) Earth’s core contains molten iron. This form of iron is not ferromagnetic. Hence, this is not considered as a source of earth’s magnetism.

(c) The radioactivity in earth’s interior is the source of energy that sustains the currents in the outer conducting regions of earth’s core. These charged currents are considered to be responsible for earth’s magnetism.

(d) Earth reversed the direction of its field several times during its history of 4 to 5 billion years. These magnetic fields got weakly recorded in rocks during their solidification. One can get clues about the geomagnetic history from the analysis of this rock magnetism.

(e) Earth’s field departs from its dipole shape substantially at large distances (greater than about 30,000 km) because of the presence of the ionosphere. In this region, earth’s field gets modified because of the field of single ions. While in motion, these ions produce the magnetic field associated with them.

(f) An extremely weak magnetic field can bend charged particles moving in a circle. This may not be noticeable for a large radius path. With reference to the gigantic interstellar space, the deflection can affect the passage of charged particles.

Question 3.
A short bar magnet placed with its axis at 30° with a uniform external magnetic field of 0.25 T experiences a torque of magnitude equal to 4.5 × 10^-2 J. What is the magnitude of magnetic moment of the magnet?
Answer:
Magnetic field strength, B = 0.25 T
Torque on the bar magnet, τ = 4.5 × 10^-2J
Angle between the bar magnet and the external magnetic field, θ = 30°
Torque is related to magnetic moment (M) as
τ = MB sinθ
∴ M = \(\frac{\tau}{B \sin \theta}\)
= \(\frac{4.5 \times 10^{-2}}{0.25 \times \sin 30^{\circ}}\)
\(\frac{4.5 \times 10^{-2} \times 2}{0.25 \times 1}\)
(∵ sin30° = \(\frac{1}{2}\))
= 0.36 JT^-1
Hence, the magnetic moment of the magnet is 0.36 JT^-1.

Question 4.
A short bar magnet of magnetic moment m = 0.32 JT^-1 is placed in a uniform magnetic field of 0.15 T. If the bar is free to rotate in the plane of the field, which orientation would correspond to its (a) stable, and (b) unstable equilibrium? What is the potential energy of the magnet in each case?
Answer:
Given, M = 0.32 JT^-1, B = 0.15T,U = ?
(a) Stable Equilibrium: The magnetic moment should be parallel to the magnetic field. In this position, the potential energy is
U = -MB cos θ =0.32 × 0.15 × 1
= -0.048 J or-4.8 × 10^-2 J\

(b) Unstable Equilibrium: The magnetic moment should be antiparallel to the magnetic field. In this position, the potential energy is
U = -MBcosθ = 0.32 × 0.15 × (-1)
= +0.048 J or + 4.8 × 10^-2 J

Question 5.
A closely wound solenoid of 800 turns and area of cross-section 2.5 × 10^-4 m² carries a current of 3.0 A. Explain the sense in which the solenoid acts like a bar magnet. What is its associated magnetic moment?
Answer:
Solenoid acts as a bar magnet, its magnetic moment is along the axis of the solenoid, the direction determined by the sense of flow of current. The magnetic moment of a current carrying loop having N turns
= NIA = 800 × 3 × 2.5 × 10^-4
= 6 × 10^-1
= 0.60 A-m²
= 0.60 JT^-1

Question 6.
If the solenoid in Exercise 5.5 is free to turn about the vertical direction and a uniform horizontal magnetic field of 0.25 T is applied, what is the magnitude of torque on the solenoid when its axis makes an angle of 30° with the direction of applied field?
Answer:
Magnetic field strength, B = 0.25 T
Magnetic moment, M = 0.6 JT^-1
The angle θ, between the axis of the solenoid and the direction of the applied field is 30°.
Therefore, the torque acting on the solenoid is given as
τ = MB sinθ
= 0.6 × 0.25 × sin30°
= 0.6 × 0.25 × \(\frac{1}{2}\)
= 0.075 N-m

Question 7.
A bar magnet of magnetic moment 1.5 J T^-1 lies aligned with the direction of a uniform magnetic field of 0.22 T.
(a) What is the amount of work required by an external torque to turn the magnet so as to align its magnetic moment:
(i) normal to the field direction,
(ii) opposite to the field direction?

(b) What is the torque on the magnet in cases (i) and (ii)?
Answer:
Given, M = 1.5 JT^-1,B = 0.22 T,θ₁ =0°

(a) To align the dipole normal to the field direction θ₂ = 90°. Therefore,
W = MB(cosθ₁ – cosθ₂)
W = 1.5 × 0.22(cos0° – cos90°) = 0.33 J
Also, τ = MB sinθ₂
or τ = 1.5 × 0.22sin90° = 0.33 Nm

(b) To align the dipole opposite to the field direction θ₂ = 180°. Therefore,
W =MB(cosθ₁ – cosθ₂)
W = 1.5 × 0.22(cos0° – cos180°) = 0.66 J
Also, τ = MB sinθ₂
or τ = 1.5 × 0.22sinl80° = 0 Nm

Question 8.
A closely wound solenoid of2000 turns and area of cross-section 1.6 × 10^-4 m², carrying a current of 4.0 A, is suspended through its centre allowing it to turn in a horizontal plane.
(a) What is the magnetic moment associated with the solenoid?
(b) What is the force and torque on the solenoid if a uniform horizontal magnetic field of 7.5 × 10^-2 T is set up at an angle of 30° with the axis of the solenoid?
Answer:
Number of turns on the solenoid, N = 2000
Area of cross-section of the solenoid, A = 1.6 × 10^-4 m^{2</sup
Current in the solenoid, I = 4 A}

(a) Let M = magnetic moment of the solenoid.
∴ Using the relation M = NIA, we get
M = 2000 × 4.0 × 1.6 × 10^{-4</sup
= 1.28 JT-1</sup}

The direction of \(\vec{M}\) is along the axis of the solenoid in the direction related to the sense of current according to right-handed screw rule.

(b) Here θ = 30°
\(\vec{B}\) = 7.5 × 10^-2 T
Let F = force on the solenoid = ?
τ = torque on the solenoid = ?
The solenoid behaves as a bar magnet placed in a uniform magnetic field, so the force is
F = m \(\vec{B}\) + (-m \(\vec{B}\)) = 0
where m = pole strength of the magnet.

Using the relation, τ = MB sinθ, we get
τ = 1.28 × 7.5 × 10^-2 × sin30°
= 1.28 × 7.5 × 10^-2 × \(\frac{1}{2}\) = 0.048 J
The direction of the torque is such that it tends to align the axis of the solenoid (i. e., magnetic moment vector \(\vec{M}\)) along \(\vec{B}\).

Question 9.
A circular coil of 16 turns and radius 10 cm carrying a current of 0.75 A rests with its plane normal to an external field of magnitude 5.0 × 10^-2 T. The coil is free to turn about an axis in its plane perpendicular to the field direction. When the coil is turned slightly and released, it oscillates about its stable equilibrium with a frequency of 2.0 s^-1. What is the moment of inertia of the coil about its axis of rotation?
Answer:
Number of turns in the circular coil, N = 16
Radius of the coil, r = 10cm = 0.1m
Cross-section of the coil, A = πr² = π × (0.1)² m²
Current in the coil, I = 0.75 A
Magnetic field strength, B = 5.0 × 10^-2 T
Frequency of oscillations of the coil, v = 2.0 s^-1
∴ Magnetic moment, M = NIA = 16 × 0.75 × π × (0.1)² = 0.377 JT^-1
Frequency is given by the relation
v = \(\frac{1}{2 \pi} \sqrt{\frac{M B}{I}}\)
where, I = Moment of inertia of the coil
I = \(\frac{M B}{4 \pi^{2} v^{2}}\) = \(\frac{0.377 \times 5 \times 10^{-2}}{4 \pi^{2} \times(2)^{2}}\)
= 1.19 × 10^-4 kg m²
Hence, the moment of inertia of the coil about its axis of rotation is 1.19 × 10^-4 kg m².

Question 10.
A magnetic needle free to rotate in a vertical plane parallel to the magnetic meridian has its north tip pointing down at 22° with the horizontal. The horizontal component of the earth’s magnetic field at the place is known to be 0.35 G. Determine the magnitude of the earth’s magnetic field at the place.
Answer:
Horizontal component of earth’s magnetic field, B_H = 0.35 G
Angle made by the needle with the horizontal plane
= Angle of dip = δ = 22°
Earth’s magnetic field strength = B
We can relate B and B_H as
B_H = B cosδ
∴ B = \(\frac{B_{H}}{\cos \delta}=\frac{0.35}{\cos 22^{\circ}}=\frac{0.35}{0.9272}\) = 0.377 G
Hence, the strength of earth’s magnetic field at the given location is 0.377 G.

Question 11.
At a certain location in Africa, a compass points 12° west of the geographic north. The north tip of the magnetic needle of a dip circle placed in the plane of magnetic meridian points 60° above the horizontal. The horizontal component of the earth’s field is measured to he 0.16 G. Specify the direction and magnitude of the earth’s field at the location.
Answer:
Angle of declination, θ = 12°
Angle of dip, δ = 60°
Horizontal component of earth’s magnetic field, B_H = 0.16 G
Earth’s magnetic field at the given location = B
We can relate B and B_H as
B_H = B cosδ
B= \(\frac{B_{H}}{\cos \delta}\) = \(=\frac{0.16}{\cos 60^{\circ}}\) = \(\frac{0.16}{\left(\frac{1}{2}\right)}\) = 0.16 × 2 = 0.32 G
Earth’s magnetic field lies in the vertical plane, 12° west of the geographic meridian, making an angle of 60° (Upward) with the horizontal direction. Its magnitude is 0.32 G.

Question 12.
A short bar magnet has a magnetic moment of 0.48 JT^-1. Give the direction and magnitude of the magnetic field produced by the magnet at a distance of 10 cm from the centre of the magnet on (a) the axis, (b) the equatorial lines (normal bisector) of the magnet.
Answer:
Magnetic moment of the bar magnet, M = 0.48 JT^-1
Distance, d = 10cm = 0.1m

(a) The magnetic field at distance d, from the centre of the magnet on the axis is given by the relation,
B = \(\frac{\mu_{0}}{4 \pi} \frac{2 M}{d^{3}}\)
∴ B = \(\frac{4 \pi \times 10^{-7} \times 2 \times 0.48}{4 \pi \times(0.1)^{3}}\)
= 0.96 × 10^-4 T = 0.96 G
The magnetic field is along the S-N direction.

(b) The magnetic field at a distance of 10 cm (i.e., d = 0.1 m) on the equatorial line of the magnet is given as,
B = \(\frac{\mu_{0} \times M}{4 \pi \times d^{3}}\)
∴ B = \(\frac{4 \pi \times 10^{-7} \times 0.48}{4 \pi \times(0.1)^{3}}\) = 0.48G
The magnetic field is along the N-S direction.

Question 13.
A short bar magnet placed in a horizontal plane has its axis aligned along the magnetic north-south direction. Null points are found on the axis of the magnet at 14 cm from the centre of the magnet. The earth’s magnetic field at the place is 0.36 G and the angle of dip is zero. What is the total magnetic field on the normal bisector of the magnet at the same distance as the null-point (i. e., 14 cm) from the centre of the magnet? (At null points, field due to a magnet is equal and opposite to the horizontal component of earth’s magnetic field.)
Answer:
Distance of the null point from the centre of magnet
d = 14 cm = 0.14 m
The earth’s magnetic field where the angle of dip is zero, is the horizontal component of earth’s magnetic field. i.e., H = 0.36 G
Initially, the null points are on the axis of the magnet. We use the formula of magnetic field on axial line (consider that the magnet is short in length).
B₁ = \(\frac{\mu_{0}}{4 \pi} \cdot \frac{2 m}{d^{3}}\)
This magnetic field is equal to the horizontal component of earth’s magnetic field.
i.e., B₁ = \(\frac{\mu_{0}}{4 \pi} \cdot \frac{2 m}{d^{3}}\) = H ……….(1)
On the equitorial line of magnet at same distance (d) magnetic field due to the magnet
B₂ = \(\frac{\mu_{0}}{4 \pi} \cdot \frac{m}{d^{3}}=\frac{B_{1}}{2}=\frac{H}{2}\) …………….. (2)
The total magnetic field on equitorial line at this point (as given in question)
B = B₂ + H = \(\frac{H}{2}\) + H = \(\frac{3}{2}\)H = \(\frac{3}{2}\) × 0.36 = 0.54G
The direction of magnetic field is in the direction of earth’s field.

Question 14.
If the bar magnet in exercise 5.13 is turned around by 180°, where will the new null points be located?
Answer:
When the bar magnet is turned by 180°, then the null points are obtained on the equitorial line.
So, magnetic field on the equitorial line at distance d’ is
B’ = \(\frac{\mu_{0}}{4 \pi} \cdot \frac{m}{d^{3}}\)
This magnetic field is equal to the horizontal component of earth’s magnetic field
B’ = \(\frac{\mu_{0}}{4 \pi} \cdot \frac{m}{d^{3}}\) = H ………… (1)
From Q.No. 13 MISS
Magnetic field B₁ = \(\frac{\mu_{0}}{4 \pi} \cdot \frac{2 m}{d^{3}}\) = H ………….. (2)
From eqs. (1) and (2), we get

Thus, the null points are located on the equitorial line at a distance of 11.1 cm.

Question 15.
A short bar magnet of magnetic moment 5.25 × 10^-2 JT^-1 is placed with its axis perpendicular to the earth’s field direction. At what distance from the centre of the magnet, the resultant field is inclined at 45° with earth’s field on (a) its normal bisector and (b) its axis. Magnitude of the earth’s field at the place is given to be 0.42 G. Ignore the length of the magnet in comparison to the distances involved.
Answer:
Given, magnetic moment m = 5.25 × 10^-2 J/T
Let the resultant magnetic field is B_net. It makes an angle of 45° with B_e.
∴ B_e = 0.42G =0.42 × 10^-4 T
(a) At normal bisector
Let r is the distance between axial line and point P.
The magnetic field at point P, due to a short magnet
B = \(\frac{\mu_{0}}{4 \pi} \cdot \frac{m}{r^{3}}\) …………. (1)

The direction of B is along PB, i.e., along N pole to S pole.
According to the vector analysis,

r = 0.05 m
r = 5 cm

(b) When point lies on axial line
Let the resultant magnetic field B_net makes an angle 45° from B_e. The magnetic field on the axial line of the magnet at a distance of r from the centre of magnet
B’ = \(\frac{\mu_{0}}{4 \pi} \cdot \frac{2 m}{r^{3}}\) (S to N)

Direction of magnetic field is from S to N.
According to the vector analysis,

Question 16.
Answer the following questions :
(a) Why does a paramagnetic sample display greater magnetisation (for the same magnetising field) when cooled?

(b) Why is diamagnetism, in contrast, almost independent of temperature?

(c) If a toroid uses bismuth for its core, will the field in the core be (slightly) greater or (slightly) less than when the core is empty?

(d) Is the permeability of a ferromagnetic material independent of the magnetic field? If not, is it more for lower or higher fields?

(e) Magnetic field lines are always nearly normal to the surface of a ferromagnet at every point. (This fact is analogous to the static electric field lines being normal to the surface of a conductor at every point). Why?

(f) Would the maximum possible magnetisation of a paramagnetic sample be of the same order of magnitude as the magnetisation of a ferromagnet?
Answer:
(a) Owing to the random thermal motion of molecules, the alignments of dipoles get disrupted at high temperatures. On cooling, this disruption is reduced.’Hence, a paramagnetic sample displays greater magnetisation when cooled.

(b) The induced dipole moment in a diamagnetic substance is always opposite to the magnetising field. Hence, the internal motion of the atoms (which is related to the temperature) does not affect the diamagnetism of a material.

(c) Bismuth is a diamagnetic substance. Hence, a toroid with a bismuth core has a magnetic field slightly greater than a toroid whose core is empty.

(d) The permeability of ferromagnetic materials is not independent of the applied magnetic field. It is greater for a lower field and vice versa.

(e) The permeability of ferromagnetic materials is not less than one. It is always greater than one. Hence, magnetic field lines are always nearly normal to the surface of such materials at every point.

(f) The maximum possible magnetisation of a paramagnetic sample can be of the same order of magnitude as the magnetisation of a ferromagnet. This requires high magnetising fields for saturation.

Question 17.
Answer the following questions:
(a) Explain qualitatively on the basis of domain picture the irreversibility in the magnetisation curve of a ferromagnet.

(b) The hysteresis loop of a soft iron piece has a much smaller area than that of a carbon steel piece. If the material is to go through repeated cycles of magnetisation, which piece will dissipate greater heat energy?

(c) ‘A system displaying a hysteresis loop such as a ferromagnet, is a device for storing memory?’ Explain the meaning of this statement.

(d) What kind of ferromagnetic material is used for coating magnetic tapes in a cassette player,’ or for building ‘memory stores’ in a modern computer?

(e) A certain region of space is to be shielded from magnetic fields. Suggest a method.
Answer:
(a) The hysteresis curve (B-H curve) of a ferromagnetic material is shown in the following figure

It can be observed from the given curve that magnetisation persists even when the external field is removed. This reflects the irreversibility of a ferromagnet.

(b) The dissipated heat energy is directly proportional to the area of a hysteresis loop. A carbon steel piede has a greater hysteresis curve area. Hence, it dissipates greater heat energy.

(c) The value of magnetisation is memory or record of hysteresis loop cycles of magnetisation. These bits of information correspond to the cycle of magnetisation. Hysteresis loops can be used for storing information.

(d) Ceramic is used for coating magnetic tapes in cassette players and for building memory stores in modern computers.

(e) A certain region of space can be shielded from magnetic fields if it is – surrounded by soft iron rings. In such arrangements, the magnetic lines are drawn out of the region.

Question 18.
A long straight horizontal cable carries a current of 2.5 A in the direction 10° south of west to 10° north of east. The magnetic meridian of the place happens to be 10° west of the geographic meridian. The earth’s magnetic field at the location is 0.33 G, and the angle of dip is zero. Locate the line of neutral points (ignore the thickness of the cable). (At neutral points, magnetic field due to a current-carrying cable is equal and opposite to the horizontal component of earth’s magnetic field.)
Answer:
Given, current in the cable
I = 2.5 A

Magnetic meridian M_NM_S is 10° west of geographical meridian G_NG_S earth’s magnetic field R = 0.33 G
= 0.33 × 10^-4 T ……….. (1)
Angle of dip δ = _S0°
The neutral point is the point where the magnetic field due to the current carrying cable is equal to the horizontal component of earth’s magnetic field.
Horizontal component of earth’s magnetic field
H = Rcosθ = 0.33 × 10-4 cos0°
= 0.33 × 10^-4 T
Using the formula of magnetic field at distance r due to an infinite long current carrying conductor
B = \(\frac{\mu_{0}}{4 \pi} \cdot \frac{2 I}{r}\)
At neutral points,
H = B
0.33 × 10^-4 = \(\frac{\mu_{0}}{4 \pi} \cdot \frac{2 I}{r}\)
0.33 × 10^-4 = \(\frac{10^{-7} \times 2 \times 2.5}{r}\)
or r = \(\frac{5 \times 10^{-7}}{0.33 \times 10^{-4}}\)
or r = 1.5 × 10^-2 m = 1.5cm
Thus, the line of neutral points is at a distance of 1.5 cm from the cable.

Question 19.
A telephone cable at a place has four long straight horizontal wires carrying a current of 1.0 A in the same direction east to west. The earth’s magnetic field at the place is 0.39 G, and the angle of dip is 35°. The magnetic declination is nearly zero. What are the resultant magnetic fields at points 4.0 cm above and below the cable?
Answer:
Number of horizontal wires in the telephone cable, n = 4
Current in each wire, I = 1.0 A
Earth’s magnetic field at the location, H = 0.39 G = 0.39 × 10^-4 T
Angle of dip at the location, δ = 35°
Angle of declination, θ = 0°
For a point 4 cm below the cable
Distance, r = 4 cm = 0.04 m
The horizontal component of earth’s magnetic field can be written as
H_h, = Hcosδ – B
where,
B = Magnetic field at 4 cm due to current I in the four wires
= 4 × \(\frac{\mu_{0} I}{2 \pi r}\)
μ₀ = 4π × 10^-7 TmA^-1
∴ B = 4 × \(\frac{4 \pi \times 10^{-7} \times 1}{2 \pi \times 0.04}\)
= 0.2 × 10^-4 T = 0.2G
∴ H_h = 0.39 cos35°- 0.2
= 0.39 × 0.819 – 0.2 ≈ 0.12 G

The vertical component of earth’s magnetic field is given as
H_v = H sinδ
= 0.39 sin35°= 0.22 G
The angle made by the field with its horizontal component is given as
θ = tan^-1 \(\frac{H_{v}}{H_{b}}\)
= tan^-1 \(\frac{0.22}{0.12}\) = 61.39°
The resultant field at the point is given as
H₁ = \(\sqrt{\left(H_{v}\right)^{2}+\left(H_{h}\right)^{2}}\)
= \(\sqrt{(0.22)^{2}+(0.12)^{2}}\) = 0.25 G

For a point 4 cm above the cable
Horizontal component of earth’s magnetic field
H_h = Hcosδ +B = 0.39 cos35° + 0.2 = 0.52 G
Vertical component of earth’s magnetic field
H_v = H sinδ
= 0.39 sin35° = 0.22 G
Angle, θ = tan^-1 \(\frac{H_{v}}{H_{h}}\) = tan^-1\(\frac{0.22}{0.52}\) = 22.90
And resultant field
H₂ = \(\sqrt{\left(H_{v}\right)^{2}+\left(H_{h}\right)^{2}}\)
= \(\sqrt{(0.22)^{2}+(0.52)^{2}}\) = 0.56 G

Question 20.
A compass needle free to turn in a horizontal plane is placed at the centre of circular coil of 30 turns and radius 12 cm. The coil is in a vertical plane making an angle of 45° with the magnetic meridian. When the current in the coil is 0.35 A, the needle points west to east.
(a) Determine the horizontal component of the earth’s magnetic field at the location.
(b) The current in the coil is reversed, and the coil is rotated about its vertical axis by an angle of 90° in the anticlockwise sense looking from above. Predict the direction of the needle. Take the magnetic declination at the places to be zero.
Answer:
Number of turns in the circular coil, N = 30
Radius of the circular coil, r = 12cm = 0.12m
Current in the coil, I = 0.35 A
Angle of dip, δ = 45°

(a) The magnetic field due to current I, at a distance r, is given as
B = \(\frac{\mu_{0} 2 \pi N I}{4 \pi r}\)
∴ B = \(\frac{4 \pi \times 10^{-7} \times 2 \pi \times 30 \times 0.35}{4 \pi \times 0.12}\)
= 5.49 × 10^-5 T
The compass needle points from west to east. Hence, the horizontal component of earth’s magnetic field is given as
B_H = B sinδ
= 5.49 × 10^-5 sin 45°
= 3.88 × 10^-5 T = 0.388G

(b) When the current in the coil is reversed and the coil is rotated about its vertical axis by an angle of 90°, the needle will reverse its original direction. In this case, the needle will point from east to west.

Question 21.
A magnetic dipole is under the influence of two magnetic fields. The angle between the field directions is 60°, and one of the fields has a magnitude of 1.2 × 10^-2 T. If the dipole comes to stable equilibrium at an angle of 15° with this field, what is the magnitude of the other field?
Answer:
The two fields \(\vec{B}\)₁ and \(\vec{B}\)₂ are shown in the figure

here in which a magnet is placed s.t.
∠NOB = 15°
∠B₁OB₂ = 60°
∴ ∠NOB₂ = 60 – 15 = 45°
B₁ = 1.2 × 10^-2 T
B₂ = ?
Let θ₁ and θ₂ he the inclination of the dipole
with \(\vec{B}\)₁ and \(\vec{B}\)₂ respectively.
∴ θ₁ = 15°,θ₂ = 45°
If τ₁ and τ₂ be the torques on the dipole due to \(\vec{B}\)₁ and \(\vec{B}\)₂ respectively, then
Using the relation,
τ = MB sin θ, we get
τ₁ = MB₁ sinθ₁
and τ₂ = MB₂ sinθ₂
As the dipole is in equilibrium, the torques on the dipole due to \(\vec{B}\)₁ and \(\vec{B}\)₂ are equal and opposite, i. e.,

Question 22.
A monoenergetic (18 keV) electron beam initially in the horizontal direction is subjected to a horizontal magnetic field of 0.40 G normal to the initial direction. Estimate the up or down deflection of the beam over a distance of 30 cm (me = 9.11 × 10^-31 kg). [Note : Data in this exercise are so chosen that the answer will give you an idea of the effect of earth’s magnetic field on the motion of the electron beam from the electron gun to the screen in a TV set.]
Answer:
Here, energy = E = 18 KeV = 18 × 1.6 × 10^-16 J
(∵ 1 KeV=10³eV = 10³ × 1.6 × 10^-19 J)
B = horizontal magnetic field = 0.40 G = 0.40 × 10^-4 J
m = 9.11 × 10^-31 kg, e = 1.6 × 10^-19 C
x = 30 cm = 0.30 m
As the magnetic field is normal to the velocity, the charged particle follows circular path in magnetic field. The centrepetal force \(\frac{m v^{2}}{r}\) required for this purpose is provided by force on electron due to magnetic field i. e., BeV

Question 23.
A sample of paramagnetic salt contains 2.0 × 10²⁴ atomic dipoles each of dipole moment 1.5 × 10^-23 JT^-1. The sample is placed under a homogeneous magnetic field of 0.64 T, and cooled to a temperature of 4.2 K. The degree of magnetic saturation achieved is equal to 15%. What is the total dipole moment of the sample for a magnetic field of 0.98 T and a temperature of 2.8 K? (Assume Curie’s law)
Answer:
Number of atomic dipoles, n = 2.0 × 10²⁴
Dipole moment of each atomic dipole, M = 1.5 × 10^-23 JT^-1
When the magnetic field, B₁ = 0.64 T
The sample is cooled to a temperature, T₁ = 4.2 K
Total dipole moment of the atomic dipole, M_tot = n × M
= 2 × 10²⁴ × 1.5 × 10^-23 = 30 JT^-1
Magnetic saturation is achieved at 15%.
Hence, effective dipole moment, M₁ = \(\frac{15}{100}\) × 30 = 4.5 JT^-1
When the magnetic field, B₂ = 0.98 T
Temperature, T₂ = 2.8 K
Its total dipole moment = M₂
According to Curie’s law, we have the ratio of two magnetic dipoles as
\(\frac{M_{2}}{M_{1}}=\frac{B_{2}}{B_{1}} \times \frac{T_{1}}{T_{2}}\)
∴ M₂ = \(\frac{B_{2} T_{1} M_{1}}{B_{1} T_{2}}\) = \(\frac{0.98 \times 4.2 \times 4.5}{2.8 \times 0.64}\) = 10 336 JT^-1
Therefore, 10.336 J T^-1 is the total dipole moment of the sample for a magnetic field of 0.98 T and a temperature of 2.8 K.

Question 24.
A Rowland ring of mean radius 15 cm has 3500 turns of wire wound on a ferromagnetic core of relative permeability 800. What is the magnetic field B in the core for a magnetising current of 1.2 A?
Answer:
Mean radius of the Rowland ring, r = 15 cm = 0.15 m
Number of turns on the ferromagnetic core, N = 3500
Relative permeability of the core material, μ_r = 800
Magnetising current, I = 1.2 A
The magnetic field is given by the relation
B = \(\frac{\mu_{r} \mu_{0} I N}{2 \pi r}\)
B = \(\frac{800 \times 4 \pi \times 10^{-7} \times 1.2 \times 3500}{2 \pi \times 0.15}\) = 4.48T
Therefore, the magnetic field in the core is 4.48 T.

Question 25.
The magnetic moment vectors μ_s and μ_l associated with the intrinsic spin angular momentum S and orbital angular momentum l, respectively, of an electron are predicted by quantum theory (and verified experimentally to a high accuracy) to be given by
μ_g = -(e/m)S, μ_l = -(e/2m)l
Which of these relations is in accordance with the result expected classically? Outline the derivation of the classical result.
Answer:
Out of these two relations, \(\overrightarrow{\mu_{l}}=-\frac{e}{2 m} \vec{l}\) is in accordance with classical physics and can be derived as follows

We know that electrons revolving around the nucleus of an atom in circular orbits behave as tiny current loops having angular momentum \(\vec{\imath}\) given in magnitude as
\(\vec{\imath}\) = mvr …………. (1)
where m = mass of an electron
v = its orbital velocity
r = radius of the circular orbit.
or v_r = \(\frac{l}{m}\) …………… (2)

\(\vec{\imath}\) acts along the normal to the plane of the orbit in upward direction. The orbital motion of electron is taken as equivalent to the flow of conventional current I given by

where – ve sign shows that the electron is negatively
charged. The eqn. (3) shows that μ_e and \(\vec{\imath}\) are opposite to each other i. e., antiparallel and both being normal to the plane of the orbit as shown in the figure

∴ \(\overrightarrow{\mu_{l}}=-\frac{e}{2 m} \cdot \vec{l}\)
\(\frac{\mu_{s}}{S}\) in contrast to \(\frac{\mu_{l}}{\vec{l}}\) is \(\frac{e}{m}\) i.e., twice the classically
expected value. This latter result is an outstanding consequence of modern quantum theory and cannot be obtained classically.

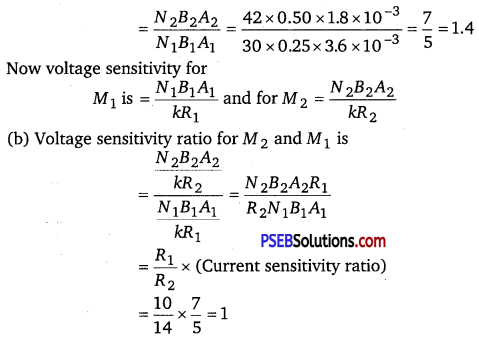
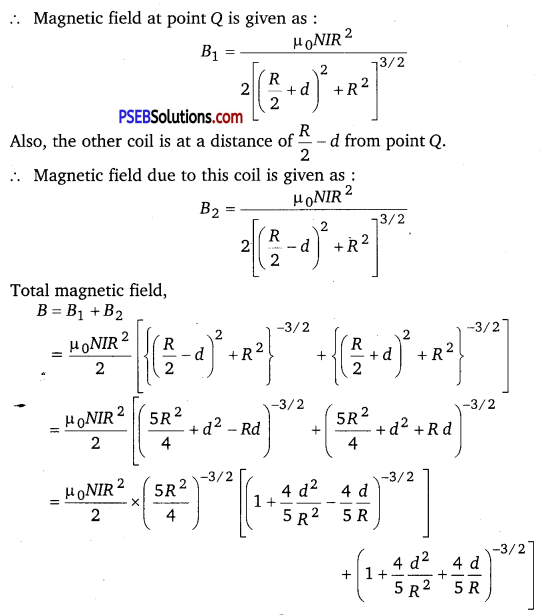
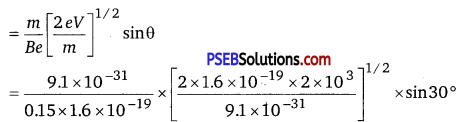
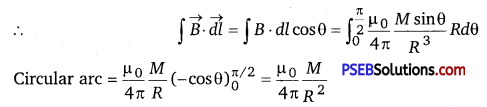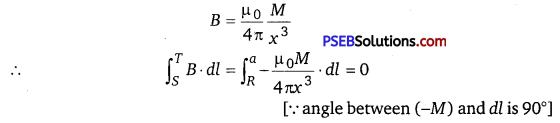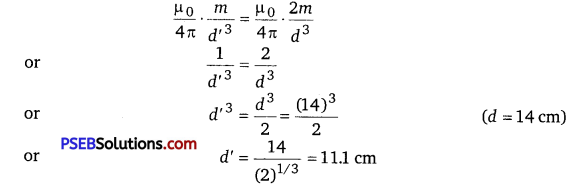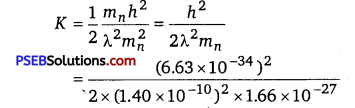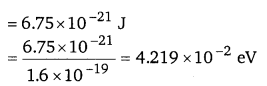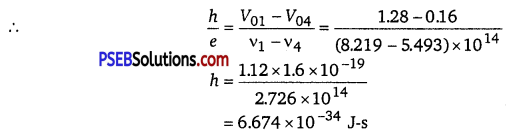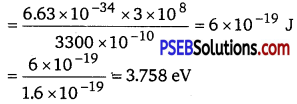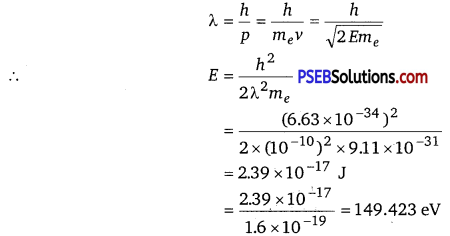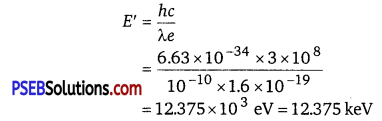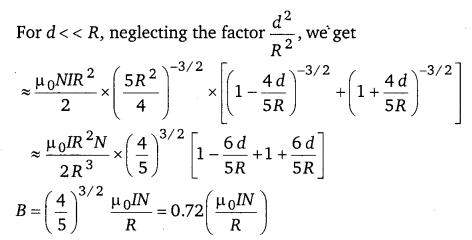Punjab State Board PSEB 12th Class Physics Book Solutions Chapter 11 Dual Nature of Radiation and Matter Textbook Exercise Questions and Answers.

PSEB Solutions for Class 12 Physics Chapter 11 Dual Nature of Radiation and Matter

PSEB 12th Class Physics Guide Dual Nature of Radiation and Matter Textbook Questions and Answers

Question 1.
Find the
(a) maximum frequency, and
(b) minimum wavelength of X-rays produced by 30 kV electrons.
Answer:
Potentialoftheelectrons, V=30 kV= 3O x 10³ V=3 x 10⁴ V
Hence, energy of the electrons, E = 3 x 10⁴ eV
where, e = Charge on an electron = 1.6 x 10^-19C
(a) Maximum frequency produced by the X-rays = v
The energy of the electrons is given by the relation
E=eV=hv
where, h = Planck’s constant = 6.63 x 10^-34 Js
∴ v = \(\frac{e V}{h} \) (∵ E = eV)
= \(\frac{1.6 \times 10^{-19} \times 3 \times 10^{4}}{6.63 \times 10^{-34}}\) = 7.24 x 10¹⁸ Hz
Hence, the maximum frequency of X-rays produced is 7.24 x 10¹⁸ Hz

(b) The minimum wavelength produced by the X-rays is given as
λ = \(\frac{c}{v}\)
= \(\frac{3 \times 10^{8}}{7.24 \times 10^{18}}\)
= 0.414 x 10^-10
= 0.0414 x 10^-9 m
= 0.0414 nm
Hence, the minimum wavelength of X-rays produced is 0.0414 nm.

Question 2.
The work function of caesium metal is 2.14 eV. When light of frequency 6 x 10¹⁴ Hz is incident on the metal surface, photoemission of electrons occurs.
What is the
(a) maximum kinetic energy of the emitted electrons,
(b) stopping potential, and
(c) maximum speed of the emitted photoelectrons?
Answer:
Work function of caesium metal, Φ₀ = 2.14 eV
Frequency of light, v = 6.0 x 10¹⁴ Hz
The maximum kinetic energy is given by the photoelectric effect as
K = hv- Φ₀
where, h = Planck’s constant = 6.63 x 10^-34 Js .
∴ k = \(\frac{6.63 \times 10^{-34} \times 6 \times 10^{14}}{1.6 \times 10^{-19}}-2.14 \)
( ∵ e=1.6 x 10^-19)
= 2.485-2.140 =0.345eV
Hence, the maximum kinetic energy of the emitted electrons is 0.345 eV.

(b) For stopping potential V₀, we can write the equation for kinetic energy
as K=eV₀
∴ V₀ = \(\frac{K}{e}\) (∵ e=1.6×10¹⁹)
= \(\frac{0.345 \times 1.6 \times 10^{-19}}{1.6 \times 10^{-19}}\) =0.345V
Hence, the stopping potential of the material is 0.345 V.

(c) Maximum speed of the emitted photoelectrons = y
Hence, the relation for kinetic energy can be written as
K = \(\frac{1}{2}\) mv²
where, m = mass of an electron = 9.1 x 10^-31 kg
(∴ e=1.6 x 10^-19)
= \(\frac{2 \times 0.345 \times 1.6 \times 10^{-19}}{9.1 \times 10^{-31}}\) = 0.1104 x 10¹²
∴ v = 3.323 x 10⁵ m/s = 332.3 km/s
Hence, the maximum speed of the emitted photoelectrons is 332.3 km/s.

Question 3.
The photoelectric cut off voltage n a certain experiment is 1.5 V. What is the maximum kinetic energy of photoelectrons emitted?
Answer:
Cut-off voltage, V₀ = 1.5 V
Maximum kinetic energy of photoelectrons
E_K =eV₀ =1.5eV=1.5 x 1.6 x 10^-19J
=2.4 x 10^-19J.

Question 4.
Monochromatic light of wavelength 632.8 mn is produced by a helium-neon laser. The power emitted is 9.42 mW.
(a) Find the energy and momentum of each photon in the light beam,
(b) How many photons per second, on the average, arrive at a target irradiated by this beam? (Assume the beam to have uniform cross-section which is less than the target area), and
(c) How fast does a hydrogen atom have to travel in order to have the same momentum as that of the photon?
Answer:
Wavelength of the monochromatic light, 632.8 nm = 632.8 x 10^-9 m
Power emitted by the laser, P = 9.42 mW = 9.42 x 10^-3 W
Planck’s constant, h = 6.63 x 10^-34Js
Speed of light, c=3 x 10⁸ m/s
Mass of a hydrogen atom, m =1.66 x 10^-27 kg
(a) The energy of each photon is given as
E = \(\frac{h c}{\lambda}\)
= \(\frac{6.63 \times 10^{-34} \times 3 \times 10^{8}}{632.8 \times 10^{-9}}\)
= 3.141 x 10^-19

The momentum of each photon is given as
p = \(\frac{h}{\lambda}\)
= \(\frac{6.63 \times 10^{-34}}{632.8 \times 10^{-9}}=1.047 \times 10^{-27} \mathrm{~kg} \mathrm{~ms}^{-1} \)

(b) Number of photons arriving per second, at a target irradiated by the beam = n.
Assume that the beam has a uniform cross-section that is less than the
target area.
Hence, the equation for power can be written as
P=nE
∴ n= \(\frac{P}{E}\)
= \(\frac{9.42 \times 10^{-3}}{3.141 \times 10^{-19}}\) = 3 x 10¹⁶

(c) Momentum of the hydrogen atom is the same as the momentum of the photon, .
p=1.047 x 10²⁷ kgms^-1
Momentum is given as
p = mv
where, v = speed of the hydrogen atom
v = \(\frac{p}{m}\)
= \(=\frac{1.047 \times 10^{-27}}{1.66 \times 10^{-27}}\) = 0.630m/s

Question 5.
The enery flux of sunlight reaching the surface of the earth is 1.388 x 10³ W/m².
How many photons (nearly) per square metre are incident on the Earth per second? Assume that the photons in the sunlight have an average wavelength of 550 rims.
Answer:
Energy flux of sunlight reaching the surface of earth,
Φ = 1.388 x 10³ W/m²
Hence, power of sunlight per square metre, P = 1.388 x 10³W
Speed of light, c = 3 x 10⁸ m/s
Planck’s constant, h = 6.63 x 10^-34 Js
Average wavelength of photons present in sunlight, λ = 550 nm.
=550 x 10^-9m
Number of photons per square metre incident on earth per second = n

Hence, the equation for power can be written as
P = nE
∴ n = \(\frac{P}{E}=\frac{P \lambda}{h c}=\frac{1.388 \times 10^{3} \times 550 \times 10^{-9}}{6.63 \times 10^{-34} \times 3 \times 10^{8}}\)
= 3.84 x 10²¹ photons/m²/s
Therefore, every second, 3.84×1021 photons are incident per square metre on earth.

Question 6.
In an experiment on photoelectric effect, the slope of the cut-off voltage versus frequency of incident light is found to be 4.12 x 10^-15 Vs. Calculate the value of Planck’s constant.
Answer:
The slope of the cut-off voltage (V) versus frequency (v) of an incident light is given as
\(\frac{V}{v}\) = 4.12 x 10^-15 Vs
V is related to frequency by the equation
hv = eV

where, e = charge on an electron = 1.6 x 10^-19
h = Planck’s constant
∴ h = e x \(\frac{V}{v}\)
= 1.6 x 10^-19 x 4.12 x 10^-15
= 6.592 x 10^-34 Js
Therefore, the value of Planck’s constant is 6.592 x 10^-34 Js.

Question 7.
A 100 W sodium lamp radiates energy uniformly in all directions. The lamp is located at the centre of a large sphere that absorbs all the sodium light which is incident on it. The wavelength of the sodium light is 589 nm.
(a) What is the energy per photon associated with the sodium light?
(b) At what rate are the photons delivered to the sphere?
Answer:
Power of the sodium lamp,. P = 100 W
Wavelength of the emitted sodium light, λ = 589 nm = 589 x 10⁹ m
Planck’s constant, h = 6.63 x 10^-34 Js
Speed of light, c = 3 x 10⁸ m/s
The energy per photon associated with the sodium light is given as
E= \(\frac{h c}{\lambda}\)

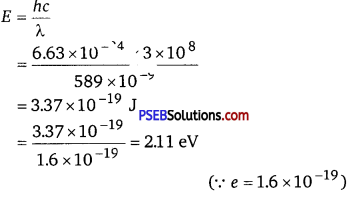
(b) Number of photons delivered to the sphere = n
The equation for power can be written as
P=nE
∴ n = \(\frac{P}{E}=\frac{100}{3.37 \times 10^{-19}}\) = 2.96 x 10²⁰ photons/s
Therefore, every second, 2.96 x 10²⁰ photons are delivered to the sphere.

Question 8.
The threshold frequency for a certain metal is 3.3 x 10¹⁴ Hz. If light of frequency 8.2 x 10¹⁴ Hz is incident on the metal, predict the cut-off voltage for the photoelectric emission.
Answer:
Threshold frequency of the metal, v₀ = 3.3 x 10¹⁴ Hz
Frequency of light incident on the metal, v = 82 x 10¹⁴ Hz
Charge on’an electron, e = 1.6 x 10^-19 C .
Planck’s constant, h = 6.63 x 10^-34 Js
Cut-off voltage for the photoelectric emission from the metal = V₀
The equation for the cut-off energy is given as
eV₀ = h(v-v₀)
V_o = \(\frac{h\left(v-v_{0}\right)}{e}\)
= \(\frac{6.63 \times 10^{-14} \times\left(8.2 \times 10^{14}-3.3 \times 10^{14}\right)}{1.6 \times 10^{-19}}\)
= 2.0292 V
Therefore, the cut-off voltage for the photoelectric emission is 2.0292 V.

Question 9.
The work function for a certain metal Is 4.2 eV. Will this metal give photoelectric emission for incident radiation of
wavelength 330 nm?
Answer:
The energy of incident radiations
E = \(\frac{h c}{\lambda}\) = \(\frac{6.63 \times 10^{-34} \times 3 \times 10^{8}}{330 \times 10^{-9}}\) J
= 6.03 x 10^-19 J
= \(\frac{6.03 \times 10^{-19}}{1.6 \times 10^{-19}}\)eV = 3.77 eV
The work function of photometal, Φ₀ = 4.2 eV
As energy of incident photon is less than work function, photoemission is not possible.

Question 10.
Light of frequency 7.21 x 10¹⁴ Hz is incident on a metal surface. Electrons with a maximum speed of 6.0 x10s m/s are ejected from the surface. What is the threshold frequency for photoemission of electrons?
Answer:
Frequency of light incident on the metal surface, v = 7.21 x 10¹⁴ Hz
Maximum speed of the electrons, v = 6.0 x 10⁵ m/s
Planck’s constant, h = 6.63 x 10^-34 Js
Mass of an electron, m = 9.1 x 10^-31 kg
For threshold frequency v₀, the relation for kinetic energy is written asFor threshold frequency y0, the relation for kinetic energy is written as
\(\frac{1}{2} m v^{2}\) = h(v-v₀)
v₀ = v – \(\frac{m v^{2}}{2 h}\)

Therefore, the threshold frequency for the photoemission of electrons is 4.738 x 10¹⁴ Hz.

Question 11.
Light of wavelength 488 mn is produced by an argon laser which is used in the photoelectric effect. When light from this spectral line is incident on the emitter, the stopping (cut-off) potential of photoelectrons is 0.38 V. Find the work function of the material from which the emitter is made.
Answer:
Wavelength of light produced by the argon laser,
λ = 488 nm = 488 x 10^-9 m
Stopping potential of the photoelectrons, V₀ = 0.38 V
1 eV=l.6 x 10^-19 J
∴ V₀= \(\frac{0.38}{1.6 \times 10^{-19}}\) eV
Planck’s constant, h = 6.63 x 10^-34 Js
Charge on an electron, e = 1.6 x 10^-19 C
Speed of light, c =3 x 10 m/s
From Einstein’s photoelectric effect, we have the relation involving the work function Φ₀ of the material of the emitter as

Therefore, the material with which the emitter is made has the work function of 2.16 eV.

Question 12.
Calculate the
(a) momentum, and
(b) de Broglie wavelength of the electrons accelerated through a potential difference of 56 V.
Answer:
Potential difference, V = 56 V
Planck’s constant, h = 6.63 x 10^-34 Js
Mass of an electron, m = 9.1 x 10^-31 kg
Charge on an electron, e = 1.6 x 10^-19 C
(a) At equilibrium, the kinetic energy of each electron is equal to the accelerating potential, i.e., we can write the relation for velocity (v) of each electron as

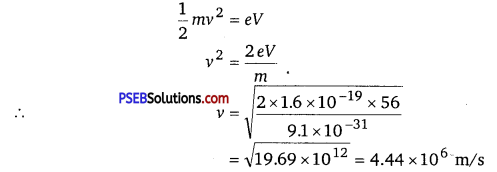
The momentum of each accelerated electron is given as
P = mv
= 9.1 x 10^-31 x 4.44 x 10⁶
= 4.04 x 10^-24 kg m s^-1
Therefore, the momentum of each electron is 4.04 x 10^-24 kg m s^-1.

(b) de Broglie wavelength of an electron accelerating through a potential V is given by the relation

Therefore, the de Broglie wavelength of each electron is 0.1639 nm.

Question 13.
What is the
(a) momentum,
(b) speed, and
(c) de Broglie wavelength of an electron with kinetic energy of 120 eV.
Answer:
Kinetic energy of the electron, E_k = 120 eV
Planck’s constant, h = 6.63 x 10^-34 Js
Mass of an electron, m = 9.1 x 10^-31
Charge on an electron, e = 1.6 x 10^-19 C

(a) For the electron, we can write the relation for kinetic energy as
Ek = \(\frac{1}{2}\) mv²
where, v = speed of the electron
∴ v² = \(\sqrt{\frac{2 e E_{k}}{m}}=\sqrt{\frac{2 \times 1.6 \times 10^{-19} \times 120}{9.1 \times 10^{-31}}}\)
= \(\sqrt{42.198 \times 10^{12}}\) = 6.496 x 10⁶ m/s
Momentum of the electron, p = mv = 9.1 x 10^-31 x 6.496 x 10⁶
=5.91 x 10²⁴ kg ms^-1
Therefore, the momentum of the electron is 5.91 x 10²⁴ kg ms^-1.

Question 14.
The wavelength of light from the spectral emission line of sodium is 589 nm. Find the kinetic energy at which an electron, and a neutron, would have the same de Broglie wavelength.
Answer:
Wavelength of light of sodium line, λ = 589 nm = 589 x 10^-9 m
Mass of an electron, m_e = 9.1 x 10^-31 kg
Mass of a neutron, m_n = 1.66 x 10^-27 kg
Planck’s constant, h = 6.63 x 10^-34 Js

(a) For the kinetic energy K, of an electron accelerating with a velocity v, we have the relation
K = \(\frac{1}{2}\) m_ev² ………………………… (1)
We have the relation for de Broglie wavelength as
λ = \(\frac{h}{m_{e} v}\)
∴ v² = \(\frac{h^{2}}{\lambda^{2} m_{e}^{2}}\) ………………………… (2)
Substituting equation (2) in equation (1), we get the relation
K = \(\frac{1}{2} \frac{m_{e} h^{2}}{\lambda^{2} m_{e}^{2}}=\frac{h^{2}}{2 \lambda^{2} m_{e}}\) ………….. (3)

Hence, the kinetic energy of the electron is 6.9 x 10^-25 J or 4.31 µeV.

(b) Using equation (3), we can write the relation for the kinetic energy of the neutron as = \(\frac{h^{2}}{2 \lambda^{2} m_{n}}\)
= \(\frac{\left(6.63 \times 10^{-34}\right)^{2}}{2 \times\left(589 \times 10^{-9}\right)^{2} \times 1.66 \times 10^{-27}}\)
= 3.78 x 10^-28
= \(\frac{3.78 \times 10^{-28}}{1.6 \times 10^{-19}} \) = 2.36 x 10^-9 eV
= 2.36 neV
Hence, the kinetic energy of the neutron is 3.78 x 10^-28 J or 2.36 neV.

Question 15.
What is the de Broglie wavelength of
(a) a bullet of mass 0.040 kg travelling at the speed of 1.0 km/s,
(b) a ball of mass 0.060 kg moving at a speed of 1.0 m/s, and
(c) a dust particle of mass 1.0 x 10^-9 kg drifting with a speed of 2.2 m/s?
Answer:
(a) Mass of the bullet, m = 0.040 kg
Speed of the bullet, v = 1.0 km/s = 1000 m/s
Planck’s constant, h = 6.63 x 10^-34 Js
de Broglie wavelength of the bullet is given by the relation
λ = \(\frac{h}{m v}\)
= \(\frac{6.63 \times 10^{-34}}{0.040 \times 1000} \) = 1.65 x 10^-35 m

(b) Mass of the ball, m = 0.060 kg
Speed of the ball, v =1.0 m/s
de Brogue wavelength of the ball is given by the relation
λ = \(\frac{h}{m v}\)
= \(\frac{6.63 \times 10^{-34}}{0.060 \times 1}\) = 1.1 x 10^-32 m

(c) Mass of the dust particle, m = 1 x 10^-9 kg
Speed of the dust particle, v = 2.2 m/s
de Brogue wavelength of the dust particle is given by the relation
λ = \(\frac{h}{m v}\)
= \(\frac{6.63 \times 10^{-34}}{2.2 \times 1 \times 10^{-9}}\) = 3.0 x 10^-25 m.

Question 16.
An electron and a photon each have a wavelength of 1.00 run. Find
(a) their momenta,
(b) the energy of the photon, and
(c) the kinetic energy of electrons.
Answer:
Wavelength of an electron (λe) and a photon (λp),λe = λp = λ = 1 nm
= 1 x 10^-9 m
Planck’s constant, h = 6.63 x 10^-34 Js

(a) The momentum of an elementary particle is given by de Broglie relation
λ = \(\frac{h}{p}\)
p = \(\frac{h}{\lambda}\)
It is clear that momentum depends only on the wavelength of the particle. Since the wavelengths of an electron and a photon are equal, both have an equal momentum.
∴ p= \(\frac{6.63 \times 10^{-34}}{1 \times 10^{-9}} \) =6.63 x 10^-25 kgms^-1

(b) The energy of a photon is given by the relation
E= \(\frac{h c}{\lambda}\)
where, speed of light, c =3 x 10⁸ m/s
∴ E = \(\frac{6.63 \times 10^{-34} \times 3 \times 10^{8}}{1 \times 10^{-9} \times 1.6 \times 10^{-19}}\)
= 1243.1 eV = 1.243 keV
Therefore, the energy of the photon is 1.243 keV.

(c) The kinetic energy (K) of an electron having momentum p, is given by the relation
K = \(\frac{1}{2} \frac{p^{2}}{m}\)
where, m = mass of the electron = 9.1 x 10^-31 kg;
p = 6.63 x 10^-25 kgm s^-1

∴ K = \(\frac{1}{2} \times \frac{\left(6.63 \times 10^{-25}\right)^{2}}{9.1 \times 10^{-31}}\) = 2.415 x 10^-19 J
= \(\frac{2.415 \times 10^{-19}}{1.6 \times 10^{-19}}\) = 1.51 eV
Hence, the kinetic energy of the electron is 1.51 eV.

Question 17.
(a) For what kinetic energy of a neutron will the associated de Broglie wavelength be 1.40x 10^-10 m?
(b) Also, find the de Brogue wavelength of a neutron, in thermal equilibrium with matter, having an average kinetic energy of (3/2) kT at 300 K.
Answer:
(a) de Brogue wavelength of the neutron, λ =1.40 x 10^-10 m
Mass of a neutron,m_n =1. 66 x 10^-27 kg
Planck’s constant, h = 6.63 x 10^-34 Js
Kinetic energy (K) and velocity ( v) are related as
K = \( \frac{1}{2} m_{n} v^{2}\) ……………………………… (1)
de Brogue wavelength (λ) and velocity (v) are related as
λ= \(\frac{h}{m_{n} v}\) ……………………………….. (2)
Using equation (2) in equation (1), we get


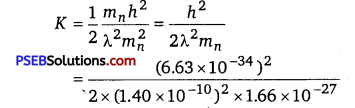
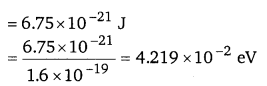
Hence, the kinetic energy of the neutron is 6.75 x 10^-21 J or 4.219 x 10^-2 eV.

(b) Temperature of the neutron, T = 300 K
Boltzmann’s constant, k =1.38 x 10^-23 kg m² s^-2 K^-1
Average kinetic energy of the neutron,
K’ = \(\frac{3}{2} \) kT.
= \(\frac{3}{2} \) x 1.38 x 10^-23 x 300 = 6.21 x 10^-21 J
The relation for the de Broglie wavelength is given as
λ ‘ = \(\frac{h}{\sqrt{2 K^{\prime} m_{n}}}\)

where, m_n = 1.66 x 10^-27 kg
h = 6.63 x 10^-34 Js
K’ = 6.21 x 10^-21 J
∴ λ’ = \(\frac{6.63 \times 10^{-34}}{\sqrt{2 \times 6.21 \times 10^{-21} \times 1.66 \times 10^{-27}}}\)
=1.46 x 10^-10
m = 0.146 nm
Therefore, the de Broglie wavelength of the neutron is 0.146 nm.

Question 18.
Show that the wavelength cf electromagnetic radiation is equal to the de Broglie wavelength of its quantum (photon).
Answer:
The momentum of a photon having energy (hv) is given as ’
p = \(\frac{h v}{c}=\frac{h}{\lambda}\)
λ = \(\frac{h}{p}\) ………………………….. (1)
where, λ = wavelength of the electromagnetic radiation
c = speed of light
h = Planck’s constant
de Broglie wavelength of the photon is given as
λ = \(\frac{h}{m v}\)
But p = mv
∴ λ =\(\frac{h}{p}\) ………………………………….. (2)
where, m = mass of the photon
v = velocity of the photon
Hence, it can be inferred from equations (1) and (2) that the wavelength of the electromagnetic radiation is equal to the de Broglie wavelength of the photon.

Question 19.
What is the de Broglie wavelength of a nitrogen molecule in air at 300 K?
Assume that the molecule is moving with the root mean square speed of molecules at this temperature. (Atomic mass of nitrogen = 140076 u)
Answer:
Temperature of the nitrogen molecule, T = 300 K
Atomic mass of nitrogen = 14.0076 u
Hence, mass of the nitrogen molecule, m = 2 x 14.0076 = 28.0152 u
But 1 u = 1.66 x 10^-27 kg
∴ m=28.0152 x 1.66 x 10^-27 kg
Planck’s constant, h = 6.63 x 10^-34 Js
Boltzmann’s constant, k = 1.38 x 10^-23 K^-1
We have the expression that relates mean kinetic energy \(\left(\frac{3}{2} k T\right)\) of the nitrogen molecuLe with the root mean square speed (v_rms) as

Hence, the de Broglie wavelength of the nitrogen molecule is given as
λ = \(\frac{h}{m v_{\text {rms }}}=\frac{h}{\sqrt{3 m k T}}\)
= \(\frac{6.63 \times 10^{-34}}{\sqrt{3 \times 28.0152 \times 1.66 \times 10^{-27} \times 1.38 \times 10^{-23} \times 300}}\)
= 0.028 x 10^-9 m
= 0.028 nm
Therefore, the de Broglie wavelength of the nitrogen molecule is 0.028 nm.

[Additional Exercises]

Question 20.
(a) Estimate the speed with which electrons emitted from a heated emitter of an evacuated tube impinge on the collector maintained at a potential difference of 500 V with respect to the emitter.
Ignore the small initial speeds of the electrons. The specific charge of the electron, i.e., its elm, is given to be 1.76 x 10¹¹ Ckg^-1.
(b) Use the same formula you employ in (a) to obtain electron speed for an collector potential of 10 MV. Do you see what is wrong? In what way is the formula to be modified?
Answer:
(a) Potential difference across the evacuated tube, V = 500 V
Specific charge of the electron, e/m = 1.76 x 10¹¹ C kg^-1
The speed of each emitted electron is given by the relation for kinetic energy as

KE = \(\frac{1}{2}\) mv²
Therefore, the speed of each emitted electron is
KE =\(\frac{1}{2}\) mv² = eV
∴ v = \(\left(\frac{2 e V}{m}\right)^{1 / 2}=\left(2 V \times \frac{e}{m}\right)^{1 / 2} \)
= (2x 500 xl.76 x 10¹¹)^1/2
= 1.327 x 10⁷ m/s

(b) Potential of the anode, V = 10 MV = 10 x 10⁶ = 10⁷ V
The speed of each electron is given as
v = \(\left(2 V \frac{e}{m}\right)^{1 / 2}\)
= (2 x 10⁷x 1.76 x 10¹¹)^1/2
= 1.88 x 10⁹ m/s .
This result is wrong because nothing can move faster than light. In the above formula, the expression (mv² / 2) for energy can only be used in the non-relativistic limit, i. e., for v < < c.
For very high speed problems, relativistic equations must be considered for solving them. In the relativistic limit, the total energy is given as E = mc²
where, m = relativistic mass
m₀ = \(\left(1-\frac{v^{2}}{c^{2}}\right)^{1 / 2}\) = mass of the particle at rest
Kinetic energy is given as
K = mc² – m₀c²

Question 21.
(a) A monoenergetic electron beam with electron speed of 5.20x 10⁶ ms^-1 is subject to a magnetic field of 1.30 x 10 ⁴T normal to the beam velocity. What is the radius of the circle traced by the beam, given elm for electron equals 1.76x 10¹¹ C kg^-1
(b) Is the formula you employ in (a) valid for calculating radius of the path of a 20 MeV electron beam? If not, in what way is it modified? [Note: Exercises 11.20 (b) and 11.21 (b) take you to relativistic mechanics which is beyond the scope of this book. They have been inserted here simply to emphasise the point that the formulas you use in part (a) of the exercises are not valid at very high speeds or energies. See answers at the end to know what ‘very high speed or energy’ means.]
Answer:
(a) Speed of the electron, v = 5.20 x 10⁶ m/s
Magnetic field experienced by the electron, B = 1.30 x 10^-4 T
Specific charge of the electron, e/m = 1.76 x 10¹¹ C kg’
where, e = charge on the electron = 1.6 x 10^-19 C
m = mass of the electron = 9.1 x 10^-31 kg
The force exerted on the electron is given as
F = e\(|\vec{v} \times \vec{B}|\)
= evBsinθ
θ = angle between the magnetic field and the beam velocity.

The magnetic field is normal to the direction of beam.
∴ θ = 90°
F = evB ……………………………. (1)
The beam traces a circular path of radius, r. It is the magnetic field, due to its bending nature, that provides the centripetal force \(\left(F=\frac{m v^{2}}{r}\right)\) for the
beam.
Hence, equation (1) reduces to
evB = \(\frac{m v^{2}}{r}\)
∴ r = \(\frac{m v}{e B}=\frac{v}{\left(\frac{e}{m}\right) B}=\frac{5.20 \times 10^{6}}{\left(1.76 \times 10^{11}\right) \times 1.30 \times 10^{-4}}\)
= 0.227 m
= 0.227 x 100 cm = 22.7 cm
Therefore, the radius of the circular path is 22.7 cm.

(b) Energy of the electron beam, E = 20 MeV = 20 x 10⁶ x 1.6 x 10^-19 J
The energy of the electron is given as
E = \(\frac{1}{2} \) mv²
∴ v = \(\left(\frac{2 E}{m}\right)^{1 / 2}\)
= \(\sqrt{\frac{2 \times 20 \times 10^{6} \times 1.6 \times 10^{-19}}{9.1 \times 10^{-31}}}\) = 2.652 x 10⁹ m/s

This result is incorrect because nothing can move faster than light. In the above formula, the expression (mv² / 2) for energy can only be used in the non-relativistic limit, i. e., for v« c.

When very high speeds are concerned, the relativistic domain comes into consideration. In the relativistic domain, mass is given as
m = m₀ \(\left[1-\frac{v^{2}}{c^{2}}\right]^{1 / 2}\)
where, m₀ = mass of the particle at rest

Hence, the radius of the circular path is given as
r = mv/eB = \(\frac{m_{0} v}{e B \sqrt{\frac{c^{2}-v^{2}}{c^{2}}}}\).

Question 22.
An electron gun with its collector at a potential of 100 V fires out electrons in a spherical hulh containing hydrogen gas at low pressure (~ 10^-2 nun of Hg). A magnetic field of 2.83 x 10^-4 T curves the path of the electrons in a circular orbit of radius 12.0 cm. (The path can be viewed because the gas ions in the path focus the beam by attracting electrons, and emitting light by electron capture; this method is known as the ‘fine beam tube’ method). Determine elm from the data.
Answer:
Potential of the anode, V = 100 V
Magnetic field experienced by the electrons, B = 2.83 x 10,sup>-4 T
Radius of the circular orbit r = 12.0 cm = 12.0 x 10^-2 m
Mass of each electron = m
Charge on each electron = e ‘
Velocity of each electron = v

The energy of each electron is equal to its kinetic energy, i. e.,
\(\frac{1}{2}\) mv² = eV
v² = \(\frac{2 e V}{m}\)
It is the magnetic field, due to its bending nature, that provides the \(\left(F=\frac{m v^{2}}{r}\right) \) for the beam.
Hence, we can write Centripetal force = Magnetic force mv²
\(\frac{m v^{2}}{r}\) = evB
eB = \(\frac{m v}{r}\)
v = \(\frac{e B r}{m}\) ………………………………. (2)
Putting the value of y in equation (1), we get

Therefore, the specific charge ratio (e/ m) is 1.73 x 10¹¹ C kg^-1.

Question 23.
(a) An X-ray tube produces a continuous spectrum of radiation with its short wavelength end at 0.45 A. What is the maximum energy of a photon in the radiation?
(b) From your answer to (a), guess what order of accelerating voltage (for electrons) is required in such a tube?
Answer:
(a) Wavelength produced by the X-ray tube, λ= 0.45Å= 0.45 x 10^-10 m
Planck’s constant, h = 6.63 x 10^-34 Js
Speed of light, c = 3 x 10⁸ m/s
The maximum energy of a photon is given as
E = \(=\frac{h c}{\lambda}\frac{6.63 \times 10^{-34} \times 3 \times 10^{8}}{0.45 \times 10^{-10} \times 1.6 \times 10^{19}} \) = 27.6 x 10³ eV = 27.6 keV
Therefore, the maximum energy of the X-ray photon is 27.6 keV.

(b) Accelerating voltage provides energy to the electrons for producing X-rays.
To get an X-ray of 27.6 keV, the incident electrons must possess at least 27.6 keV of kinetic electric energy. Hence, an accelerating voltage of the order of 30 keV is required for producing X-rays.

Question 24.
In an accelerator experiment on high-energy collisions of electrons with positrons, a certain event is interpreted as an annihilation of an electron-positron pair of total energy 10.2 BeV into two y-rays of equal energy. What is the wavelength associated with each γ-ray? (1 BeV = 10⁹ eV)
Answer:
Total energy of two γ-rays
E = 10.2 BeV
= 10.2 x 10⁹ eV
= 10.2 x 10⁹ x 1.6 x 10^-19 J
= 10.2 x 1.6 x 10^-10

Hence, the energy of each γ-ray
E’ = \(\frac{E}{2}\)
= \(\frac{10.2 \times 1.6 \times 10^{-10}}{2}\)
= 8.16 x 10^-10 J
Planck’s constant, h = 6.63 x 10^-34 Js
Speed of light, c = 3 x 10⁸ m/s
Energy is related to wavelength as
E ‘ = \(\frac{h c}{\lambda}\)
∴ λ = \(\frac{h c}{E^{\prime}}=\frac{6.63 \times 10^{-34} \times 3 \times 10^{8}}{8.16 \times 10^{-10}}\)
= 2.436 x 10^-16 m
Therefore, the wavelength associated with each γ-ray is 2.436 x 10^-16 m.

Question 25.
Estimating the following two numbers should be interesting. The first number will tell you why radio engineers do not need to worry much about photons. The second number tells you why our eye can never ‘count photons’, even in barely detectable light.
(a) The number of photons emitted per second by a Mediumwave transmitter of 10 kW power, emitting radio waves of wavelength 500 m.
(b) The number of photons entering the pupil of our eye per second corresponding to the minimum intensity of white light that we humans can perceive (~ 10^-10 W m^-2). Take the area of the pupil to be about 0.4 cm², and the average frequency of white light to be about 6 x 10¹⁴ Hz.
Answer:
(a) Power of the medium wave transmitter,
P = 10kW = 10⁴ W = 10⁴ J/s
Hence, the energy emitted by the transmitter per second, E = 10⁴
The wavelength of the radio wave, λ = 500 m
The energy of the wave is given as E₁ = \(\frac{h c}{\lambda}\)
where, h = Planck’s constant = 6.63 x 10^-34 Js
c = speed of light = 3 x 10⁸ m/s
∴ E₁ = \(\frac{6.63 \times 10^{-34} \times 3 \times 10^{8}}{500}\) = 3.96 x 10^-28

Let n be the number of photons emitted by the transmitter.
∴ nE₁ = E
n = \(\frac{E}{E_{1}}\) =\(\frac{10^{4}}{3.96 \times 10^{-28}}\) = 2.525 x 10³¹
≈ 3 x 10³¹
The energy (E₁) of a radio photon is very less, but the number of photons (n) emitted per second in a radio wave is very large. The existence of a minimum quantum of energy can be ignored and the total energy of a radio wave can be treated as being continuous.

(b) Intensity of light perceived by the human eye, I = 10^-10 W m^-2
Area of the pupil, A = 0.4 cm² = 0.4 x 10^-4 m²
Frequency of white light, v = 6 x 10¹⁴ Hz
The energy emitted by a photon is given as
E = hv
where, h = Planck’s constant = 6.63 x 10^-34 Js
∴ E = 6.63 x 10^-34 x 6 x 10¹⁴
= 3.96 x 10^-19 J
Let n be the total number of photons falling per second, per unit area of the pupil. The total energy per unit for n falling photons is given as
E = n x 3.96 x 10^-19 Js^-1 m^-2
The energy per unit area per second is the intensity of light.
∴ E = I
n x 3.96 x 10^-19 = 10^-10
n = \(\frac{10^{-10}}{3.96 \times 10^{-19}}\)
= 2.52 x 10⁸ m² s^-1

The total number of photons entering the pupil per second is given as
n_A =n x A
= 2.52 x 10⁸ x 0.4 x 10^-4
= 1.008 x 10⁴ s^-1
This number is not as large as the one found in problem (a), but it is large enough for the human eye to never see the individual photons.

Question 26.
Ultraviolet light of wavelength 2271 Å from a 100 W mercury source irradiates a photo-cell made of molybdenum metal. If the stopping potential is -1.3 V, estimate the work function of the metal. How would the photo-cell respond to a high intensity (~10⁵ Wm^-2) red light of wavelength 6328 Å produced by a He-Ne laser?
Answer:
Wavelength of ultraviolet light, λ = 2271 Å = 2271 x 10^-10 m
Stopping potential of the metal, V₀ = 1.3 V
Planck’s constant, h = 6.63 x 10^-34 Js
Charge on an electron, e = 1.6 x 10^-19 C
Work function of the metal = Φ₀
Frequency of light = v
We have the photo-energy relation from the photoelectric effect as
Φ₀ =hv₀
v₀ = \(\frac{\phi_{0}}{h}\)
= \(\frac{6.64 \times 10^{-19}}{6.63 \times 10^{-34}} \)
= 1.006 x 10¹⁵ Hz = 4.15 eV
Let v₀ be the threshold frequency of the metal.
∴ Φ₀ = hv₀
v₀ = \(\frac{\phi_{0}}{h}\)
= \(\frac{6.64 \times 10^{-19}}{6.63 \times 10^{-34}}\)
= 1.006 x 10¹⁵ Hz

Wavelength of red light, λ_r = 6328 Å = 6328 x 10^-10
∴ Frequency of red light, v_r = \(\frac{c}{\lambda_{r}}=\frac{3 \times 10^{8}}{6328 \times 10^{-10}} \)
= 4.74 x 10¹⁴ Hz
Since v₀ > v_r, the photocell will not respond to the red light produced by the laser.

Question 27.
Monochromatic radiation of wavelength 640.2 nm (1 nm = 10^-9 m) from a neon lamp irradiates photosensitive material made of caesium on tungsten. The stopping voltage is measured to be 0.54 V. The source is replaced by an iron source and its 427.2 nm line irradiates the same photo-cell. Predict the new stopping voltage.
Answer:
Wavelength of the monochromatic radiation, λ = 640.2 nm = 640.2 x 10^-9 m
Stopping potential of the neon lamp, V₀ = 0.54 V
Charge on an electron, e = 1.6 x 10^-19 C
Planck’s constant, h = 6.63 x 10^-34 Js
Let Φ₀ the work function and v be the frequency of emitted light. We have the photo-energy relation from the photoelectric effect as

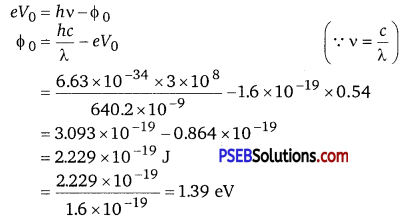
Wavelength of the radiation emitted from an iron source, λ’ = 427.2 nm = 427.2 x 10^-9 m
Let V’₀ be the new stopping potential. Hence, photo-energy is given as
eV’₀ = \(\frac{h c}{\lambda^{\prime}}-\phi_{0}\)

Hence, the new stopping potential is 1.50 eV.

Question 28.
A mercury lamp is a convenient source for studying frequency dependence of photoelectric emission, since it gives a number of spectral lines ranging from the UV to the red end of the visible spectrum. In our experiment with rubidium photo-cell, the following lines from a mercury source were used :
λ₁ =3650 Å,
λ₂ = 4047 Å,
λ₃ =4358 Å,
λ₄ =5461 Å,
λ₅ =6907 Å,
The stopping voltages, respectively, were measured to be :
V₀₁ = 1.28 V,
V₀₂ = 0.95 V,
V₀₃ = 0.74 V,
V₀₄ = 0.16 V,
V₀₅ = 0 V.
Determine the value of Planck’s constant h, the threshold frequency and work function for the material.
[Note: You will notice that to get h from the data, you will need to know e (which you can take to be 1.6 x 10^-19 C). Experiments of this kind on Na, Li, K, etc. were performed by Millikan, who, using his own value of e (from the oil-drop experiment) confirmed Einstein’s photoelectric equation and at the same time gave an independent estimate of the value of h.]
Answer:
Given, the following wavelength from a mercury source were used
λ₁ =3650 Å, = 3650 x 10^-10 m
λ₂ = 4047 Å, = 4047 x 10^-10m
λ₃ =4358 Å, = 4358 x 10^-10 m
λ₄ =5461 Å, = 5461 x 10^-10 m
λ₅ =6907 Å, = 6907 x 10^-10m
The stopping voltages are as follows
V01 =1.28 V,
V02 = 0.95V,
V03 =0.74V,
V04 =0.16 V,
V5 =0

Frequencies corresponding to wavelengths

As we know that
eV₀ = hv-Φ₀
v₀ = \(\frac{h v}{e}-\frac{\phi_{0}}{e}\)

As the graph between V₀ and frequency v is a straight line.
The slope of this graph gives the values of \(\frac{h}{e} \)

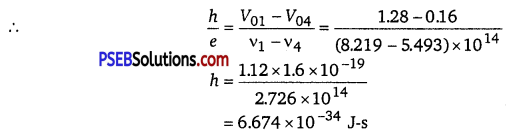
(b) Work function, Φ₀ = hv₀
= 6.574 x 10^-34 x 5 x 10¹⁴
= 32.870 x 10^-20J
= 2.05eV.

Question 29.
The work function for the following metals is given: Na: 2.75 eV; K: 2.30 eV; Mo: 4.17 eV; Ni: 5.15 eV.
Which of these metals will not give photoelectric emission for a radiation of wavelength 3300 Å from a He-Cd laser placed 1 m away from the photo-cell? What happens if the laser is brought nearer and placed 50 cm away?
Answer:
Mo and Ni will not show photoelectric emission in both cases.
Wavelength for the radiation, λ – 3300 Å = 3300 x 10^-10 m
Speed of light, c = 3 x 10⁸ m/s
Planck’s constant, h = 6.63 x 10^-34 Js
The energy of incident radiation is given as
E = \(\frac{h c}{\lambda}\)

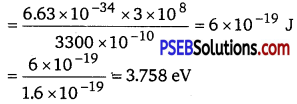
= 3.758eV
It can be observed that the energy of the incident radiation is greater than the work function of Na and K only. It is less for Mo and Ni. Hence, Mo and Ni will not show photoelectric emission. If the source of light is brought near the photocells and placed 50 cm away from them, then the intensity of radiation will increase. This does not affect the energy of the radiation. Hence, the result will be the same as before. However, the photoelectrons emitted from Na and K will increase in proportion to intensity.

Question 30.
Light of intensity 10^-5 W m^-2 falls on a sodium photo-cell of surface area 2 cm². Assuming that the top 5 layers of sodium absorb the incident energy, estimate time required for photoelectric emission in the wave-picture of radiation. The work function for the metal is given to be about 2 eV. What is the implication of your answer?
Answer:
Given, intensity of light = 10^-5 W/m²
Area = 2 cm² = 2 x 10^-4 m²
Work function for the metal Φ₀ = 2 eV
Let t be the time.
The effective atomic area of Na = 10^-20 m² and it contains one conduction electron per atom.
Number of conduction electrons in five layers
= \(\frac{5 \times \text { Area of one layer }}{\text { Effective atomic area }}=\frac{5 \times 2 \times 10^{-4}}{10^{-20}}\)
= 10⁷
We know that sodium has one free electron (or conduction electron) per atom.
Incident power on the surface area of photocell
= Incident intensity x Area on the surface area of photocell
= 10^-5 x 2 x 10^-4
= 2 x 10^-9W
The electron present in all the 5 layers of sodium will share the incident energy equally.
Energy absorbed per second per electron,
E = \(\frac{\text { Incident power }}{\text { Number of electrons in five layers }} \)
= \(\frac{2 \times 10^{-9}}{10^{17}}\) = 2 x 10^-26W
Time required for emission by each electron, t = \(\frac{\text { Energy required per electron }}{\text { Energy absorbed per second }} \) = \(\frac{2 \times 1.6 \times 10^{-19}}{2 \times 10^{-26}} \) = 1.6 x 10⁷s
which is about 0.5 yr.
The answer obtained implies that the time of emission of electrons is very large and is not agreement with the observed time of emission. There is no time lag between the incidence of light and the emission of photoelectrons. Thus, it is implied that the wave theory cannot be applied in this experiment.

Question 31.
Crystal diffraction experiments can be performed using X-rays, or electrons accelerated through appropriate voltage. Which probe has greater energy? (For quantitative comparison, take the wavelength of the probe equal to 1 Å, which is of the order of interatomic spacing in the lattice) (m_e = 9.11 x 10^-31 kg).
Answer:
An X-ray probe has a greater energy than an electron probe for the same wavelength.
Wavelength of light emitted from the probe, λ = 1 Å = 10^-10 m
Mass of an electron, me = 9.11 x 10^-31 kg
Planck’s constant, h = 6.63 x 10^-34 Js
Charge on an electron, e = 1.6 x 10^-19 C
The kinetic energy of the electron is given as
K = \(\frac{1}{2} m_{n} v^{2}\)
m_nv = \(\sqrt{2 K m_{n}}\)
where, v = velocity of the electron
m_nv = momentum (p) of the electron

According to the de Brogue principle, the de Brogue wavelength is given as

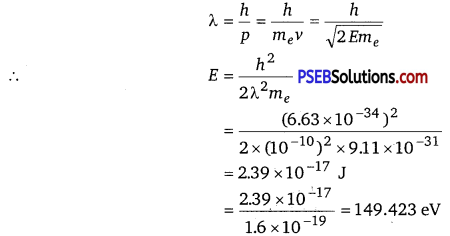
The energy of a photon,

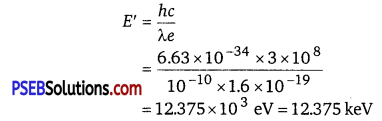
Hence, a photon has a greater energy than an electron for the same wavelength.

Question 32.
(a) Obtain the de Broglie wavelength of a neutron of kinetic energy 150 eV. As you have seen in Exercise 11.31, an electron beam of this energy is suitable for crystal diffraction experiments. Would a neutron beam of the same energy be equally suitable? Explain. (m = 1.675x 10^-27 kg)
(b) Obtain the de Brogue wavelength associated with thermal neutrons at room temperature (27C). Hence, explain why a fast neutron beam needs to be thermalised with the environment before it can be used for neutron diffraction experiments.
Answer:
(a) de Brogue wavelength= 2.327 x 10^-12 m; neutron is not suitable for
the diffraction experiment Kinetic energy of the neutron, K = 150 eV
= 150 x 1.6 X 10^-19
=2.4 x 10^-17 J
Mass of a neutron, m_n = l.675 x 10^-27 kg
The kinetic energy of the neutron is given by the relation
K = \(\frac{1}{2} m_{n} v^{2}\)
m_nv = \(\sqrt{2 K m_{n}}\)
where, y = velocity of the neutron
m_nv = momentum of the neutron

deBroglie wavelength of the neutron is given as
λ = \(\frac{h}{m_{n} v}=\frac{h}{\sqrt{2 K m_{n}}}\)
It is clear that wavelength is inversely proportional to the square root of mass.
Hence, wavelength decreases with increase in mass and vice versa.
∴ λ = \(\frac{6.63 \times 10^{-34}}{\sqrt{2 \times 2.4 \times 10^{-17} \times 1.675 \times 10^{-27}}}\)
= 2.327 x 10^-12 m
It is given in the previous problem that the interatomic spacing of a crystal is about 1 Å, i.e., 10^-10 m.
Hence, the interatomic spacing is about a hundred times greater. Hence, a neutron beam of energy 150 eV is not
suitable for diffraction experiments.

(b) de Brogue wavelength =1.447 x 10^-10 m
Room temperature, T = 27°C = 27+ 273 = 300 K
The average kinetic energy of the neutron is given as
E=\(\frac{3}{2}\) kT
where, k = Boltzmann’s constant = 1.38 x10^-23 J mol^-1K^-1
The wavelength of the neutron is given as
λ = \(\frac{h}{\sqrt{2 m_{n} E}}=\frac{h}{\sqrt{3 m_{n} k T}}\)
= \(\frac{6.63 \times 10^{-34}}{\sqrt{3 \times 1.675 \times 10^{-27} \times 1.38 \times 10^{-23} \times 300}}\)
=1.447 x 10^-10 m
This wavelength is comparable to the interatomic spacing of a crystal. Hence, the high energy neutron beam should first be thermalised, before using it for diffraction.

Question 33.
An electron microscope uses electrons accelerated by a voltage of 50 kV. Determine the de Brogue wavelength associated with the electrons, if other factors (such as numerical aperture, etc.) are taken to be roughly the same, how does the resolving power of an electron microscope compare with that of an optical microscope which uses yellow light?
Answer:
Electrons are accelerated by a voltage, V = 50 kV = 50 x 10³ V
Charge on an electron, e 1.6 x 10^-19 C
Mass of an electron, me = 9.11 x 10^-31 kg
Wavelength of yellow light = 5.9 x 10^-7 m

The kinetic energy of the electron is given as
E=eV
=l.6 x 10^-19x 50x 10³
= 8 x 10^-15 J
de Brogue wavelength is given by the relation
λ = \(\frac{h}{\sqrt{2 m_{e} E}}\)
= \(\frac{6.63 \times 10^{-34}}{\sqrt{2 \times 9.11 \times 10^{-31} \times 8 \times 10^{-15}}}\)
= 5.467 x 10^-12 m
This wavelength is nearly 10⁵ times less than the wavelength of yellow light. The resolving power of a microscope is inversely proportional to the wavelength of light used. Thus, the resolving power of an electron microscope is nearly 10⁵ times that of an optical microscope.

Question 34.
The wavelength of a probe is roughly a measure of the size of a structure that it can probe in some detail. The quark structure of protons and neutrons appears at the minute length scale of 10^-15 m or less. This structure was first, probed in early 1970s using high energy electron beams produced by a linear accelerator at Stanford, USA. Guess what might have been the order of energy of these electron beams. (Rest mass energy of electron = 0.511 MeV.)
Answer:
Wavelength of a proton or a neutron, λ ≈ 10^-15 m
Rest mass energy of an electron,
m₀c² =0.511 MeV
= 0.511 x 10⁶ x 1.6 x 10^-19
= 0.8176 x 10^-13 J
Planck’s constant, h = 6.63 x 10^-34 Js
Speed of light, c = 3 x 10⁸ m/s ,

The momentum of a proton or a neutron is given as
p = \(\frac{h}{\lambda}\)
= \(\frac{6.63 \times 10^{-34}}{10^{-15}}\) = 6.6 x 10^-19 kg m/s
The relativistic relation for energy (E) is given as
E² = p²c² +m₀²c⁴
= (6.6x 10^-19 x 3 x 10⁸)² + (0.8176 x 10^-13)²
= 392.04 x 10^-22 +0.6685 x 10^-26
≈ 392.04 x 10^-22
∴ E = 1.98x 10^-10 J
Thus, the electron energy emitted from the accelerator at Stanford, USA might be of the order of 1.24 BeV.

Question 35.
Find the typical de Broglie wavelength associated with a He atom in helium gas at room temperature (27°C) and 1 atm pressure, and compare it with the mean separation between two atoms under these conditions.
Answer:
de Broglie wavelength associated with He atom = 0.7268 x 10^-10 m .
Room temperature, T = 27°C =27 + 273 = 300 K
Atmospheric pressure, P = 1 atm = 1.01 x 10⁵ Pa
Atomic weight of a He atom = 4
Avogadro’s number, N_A = 6.023 x 10²³
Boltzmann’s constant, k = 1.38 x 10^-23 J mol^-1 K^-1

Average energy of a gas at temperature T, is given as
E = \(\frac{3}{2}\) kT
de Broglie wavelength is given by the relation
λ = \(\frac{h}{\sqrt{2 m E}}\)
where, m = mass of a He atom

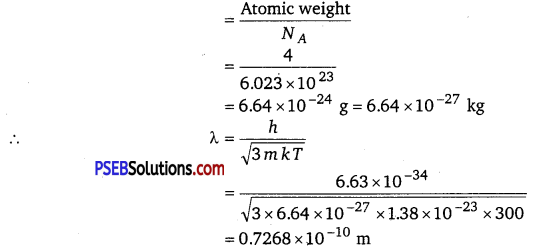
We have the ideal gas formula
PV = RT
PV = kNT
∵ \(\frac{V}{N}=\frac{k T}{P}\)
where V = volume of the gas
N = number of moles of the gas
Mean separation between two atoms of the gas is given by the relation
r = \(\left(\frac{V}{N}\right)^{1 / 3}=\left(\frac{k T}{P}\right)^{1 / 3}=\left[\frac{1.38 \times 10^{-23} \times 300}{1.01 \times 10^{5}}\right]^{1 / 3}\)
= 3.35 x 10^-9 m
Hence, the mean separation between the atoms is much greater than the de Broglie wavelength.

Question 36.
Compute the typical de Broglie wavelength of an electron in a metal at 2 7° C and compare it with the mean separation between two electrons in a metal which is given to be about 2 x 10¹⁰ m. [Note: Exercises 11.35 and 11.36 reveal that while the wave-packets associated with gaseous molecules under ordinary conditions are non-overlapping, the electron wave- packets in a metal strongly overlap with one another. This suggests that whereas molecules in an ordinary gas can be distinguished apart, electrons in a metal cannot be distinguished apart from one another. This indistinguishability has many fundamental implications which you will explore in more advanced Physics courses.]
Answer:
Temperature, T = 27°C = 27 +273 = 300 K
Mean separation between two electrons, r = 2 x 10^-10 m
de Broglie wavelength of an electron is given as
λ = \(\frac{h}{\sqrt{3 m k T}}\)
where,
h = Planck’s constant = 6.63 x 10^-34 Js
m = Mass of an electron = 9.11 x 10 ^-31 kg
k = Boltzmann’s constant = 1.38 x 10^-23 J mol^-1 K^-1
∴ λ = \(\frac{6.63 \times 10^{-34}}{\sqrt{3 \times 9.11 \times 10^{-31} \times 1.38 \times 10^{-23} \times 300}}\)
= 6.2 x 10⁹ m
Hence, the de Broglie wavelength is much greater than the given inter-electron separation.

Question 37.
Answer the following questions :
(a) Quarks inside protons and neutrons are thought to carry fractional charges [(+2/3)e ; (-1/3)e]. Why do they not show up in Millikan’s oil-drop experiment?
(b) What is so special about the combination e/m? Why do we not simply talk of e and m separately?
(c) Why should gases be insulators at ordinary pressures and start conducting at very low pressures?
(d) Every metal has a definite work function. Why do all photoelectrons not come out with the same energy if incident radiation is monochromatic? Why is there an energy distribution of photoelectrons?
(e) The energy and momentum of an electron are related to the frequency and wavelength of the associated matter wave by the relations :
E = hv, p = \(\frac{\boldsymbol{h}}{\lambda}\)
But while the value of λ is physically significant, the value of v (and therefore, the value of the phase speed vλ) has no physical significance. Why?
Answer:
(a) Quarks inside protons and neutrons carry fractional charges. This is because nuclear force increases extremely if they are pulled apart. Therefore, fractional charges may exist in nature; observable charges are siti the integral multiple of an electrical charge.

(b) Thè basic relations for electric field and magnetic field are \(\left(e V=\frac{1}{2} m v^{2}\right)\) and \(\left(e B v=\frac{m v^{2}}{r}\right) \) respectively.

These relations include e (electric charge), y (velocity), m (mass), V (potential), r (radius), and B (magnetic field)._These relations give the value of velocity of an electron as \(\left(v=\sqrt{2 v\left(\frac{e}{m}\right)}\right) \text { and }\left(v=B r\left(\frac{e}{m}\right)\right)\) respectively. It can be observed from these relations that the dynamics of an electron is determined not by e and m separately, but by the ratio e / m.

(c) At atmospheric pressure, the ions of gases have no chance of reaching their respective electrons because of collision and recombination with other gas molecules. Hence, gases are insulators at atmospheric pressure. At low pressure, ions have a chance of reaching their respective electrodes and constitute a current. Hence, they conduct electricity at these pressures.

(d) The work function of a metal is the minimum energy required for a conduction electron to get out of the metal surface. All the electrons in an atom do not have the same energy level. When a ray having some photon energy is incident on a metal surface, the electrons come out from different levels with different energies. Hence, these emitted electrons show different energy distributions.

(e) The absolute value of energy of a particle is arbitrary within the additive constant. Hence, wavelength (λ) is significant, but the frequency (v) associated with an electron has no direct physical significance. Therefore, the product vλ (phase speed) has no physical significance. Group speed is given as

This Quantity has a physical meaning.

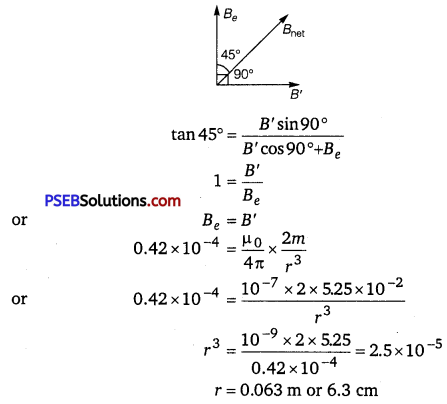
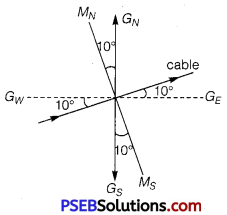
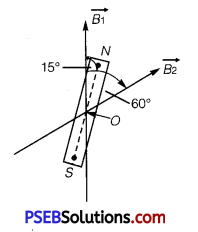
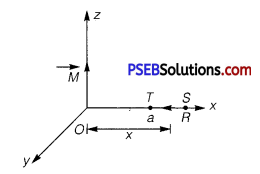
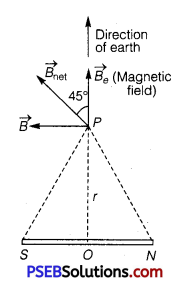
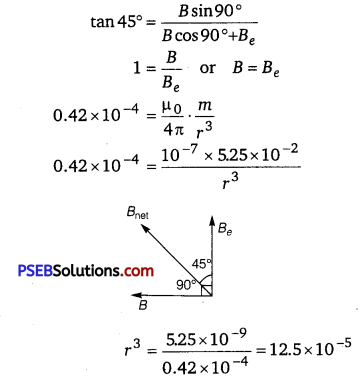
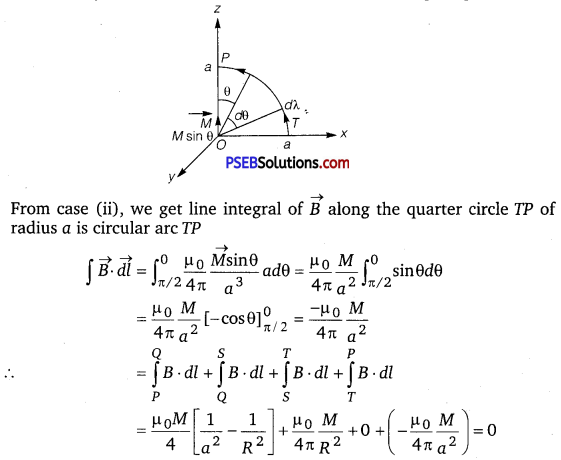
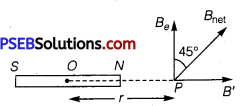
Punjab State Board PSEB 12th Class Physics Book Solutions Chapter 4 Moving Charges and Magnetism Textbook Exercise Questions and Answers.

PSEB Solutions for Class 12 Physics Chapter 4 Moving Charges and Magnetism

PSEB 12th Class Physics Guide Moving Charges and Magnetism Textbook Questions and Answers

Question 1.
A circular coil of wire consisting of 100 turns, each of radius
8.0 cm carries a current of 0.40 A. What is the magnitude of the magnetic field B at the centre of the coil?
Answer:
Number of turns on the circular coil, n = 100
Radius of each turn, r = 8.0 cm = 0.08 m
Current flowing in the coil, I = 0.4 A
Magnitude of the magnetic field at the centre of the coil is given by the relation,
\(|B|=\frac{\mu_{0}}{4 \pi} \frac{2 \pi n I}{r}\)
where, μ₀ = 4π × 10^-7 TmA^-1
\(|B|\) = \(\frac{4 \pi \times 10^{-7}}{4 \pi}\) × \(\frac{2 \pi \times 100 \times 0.4}{0.08}\)
= 3.14 × 10^-4T
Hence, the magnitude of the magnetic field is 3.14 × 10^-4 T

Question 2.
A long straight wire carries a current of 35 A. What is the magnitude of the field B at a point 20 cm from the wire?
Answer:
Current in the wire, I = 35 A
Distance of the point from the wire, r = 20 cm = 0.2 m
Magnitude of the magnetic field at this point is given as
B = \(\frac{\mu_{0}}{4 \pi} \frac{2 I}{r}\)
B = \(\frac{4 \pi \times 10^{-7} \times 2 \times 35}{4 \pi \times 0.2}\)
= 3.5 × 10^-5T
Hence, the magnitude of the magnetic field at a point 20 cm from the wire is 3.5 × 10^-5 T.

Question 3.
A long straight wire in the horizontal plane carries a current of 50 A in north to south direction. Give the magnitude and direction of B at a point 2.5 m east of the wire.
Answer:
Current in the wire, I = 50 A
A point is 2.5 m away from the east of the wire.
∴ Magnitude of the distance of the point from the wire, r = 2.5 m
Moving Charges and Magnetism ini
Magnitude of the magnetic field at that point is given by the relation,
B = \(\frac{\mu_{0} 2 I}{4 \pi r}\)
= \(\frac{4 \pi \times 10^{-7} \times 2 \times 50}{4 \pi \times 2.5}\)
= 4 × 10^-6 T
The point is located normal to the wire length at a distance of 2.5 m. The direction of the current in the wire is vertically downward. Hence, according to the Maxwell’s right hand thumb rule, the direction of the magnetic field at the given point is vertically upward.

Question 4.
A horizontal overhead power line carries a current of 90 A in east to west direction. What is the magnitude and direction of the magnetic field due to the current 1.5 m below the line?
Answer:
Current in the power line, I = 90 A
Point is located below the power line at distance, r = 1.5 m
Hence, magnetic field at that point is given by the relation,
B = \(\frac{\mu_{0} 2 I}{4 \pi r}\)
= \(\frac{4 \pi \times 10^{-7} \times 2 \times 90}{4 \pi \times 1.5}\) = 1.2 × 10^-5T
The current is flowing from east to west. The point is below the power line. Hence, according to Maxwell’s right hand thumb rule, the direction of the magnetic field is towards the south.

Question 5.
What is the magnitude of magnetic force per unit length on a wire carrying a current of 8 A and making an angle of 30° with the direction of a uniform magnetic field of 0.15 T?
Answer:
Current in the wire, I = 8 A
Magnitude of the uniform magnetic field, B = 0.15 T
Angle between the wire and magnetic field, θ = 30°.
Magnetic force per unit length on the wire is given as,
F = BI sinθ
= 0.15 × 8 × sin30°
= 0.15 × 8 × \(\frac{1}{2}\)
= 0.15 × 4 = 0.6 Nm^-1
Hence, the magnetic force per unit length on the wire is 0.6 Nm^-1.

Question 6.
A 3.0 cm wire carrying a current of 10 A is placed inside a solenoid perpendicular to its axis. The magnetic field inside the solenoid is given to be 0.27 T. What is the magnetic force on the wire?
Answer:
Length of the wire, l = 3 cm = 0.03 m
Current flowing in the wire, I = 10 A
Magnetic field, B = 0.27 T
Angle between the current and magnetic field, θ = 90°
Magnetic force exerted on the wire is given as,
F = BIl sinθ
= 0.27 × 10 × 0.03 × sin 90°
= 8.1 × 10^-2N
Hence, the magnetic force on the wire is 8.1 × 10^-2 N.

Question 7.
Two long and parallel straight wires A and B carrying currents of
8.0 A and 5.0 A in the same direction are separated by a distance of 4.0 cm. Estimate the force on a 10 cm section of wire A.
Answer:
Here, let I₁ and I₂ be the currents flowing in the straight long and parallel wires A and B respectively.
∴ I₁ = 8.0 A, I₂ = 5.0 A flowing in the same direction
r = distance between A and B = 4.0 cm = 4 × 10^-2 m
If F’ be the force per unit length on wire A, then using
F’ = \(\frac{\mu_{0}}{4 \pi} \cdot \frac{2 I_{1} I_{2}}{r}\), we get
F’ = 10^-7 × \(\frac{2 \times 8 \times 5}{4 \times 10^{-2}}\) Nm^-1
= 20 × 10^-5 Nm^-1

If F be the force on a section of length 10 cm of wire A, then
F = F’ × l (Here,l = 10 × 10^-2m)
= 20 × 10^-5 × 10 × 10^-2N
= 2 × 10^-5N

Question 8.
A closely wound solenoid 80 cm long has 5 layers of windings of 400 turns each. The diameter of the solenoid is 1.8 cm. If the current carried is 8.0 A, estimate the magnitude of B inside the solenoid near its centre.
Answer:
Length of the solenoid, l = 80 cm = 0.8 m
Number of turns in each layer = 400
Number of layers in the solenoid = 5
∴ Total number of turns on the solenoid, N = 5 × 400 = 2000
Diameter of the solenoid, D = 1.8 cm = 0.018 m
Current carried by the solenoid, I = 8.0 A
Magnitude of the magnetic field inside the solenoid near its centre is given by the relation,
g.hoM
B = \(=\frac{\mu_{0} N I}{l}\)
B = \(\frac{4 \pi \times 10^{-7} \times 2000 \times 8}{0.8}\)
= 8 π × 10^-3 = 2.512 × 10^-2 T
Hence, the magnitude of the magnetic field inside the solenoid near its centre is 2.512 × 10^-2 T.

Question 9.
A square coil of side 10 cm consists of 20 turns and carries a current of 12 A. The coil is suspended vertically and the normal to the plane of the coil makes an angle of 30° with the direction of a uniform horizontal magnetic field of magnitude 0.80 T. What is the magnitude of torque experienced by the coil?
Answer:
Length of a side of the square coil, l = 10 cm = 0.1 m
Current flowing in the coil, I = 12 A
Number of turns on the coil, N = 20
Angle made by the plane of the coil with magnetic field, θ = 30°
Strength of magnetic field, B = 0.80 T
Magnitude of the torque experienced by the coil in the magnetic field is given by the relation,
τ = NBIAsinθ
where, A = Area of the square coil
⇒ l × l = 0.1 × 0.1 = 0.01 m²
∴ τ = 20 × 0.80 × 12 × 0.01 × sin30°
= 20 × 0.80 × 12 × 0.01 × \(\frac{1}{2}\)
= 0.96 N m
Hence, the magnitude of the torque experienced by the coil is 0.96 N m.

Question 10.
Two moving coil meters, M₁ and M₂ have the following particulars:
R₁ = 10 Ω, N₁ = 30,
A₁ = 3.6 × 10^-3 m², B₁ = 0.25T
R₂ = 14Ω, N₂ = 42,
A₂ = 1.8 × 10^-3 m², B₂ = 0.50 T
(The spring constants are identical for the two meters). Determine the ratio of (a) current sensitivity and (b) voltage sensitivity of M₂ and M₁.
Answer:
Here,R₁ = 10 n, N₁ = 30, A₁ = 3.6 x 10^-3 m²,B₁ = 0.25T for coil M₁
R₂ = 14 Q, N₂ = 42, A₂ = 1.8 x 10^-3 m²,B₂ = 0.50T for coil M₂.
We know that current sensitivity and voltage sensitivity are given by the formulae
Current sensitivity = \(\frac{N B A}{k}\)
and Voltage sensitivity = \(\frac{N B A}{k R}\)
Here, k₁ = k₂ for the two coils = k (say)
∴ Current sensitivity for M₁ is given by = N₁B₁A₁/ k and for M₂ = N₂B₂A₂ / k

(a) Current sensitivity ratio for M₂ and M₁ is given by
= \(\frac{\frac{N_{2} B_{2} A_{2}}{k}}{\frac{N_{1} B_{1} A_{1}}{k}}\)

Question 11.
In a chamber, a uniform magnetic field of 6.5 G (1 G = 10^-4 T) is maintained. An electron is shot into the field with a speed of 4.8 × 10⁶ ms^-1 normal to the field. Explain why the path of the electron is a circle. Determine the radius of the circuit orbit.
e,= 1.6 × 10^-19 (m_e = 9.1 × 10 ^-31 kg
Answer:
Magnetic field strength, B = 6.5 G = 6.5 × 10^-4 T
Speed of the electron, y = 4.8 × 10⁶ m/s
Charge on the electron, e,= 1.6 × 10^-19 C
Mass of the electron, me 9.1 × 10^-31 kg
Angle between the electron and magnetic field, θ = 90°
Magnetic force exerted on the electron in the magnetic field is given as :
F = evBsinθ
This force provides centripetal force to the moving electron. Hence, the electron starts moving in a circular path of radius r.
Hence, centripetal force exerted on the electron,
F_e = \(\frac{m v^{2}}{r}\)
In equilibrium, the centripetal force exerted on the electron is equal to the magnetic force t.e.,
F_e = F
\(\frac{m v^{2}}{r}\) = evBsinθ
r = \(\frac{m v}{B e \sin \theta}\)
= \(\frac{9.1 \times 10^{-31} \times 4.8 \times 10^{6}}{6.5 \times 10^{-4} \times 1.6 \times 10^{-19} \times \sin 90^{\circ}}\)
= 4.2 × 10^-2 m = 4.2 cm
Hence, the radius of the circular orbit of the electron is 4.2 cm.

Question 12.
In Exercise 4.11 obtain the frequency of revolution of the electron in its circular orbit. Does the answer depend on the speed of the electron? Explain.
Answer:
Magnetic field strength, B = 6.5 × 10^-4 T
Charge on the electron, e = 1.6 × 10^-19 C
Mass of the electron, m_e = 9.1 × 10^-31 kg
Velocity of the electron, v = 4.8 × 10⁶ m/s
Radius of the orbit, r = 4.2 cm = 0.042 m
Frequency of revolution of the electron = v
Angular frequency of the electron = ω = 2πv
Velocity of the electron is related to the angular frequency as :
v = rω
In the circular orbit, the magnetic force on the electron is balanced by the centripetal force. Hence, we can write :
evB = \(\frac{m v^{2}}{r}\)
eB = \(\frac{m}{r}\) (rω) = \(\frac{m}{r}\) (r2πv)
v = \(\frac{B e}{2 \pi m}\)

This expression for frequency is independent of the speed of the electron. On substituting the known values in this expression, we get the frequency as:
V = \(=\frac{6.5 \times 10^{-4} \times 1.6 \times 10^{-19}}{2 \times 3.14 \times 9.1 \times 10^{-31}}\)
= 18.2 × 10⁶ Hz ≈ 18 MHz
Hence, the frequency of the electron is around 18 MHz and is independent of the speed of the electron.

Question 13.
(a) A circular coil of 30 turns and radius 8.0 cm carrying a current of 6.0 A is suspended vertically in a uniform horizontal magnetic field of magnitude 1.0 T. The field lines make an angle of 60° with the normal of the coil. Calculate the magnitude of the counter torque that must be applied to prevent the coil from turning.

(b) Would your answer change, if the circular coil in (a) were replaced by a planar coil of some irregular shape that encloses, the same area? (All other particulars are also unaltered.)
Answer:
(a) Number of turns on the circular coil, N = 30
Radius of the coil, r = 8.0 cm = 0.08 m
Area of the coil = πr² = π(0.08)² = 0.0201 m²
Current flowing in the coil, I = 6.0 A
Magnetic field strength, B = 1.0 T
Angle between the field lines and normal with the coil surface,
θ = 60°
The coil experiences a torque in the magnetic field. Hence, it turns. The. counter torque applied to prevent the coil from turning is given by the relation,
τ = N IBAsinθ …………… (1)
= 30 × 6 × 1 × 0.0201 × sin60°
= 180 × 0.0201 × \(\frac{\sqrt{3}}{2}\)
= 3.133 Nm

(b) It can be inferred from relation (1) that the magnitude of the applied torque is not dependent on the shape of the coil. It depends on the area of the coil. Hence, the answer would not change if the circular coil in the above case is replaced by a planar coil of some irregular shape that encloses the same area.

Question 14.
Two concentric circular coils X and Y of radii 16 cm and 10 cm, respectively, lie in the same vertical plane containing the north to south direction. Coil X has 20 turns and carries a current of 16 A; coil Y has 25 turns and carries a current of 18 A. The sense of the current in X is anticlockwise, and clockwise in Y, for an observer looking at the coils facing west. Give the magnitude and direction of the net magnetic field due to the coils at their centre.
Answer:
Radius of coil X, r₁ = 16 cm = 0.16 m
Radius of coil Y, r₂ = 10 cm = 0.10 m
Number of turns on coil X, n₁ = 20
Number of turns on coil Y, n₂ = 25
Current in coil X,I₁ =16 A
Current in coil Y, I₂ = 18 A
Magnetic field due to coil X at their centre is given by the relation,
B₁ = \(\frac{\mu_{0} n_{1} I_{1}}{2 r_{1}}\)
∴ B₁ = \(\frac{4 \pi \times 10^{-7} \times 20 \times 16}{2 \times 0.16}\)
= 4π × 10^-4 T (towards East)
Magnetic field due to coil Y at their centre is given by the relation,
B₂ = \(\frac{\mu_{0} n_{2} I_{2}}{2 r_{2}}\)
\(\frac{4 \pi \times 10^{-7} \times 25 \times 18}{2 \times 0.10}\)
= 9π × 10^-4 T (towards West)

Hence, net magnetic field can be obtained as:
B = B₂ – B₁
= 9π × 10^-4 – 4π × 10^-4
= 5π × 10 T
= 1.57 × 10^-3 T (towards West)

Question 15.
A magnetic field of 100 G (1 G = 10^-4 T) is required which is uniform in a region of linear dimension about 10 cm and area of cross-section about 10^-3 m². The maximum current-carrying capacity of a given coil of wire is 15 A and the number of turns per unit length that can be wound round a core is at most 1000 turns m^-1 . Suggest some appropriate design particulars of a solenoid for the required purpose. Assume the core is not ferromagnetic.
Answer:
Here, B = magnetic field = 100 G = 100 × 10^-4 = 10^-2 T,
I_max = maximum current carried by the coil = 15 A
n = number of turns per unit length = 1000 turns m^-1 = 10 tums/cm
l = length of linear region = 10 cm
A = area of cross-section = 10^-3 m².

To produce a magnetic field in the above mentioned region, a solenoid can be made so that well within the solenoid, the magnetic field is uniform. To do so, we may take the length L of the solenoid 5 times the length of the region and area of the solenoid 5 times the area of region.

∴ L = 5l = 5 × 10 = 50 cm = 0.5m
and A = 5 × 10^-3 m²
∴ If r be the radius of the solenoid, then
πr² = A = 5 × 10^-3
or r = \(\sqrt{\frac{5 \times 10^{-3}}{3.14}}\) = 0.04 m = 4 cm
Also let us wind 500 turns on the coil so that the number of turns per m is
n = \(\frac{500}{0.5}\) = 1000 turns m^-1
∴ Using formula, μ₀nI = B, we get
I = \(\frac{B}{\mu_{0} n}\) = \(\frac{10^{-2}}{4 \pi \times 10^{-7} \times 1000}\) = 7.96 A ≈ 8A

So, a current of 8 A can be passed through it to produce a uniform magnetic field of 100 G in the region. But this is not a unique way. If we wind 300 turns on the solenoid, then number of turns is
n = \(\frac{300}{0.5}\) = 600 per m.
∴ I= \(\frac{B}{\mu_{0} n}\) = \(\frac{10^{-2}}{4 \pi \times 10^{-7} \times 600}\) = 13.3 A
i. e., a current of 13.3 A can be passed through it to produce the magnetic field of loo G.
Similarly, if no. of turns = 400,
then, n = \(\frac{400}{0.5}\) = 800 per m.
∴ I = \(\frac{B}{\mu_{0} n}\) = \(\frac{10^{-2}}{4 \pi \times 10^{-7} \times 800}\) = 9.95 A
i. e., a current of 10 A can be passed ≈ 10 A
Through it to produce B = 100 G
Thus we may achieve the result in a number of ways.

Question 16.
For a circular coil of radius R and N turns carrying current J, the magnitude of the magnetic field at a point on its axis at a distance x from its centre is given by,
B = \(\frac{\mu_{0} I R^{2} N}{2\left(x^{2}+R^{2}\right)^{3 / 2}}\)
(a) Show that this reduces to the familiar result for field at the centre of the coil.
(b) Consider two parallel co-axial circular coils of equal radius R, and number of turns N, carrying equal currents in the same direction, and separated by a distance R. Show that the field on the axis around the mid-point between the coils is uniform over a distance that is small as compared to JR, and is given by,
B = 0.72 \(\frac{\mu_{0} \boldsymbol{N I}}{\boldsymbol{R}}\), approximately.
[Such an arrangement to produce a nearly uniform magnetic field over a small region is known as Helmholtz coils.]
Answer:
Radius of circular coil = R
Number of turns on the coil = N
Current in the coil = I
Magnetic field at a point on its axis at distance x from its centre is given by the relation,
B = \(\frac{\mu_{0} I R^{2} N}{2\left(x^{2}+R^{2}\right)^{3 / 2}}\)

(a) If the magnetic field at the centre of the coil is considered, then x = 0
∴ B = \(\frac{\mu_{0} I R^{2} N}{2 R^{3}}=\frac{\mu_{0} I N}{2 R}\)
This is the familiar result for magnetic field at the centre of the coil,

(b) Radii of two parallel co-axial circular coils = R
Number of turns on each coil = N
Current in both coils = I
Distance between both the coils = R
Let us consider point Q at distance d from the centre.
Then, one coil is at a distance of \(\frac{R}{2}\) + d from point Q.


Hence, it is proved that the field on the axis around the mid-point between the coils is uniform.

Question 17.
A toroid has a core (non-ferromagnetic) of inner radius 25 cm and outer radius 26 cm, around which 3500 turns of a wire are wound. If the current in the wire is 11 A, what is the magnetic field (a) outside the toroid, (b) inside the core of the toroid, and (c) in the empty space surrounded by the toroid.
Answer:
Here, I = 11 A,
Total number of turns = 3500
Mean radius of toroid, r = \(\frac{25+26}{2}\)
r = 25.5cm = 25.5 × 10^-2 m
Total length of the toroid = 2πr = 2π × 25.5 × 10^-2
= 51π × 10^-2m
Therefore, number of turns per unit length,
n = \(\frac{3500}{51 \pi \times 10^{-2}}\)

(a) The field is non-zero only inside the core surrounded by the windings of the toroid. Therefore, the field outside the toroid is zero.

(b) The field inside the core of the toroid
B = μ₀nI
B = 4π × 10^-7 × \(\frac{3500}{51 \pi \times 10^{-2}}\) × 11
B = 3.02 × 10^-2 T

(c) For the reason given in (a), the field in the empty space surrounded by toroid is also zero.

Question 18.
Answer the following questions:
(a) A magnetic field that varies in magnitude from point to point but has a constant direction (east to west) is set up in a chamber. A charged particle enters the chamber and travels undeflected along a straight path with constant speed. What can you say about the initial velocity of the particle?

(b) A charged particle enters an environment of a strong and non-uniform magnetic field varying from point to point both in magnitude and direction and comes out of it following a complicated trajectory. Would its final speed equal the initial speed if it suffered no collisions with the environment?

(c) An electron travelling west to east enters a chamber having a uniform electrostatic field in north to south direction. Specify the direction in which a uniform magnetic field should be set up to prevent the electron from deflecting from its straight line path.
Answer:
(a) The initial velocity of the particle is either parallel or anti-parallel to the magnetic field. Hence, it travels along a straight path without suffering any deflection in the field.

(b) Yes, the final speed’ of the charged particle will be equal to its initial speed. This is because magnetic force can change the direction of velocity, but not its magnitude.

(c) An electron travelling from west to east enters a chamber having a uniform electrostatic field in the north-south direction. This moving electron can remain undeflected if the electric force acting on it is equal and opposite of magnetic field. Magnetic force is directed towards the south. According to Fleming’s left hand rule, magnetic field should be applied in a vertically downward direction.

Question 19.
An electron emitted by a heated cathode and accelerated through a potential difference of 2.0 kV, enters a region with uniform magnetic field of 0.15 T. Determine the trajectory of the electron if the field (a) is transverse to its initial velocity, (b) makes an angle of 30° with the initial velocity.
Answer:
Magnetic field strength, B = 0.15 T
Charge on the electron, e = 1.6 × 10^-19C
Mass of the electron, m = 9.1 × 10^-31 kg
Potential difference, V = 2.0 kV = 2 × 10³ V
Thus, kinetic energy of the electron = eV
⇒ eV = \(\frac{1}{2}\)mv²
v = \(\sqrt{\frac{2 e V}{m}}\) ……………. (1)
where, v = Velocity of the electron
Magnetic force on the electron provides the required centripetal force of the electron. Hence, the electron traces a circular path of radius r.

(a) When the magnetic field is transverse to the initial velocity. The force on the electron due to transverse magnetic field = Bev

= 100.55 × 10^-5
= 1.01 × 10^-3 m = 1 mm
Hence, the electron has a circular trajectory of radius 1.0 mm normal to the magnetic field.

(b) When the magnetic field makes an angle θ of 30° with initial velocity, the initial velocity will be,
v₁ = vsinθ
From equation (2), we can write the expression for new radius as :
r₁ = \(\frac{m v_{1}}{B e}\)
= \(\frac{m v \sin \theta}{B e}\)

= 0.5 × 10^-3 m = 0.5mm
Hence, the electron has a helical trajectory of radius 0.5 mm along the magnetic field direction.

Question 20.
A magnetic field set up using Helmholtz coils (described in Exercise 4.16) is uniform in a small region and has a magnitude of 0.75 T. In the same region, a uniform electrostatic field is maintained in a direction normal to the common axis of the coils. A narrow beam of (single species) charged particles all accelerated through 15 kV enters this region in a direction perpendicular to both the axis of the coils and the electrostatic Held. If the beam remains undeflected when the electrostatic field is 9.0 × 10^-5 V m^-1, make a simple guess as to what the beam contains. Why is the answer not unique?
Answer:
Magnetic field, B = 0.75 T
Accelerating voltage, V = 15 kV = 15 × 10³ V
Electrostatic field, E = 9 × 10^-5 Vm^-1
Mass of the electron = m
Charge on the electron = e
Velocity of the electron = v
Kinetic energy of the electron = eV
⇒ \(\frac{1}{2}\)mv² = eV
∴ \(\frac{e}{m}=\frac{v^{2}}{2 V}\) ……………. (1)
Since the particle remains undeflected by electric and magnetic fields, we can infer that the electric field is balancing the magnetic field.
∴ eE = evB
v = \(\frac{E}{B}\) …………. (2)
Putting equation (2) in equation (1), we get

= 4.8 × 10⁷ C/kg
Also, we know that \(\frac{e}{m}\) for proton is 9.6 × 10^-7 C kg^-1 . It follows that the charged particle under reference has the value of \(\frac{e}{m}\) half of that for the
proton, so its mass is clearly double the mass of proton. Thus the beam may be of deutrons.
The answer is not unique as the ratio of charge to mass i. e.,
4.8 × 10⁷ C kg^-1 may be satisfied by many other charged particles, surch as
He⁺⁺(\(\frac{2 e}{2 m}\)) and Li³⁺ (\(\frac{3 e}{3 m}\))
which have the same value of \(\frac{e}{m}\).

Question 21.
A straight horizontal conducting rod of length 0.45 m and mass 60 g is suspended by two vertical wires at its ends. A current of 5.0 A is set up in the rod through the wires.
(a) What magnetic field should be set up normal to the conductor in order that the tension in the wires is zero?
(b) What will be the total tension in the wires if the direction of current is reversed keeping the magnetic field same as before? (Ignore the mass of the wires.) g = 9.8 ms^-2.
Answer:
Length of the rod, l = 0.45 m
Mass of the rod, m = 60 g = 60 × 10^-3 kg
Acceleration due to gravity, g = 9.8 m/s²
Current in the rod flowing through the wire, I = 5 A

(a) Magnetic field (B) is equal and opposite to the weight of the rod i.e.,
BIl = mg
∴ B = \(\frac{m g}{I l}\) = \(\frac{60 \times 10^{-3} \times 9.8}{5 \times 0.45}\) = 0.26T
A horizontal magnetic field of 0.26 T normal to the length of the conductor should be set up in order to get zero tension in the wire. The magnetic field should be such that Fleming’s left hand rule gives an upward magnetic force.

(b) If the direction of the current is reversed, then the force due to magnetic field and the weight of the rod acts in a vertically downward direction.
∴ Total tension in the wire = BIl + mg
= 0.26 × 5 × 0.45 + (60 × 10^-3) × 9.8
= 1.176 N

Question 22.
The wires which connect the battery of an automobile to its starting motor carry a current of 300 A (for a short time). What is the force per unit length between the wires if they are 70 cm long and 1.5 cm apart? Is the force attractive or repulsive?
Answer:
Current in both wires, I = 300 A
Distance between the wires, r = 1.5 cm = 0.015 m
Length of the both wires, l = 70 cm = 0.7 m
Force between the two wires is given by the relation,
F = \(\frac{\mu_{0} I^{2}}{2 \pi r}\)
∴ F = \(\frac{4 \pi \times 10^{-7} \times(300)^{2}}{2 \pi \times 0.015}\) = 1.2 N/M
Since the direction of the current in the wires is opposite, a repulsive force exists between them.

Question 23.
A uniform magnetic field of 1.5 T exists in a cylindrical region of radius 10.0 cm, its direction parallel to the axis along east to west. A wire carrying current of 7.0 A in the north to south direction passes through this region. What is the magnitude and direction of the force on the wire if,
(a) the wire intersects the axis,
(b) the wire is turned from N-S to northeast-northwest direction,
(c) the wire in the N-S direction is lowered from the axis by a distance of 6.0 cm?
Answer:
Magnetic field strength, B = 1.5 T
Radius of the cylindrical region, r = 10cm = 0.1m
Current in the wire passing through the cylindrical region, I = 7 A

(a) If the wire intersects the axis, then the length of the wire is the diameter of the cylindrical region.
Thus, l = 2r = 2 × 0.1 = 0.2 m
Angle between magnetic field and current, θ = 90°
Magnetic force acting on the wire is given by the relation,
F = BIl sinθ
= 1.5 × 7 × 0.2 × sin90° = 2.1N
Hence, a force of 2.1 N acts on the wire in a vertically downward direction.

(b) New length of the wire after turning it to the northeast-northwest direction can be given as:
l₁ = \(\frac{l}{\sin \theta}\)
Angle between magnetic field and current, θ = 45°
Force on the wire,
F₁ = BIl₁ sinθ
\(\frac{B I l}{\sin \theta}\) = sinθ
= BIl = 1.5 × 7 × 0.2 = 2.1 N
Hence, a force of 2.1 N acts vertically downward on the wire. This is independent of angle because Z sinG is fixed.

(c) The wire is lowered from the axis by distance, d = 6.0 cm
Let l₂ be the new length of the wire.
∴ (\(\frac{l_{2}}{2}\))² = 4(d + r)
= 4 (10 + 6) = 4(16)
∴ l₂ = 8 × 2 = 16 cm = 0.16 m
Magnetic force exerted on the wire,
F₂ = BIl₂
= 1.5 × 7 × 0.16 = 1.68 N
Hence, a force of 1.68 N acts in a vertically downward direction on the wire.

Question 24.
A uniform magnetic field of 3000 G is established along the positive z-direction. A rectangular loop of sides 10 cm and 5 cm carries a current of 12 A. What is the torque on the loop in the different cases shown in Fig. 4.28? What is the force on each case? Which case corresponds to stable equilibrium?

Answer:
Here,
B = uniform magnetic field
= 3000 gauss along z-axis
= 3000 × 10^-4T = 0.3 T
l = length of rectangular loop
= 10 cm = 0.1 m
b = breath of rectangular loop
= 5 cm = 0.05 m
∴ A = area of rectangular loop
= l × b = 10 × 5 = 50cm² = 50 × 10^-4 m²
Torque on the loop is given by
\(\vec{\tau}\) = (I\(\vec{A}\)) × \(\vec{B}\)
IA = 50 × 10^-4 × 12 = 0.06 Am⁺²

(a) Here, I\(\vec{A}\) = 0.06î Am², \(\vec{B}\) = 0.3k̂T
∴ \(\vec{\tau}\) = 0.06î × 0.3k̂= -1.8 × 10^-2 Nm ĵ
i.e., τ = 1.8 × 10^-2 Nm and acts along negative y-axis.



Net force on a planar loop in a magnetic field is always zero, so force is
zero in each case.
Case (e) corresponds to stable equilibrium as 7 A is aligned with B while (f) corresponds to unstable equilibrium as 7 A is antiparallel to B.

Question 25.
A circular coil of 20 turns and radius 10 cm is placed in a uniform magnetic field of 0.10 T normal to the plane of the coil. If the current in the coil is 5.0 A, what is the
(a) total torque on the coil,
(b) total force on the coil,
(c) average force on each electron in the coil due’ to the magnetic field?
(The coil is made of copper wire of cross-sectional area 10^-5 m², and the free electron density in copper is given to be about 10²⁹ m^-3.)
Answer:
Number of turns on the circular coil, n = 20
Radius of the coil, r = 10cm = 0.1m
Magnetic field strength, B = 0.10 T
Current in the coil, I = 5.0 A
(a) The total torque on the coil is zero because the field is uniform.
(b) The total force on the coil is zero because the field is uniform.
(c) Cross-sectional area of copper coil, A = 10^-5 m²
Number of free electrons per cubic meter in copper, N = 10²⁹ / m³
Charge on the electron, e = 1.6 × 10^-19C
Magnetic force, F = Bev_d
Where, v_d = \(\frac{I}{N e A}\)
∴ F = \(\frac{B e I}{N e A}=\frac{B I}{N A}\) = \(\frac{0.10 \times 5.0}{10^{29} \times 10^{-5}}\) 5 × 10^-25N
Hence, the average force on each electron is 5 × 10^-25 N.

Question 26.
A solenoid 60 cm long and of radius 4.0 cm has 3 layers of windings of 300 turns each. A 2.0 cm long wire of mass 2.5 g lies inside the solenoid (near its centre) normal to its axis; both the wire and the axis of the solenoid are in the horizontal plane. The wire is connected through two leads parallel to the axis of the solenoid to an external battery which supplies a current of 6.0 A in the wire. What value of current (with appropriate sense of circulation) in the windings of the solenoid can support the weight of the wire? g = 9.8 ms^-2.
Length of the solenoid, L = 60 cm = 0.6 m
Radius of the solenoid, r = 4.0 cm = 0.04 m
It is given that there are 3 layers of windings of 300 turns each.
∴ Total number of turns, n = 3 × 300 = 900
Length of the wire, l = 2 cm = 0.02 m
Mass of the wire, m = 2.5 g = 2.5 × 10 ^-3 kg
Current flowing through the wire, i = 6 A
Acceleration due to gravity, g=9.8m/s²
Magnetic field produced inside the solenoid, B = \(\frac{\mu_{0} n I}{L}\)
where, μ₀ = 4π × 10^-7 TmA^-1
I = Current flowing through the windings of the solenoid Magnetic force is given by the relation,
F = Bil = \(\frac{\mu_{0} n i I}{L}\)l
Also, the force on the wire is equal to the weight of the wire.
∴ mg = \(\frac{\mu_{0} n \text { Iil }}{L}\)
I = \(\frac{m g L}{\mu_{0} \text { nil }}\)
= \(\frac{2.5 \times 10^{-3} \times 9.8 \times 0.6}{4 \pi \times 10^{-7} \times 900 \times 0.02 \times 6}\) = 108A
Hence, the current flowing through the solenoid is 108 A.

Question 27.
A galvanometer coil has a resistance of 12 Ω and the metre shows full scale deflection for a current of 3 mA. How will you convert the metre into a voltmeter of range 0 to 18 V?
Answer:
Resistance of the galvanometer coil, G = 12 Ω
Current for which there is full scale deflection, I_g = 3 mA = 3 × 10^-3 A
Range of the voltmeter is 0, which needs to be converted to 18 V.
∴ V = 18 V
Let a resistor of resistance R be connected in series with the galvanometer to convert it into a voltmeter. This resistance is given as
R = \(\frac{V}{I_{g}}\) – G
= \(\frac{18}{3 \times 10^{-3}}\) – 12 = 6000 – 12 = 5988 Ω
Hence, a resistor of resistance 5988 Ω is to be connected in series with the galvanometer.

Question 28.
A galvanometer coil has a resistance of 15 Ω and the metre shows full scale deflection for a current of 4 mA. How will you convert the metre into an ammeter of range 0 to 6 A?
Answer:
Resistance of the galvanometer coil, G = 15 Ω
Current for which the galvanometer shows full scale deflection,
I_g = 4 mA = 4 × 10^-3A
Range of the ammeter is 0, which needs to be converted to 6 A.
∴ Current, I = 6 A
A shunt resistor of resistance S is to be connected in parallel with the galvanometer to convert it into an ammeter. The value of S is given as :
S = \(\frac{I_{g} G}{I-I_{g}}\) = \(\frac{4 \times 10^{-3} \times 15}{6-4 \times 10^{-3}}\)
S = \(\frac{6 \times 10^{-2}}{6-0.004}=\frac{0.06}{5.996}\) ≈ 0.01Ω = 10mΩ
Hence, a 10 mΩ shunt resistor is to be connected in parallel with the galvanometer.

Punjab State Board PSEB 12th Class Physics Book Solutions Chapter 12 Atoms Textbook Exercise Questions and Answers.

PSEB Solutions for Class 12 Physics Chapter 12 Atoms

PSEB 12th Class Physics Guide Atoms Textbook Questions and Answers

Question 1.
Choose the correct alternative from the clues given at the end of the each statement:
(a) The size of the atom in Thomson’s model is ………………….. the atomic size in Rutherford’s model, (much greater than/no different from/much less than.)
(b) In the ground state of ………………………………… electrons are in stable equilibrium, while in …………………….. electrons always experience a net force. (Thomson’s model/Rutherford’s model.)
(c) A classical atom based on ……………………………. is doomed to collapse. (Thomson’s model/Rutherford’s model.)
(d) An atom has a nearly continuous mass distribution in a ………………………… but has a highly non-uniform mass distribution in …………………….. (Thomson’s model/Rutherford’s model.)
(e) The positively charged part of the atom possesses most of ………………………. the mass in ………………….. (Rutherford’s model/both the models.)
Answer:
(a) The size of the atom in Thomson’s model is no different from the atomic size in Rutherford’s model.
(b) In the ground state of Thomson’s model, electrons are in stable equilibrium while, in Rutherford’s model, electrons always experience a net force.
(c) A classical atom based on Rutherford’s model is doomed to collapse.
(d) An atom has a nearly continuous mass distribution in Thomson’s model but has a highly non-uniform mass distribution in Rutherford’s model.
(e) The positively charged part of the atom possesses most of the mass in both the models.

Question 2.
Suppose you are given a chance to repeat the alpha-particle scattering experiment using a thin sheet of solid hydrogen in place of the gold foil. (Hydrogen is a solid at temperatures below 14 K.) What results do you expect?
Answer:
The basic purpose of scattering experiment is not completed because solid hydrogen will be a much lighter target as compared to the alpha particle acting as a projectile. By using the conditions of elastic collisions, the hydrogen will move much faster as compared to alpha after the collision. We cannot determine the size of hydrogen nucleus.

Question 3.
What is the shortest wavelength present in the Paschen series of spectral lines?
Answer:
Rydberg’s formula is given as
\(\frac{h c}{\lambda}\) = \(21.76 \times 10^{-19}\left[\frac{1}{n_{1}^{2}}-\frac{1}{n_{2}^{2}}\right]\)
where, h = Planck’s constant = 6.63 x 10^-34 Js
c=Speed oflight=3 x 10⁸ m/s (n₁ and n₂ are integers)
The shortest wavelength present in the Paschen series of the spectral lines
is given for values n₁ = 3 and n₂ = ∞

= 822.65 nm

Question 4.
A difference of 2.3 eV separates two energy levels in an atom. What is the frequency of radiation emitted when the atom makes a transition from the upper level to the lower level?
Answer:
According to Bohr’s postulate
E₂ – E₁ = hv
∴ Frequency of emitted radiation

Question 5.
The ground state energy of hydrogen atom is -13.6 eV. What are the kinetic and potential energies of the electron in this state?
Answer:
Given, the ground state energy of hydrogen atom
E=-13.6eV
We know that,
Kinetic Energy, E_K = -E = 13.6 eV
Potential Energy E_p = -2KE =-2 x 13.6 = -27.2eV

Question 6.
A hydrogen atom initially In the ground level absorbs a photon, which excites it to the n = 4 level. Determine the wavelength and frequency of photons.
Answer:
The energy levels of H-atom are given by
E_n = \(-\frac{R h c}{n^{2}}\)
For given transition n₁ =1, n₂ = 4
∴ E₁ = \(-\frac{R h c}{1^{2}}\) ,E₂= \(-\frac{R h c}{4^{2}}\)
∴ Energy of absorbed photon
ΔE=E₂ -E₁ =Rhc \(\left(\frac{1}{1^{2}}-\frac{1}{4^{2}}\right)\)
or
ΔE = \(\frac{15}{16}\) Rhc ………………………….. (1)
∴ The wavelength of absorbed photon λ is given by

Question 7.
(a) Using the Bohr’s model, calculate the speed of the electron in a hydrogen atom in the n =1, 2, and 3 levels.
(b) Calculate the orbital period in each of these levels.
Answer:
(a) Let y1 be the orbital speed of the electron in a hydrogen atom in the ground state level, n₁ =1.
For charge (e) of an electron, v₁ is given by the relation,
v₁ = \(\frac{e^{2}}{n_{1} 4 \pi \varepsilon_{0}\left(\frac{h}{2 \pi}\right)}=\frac{e^{2}}{2 \varepsilon_{0} h} \)
where, e=1.6 x 10^-19 C
\(\varepsilon_{0}\) = Permittivity of free space = 8.85 x 10^-12 N^-1 C²m²
h = Planck’s constant = 6.63 x 10^-34 Js
∴ v₁ = \(\frac{\left(1.6 \times 10^{-19}\right)^{2}}{2 \times 8.85 \times 10^{-12} \times 6.63 \times 10^{-34}}\)
= 0.0218 x 10⁸ =2.18 x 10⁶ m/s

For level n₂ =2, we can write the relation for the corresponding orbital speed as
v₂ = \(\frac{e^{2}}{n_{2} 2 \varepsilon_{0} h}\) = \(\frac{\left(1.6 \times 10^{-19}\right)^{2}}{2 \times 2 \times 8.85 \times 10^{-12} \times 6.63 \times 10^{-34}}\) = 1.09 x 10⁶ m/s
And, for n₃ =3, we can write the relation for the corresponding orbital speed as


Hence, the speed of the electron in a hydrogen atom in n = 1, n = 2 and n = 3 is 2.18 x 10⁶ m/s,
1.09 x 10⁶ m/s, 7.27 x 10⁵ m/s respectively.

(b) Orbital period of electron is given by
T = \(\frac{2 \pi r}{v}\)
Radius of nth orbit
r_n = \(\frac{n^{2} h^{2}}{4 \pi^{2} K m e^{2}}\)
∴ r₁ = \(\frac{(1)^{2} \times\left(6.63 \times 10^{-34}\right)^{2}}{4 \times 9.87 \times\left(9 \times 10^{9}\right) \times 9 \times 10^{-31} \times\left(1.6 \times 10^{-19}\right)}\)
= 0.53 x 10^-10 m
For n=1, T₁ = \(\frac{2 \pi r_{1}}{v_{1}}\)
= \(\frac{2 \times 3.14 \times 0.53 \times 10^{-10}}{2.19 \times 10^{6}}\) = 1.52 x 10^-16s

For n = 2, radius r_n = n²r₁
∴ r₂ =’2².r₁ =4 x0.53 x 10^-10
and velocity v_n, = \(\frac{v_{1}}{n}\)
∴ v₂ = \(\frac{v_{1}}{2}=\frac{2.19 \times 10^{6}}{2}\)
Time period T₂ = \(\frac{2 \times 3.14 \times 4 \times 0.53 \times 10^{-10} \times 2}{2.19 \times 10^{6}}\)
=1216 x 10^-15 s
For n=3,radius r³ =3²,r₁ =9r₁ =9 x 0.53 x 10^-10m and velocity v₃ = \(\frac{v_{1}}{3}=\frac{2.19 \times 10^{6}}{3}\) m/s
Time period T₃ = \(\frac{2 \pi r_{3}}{v_{3}}=\frac{2 \times 3.14 \times 9 \times 0.53 \times 10^{-10} \times 3}{2.19 \times 10^{6}}\) = 4.1 x 10^-15 s

Question 8.
The radius of the innermost electron orbit of a hydrogen atom is 5.3 x 10^-11 m. What are the radii of the n = 2 and n = 3 orbits?
Answer:
The radius of the innermost electron orbit of a hydrogen atom, r₁ = 5.3 x 10^-11 m.
Let r₂ be the radius of the orbit at n = 2.
It is related to the radius of the innermost orbit as r₂ = (n)²r₁ = (2)² x 5.3 x 10^-11
= 4 x 5.3 x 10^-11 = 2.12 x 10^-10m
For n = 3, we can write the corresponding electron radius as
r³ =(n)²r₁ = (3)² x 5.3 x 10^-11
n = 9 x 5.3 x 10^-11 = 4.77 x 10^-10m
Hence, the radii of an electron for n = 2 and n = 3 orbits are 2.12 x 10^-10 m and 4.77 x 10^-10 m respectively.

Question 9.
A 12.5 eV electron beam is used to bombard gaseous hydrogen at room temperature. What series of wavelengths will be emitted?
Answer:
It is given that the energy of the electron beam used to bombard gaseous hydrogen at room temperature is 12.5 eV. Also, the energy of the gaseous hydrogen in its ground state at room temperature is -13.6 eV. When gaseous hydrogen is bombarded with an electron beam, the energy of the gaseous hydrogen becomes -13.6 + 12.5 eV i. e., -1.1 eV.

Orbital energy is related to orbit level (n) as
E = \(\frac{-13.6}{(n)^{2}}\)eV
For n=3, E = \(\frac{-13.6}{(3)^{2}}=\frac{-13.6}{9}\) = -1.5 eV
This energy is approximately equal to the energy of gaseous hydrogen. it can be concluded that the electron has jumped from n I to n = 3 level.

During its de-excitation, the electrons can jump from n = 3 to n = 1 directly, which forms a line of the Lyman series of the hydrogen spectrum.
We have the relation for wave number for Lyman series as
\(\frac{1}{\lambda}=R_{y}\left(\frac{1}{1^{2}}-\frac{1}{n^{2}}\right)\)
where, R_y =Rydberg constant = 1.097 x 10⁷ m^-1,
λ = Wavelength of radiation emitted by the transition of the electron for
n =3,
We can obtain λ as
\(\frac{1}{\lambda}\) = 1.097 x 10⁷\(\left(\frac{1}{1^{2}}-\frac{1}{3^{2}}\right)\)
= 1.097 x 10⁷ \(\left(1-\frac{1}{9}\right)\) = 1.097 x 10⁷x \(\frac{8}{9}\)

λ = \(\frac{9}{8 \times 1.097 \times 10^{7}}\) = 102.55nm
If the electron jumps from n = 2 to n = 1, then the wavelength of the radiation is given as
\(\frac{1}{\lambda}\) = 1.097 x 10⁷ \(\left(\frac{1}{1^{2}}-\frac{1}{2^{2}}\right)\)
= 1.097 x 10⁷\(\left(1-\frac{1}{4}\right)\) = 1.097 x 10⁷x \(\frac{3}{4}\)
λ = \(\frac{4}{1.097 \times 10^{7} \times 3}\) = 121.54 nm

If the transition takes place from n = 3 to n = 2, then the wavelength of the radiation is given as

This radiation corresponds to the Balmer series of the hydrogen spectrum. Hence, in Lyman series, two wavelengths i. e., 102.54 nm, and 121.55 nm are emitted. And in the Balmer series, one wavelength i. e., 656.33 nm is emitted.

Question 10.
In accordance with the Bohr’s model, find the quantum number that characterizes the earth’s revolution around the sun in an orbit of radius 1.5 x 10¹¹ m with orbital speed 3 x 10⁴ m/s. (Mass of earth = 6.0 x 10²⁴ kg.)
Answer:
Radius of the orbit of the Earth around the Sun, r = 1.5 x 10¹¹ m
Orbital speed of the Earth, v = 3 x 10⁴ m/s
Mass of the Earth, m = 6.0 x 10²⁴ kg
According to Bohr’s model, angular momentum is quantized and given as
mvr = \(\frac{n h}{2 \pi}\)

where, h = Planck’s constant = 6.63 x 10^-34 Js
n = Quantum number
∴ n = \(\frac{m v r 2 \pi}{h}\)
= \(\frac{2 \pi \times 6 \times 10^{24} \times 3 \times 10^{4} \times 1.5 \times 10^{11}}{6.63 \times 10^{-34}} \) = 25.61 x 10⁷³ = 2.6 x 10⁷⁴
Hence, the quanta number that characterizes the Earth’s revolution is 2.6 x 10⁷⁴.

Additional Exercises

Question 11.
Answer the following questions, which help you to understand the difference between Thomson’s model and Rutherford’s model better.
(a) Is the average angle of deflection of α-particles by a thin gold foil predicted by Thomson’s model much less, about the same, or much greater than that predicted by Rutherford’s model?

(b) Is the probability of backward scattering (i. e., scattering of α-particles at angles greater than 90°) predicted by Thomson’s model much less, about the same, or much greater than that predicted by Rutherford’s model?

(c) Keeping other factors fixed, it is found experimentally that for small thickness t, the number of α-particles scattered at moderate angles is proportional to t. What clue does this linear dependence on t provide?

(d) In which model is it completely wrong to ignore multiple scattering for the calculation of average angle of scattering of α-particles by. a thin foil?
Answer:
(a) The average angle of deflection of α-particles by a thin gold foil predicted by Thomson’s model is about the same size as predicted by Rutherford’s model. This is because the average angle was taken in both models.

(b) The probability of scattering of α-particles at angles greater than 90° predicted by Thomson’s model is much less than that predicted by Rutherford’s model. This is because there is no such massive central core called the nucleus in Rutherford’s model.

(c) Scattering is mainly due to single collisions. The chances of a single collision increase linearly with the number of target atoms. Since the number of target atoms increases with an increase in thickness, the collision probability depends linearly on the thickness of the target.

(d) It is wrong to ignore multiple scattering in Thomson’s model for the calculation of average angle of scattering of α-particles by a thin foil. This is because a single collision causes very little deflection in this model. Hence, the observed average scattering angle can be explained only by considering multiple scattering.

Question 12.
The gravitational attraction between electron and proton in a hydrogen atom is weaker than the Coulomb attraction by a factor of about 10^-40. An alternative way of looking at this fact is to estimate the radius of the first Bohr orbit of a hydrogen atom if the electron and proton were bound by gravitational attraction. You will find the answer interesting.
Answer:
Radius of the first Bohr orbit is given by the relation,
r₁ = \(\frac{4 \pi \varepsilon_{0}\left(\frac{h}{2 \pi}\right)^{2}}{m_{e} e^{2}}\) ……………….. (i)
where, ε₀ = Permittivity of free space
h = Planck’s constant = 6.63 x 10^-34 Js
m_e = Mass of an electron = 9.1 x 10^-31 kg
e = Charge of an electron = 1.9x 10^-19C
m_p = Mass of a proton = 1.67 x 10^-27 kg
r = Distance between the electron and the proton Coulomb attraction between an electron and a proton is given as
F_C = \(\frac{e^{2}}{4 \pi \varepsilon_{0} r^{2}} \) ………………………….. (2)

Gravitational force of attraction between an electron and a proton is
F_G = \(\frac{G m_{p} m_{e}}{r^{2}}\) ……………………………………. (3)
where, G = Gravitational constant = 6.67 x 10^-11 N m²/kg²
If the electrostatic (Coulomb) force and the gravitational force between an electron and a proton are equal, then we can write
∴ F_G = F_C
\(\frac{G m_{p} m_{e}}{r^{2}}\) = \(\frac{e^{2}}{4 \pi \varepsilon_{0} r^{2}}\)
\(\frac{e^{2}}{4 \pi \varepsilon_{0} r^{2}}\) = Gm_pm_e …………………………. (4)
Putting the value of equation (4) in equation (1), we get

Question 13.
Obtain an expression for the frequency of radiation emitted when a hydrogen atom de-excites from level n to level (n -1). For large n, show that this frequency equals the classical frequency of revolution of the electron in the orbit.
Answer:
It is given that a hydrogen atom de-excites from an upper level (n) to a lower level (n —1). We have the relation for energy (E₁) of radiation at level n as
E₁ = hv₁ = \(\frac{h m e^{4}}{(4 \pi)^{3} \varepsilon_{0}^{2}\left(\frac{h}{2 \pi}\right)^{3}} \times\left(\frac{1}{n^{2}}\right)\)
where, v₁ = Frequency of radiation at level n
h = Planck’s constant
m = Mass of hydrogen atom
e = Charge on an electron
ε_o = Permittivity of free space

Now, the relation for energy (E₂) of radiation at level (n -1) is given as
E₂ = hv₂ = \(\frac{h m e^{4}}{(4 \pi)^{3} \varepsilon_{0}^{2}\left(\frac{h}{2 \pi}\right)^{3}} \times \frac{1}{(n-1)^{2}}\) ………………………… (2)
where, v₂ = Frequency of radiation at level (n -1)
Energy (E) released as a result of de-excitation
E = E₂ – E₁ hv= E₂ – E ₁ ………………….. (3)
where, v = Frequency of radiation emitted
Putting values from equations (1) and (2) in equation (3), we get

For large n, we can write (2 n -1) ≈ 2 n and (n-1) ≈ n.
V = \(\frac{m e^{4}}{32 \pi^{3} \varepsilon_{0}^{2}\left(\frac{h}{2 \pi}\right)^{3} n^{3}} \)
∵ v = \(\frac{m e^{4}}{32 \pi^{3} \varepsilon_{0}^{2}\left(\frac{h}{2 \pi}\right)^{3} n^{3}}\) ………………….. (4)
Classical relation of frequency of revolution of an electron is given as
V_c = \(\frac{v}{2 \pi r}\) ……………………………….. (5)
where, velocity of the electron in the n^th orbit is given as
v = \(\frac{e^{2}}{4 \pi \varepsilon_{0}\left(\frac{h}{2 \pi}\right) n}\) ……………………………… (5)
And, radius of the n^th orbit is given as
v = \(\frac{e^{2}}{4 \pi \varepsilon_{0}\left(\frac{h}{2 \pi}\right) n}\) ………………………………(6)
Putting the values of equations (6) and (7) in equation (5), we get
V_c = \( \frac{m e^{4}}{32 \pi^{3} \varepsilon_{0}^{2}\left(\frac{h}{2 \pi}\right)^{3} n^{3}}\)
Hence, the frequency of radiation emitted by the hydrogen atom is equal to its classical orbital frequency.

Question 14.
Classically, an electron can be in any orbit around the nucleus of an atom. Then what determines the typical atomic size? Why is an atom not, say, a thousand times bigger than its typical size? The question had greatly puzzled Bohr before he arrived at his famous model of the atom that you have learnt in the text.

To simulate what he might well have done before his discovery, let us play as follows with the basic constants of nature and see if we can get a quantity with the dimensions of length that is roughly equal to the known size of an atom (~10^-10 m).

(a) Construct a quantity with the dimensions of length from the fundamental constants e, me and c. Determine its numerical value.

(b) You will find that the length obtained in (a) is many orders of magnitude smaller than the atomic dimensions. Further, it involves c. But energies of atoms are mostly in non-relativistic domain where c is not expected to play any role. This is what may have suggested Bohr to discard c and look for ‘something else’ to get the right atomic size. Now, the Planck’s constant h had already made its appearance elsewhere. Bohr’s great insight lay in recognizing that h, me, and e will yield the right atomic size.

Construct a quantity with the dimension of length from h, me, and e and confirm that its numerical value has indeed the correct order of magnitude.
Answer:
(a) Charge on an electron, e = 1.6 x 10^-19 C
Mass of an electron, m_e = 9.1 x 10^-31 kg
Speed of light, c = 3 x 10⁸ m/s
Let us take a quantity involving the given quantities as \(\left(\frac{e^{2}}{4 \pi \varepsilon_{0} m_{e} c^{2}}\right)\)
where, ε₀ = Permittivity of free space and, \(\frac{1}{4 \pi \varepsilon_{0}}\) = 9 x 10⁹ Nm²C^-2 .
The numerical value of the taken quantity will be

Hence, the numerical value of the taken quantity is much smaller than the typical size of an atom.

(b) Charge on an electron, e = 1.6 x 10^-19 C
Mass of an electron, me = 9.1 x 10^-31 kg
Planck’s constant, h = 6.63 x 10^-34 Js
Let us take a quantity involving the given quantities as \(\frac{4 \pi \varepsilon_{0}\left(\frac{h}{2 \pi}\right)^{2}}{m_{e} e^{2}}\)
where, ε₀ = Permittivity of free space
and, \(\frac{1}{4 \pi \varepsilon_{0}}\) = 9 x 10⁹Nm²C^-2

The numerical value of the taken quantity will be
\(\frac{1}{4 \pi \varepsilon_{0}} \times \frac{\left(\frac{h}{2 \pi}\right)^{2}}{m_{e} e^{2}}=9 \times 10^{9} \times \frac{\left(\frac{6.63 \times 10^{-34}}{2 \times 3.14}\right)^{2}}{9.1 \times 10^{-31} \times\left(1.6 \times 10^{-19}\right)^{2}} \)
= 0.53 x 10^-10 m
Hence, the value of the quantity taken is of the order of the atomic size.

Question 15.
The total energy of an electron in the first excited state of the hydrogen atom is about -3.4 eV.
(a) What is the kinetic energy of the electron in this state?
(b) What is the potential energy of the electron in this state?
(c) Which of the answers above would change if the choice of the zero of potential energy is changed?
Answer:
(a) Total energy of the electron, E = -3.4 eV ’
Kinetic energy of the electron is equal to the negative of the total energy.
⇒ K = -E
= -(-3.4) = + 3.4 eV
Hence, the kinetic energy of the electron in the given state is + 3.4 eV.

(b) Potential energy (JJ) of the electron is equal to the negative of twice of its kinetic energy.
⇒ U = -2 K
= -2 x 3.4 = -6.8 eV
Hence, the potential energy of the electron in the given state is -6.8 eV.

(c) The potential energy of a system depends on the reference point taken. Here, the potential energy of the reference point is taken as zero. If the reference point is changed, then the value of the potential energy of the system also changes. Since total energy is the sum of kinetic and potential energies, total energy of the system will also change.

Question 16.
If Bohr’s quantization postulate (angular momentum = nh/2π) is a basic law of nature, it should be equally valid for the case of planetary motion also. Why then do we never speak of quantization of orbits of planets around the sun?
Answer:
We never speak of quantization of orbits of planets around the Sun because the angular momentum associated with planetary motion is largely relative to the value of Planck’s constant (h).
The angular momentum of the Earth in its orbit is of the order of 10⁷⁰h. This leads to a very high value of quantum levels n of the order of 10⁷⁰.
For large values of n, successive energies and angular momenta are relatively very small. Hence, the quantum levels for planetary motion are considered continuous.

Question 17.
Obtain the first Bohr’s radius and the ground state energy of a muonic hydrogen atom an atom in which a negatively
charged muon (μ^– ) of mass about 207 m_e orbits around a proton.
Answer:
Muonic hydrogen is the atom in which a negatively charged muon of mass about 207 m_e revolves around a proton.
In Bohr’s atom model, r ∝ \(\frac{1}{m}\)
∵ \(\frac{r_{\text {muon }}}{r_{\text {electron }}}=\frac{m_{e}}{m_{\mu}}=\frac{m_{e}}{207 m_{e}}=\frac{1}{207}\) [ ∵m_μ = 207 m_e]
Here, r_e is radius of orbit of electron in hydrogen atom is 0.53 Å.