Bhagya

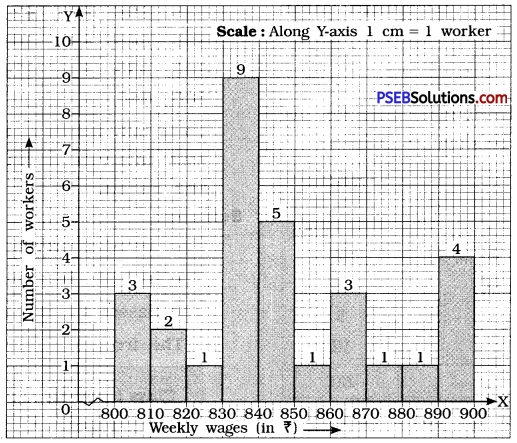
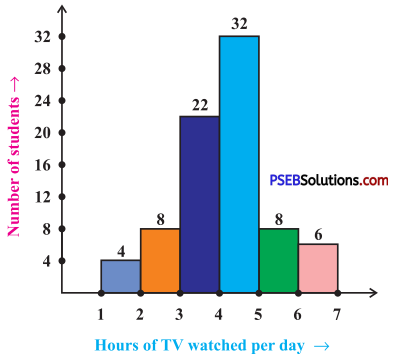
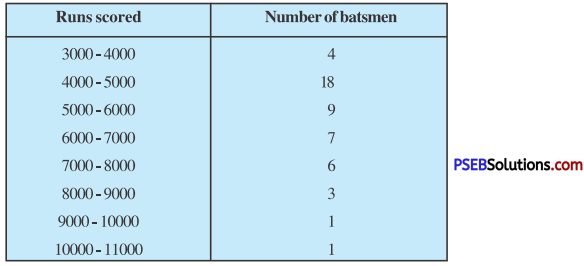
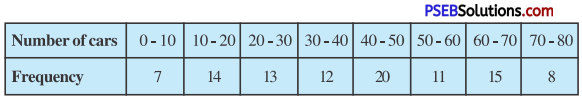
Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 5 Data Handling Ex 5.2 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 8 Maths Chapter 5 Data Handling Ex 5.2

1. A survey was made to find the type of music that a certain group of young people liked in a city. Given pie chart shows the findings of this survey.

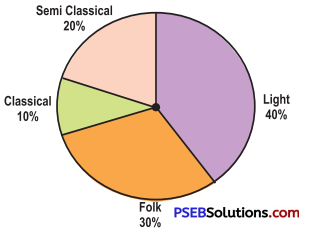
From this pie chart answer the following:

Question (i).
If 20 people liked classical music, how many young people were surveyed ?
Solution:
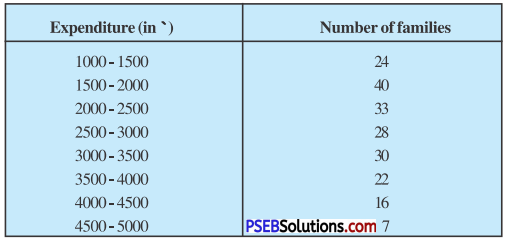
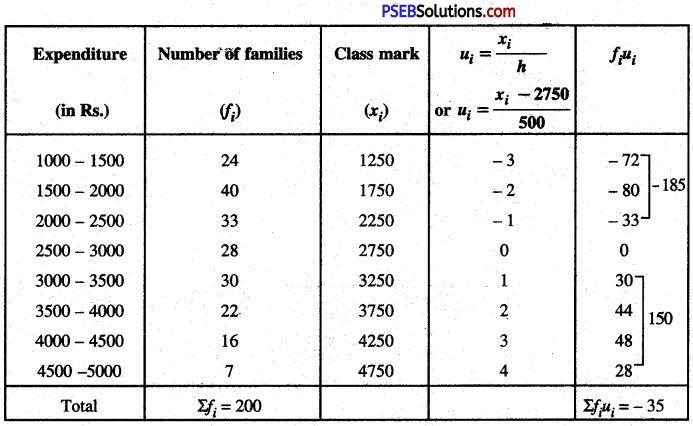
Let the required number of total young people surveyed = x
∴ 10% of x = 20
∴ \(\frac {10}{100}\) × x = 20
∴ x = \(\frac{20 \times 100}{10}\)
∴ x = 200
200 young people were surveyed.

Question (ii).
Which type of music is liked by the maximum number of people ?
Solution:
Maximum number of people like the light music.

Question (iii).
If a cassette company were to make 1000 CD’s, how many of each type would they make ?
Solution:
Total number of CD’s = 1000
(a) Number of CD’s for semi classical music = 20 % of 1000
= \(\frac {20}{100}\) × 1000 = 200
(b) Number of CD’s for classical music = 10 % of 1000
= \(\frac {10}{100}\) × 1000 = 100
(c) Number of CD’s for folk music = 30 % of 1000
= \(\frac {30}{100}\) × 1000 = 300
(d) Number of CD’s for light music = 40 % of 1000
= \(\frac {40}{100}\) × 1000 = 400

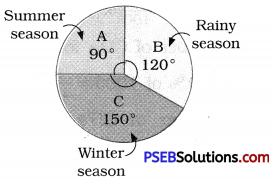
2. A group of 360 people were asked to vote for their favourite season from the ? three seasons rainy, winter and summer:

Question (i).
Which season got the most votes ?
Solution:
Winter season got the most votes (150).

Question (ii).
Find the central angle of each sector.
Solution:
Total Votes = 90 + 120 + 150 = 360
∴ Central angle of the sector corresponding to :
Summer season = \(\frac {90}{360}\) × 360° = 90°
Rainy season = \(\frac {120}{360}\) × 360° = 120°
Winter season = \(\frac {150}{360}\) × 360° = 150°

Question (iii).
Draw a pie chart to show this information.
Solution:
Draw three radi in such a way that it makes angles of 90°, 120° and 150° at the centre.

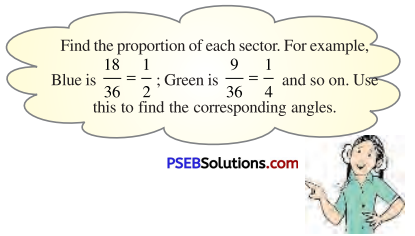
3. Draw a pie chart showing the following information. The table shows the colours preferred by a group of people.

Colours	Number of people
Blue	18
Green	9
Red	6
Yellow	3
Total	36

Solution:
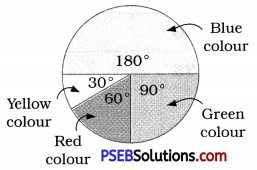
Central angle of the sector corresponding to :
(i) The blue colour = \(\frac {18}{36}\) × 360°
= 18 × 10° = 180°
(ii) The green colour = \(\frac {9}{36}\) × 360° = 90°
(iii) The red colour = \(\frac {6}{36}\) × 360° = 60°
(iv) The yellow colour = \(\frac {3}{36}\) × 360° = 30°
Thus, the required pie chart is given below.

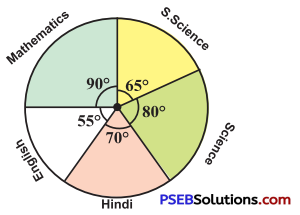
4. The given pie chart gives the marks scored in an examination by a student in Hindi, English, Mathematics, Social Science and Science. If the total marks obtained by the students were 540, answer the following questions.

Question (i).
In which subject did the student score 105 marks ?
[Hint: For 540 marks, the central angle = 360°. So for 105 marks, what is the central angle ?]
Solution:
Toted marks = 540
∴ Central angle corresponding to 540 marks = 360°
∴ Central angle corresponding to 105 marks = \(\frac {360}{540}\) × 105° = 70°
The sector having central angle 70° is corresponding to Hindi.
Thus, the student scored 105 marks in Hindi.

Question (ii).
How many more marks were obtained by the student in Mathematics than in Hindi?
Solution:
The central angle corresponding to the sector of Mathematics = 90°
∴ Marks scored in Mathematics = \(\frac{90^{\circ}}{360^{\circ}}\) × 540 = 135
30 (135 – 105) more marks were obtained by the student in Mathematics than in Hindi.

Question (iii).
Examine whether the sum of the marks obtained in Social Science and Mathematics is more than that in Science and Hindi.
[Hint:Just study the central angles.]
Solution:
The sum of the central angles for Social Science and Mathematics = 65° + 90° = 155°
The sum of the central angles for Science and Hindi = 80° + 70° = 150°
∴ Marks obtained in Social Science and Mathematics is more than the marks scored in Science and Hindi.

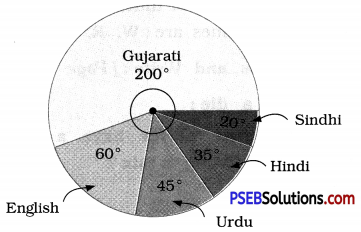
5. The number of students in a hostel, speaking different languages is given below. Display the data in a pie chart:

Solution:
Central angle of the sector representing:
(i) Gujarati language = \(\frac {40}{72}\) × 360°
= 40 × 5° = 200°
(ii) English language = \(\frac {12}{72}\) × 360°
= 12 × 5°= 80°
(iii) Urdu language = \(\frac {9}{72}\) × 360°
= 9 × 5° = 45°
(iv) Hindi language = \(\frac {7}{72}\) × 360°
= 7 × 5° = 35°
(v) Sindhi language = \(\frac {4}{72}\) × 360°
= 4 × 5° = 20°
The required pie chart is as follows.

Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 11 Mensuration Ex 11.2 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 8 Maths Chapter 11 Mensuration Ex 11.2

1. The shape of the top surface of a table is a trapezium. Find its area if its parallel sides are 1 m and 1.2 m and perpendicular distance between them is 0.8 m.

Solution:
Area of top surface of a table = \(\frac {1}{2}\) × (sum of parallel sides) × perpendicular distance between the parallel sides
= \(\frac {1}{2}\) × (1.2 + 1) × 0.8
= \(\frac {1}{2}\) × 2.2 × 0.8
= 0.88 m²
Hence, area of top surface of table is 0.88 m².

2. The area of a trapezium is 34 cm² and the length of one of the parallel sides is 10 cm and its height is 4 cm. Find the length of the other parallel side.
Solution:

Let the length of the other parallel side be xcm.
Area of trapezium
= \(\frac {1}{2}\) × (sum of parallel sides) × height
= \(\frac {1}{2}\) × (10 + x) × 4
= (10 + x) × 2
= 20 + 2x
Area of trapezium = 34 cm² (given)
∴ 20 + 2x = 34
∴ 2x = 34 – 20
∴ 2x = 14
∴ x = 7
Hence, length of the other parallel side is 7 cm.

3. Length of the fence of a trapezium-shaped field ABCD is 120 m. If BC = 48 m, CD = 17 m and AD = 40 m, find the area of this field. Side AB is perpendicular to the parallel sides AD and BC.

Solution:
Perimeter of a field = length of fence of a held
∴ AB + BC + CD + DA = 120
∴ AB + 48 + 17 + 40 = 120
∴ AB + 105 = 120
∴ AB = 120 – 105
∴ AB = 15 m
Now, Area of trapezium ABCD = \(\frac {1}{2}\) × (sum of parallel sides)
× perpendicular distance between the parallel sides
= \(\frac {1}{2}\) × (AD + BC) × AB
= \(\frac {1}{2}\) × (40 + 48) × 15
= \(\frac {1}{2}\) × 88 × 15 = 660 m²
Hence, area of the field is 660 m².

4. The diagonal of a quadrilateral shaped field is 24 m and B the perpendiculars dropped on it from c the remaining opposite vertices are 8 m and 13 m. Find the area of the field.

Solution:
Area of quadrilateral ABCD
Area of Δ ABD + Area of Δ BCD
= \(\frac {1}{2}\) × BD × AM + \(\frac {1}{2}\) × BD x CN
= \(\frac {1}{2}\) × 24 × 13 + \(\frac {1}{2}\) × 24 × 8
= 12 × 13 + 12 × 8
= 156 + 96
= 252 m²
Hence, area of the field is 252 m².

5. The diagonals of a rhombus are 7.5 cm and 12 cm. Find its area.
Solution:
d₁ = 7.5 cm, d₂ = 12 cm
Area of a rhombus = \(\frac {1}{2}\) × d₁ × d₂
= \(\frac {1}{2}\) × 7.5 × 12
= 7.5 × 6 = 45 cm².
Hence, area of rhombus is 45 cm².

6. Find the area of a rhombus whose side is 5 cm and whose altitude is 4.8 cm. If one of its diagonals is 8 cm long, find the length of the other diagonal.
Solution:
Rhombus is a parallelogram too.
∴ Area of rhombus = base × height
= 5 × 4.8
= 5 × \(\frac {48}{10}\)
= 24 cm²
Now, area of rhombus = \(\frac {1}{2}\) × d₁ × d₂
∴ 24 = \(\frac {1}{2}\) × 8 × d₂
∴ 24 = 4 × d₂
∴ d₂ = \(\frac {24}{4}\) = 6 Cm

Hence, length of the other diagonal of rhombus is 6 cm.

7. The floor of a building consists of 3000 tiles which are rhombus-shaped and each of its diagonals are 45 cm and 30 cm in length. Find the total cost of polishing the floor, if the cost per m² is ₹4.
Solution:
The shape of a floor tile is rhombus.
d₁ = 45 cm, d₂ = 30 cm
Area of a tile = \(\frac {1}{2}\) × d₁ × d₂
= \(\frac {1}{2}\) × 45 × 30
= 45 × 15
= 675 cm²
Now, floor of a building consists of total 3000 tiles.
∴ Area of floor = Number of tiles × Area of one tile
= 3000 × 675
= 20,25,000 cm²
1cm = \(\frac {1}{100}\)m
∴ 1cm² = \(\frac{1}{100 \times 100}\)m² = \(\frac {1}{10000}\)m²
∴ 20,25,000 cm² = \(\frac{2025000}{10000}\)m²
= 202.5 m²
∴ Cost of polishing the floor = ₹ 4 per m²
∴ Total cost of polishing the floor
= ₹ 4 × 202.5 = ₹ 810
Hence, total cost of polishing the floor is ₹ 810

8. Mohan wants to buy a trapezium-shaped field. Its side along the river is parallel to and twice the sidealong the road. If the area of this field is 10,500 m² and the perpendicular distance between the two parallel sides is 100 m, And the length of the side along the river.

Solution:
The side along the river is parallel to and twice the side along the road.
Let the length of side along the road be x m.
Then, the length of side along the river = 2xm
Area of trapezium
= \(\frac {1}{2}\) × (sum of parallel sides) × height
= \(\frac {1}{2}\) × (x + 2x) × 100
= \(\frac {1}{2}\) × 3x × 100
= 3x × 50 = 150 x
But, area of field = 10500 m² (given)
∴ 150x = 10500
∴ x = \(\frac {10500}{150}\)
∴ x = 70m
∴ 2x = 2 × 70 = 140 m
Hence, the length of the side along the river is 140 m.

9. Top surface of a raised platform is in the shape of a regular octagon as shown in the figure. Find the area of the octagonal surface.

Solution:
Let us, divide regular octagon into two congruent trapezium and a rectangle. Then add areas of all these three shapes.
Here, height of trapezium = 4 m Length of two parallel sides = 11 m and 5 m respectively.
∴ Area of a trapezium = \(\frac {1}{2}\) × (11 + 5) × 4
= \(\frac {1}{2}\) × 16 × 4
= 8 × 4
= 32 m²
∴ Area of two trapeziums = 2 × 32
= 64m² …….. (i)
Area of a rectangle = length × breadth
= 11 × 5 = 55m² …….. (ii)
∴ Area of regular octagonal platform
= (64 + 55) m² [From (i) and (ii)]
= 119 m²

10. There is a pentagonal-shaped park as shown in the figure. For finding its area Jyoti and Kavita divided it in two different ways.

Find the area of this park using both ways. Can you suggest some other way of finding its area?
Solution:
Jyoti has divided given pentagon into two congruent trapeziums.
∴ Area of a trapezium = \(\frac {1}{2}\) × (sum of parallel sides) × perpendicular distance between two parallel sides
= \(\frac {1}{2}\) × (15 + 30) × \(\frac {15}{2}\)
= \(\frac {1}{2}\) × 45 × \(\frac {15}{2}\)
= \(\frac {675}{4}\) m²
∴ Area of two trapeziums = 2 × \(\frac {675}{4}\)
= \(\frac {675}{2}\)
= 337.5 m²
Now, Kavita has divided given pentagon into one triangle and the other square.
∴ Area of a triangle = \(\frac {1}{2}\) × b × h
= \(\frac {1}{2}\) × 15 × 15
= \(\frac {225}{2}\)
= 112.5m² …………….(i)
∴ Area of a square = (side)²
= (15)² = 15 × 15
= 225 m² ……….(ii)
Area of a pentagon
= (112.5 + 225) m² [From (i) and (ii)]
= 337.5 m²
Now, let us use our idea and find another method to find area of a given pentagon. Here, we have divided given pentagon into three triangles.

Area of ∆ a = \(\frac {1}{2}\) × 15 × 15
= \(\frac {225}{2}\)
= 112.5m² … (i)
Area of ∆ b = \(\frac {1}{2}\) × 15 × 15
= \(\frac {225}{2}\)
= 112.5 m² ………… (ii)
Area of ∆ c = \(\frac {1}{2}\) × 15 × 15
= \(\frac {225}{2}\)
= 112.5 m² ……. (iii)
From (i), (ii) and (iii) the area of a given pentagon
= (i) + (ii) + (iii)
= (112.5 + 112.5 + 112.5) m²
= 337.5 m²
Hence, area of the given pentagon is 337.5 m².
[Note : By using such ideas, i.e., dividing any shape into convienient shapes, you can find area of given figure.]

11. Diagram of the adjacent picture frame has outer dimensions = 24 cm × 28 cm and inner dimensions 16 cm × 20 cm. Find the area of each section of the frame, if the width of each section is same.

Solution:
Here, picture frame is divided into four trapeziums, such that a, b, c and d.
The sides of opposite trapeziums have same measurement so they have equal area.
∴ Area of a = area of c and area of b = area of d

For trapezium a and c:
Length of parallel sides = 24 cm, 16 cm
Height = \(\frac{28-20}{2}=\frac{8}{2}\) = 4 cm
∴ Area of trapezium a
= \(\frac {1}{2}\) × (sum of parallel sides) × perpendicular distance between parallel sides
= \(\frac {1}{2}\) × (24 + 16) × 4
= \(\frac {1}{2}\) × 40 × 4
= 80 cm²
So area of trapezium c = 80 cm².

For trapezium b and d:
Length of parallel sides = 28 cm, 20 cm
Height = \(\frac{24-16}{2}=\frac{8}{2}\) = 4 cm
∴ Area of trapezium b
= \(\frac {1}{2}\) × (sum of parallel sides) × perpendicular distance between parallel sides
= \(\frac {1}{2}\) × (28 + 20) × 4
= \(\frac {1}{2}\) × 48 × 4
= 96 cm²
So area of trapezium d = 96 cm².
Hence, area of each section of frame is as follows.
Area of section a = 80 cm²
Area of section b = 96 cm²
Area of section c = 80 cm²
Area of section d = 96 cm²
To find the total surface area, we find the area of each face and then add them.

Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 5 Data Handling Ex 5.1 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 8 Maths Chapter 5 Data Handling Ex 5.1

Question 1.
For which of these would you use a histogram to show the data?
(a) The number of letters for different areas in a postman’s bag.
(b) The height of competitors in an athletics meet.
(c) The number of cassettes produced by 5 companies.
(d) The number of passengers boarding trains from 7:00 a.m. to 7:00 p.m. at a station.
Give reasons for each.
Solution:
We represent those data by a histogram which can be grouped into class intervals. Obviously, for (b) and (d), the data can be represented by histograms.

Question 2.
The shoppers who come to a departmental store are marked as: man (M), woman (W), boy (B) or girl (G). The following list gives the shoppers who came during the first hour in the morning:
W W W G B W W M G G M M W W W W G B M W B G G M W W M M W W W M W B W G M W W W W G W M M W W M W G W M G W M M B G G W
Make a frequency distribution table using tally marks. Draw a bar graph to illustrate it.
Solution:
The frequency distribution table for the above data can be done as follows :

We can represent the above data by a bar graph as given below:

Question 3.
The weekly wages (in ₹) of 30 workers in a factory are:
830, 835, 890, 810, 835, 836, 869, 845, 898, 890, 820, 860, 832, 833, 855, 845, 804, 808, 812, 840, 885, 835, 835, 836, 878, 840, 868, 890, 806, 840
Using tally marks make a frequency table with intervals as 800-810, 810-820 and so on.
Solution:
The lowest observation = 804
The highest observation = 898
The classes are 800-810, 810-820, etc.
∴ The frequency distribution table is

Question 4.
Draw a histogram for the frequency table made for the data in Question 3, and answer the following questions:
(i) Which group has the maximum number of workers?
(ii) How many workers earn ₹ 850 and more ?
(iii) How many workers earn less than ₹ 850 ?
Solution:
The histogram from the above frequency table is given below.
Here we have represented the class intervals along X-axis and frequencies of the class intervals along the Y-axis.

(i) The group 830 – 840 has the maximum number of workers.
(ii) Number of workers earning ₹ 850 or more = 1 + 3 + 1 + 1 – 1 – 4 = 10
(iii) Number of workers earning less than ₹ 850 = 3 + 2 + 1 + 9 + 5 = 20

Question 5.
The number of hours for which students of a particular class watched television during holidays is shown through the given graph.
Answer the following:
(i ) For how many hours did the maximum number of students watch TV ?
(ii) How many students watched TV for less than 4 hours ?
(iii) How many students spent more than 5 hours in watching TV ?

Solution:
(i) The maximum number of students watched TV for 4 to 5 hours.
(ii) 34 students watched TV for less than 4 hours (4 + 8 + 22 = 34).
(iii) 14 students spent more than 5 hours in watching TV (8 + 6 = 14).

Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 11 Mensuration Ex 11.1 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 8 Maths Chapter 11 Mensuration Ex 11.1

1. A square and a rectangular field with measurements as given in the figure have the same perimeter. Which field has a larger area?

Question (a)

Solution:
Side of a square field = 60 m
∴ Perimeter of a square field = 4 × side
= 4 × 60 = 240 m
Area of a square field = (side)²
= (60)²
= 60 × 60
= 3600m²

Question (b)

Solution:
Perimeter of a rectangular field = Perimeter of square field
∴ Perimeter of a rectangular held = 240
∴ 2 (length + breadth) = 240
∴ 2 (80 + breadth) = 240
∴ 80 + breadth = \(\frac {240}{2}\)
∴ 80 + breadth =120
∴ breadth = 120 – 80
∴ breadth = 40
Breadth of rectangular field = 40 m
∴ Area of rectangular field = length × breadth
= (80 × 40)
= 3200 m²
Area of square field > Area of rectangular field
Thus, area of square field (a) is larger.

2. Mrs. Kaushik has a square plot with the measurement as shown g in the figure. She wants to construct a house in the middle of the plot. A garden is developed around the house. Find the total cost of developing a garden around the house at the rate of ₹ 55 per m².

Solution:
Side of the square plot = 25 m
∴ Area of the square plot = (side)²
= (25 × 25) m²
= 625 m²
In square plot, a rectangular-shaped house is to be constructed.
∴ Area of the constructed house
= length × breadth
= (20 × 15) m²
= 300 m²
∴ Area of the garden = Area of square plot – Area of constructed house
= 625 – 300 = 325 m²
Cost of developing garden of 1 m² is ₹ 55
∴ Cost of developing garden of 325 m²
= ₹ (55 × 325)
= ₹ 17,875
Thus, total cost of developing garden is ₹ 17,875.

3. The shape of a garden is rectangular in the middle and semicircular at the ends as shown in the diagram. Find the area and the perimeter of this garden [Length of rectangle is 20 – (3.5+ 3.5) metres].

Solution:
[Note: Here 2 semicircles at the ends of a rectangular garden makes a whole circle. So first find area of a circle and then area of a rectangle. Sum of these two areas is total area. Follow same pattern to find perimeter too. For perimeter of a garden, take only length as rectangle is between two semicircles. Diameter of a circle = Breadth of a rectangle = 7 m]
For semicircle:
∴ Radius = \(\frac{\text { diameter }}{\text { 2 }}\) = \(\frac {7}{2}\)m
Area of circle = πr²
Area of a semicircle = \(\frac {1}{2}\)πr²
∴ Area of 2 semicircles = 2(\(\frac {1}{2}\)πr²)
= \(\frac{22}{7} \times \frac{7}{2} \times \frac{7}{2}\)m²
= 38.5 m²
Circumference of two semicircles = 2πr
= 2 × \(\frac {22}{7}\) × \(\frac {7}{2}\)
= 22 m

For rectangle:
length = 20 – (3.5 + 3.5) = 20 – 7 = 13 m
breadth = 7 m
Area of the rectangle = length × breadth
= 13 × 7 = 91 m²
Perimeter of the rectangle
= 2 (length × breadth)
= 2 (13 + 0)
= 2 × 13 = 26 m
∴ Total area of the garden = (38.5 + 91) m²
= 129.5 m²
∴ Perimeter of the garden = (22 + 26) m
= 48 m
Thus, area of the garden is 129.5 m² and the perimeter is 48 m.

4. A flooring tile has the shape of a parallelogram whose base is 24 cm and the corresponding height is 10 cm. How many such tiles are required to cover a floor of area 1080 m²? (If required you can split the tiles in whatever way you want to fill up the corners.)
Solution:
[Note : To find number of tiles, divide the area of the floor by area of a tile. Let us do it in a simple way. Unit of floor area and tile area should be same.] Here, tile is parallelogram shaped.
So it’s area = base × corresponding height
Area of a floor = 1080 m²
Base of a tile = 24 cm = \(\frac {24}{100}\) m
Corresponding height of a tile = 10 cm = \(\frac {10}{100}\) m
Number of tiles = \(\frac{\text { Area of a floor }}{\text { Area of a title }}\)
= \(\frac{1080}{\frac{24}{100} \times \frac{10}{100}}\)
= \(\frac{1080 \times 100 \times 100}{24 \times 10}\)
= 45,000
Thus, 45,000 tiles are required to cover the given floor.

5. An ant is moving around a few food pieces of different shapes scattered on the floor. For which food piece would the ant have to take a longer round? Remember, circumference of a circle can be obtained by using the expression c = 2 πr, where r is the radius of the circle.

Question (a)

Solution:
Here, the shape is semi-circular.
Diameter = 2.8 cm
Radius = \(\frac{\text { Diameter }}{2}=\frac{2.8}{2}\) = 1.4 cm
Circumference of a semicircle = πr
Perimeter of the given figure
= πr + diameter
= (\(\frac {22}{7}\) × 1.4) + 2.8
= 4.4 + 2.8
= 7.2 cm

Question (b)

Solution:
Here, given shape is semicircular at one side, (radius = \(\frac {2.8}{2}\) = 1.4 cm)
So perimeter of semicircular region (circumference) = πr
= \(\frac {22}{7}\) × 1.4
= \(\frac {22}{7}\) × \(\frac {14}{10}\)
= 4.4 cm … (i)
Perimeter of the other portion
= breadth + length + breadth
= (1.5 + 2.8 + 1.5) cm
= 5.8 cm … (ii)
∴ Perimeter of the given figure
= (4.4 + 5.8) cm [from (i) and (ii)]
= 10.2 cm

Question (c)

Solution:
Perimeter of a given part
(semi circular circumference) = πr
= \(\frac {22}{7}\) × 1.4
= 4.4 cm
∴ Perimeter of the given figure
= (4.4 + 2 + 2) cm
= 8.4 cm
Thus, 7.2 cm < 8.4 cm < 10.2 cm.
Thus, the ant would has to take a longer round for food piece (b), as it has a larger perimeter.

Punjab State Board PSEB 10th Class Maths Book Solutions Chapter Statistics Ex 14.2 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 10 Maths Chapter 14 Statistics Ex 14.2

Question 1.
The following table shows the ages of the patients admitted in a hospital during a year:

Find the mode and the mean of the data given above. Compare and interpret the two measures of central tendency.

Solution:
For mode:
In the given data, Maximum frequency is 23 and it corresponds to the class interval 35 – 45
Modal class = 35 – 45
So, l = 35; f₁ = 23; f₀ = 21; f₂ = 14 and h = 10
Using fonnula, Mode l = l + \(\left(\frac{f_{1}-f_{0}}{2 f_{1}-f_{0}-f_{2}}\right)\) × h
Mode = 35 + \(\left[\frac{23-21}{2(23)-21-14}\right]\) × 10
= 35 + \(\frac{2}{46-35}\) × 10
= 35 + \(\frac{20}{11}\) = 35 + 1.8 = 36.8.

For Mean:

From above data,
Assumed mean (a) = 30
Width of class (h) = 10
∴ \(\bar{u}=\frac{\Sigma f_{i} u_{i}}{\Sigma f_{i}}=\frac{43}{80}\) = 0.5375
Using formula, Mean \((\overline{\mathrm{X}})=a+h \bar{u}\)
\(\bar{X}\) = 30 + 10 (0.5375)
= 30 + 5.375 = 35.375 = 35.37
Hence, mode of given data is 36.8 years and mean of the given data is 35.37 years. Also, it is clear from above discussion that average age of a patient admitted in the hospital is 35.37 years and maximum number of patients admitted in the hospital are of age 36.8 years.

Question 2.
The following data gives the information on the observed lifetimes (in hours) of 225 electrical components:

Determine the ¡nodal lifetimes of the components.
Solution:
In the given data.
Maximum frequency is 61 and it corresponds to the class interval 60 – 80.
∴ Model class = 60 – 80
So, l = 60; f₁ = 61 ; f₀ = 52; f₂ = 38 and h = 20
Using formula, Mode = l + \(\left(\frac{f_{1}-f_{0}}{2 f_{1}-f_{0}-f_{2}}\right)\) × h

Mode = 60 + \(\left(\frac{61-52}{2(61)-52-38}\right)\) × 20

= 60 + \(\frac{9}{122-52-38}\) × 20

= 60 + \(\frac{9}{32}\) × 20

= 60 + \(\frac{180}{32}\)

= 60 + 5.625 = 65.625
Hence, modal Lifetimes of the components is 65.625 hours.

Question 3.
The following data gives the distribution of total monthly household expenditure of 200 families of a village. Find the modal monthly expenditure of the families. Also, find the mean monthly expenditure:

Solution:
For Mode: In the given data.
Maximum frequency is 40, and it corresponds to the class interval 1500 – 2000.
∴ Model class = 1500 – 2000
So, l = 1500; f₁ = 40; f₀ = 24; f₂ = 33 and h = 500
Using formula, Mode = l + \(\left(\frac{f_{1}-f_{0}}{2 f_{1}-f_{0}-f_{2}}\right)\) × h

= 1500 + \(\left\{\frac{40-24}{2(40)-24-33}\right\}\) × 500

= 1500 + \(\left\{\frac{16}{80-24-33}\right\}\) × 500

= 1500 + \(\frac{16 \times 500}{23}\)

= 1500 + \(\frac{8000}{23}\) = 1500 + 347.83 = 1847.83

For Mean:

From above data,

Assumed Mean (a) = 2750
Length of width (h) = 500
\(\bar{u}=\frac{\Sigma f_{i} u_{i}}{\Sigma f_{i}}=-\frac{35}{200}\) = – 0.175
Using formula, Mean \((\overline{\mathrm{X}})=a+h \bar{u}\)
\(\overline{\mathbf{X}}\) = 2750 + 500 (- 0.175)
= 2750 – 87.50 = 2662.50
Hence, the modal monthly expenditure of family is 1847.83 and the mean monthly expenditure is 2662.50.

Question 4.
The following distribution gives the slate-wise teacher-student ratio in higher secondary schools of India. Find the mode and mean of this data. Interpret the two measures.

Solution:
For Mode:
In the given data,
Maximum frequency is 10 and it corresponds to the class interval is 30 – 35.
∴ Modal class = 30 – 35.
So, l = 30; f₁ = 10; f₀ = 9; f₂ = 3 and h = 5
using formula, Mode = l + \(\left(\frac{f_{1}-f_{0}}{2 f_{1}-f_{0}-f_{2}}\right)\) × h
Mode = 30 + \(\left(\frac{10-9}{2(10)-9-3}\right)\) × 5
= 30 + \(\frac{1}{20-12}\) × 5
= 30 + \(\frac{5}{8}\) = 30 + 0.625 = 30.625 = 30.63 (approx.)

For mean:

From above data, Assumed Mean (a) = 32.5
Width of class (h) = 5
∴ \(\bar{u}=\frac{\Sigma f_{i} u_{i}}{\Sigma f_{i}}=-\frac{23}{35}\) = – 0.65

Using formula, Mean \((\overline{\mathrm{X}})=a+h \bar{u}\)
\(\overline{\mathbf{X}}\) = 32.5 + 5 (- 0.65)
= 32..5 – 3.25 = 29.25 (approx.)
Hence, mode and mean of given data is 30.63 and 29.25. Also, from above discussion, it clear that states/U.T. have student per teacher is 30.63 and on average, this ratio is 29.25.

Question 5.
The given distribution shows the number of runs scored by some top batsmen of the world in one-day international cricket matches

Find the mode of the data.
Solution:
In the given data,
Maximum frequency is 18 and it corresponds to the class interval 4000 – 5000.
∴ Modal class = 4000 – 5000
So, l = 4000; f₁ = 18; f₀ = 4; f₂ = 9 and h = 1000
Using formula, Mode = l + \(\left(\frac{f_{1}-f_{0}}{2 f_{1}-f_{0}-f_{2}}\right)\) × h

Mode = 4000 + \(\left(\frac{18-4}{2(18)-4-9}\right)\) × 1000

= 4000 + \(\frac{14}{36-13}\) × 1000

= 4000 + \(\frac{14000}{23}\) = 4000 + 608.6956

= 4000 + 608.7 = 4608.7 (approx.)
Hence, mode of the given data is 4608.7.

Question 6.
A student noted the number of cars passing through a spot on a road for 100 periods each of 3 minutes and summarised It in the table given below. Find the mode of the data:

Solution:
In the given data,
Maximum frequency is 20 and it corresponds to the class interval 40 – 50
∴ Modal Class = 40 – 50
So, l = 40; f₁ = 20; f₀ = 12; f₂= 11 and h = 10
Using formula, Mode = l + \(\left(\frac{f_{1}-f_{0}}{2 f_{1}-f_{0}-f_{2}}\right)\) × h

Mode = 40 + \(\left(\frac{20-12}{2(20)-12-11}\right)\) × 10

= 40 + \(\frac{8}{40-23}\) × 10

= 40 + \(\frac{80}{17}\) = 40 + 4.70588

= 40 + 4.7 = 44.7 (approx.)
Hence, mode of the given data is 44.7 cars.

Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 12 Exponents and Powers InText Questions and Answers.

PSEB 8th Class Maths Solutions Chapter 12 Exponents and Powers InText Questions

Try These : [Textbook Page No. 194]

1. Find the multiplicative inverse of the following:

Question (i)
2^-4
Solution:
Multiplicative inverse of 2^-4 = 2⁴

Question (ii)
10^-5
Solution:
Multiplicative inverse of 10^-5 = 10⁵

Question (iii)
7^-2
Solution:
Multiplicative inverse of 7^-2 = 7²

Question (iv)
5^-3
Solution:
Multiplicative inverse of 5^-3 = 5³

Question (v)
10^-100
Solution:
Multiplicative inverse of 10^-100 =10¹⁰⁰

Try These : [Textbook Page No. 194]

1. Expand the following numbers using exponents:
(i) 1025.63
(ii) 1256.249
Solution:

Number	Expanded form
(i) 1025.63	(1 × 1000) + (0 × 100) + (2 × 10) + (5 × 1) + (6 × \(\frac {1}{10}\)) + (3 × \(\frac {1}{100}\)) OR (1 × 103) + (2 × 101) + (5 × 100) + (6 × 10-1) + (3 × 10-2)
(ii) 1256.249	(1 × 1000) + (2 × 100) + (5 × 10) + (6 × 1) + (2 × \(\frac {1}{10}\))+ (4 x \(\frac {1}{100}\)) + (9 × \(\frac {1}{1000}\)) OR (1 × 103) + (2 × 102) + (5 × 101) + (6 × 100) + (2 × 10-1) + (4 × 10-2) + (9 × 10-3)

Try These : [Textbook Page No. 195]

1. Simplify and write in exponential form:

Question (i)
(- 2)^-3 × (- 2)^-4
Solution:
= (-2)^-3+(-4)
= (-2)^-3-4
= (-2)^-7 or \(\frac{1}{(-2)^{7}}\)

Question (ii)
p³ × p^-10
Solution:
= p^3+(-10)
= p^3-10
= (p)^-7 or \(\frac{1}{(p)^{7}}\)

Question (iii)
3² × 3^-5 × 3⁶
Solution:
= 3^2+(-5)+6
= 3^2-5+6
= 3^2+6-5
= 3³

Try These : [Textbook Page No. 199]

1. Write the following numbers in standard form:

Question (i)
0.000000564
Solution:
= \(\frac{564}{1000000000}\)
(The decimal point is shifted to nine places to the right.)
= \(\frac{5.64}{10^{9}}\)
= \(\frac{5.64}{10^{7}}\)
= 5.64 × 10^-7
∴ 0.000000564 = 5.64 × 10^-7

Question (ii)
0.0000021
Solution:
\(\frac{21}{10000000}\)
= \(\frac{2.1 \times 10}{10000000}\)
= \(\frac{2.1}{10^{6}}\)
= 2.1 × 10^-6
∴ 0.0000021 = 2.1 × 10^-6

Question (iii)
21600000
Solution:
= 216 × 100000
= 216 × 10⁵
= 2.16 × 10² × 10⁵
= 2.16 × 10⁷
∴ 21600000 = 2.16 × 10⁷

Question (iv)
15240000
Solution:
= 1524 × 10000
= 1.524 × 1000 × 10000
= 1.524 × 10³ × 10⁴
= 1.524 × 10⁷
∴ 15240000 = 1.524 × 10⁷

2. Write all the facts given in the standard form. Observe the following facts: [Textbook Page No. 198 ]

Question 1.
The distance from the Earth to the Sun is 150,600,000,000 m.
Solution:
150,600,000,000 m
= 1.506 × 10¹¹ m

Question 2.
The speed of light is 300,000,000 m/sec.
Solution:
300,000,000 m / sec
= 3 × 10⁸m/sec

Question 3.
Thickness of Class VII Mathematics book is 20 mm.
Solution:
20 = 2 × 10¹ mm

Question 4.
The average diameter of a Red Blood Cell is 0.000007 m.
Solution:
0.000007 = 7 × 10^-6 m

Question 5.
The thickness of human hair is in the range of 0.005 cm to 0.01 cm.
Solution:
0.005 = 5 × 10^-3cm and
0.01 = 1 × 10^-2 cm

Question 6.
The distance of moon from the Earth is 384,467,000 m (approx).
Solution:
384,467,000 = 3.84467 × 10⁸ m

Question 7.
The size of a plant cell is 0.00001275 m.
Solution:
0.00001275 = 1.275 × 10^-5m

Question 8.
Average radius of the Sun is 695000 km.
Solution:
695000 = 6.95 × 10⁵ km

Question 9.
Mass of propellant in a space shuttle solid rocket booster is 503600 kg.
Solution:
503600 = 5.036 × 10⁵ kg

Question 10.
Thickness of a piece of paper is 0.0016 cm.
Solution:
0.0016 = 1.6 × 10^-3 cm

Question 11.
Diameter of a wire on a computer chip is 0.000003 m.
Solution:
0.000003 = 3 × 10^-6 cm

Question 12.
The height of Mount Everest is 8848 m.
Solution:
8848 = 8.848 × 10³ m

Punjab State Board PSEB 6th Class English Book Solutions English Vocabulary Punctuation Exercise Questions and Answers, Notes.

PSEB 6th Class English Vocabulary Punctuation

Punctuation means putting full stops, commas, question marks etc. into a piece of writing. Punctuation helps to clarify the meaning of the sentence or passage.
लिखते समय उचित विराम चिन्हों, अर्थात् full stops (.), commas (,), question marks (?) आदि का प्रयोग करना Punctuation कहलाता है। ये चिन्ह वाक्य अथवा लेख के अर्थ को स्पष्ट करने में सहायता करते हैं।

The important Marks of Punctuation are-

1. Full Stop (.)
2. Comma (,)
3. Question Mark (?)
4. Exclamation Mark (!)
5. Apostrophe (’)
6. Quotation Mark (“ ”)

1. Full Stop is used:
to make the end of an assertive or imperative sentence; as-
1. The children are laughing.
2. Don’t run after the dog.

→ to make abbreviations and initials; as-
Sat. Dec. Ltd. Mr. A. Chandra
M.A. M.L.A. M.P. Mrs. N. Roy

2. Comma is used:
→ to separate words from each other; as-
1. His sister is a tall, lovely mid gentle girl.
2. She has pens, pencils, notebooks and books.
3. He did his homework neatly, quickly and correctly.
Note : A comma is generally not used before and.

→ to separate a reporting verb from the reported speech.
1. She says, “I like my school very much.
2. The saint said, “God is good and gracious.

3. Question Mark is used:
→ after a direct question; as-
1. What is your name ?
2. Have you got a car ?

→ after a tag question; as-
1. She is happy; isn’t she ?
2. He didn’t go to school, did he ?

4. Exclamation Mark is used:
→ after expressions of surprise or strong feeling.
1. How hot it is !
2. What a lovely rose !

→ after an inteijection; as-
1. O !
2. Oh !
3. Alas !
4. Hurray !
5. Pooh !

5. Apostrophe (a raised comma) is used:
→ to show that some letters or numbers have been omitted; as-
1. I’m = I am.
2. hasn’t = has not
3. ’tis = it is
4. don’t = do not

→ to show the possessive form of nouns; as-
1. man’s hat
2. girls’ hostel
3. Principal’s office
4. Mohan’s bag.

6. Quotation Marks are used:
→ to show the actual words of a speaker; as-
1. The teacher said, “Stop writing.”
2. “I can’t help you,” said my friend.

→ to show the titles of songs, poems, books, magazines, etc.
1. “The Blind Beggar” is a beautiful poem.
2. Do you read ‘The Hindustan Times’ daily ?
Note : (i) Quotation Marks are also called Inverted Commas.
(ii) In place of double commas, we can use single commas also.

7. Capital letters are used in the following cases:
1. The first letter of the first word of a sentence.
2. The speech in inverted commas begins with a capital letter.
3. The pronoun ‘P is always written in the capital form.
4. All Proper Nouns begin with a capital letter.
(Manpreet, Punjab, the Gita, the Himalayas, etc.)

Exercises (Solved)

I. Punctuate the following sentences using capital letters where necessary:

1. Shes a good dancer
2. is neeru a good dancer
3. isn’t richa a good dancer
4. madhus sister isnt a good dancer
5. richa said madhu is a good dancer
6. preeti is a good dancer said richas sister
7. what are the children doing there in the street
8. they are pulling the little dogs tail and the dog is crying

Answer:
1. She’s a good dancer.
2. Is Neeru a good dancer ?
3. Isn’t Richa a good dancer ?
4. Madhu’s sister isn’t a good dancer.
5. Richa said,“ Madhu is a good dancer.”
6. ‘‘Preeti is a good dancer,” said Richa’s sister.
7. What are the children doing there in the street ?
8. They are pulling the little dog’s tail, and the dog is crying.

II. Punctuate the following sentences using capital letters where necessary:

1. do you have a pet
2. the ladys purse was stolen
3. mrs Indu jain taught us hindi
4. this is our classroom said tony
5. what a great man gandhiji was
6. reema will sing a song said neha
7. well you may go and play outside
8. j c bose was a famous indian scientist

Answer:
1. Do you have a pet ?
2. The lady’s purse was stolen.
3. Mrs. Indu Jain taught us Hindi.
4. ‘‘This is our classroom,” said Tony.
5. What a great man Gandhiji was !
6. ‘‘Reema will sing a song,” said Neha.
7. Well ! you may go and play outside.
8. J.C. Bose was a famous Indian scientist.

Punjab State Board PSEB 6th Class English Book Solutions English Vocabulary Antonyms Exercise Questions and Answers, Notes.

PSEB 6th Class English Vocabulary Antonyms

An Antonym is a word which is opposite in meaning (विपरीतार्थक) to another word; as-

Word – Antonym
absent – present
active – passive
above – below
accept – reject
before – after
bitter – sweet

blunt – sharp
bold – timid
beautiful – ugly
bright – dim
cheap – costly
clean – dirty
clever – stupid
dark – bright
defeat – victory
difficult – easy
death – life
early – late
empty – full
enemy – friend
far – near
foolish – wise
fresh – stale
good – bad
great – small
happy – sad
high – low
hot – cold
in – out
import – export
increase – decrease
joy – sorrow
Word – Antonym
junior – senior
kind – cruel
lend – borrow
light – heavy
love – hate
long – short
old – young/new
oral – written
night – day
peace – war
poor – rich
profit – loss
right – wrong
shallow – deep
slow – fast
stale – fresh

strong – weak
summer – winter
thick – thin
top – bottom
tall – short
up – down
warm – cool
wide – narrow
wise – silly

Punjab State Board PSEB 6th Class English Book Solutions English Vocabulary Sounds of Animals Exercise Questions and Answers, Notes.

PSEB 6th Class English Vocabulary Sounds of Animals

Animal – Sound
Ass – Brays
Bee – hum
Cat/Kitten – mew
Chicks – cheep
Cows – low, moo

Crows – caw
Cuckoos – cuckoo
Cock – Crow
Deers – bell
Dogs – barks
Dolphins – click
Donkeys – hee-haw
Doves – coo
Ducks – quack
Eagles – scream
Elephants – trumpet, roar
Fly – buzz
Foxes – bark, yelp.
Frogs – croak
Geese – cackle/quack
Grasshoppers – chirp
Goat – baah
Hare – squeak
Horse – neigh
Lions – roar, growl
Monkey – scream, chatter
Owl – hoot
Parrot – talk
Sparrow – chirrup, chirp
Snakes – hiss

Sheep – bleat
Tiger – roar, growl
Tortoise – grunt.

Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 4 Practical Geometry InText Questions and Answers.

PSEB 8th Class Maths Solutions Chapter 4 Practical Geometry InText Questions

Think, Discuss and Write (Textbook Page No. 58)

Question 1.
Arshad has five measurements of a quadrilateral ABCD. These are AB = 5 cm, ∠A = 50°, AC = 4 cm, BD = 5 cm and AD = 6 cm. Can he construct a unique quadrilateral ? Give reasons for your answer.
Solution:
No, the quadrilateral ABCD cannot be constructed with the given combination of measurements. If the length of side BC or DC is given, then only □ ABCD can be constructed.

Think, Discuss and Write (Textbook Page No. 60)

Question (i).
We saw that 5 measurements of a quadrilateral can determine a quadrilateral uniquely. Do you think any five measurements of the quadrilateral can do this ?
Solution:
No, any 5 measurements can’t determine a s quadrilateral. To construct a quadrilateral, specific combination of measurements should be needed such as :

• Four sides and one diagonal
• Four sides and one angle
• Three sides and two diagonals
• Two adjacent sides and three angles
• Three sides and two included angles
• Some special properties should be given,

Question (ii).
Can you draw a parallelogram BATS where BA = 5 cm, AT = 6 cm and AS = 6.5 cm? Why?
Solution:

Here, to draw a parallelogram BATS, BA = 5 cm, AT = 6 cm and AS = 6.5 cm are given. In parallelogram length of opposite sides are equal. So ST = AB = 5 cm and SB = AT = 6 cm. First we can draw A ASB where SB = 6 cm, AB = 5 cm and AS = 6.5 cm. Then draw ΔATS where AT = 6 cm, ST = 5 cm.
Thus, we can draw a parallelogram from given measurements.

Question (iii).
Can you draw a rhombus ZEAL where ZE = 3.5 cm, diagonal EL = 5 cm ? Why?
Solution:

Here, to draw a rhombus ZEAL, ZE = 3.5 cm and EL = 5 cm are given. All of rhombus are equal to each other. So ZE = EA = AL = LZ = 3.5 cm. Moreover, diagonal EL = 5 cm Is given.
So We know all necessary measurements to draw rhombus. Yes, we can draw a rhombus ZEAL.

Question (iv).
A student attempted to draw a quadrilateral PLAY where PL = 3 cm, LA = 4 cm, AY = 4.5 cm, PY = 2 cm and LY = 6 cm, but could not draw it. What is the reason?
[Hint: Discuss it using a rough sketch. ]
Solution :
Here, to draw a quadrilateral PLAY,
PL = 3 cm, LA = 4 cm, AY = 4.5 cm, PY = 2 cm and LY = 6 cm are given.

Now, let us look at measurements of
ΔPLY. PL + PY = 3 cm + 2 cm = 5 cm while YL = 6 cm.
We know that the sum of the lengths of any two sides of triangle is always greater than the length of the third side.
So point P cannot be determined even after constructing ΔLAY. Thus, a student failed s due to this reason.

Think, Discuss and Write (Textbook Page No. 62)

Question 1.
In the above example, can we draw the quadrilateral by drawing ΔABD first and then find the fourth point C ?
Solution:
Since, the measurement of AB is not given, we cannot draw ΔABD, so question does not arise to find the foruth point C.

Thus, we cannot draw the quadrilateral

Question 2.
Can you construct a quadrilateral PQRS with PQ = 3 cm, RS = 3 cm, PS = 7.5 cm, PR = 8 cm and SQ = 4 cm ? Justify your answer.
Solution:
No, the quadrilateral PQRS cannot be constructed as in ΔQSP, SQ + PQ ≯ SP

Think, Discuss and Write (Textbook Page No. 64)

Question 1.
Can you construct the above quadrilateral MIST if we have 100° at M instead of 75° ?
Solution:
Yes. The quadrilateral MIST can be constructed with ∠M = 100° instead of 75°.
[Note : Only size of quadrilateral is changed.]

Question 2.
Can you construct the quadrilateral PLAN if PL = 6 cm, LA = 9.5 cm, ∠P = 75°, ∠L = 150° and ∠A = 140° ?
[Hint: Recall angle sum property.]
Solution:
Here, ∠P + ∠L + ∠A + ∠N
= 75° + 150° + 140° + ∠N
= 365° + ∠N
∴ Construction of quadrilateral PLAN is not possible as according to angle sum property. The sum of all the angles of a quadrilateral is 360°. Here, 365° + N > 360°.

Question 3.
In a parallelogram, the lengths of adjacent sides are known. Do we still need measures of the angles to construct as in the example above?
Solution :
In a parallelogram, the opposite sides are parallel and of equal length. Here, we know the lengths of adjacent sides, so measures of the angles are not needed.
If we know the length of a diagonal the quadrilateral can be drawn.

Think, Discuss and Write (Textbook Page No. 66)

Question 1.
In the above example, we first drew BC. Instead, what could have been be the other starting points?
Solution :
Instead of drawing BC, we can start with \(\overline{\mathrm{AB}}\) or \(\overline{\mathrm{CD}}\).

Question 2.
We used some five measurements to draw quadrilaterals so far. Can there be different sets of five measurements (other than seen so far) to draw a quadrilateral ? The following problems may help you in answering the question.
(i) Quadrilateral ABCD with AB = 5 cm, BC = 5.5 cm, CD = 4 cm, AD = 6 cm and ∠B = 80°.
(ii) Quadrilateral PQRS with PQ = 4.5 cm, ∠P = 70°, ∠Q = 100°, ∠R = 80° and ∠S = 110°.
Construct a few more examples of your own to find sufficiency/insufficiency of the data for construction of a quadrilateral.
Solution:
(i) Here, four sides and one angle are given. So given data is sufficient to construct quadrilateral ABCD.
(ii) We cannot locate the points R and S with the help of given data. So given data is insufficient to construct quadrilateral PQRS.

Few examples of sufficient data to construct quadrilaterals :

• Quadrilateral PQRS in which RS = 6 cm, QR = 5 cm, PQ = 5 cm, ∠Q = 135°, ∠R = 90°. (three sides and two angles)
• Quadrilateral ABCD in which AB = 5 cm, BC = 4 cm, ∠B = 60°, ∠A = 90° and ∠C = 135°. (two sides and three angles)

Try these (Textbook Page No. 67)

Question 1.
How will you construct a rectangle PQRS if you know only the lengths PQ and QR?
Solution:

Each angle of a rectangle is a right angle. Rectangle has opposite sides of equal lengths. Here, PQ is given, So PQ = RS.
ΔPQR can be drawn using PQ, QR and ∠Q = 90°.
ΔQRS can be drawn using QR, RS and ∠R = 90°.
Thus, the required rectangle PQRS can be constructed.

Question 2.
Construct the kite EASY if AY = 8 cm, EY = 4 cm and SY = 6 cm. Which properties of the kite did you use in the process ?
Solution:

Diagonals intersect each other at right angle. ? In kite pairs of consecutive sides are of equal lengths.
While constructing, E cannot be located.
∴ Kite EASY cannot be constructed
(∵ For Δ EYA, the sum of lengths of two sides EY + EA (4 + 4) is not greater than length of third side AY (8).