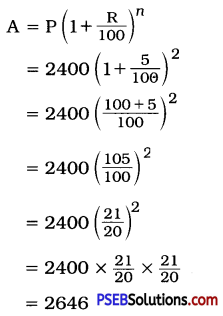Bhagya

Punjab State Board PSEB 11th Class English Book Solutions English Grammar Prepositions Exercise Questions and Answers, Notes.

PSEB 11th Class English Grammar Prepositions

ऐसे शब्द को Preposition कहा जाता है जो स्थान (place), दिशा (direction), साधन (source), विधि (method) आदि का बोध कराने के लिए किसी Noun अथवा Pronoun से पहले लगाया गया हो; जैसे-
In the room; towards the city; through the forest; by all means; into the well; beside me; between us आदि।

The Use Of Some Prepositions

(1) At का प्रयोग छोटे नगरों तथा गांवों के नामों के साथ किया जाता है।
In का प्रयोग बड़े नगरों, प्रान्तों और देशों के नामों के साथ किया जाता है।

• Mohan lives at Kathua.
• She was born at Batote.
• The last National Games were held in Nagpur.
• There are many film studios in Mumbai.

(2) On का प्रयोग तिथियों तथा दिनों के नामों के साथ किया जाता है।
In का प्रयोग महीनों तथा वर्षों के लिए किया जाता है।
At का प्रयोग Point of Time के लिए किया जाता है।

• I shall go to Delhi on Sunday.
• Our examination begins on 24th July.
• Rains started in July.
• His grandfather died in 1995.
• We had tea at 5 o’clock.
• He came at the right time.

(3) In और At के निम्नलिखित समय-सम्बन्धी प्रयोग याद रखिए
In the morning. At noon In the evening At night
In the afternoon At dawn

(4) Between का प्रयोग दो पुरुषों । स्थानों / वस्तुओं आदि के लिए किया जाता है। Among का प्रयोग दो से अधिक पुरुषों / स्थानों / वस्तुओं आदि के लिए किया जाता है।

• The two brothers quarrelled between themselves.
• The three brothers quarrelled among themselves.

(5) In स्थिरता (rest) को प्रकट करता है। Into अन्दर की ओर गति (motion) को प्रकट करता है।

• He was sitting in the room.
• The fox fell into the well.

(6) On स्थिरता (rest) को प्रकट करता है।
Upon ऊपर की ओर गति (motion) को प्रकट करता है।

• The book is on the table.
• The cat jumped upon the table.

(7) With उस यन्त्र (instrument) की ओर संकेत करता है जिसका प्रयोग कोई काम करने के लिए किया गया हो। By काम करने वाले (doer) की ओर संकेत करता है।

• He beat his servant with a stick.
• The book was written by me.

(8) Beside = निकट, असंगत
Besides = के अतिरिक्त

• She came and sat beside me.
• Your answer is beside the mark.
• I have three other pens besides this.

(9) Since तथा for का प्रयोग Perfect Tense के बाद किया जाता है।
From का प्रयोग किसी भी Tense के बाद किया जा सकता है।
Since और from का प्रयोग point of time के लिए किया जाता है।
For का प्रयोग period of time के लिए किया जाता है।
Since का प्रयोग केवल भूतकाल के सम्बन्ध में ही किया जा सकता है।
From और for का प्रयोग किसी भी काल के लिए किया जा सकता है।

• He has been ill since Monday last.
• This timetable has been in force since August.
• He studied English from the age of ten.
• This timetable will come in force from Monday.
• I have been ill for five days.

Words Followed By Appropriate Prepositions

नोट- कई शब्दों के साथ किसी विशेष अर्थ के लिए किसी विशेष Preposition का ही प्रयोग किया जा सकता है। ऐसे शब्द और उनके साथ लगने वाले उपयुक्त Prepositions याद रखने चाहिएं।

1. Abide by (पाबन्द रहना) – We must abide by the rules of the road.
2. Absorbed in (मग्न) – I was absorbed in my work.
3. Abstain from (परहेज़ करना) – We should abstain from drinking.
4. Accede to (मान लेना, स्वीकार कर लेना) – He acceded to my request.
5. Accuse of (दोष लगाना) – He was accused of murder.
6. Act upon (आचरण करना) – He acted upon my advice.
7. Addicted to (बुरी आदत में फँसे होना) – He is addicted to gambling.
8. Admit to (दाखिल करना) – He was admitted to the 9th class.
9. Agree to (स्वीकार करना) – Do you agree to this proposal ?
10. Agree with (सहमत होना) – I agree with you.
11. Alarmed at (चौंक उठना) – We were alarmed at the sight of a lion.
12. Angry with (नाराज़ होना) – He is angry with me.
13. Angry at (नाराज़ होना) – The teacher was angry at your misconduct.
14. Apologize to, for (क्षमा मांगना) – He apologized to me for his rude behaviour.
15. Appeal to, for (प्रार्थना करना) – He appealed to the judge for mercy.
16. Apply to, for (प्रार्थनापत्र देना) – He applied to the headmaster for leave.
17. Approve of (पसन्द करना) – I do not approve of your action.
18. Arrive at (पहुंचना) – He arrived at Jalandhar at six o’clock.
19. Ashamed of (लज्जित होना) – He feels ashamed of his conduct.
20. Assure of (विश्वास दिलाना) – I assure you of my help.
21. Astonished at (हैरान होना) – I was astonished at his failure.
22. Attend on (देखभाल करना) – You should attend on your ailing mother.
23. Attend to (ध्यान देना) – Attend to your lesson.
24. Aware of (सचेत होना) – I am aware of my shortcomings.
25. Believe in (विश्वास करना) – I do not believe in ghosts.
26. Belong to (सम्बन्ध रखना) – He belongs to a rich family.
27. Beware of (सावधान रहना) – Beware of thieves.
28. Bless with (आशीर्वाद देना) – She was blessed with a son.
29. Blind in (अंधा) – He is blind in one eye.
30. Blind to (अपरिचित) – You are blind to your shortcomings.
31. Boast of (शेखी मारना) – Do not boast of your riches.
32. Born in (पैदा होना) – He was born in a poor family.
33. Born of (पैदा होना) – She was born of rich parents.
34. Borrow from (उधार लेना) – I borrowed a book from my friend.
35. Busy with (व्यस्त) – I was busy with my work.
36. Call at (मिलने के लिए जाना) – I called at his house this morning.
37. Call on (बुलाना) – Tomorrow I shall call on you.
38. Call in (बुला कर लाना) – Please call in the doctor at once.
39. Care for (परवाह करना) – He does not care for his studies.
40. Charge with (दोष लगाना) – He was charged with murder.
41. Cling to (चिपटना) – The baby clings to her mother.
42. Collide with (टक्कर खाना) – My cycle collided with a tonga.
43. Compare to (उपमा देना) – We may compare the world to a stage.
44. Compare with (तुलना करना) – Compare Ashoka with Chandragupta.
45. Complain of (शिकायत करना) – He always complains of headache.
46. Complain against, to (शिकायत करना) – I complained against him to the officer.
47. Conscious of (ज्ञान होना) – I am conscious of my shortcomings.
48. Consist of (बना हुआ होना) – The class consists of forty boys.
49. Contented with (सन्तुष्ट होना) – Be contented with your lot.
50. Cure of (स्वस्थ करना) – The doctor cured him of his disease.
51. Deal in (व्यापार करना) – His father deals in cotton.
52. Deal with (व्यवहार करना) – I know how to deal with you.
53. Deprive of (वंचित करना) – He was deprived of his share.
54. Desire for (इच्छा रखना) – I have no desire for name or fame.
55. Die of (मरना) – He died of cholera.
56. Differ from (भिन्न होना) – This pen differs from that.
57. Differ with (सहमत न होना) – I differ with you on this point.
58. Dismiss from (निकाल देना) – He was dismissed from service.
59. Dispose of (बेच देना) – He disposed of his old books.
60. Due to (के कारण) – His success is due to hard work.
61. Eligible for (जो चुना जा सके) — You are not eligible for this post.
62. Engaged in (लगा हुआ) – He is engaged in writing a book.
63. Engage to (सगाई होना) – She is engaged to a doctor.
64. Enquire after (हाल पूछना) – He enquired after my health.
65. Enquire (Inquire) into (पूछताछ करना) – I will enquire into the matter.
66. Excel in (श्रेष्ठ होना) – He excels me in speaking English.
67. Familiar to (परिचित) – His face is familiar to me.
68. Familiar with (परिचित) – I am not familiar with him.
69. Famous for (के लिए प्रसिद्ध) – Ludhiana is famous for hosiery goods.
70. Feed on (निर्वाह करना) – Cows feed on grass.
71. Feel for (अनुभव करना) – We should feel for the poor.
72. Fond of (इच्छुक) – Children are fond of sweets.
73. Full of (भरा हुआ) – The glass is full of milk.
74. Glad at (प्रसन्न) – We are glad at your arrival.
75. Good at (योग्य) – He is good at Mathematics.
76. Grateful to (कृतज्ञ) – I shall be grateful to you for this kindness.
77. Grieve at (दुःखी होना) – He was grieved at his failure.
78. Guilty of (अपराधी) – He was found guilty of theft.
79. Honest in (ईमानदारी) – He is honest in his dealings.
80. Hope for (आशा करना) – Let us hope for better result this time.
81. Ignorant of (अज्ञानता में) – He was ignorant of the facts.
82. Import from (आयात करना) – India imports oil from other countries.
83. Indifferent to (लापरवाह होना) – You are indifferent to your health.
84. Inferior to (घटिया) – This pen is inferior to that.
85. Inform of (सूचना देना) – You did not inform me of his arrival.
86. Injurious to (हानिकारक) – Smoking is injurious to health.
87. Insist on (जिद करना) – He insisted on going with me.
88. Interest in (रुचि) – He has no interest in his studies.
89. Interfere with (दखल देना) – Do not interfere with my work.
90. Introduce to (परिचय कराना) – He introduced me to his friend.
91. Invite to (निमन्त्रण देना) – He invited me to dinner.
92. Jealous of (ईर्ष्यालु) – I am not jealous of his good fortune.
93. Junior to (छोटा) – He is junior to me in service.
94. Knock at (खटखटाना) – Who knocked at the door ?
95. Known to (विदित) – He is known to everybody.
96. Lame in (लंगड़ा) – He is lame in one leg.
97. Laugh at (हंसी उड़ाना) – Do not laugh at the poor.
98. Listen to (ध्यान से सुनना) – Listen to what I say.
99. Live on (गुजारा करना) – He lives on milk and fruit.
100. Long for (इच्छा करना) – I long for happy days.
101. Married to (विवाह होना) – Rama was married to Sita.
102. Match for (मुकाबले का) – Pakistan is no match for India.
103. Negligent in (लापरवाह) – He is negligent in his studies.
104. Notorious for (बदनाम) – He is notorious for gambling.
105. Obedient to (आज्ञाकारी होना) – Be obedient to your teachers.
106. Object to (आपत्ति करना) – He objected to my proposal.
107. Occur to (सूझना) – An idea occurred to me.
108. Part from (जुदा होना) – I was sorry to part from my friends.
109. Part with (जुदा होना) – I cannot part with this book.
110. Pity for (दया) – Have pity for the poor.
111. Pity on (दया) – He took pity on me.
112. Pleased with (प्रसन्न होना) – The teacher is pleased with her.
113. Polite to (नम्र) – Be polite to all.
114. Popular with (लोकप्रिय) – This teacher is popular with the students.
115. Preferable to (अच्छा) – Death is preferable to dishonour.
116. Prefer to (से अच्छा समझना) – I prefer death to dishonour.
117. Pray to (प्रार्थना करना) – I pray to God daily.
118. Prepare for (तैयार होना) – Mohan is preparing for the examination.
119. Preside over (सभापति होना) – Who presided over the meeting?
120. Prevent from (रोकना) – He prevented me from going there.
121. Prey to (शिकार होना) – He fell a prey to drugs.
122. Pride in (अभिमान) – She takes pride in her beauty.
123. Proud of (अभिमानी) – She is proud of her beauty.
124. Recover from (मुक्त होना) – He has recovered from illness.
125. Reduce to (घट जाना) – He was reduced to a skeleton.
126. Regard for (मान) – He has no regard for his teachers.
127. Related to (सम्बन्ध होना) – She is related to me.
128. Rely on (भरोसा रखना) – I rely on your support.
129. Remember to (याद करवाना) – Please remember me to your father.
130. Remind of (याद कराना) – He reminded me of my promise.
131. Repent of (पछताना) – He repented of his folly.
132. Responsible to (उत्तरदायी) – We are responsible to God for our actions.
133. Satisfied with (सन्तुष्ट होना) – I am not satisfied with his work.
134. Search for (तलाश करना) – What are you searching for ?
135. Send for (बुला भेजना) – We sent for the doctor.
136. Shock at (दुःखी होना) – I was shocked at the news of his death.
137. Sick of (तंग आना) – He is sick of his son.
138. Similar to (वैसी ही) – This knife is similar to that.
139. Slow at (सुस्त) – He is slow at Arithmetic.
140. Slow of (सुस्त) – He is slow of understanding.
141. Succeed in (सफल होना) – He succeeded in getting a job.
142. Succeed to (उत्तराधिकारी बनना) – Akbar succeeded to the throne after Humayun’s death.
143. Suffer from (पीड़ित होना) – He suffered from malaria.
144. Superior to (बढ़िया) – My cap is superior to yours.
145. Sure of (विश्वासी) – I am sure of my success.
146. Surprised at (हैरान होना) – I am surprised at your behaviour.
147. Sympathise with (सहानुभूति करना) – Always sympathise with the poor.
148. Talk to (बात करना) – I talked to him about his problem.
149. Taste for (दिलचस्पी) – I have no taste for games.
150. Tired of (तंग हो जाना) – I am tired of this type of life.
151. Tired with (थका हुआ) – I am tired with the day’s work.
152. Trust in (भरोसा करना) – Trust in God and do the right.
153. True to (के प्रति सच्चा होना) – I am true to my friends.
154. Useful for (लाभदायक, किसी उद्देश्य के लिए) – Exercise is useful for health.
155. Useful to (लाभदायक, किसी व्यक्ति के लिए) – This book is very useful to me.
156. Warn of (सचेत करना) – He warned me of the danger.
157. Warn against (चौकन्ना करना) – I warned him against false friends.
158. Wish for (इच्छा करना) – I wish for your success.
159. Worthy of (के योग्य) – I shall try to be worthy of your love.
160. Yield to (झुक जाना) – Never yield to injustice.

EXERCISE

I. Fill in the blanks with appropriate prepositions.
1. People should abide …………… the norms of society.
2. Students should abstain …………… politics.
3. He was accused …………… making inflammatory speeches.
4. He was acquitted …………… corruption charges.
5. It is not easy to adapt oneself ……………. an alien culture.
6. Children are getting addicted …………. cartoon programmes.
7. He is determined to adhere ……………. his convictions.
8. His grandfather is afflicted …………… arthritis.
9. He aimed the punch …………… my nose.
10. Hypocrisy is alien ……………. his nature.
Answer:
1. by
2. from
3. of
4. of
5. to
6. to
7. to
8. with
9. at
10. to.

II. 1. She was born …………… cultivated parents.
2. Several people burst ………………. spontaneous tears on hearing about the Columbia disaster.
3. She wastes a lot of her time in brooding …………… her past.
4. He was charged …………… neglecting his duty in the battlefield.
5. It is not easy to find a person one can confide ………..
6. Parents who connive ………….. the faults of their children spoil them.
7. She was convicted …………… making a false statement.
8. These conditions are not conducive ………….. the healthy development of a child.
9. She was deaf ……………. his plea for forgiveness.
10. A totalitarian regime deprives its people ……………. their civil rights.
Answer:
1. to
2. into
3. over
4. with
5. in
6. at
7. of
8. to
9. to
10. of.

Miscellaneous Exercise (Based On The Textbook)

Fill in the blanks with suitable prepositions.

I. 1. Sudha Murthy was surprised to be called …………. the interview.
2. Life was full ……….. fun and joy.
3. I saw an advertisement ……………. the noticeboard.
4. They have cared for higher education in India …………. 1900.
5. I was taken aback ……….. the telegram.
6. Sudha fell ………. love with the beautiful city.
7. There were six people ………. the panel.
8. I was transferred ……….. Mumbai in June 1999.
9. She hobbled ………… the house in spotless white with one hand resting on her waist.
10. She carried several stale chapattis ……….. her for the village dogs.
11. That was a turning point ………….. our friendship.
12. She took ……… feeding sparrows in the courtyard of our city house.
13. She could not help me ……….. my lessons.
14. She lay peacefully …………. bed praying and telling her beads.
15. A peaceful pallor spread ……………. her face.
Answer:
1. for
2. of
3. on
4. since
5. by
6. in
7. on
8. to
9. about
10. with
11. in
12. to
13. with
14. in
15. on.

II. 1. She had done better than most ………… her male peers.
2. Her face was a criss-cross ……….. wrinkles.
3. She used to get up ……….. the morning and get me ready …….. school.
4. He rules ……….. a vast empire.
5. I never bothered ……… learn the prayer.
6. The driver jumped ………. of the car.
7. Studies pass ……….. the character.
8. Many a learned professor took part ………. the conference.
9. Your reply must be exact and ………… the point.
10. Discipline is a restraint ……………… liberty.
11. Discipline should not be imposed ……… physical violence and fear.
12. If you hold a position of authority, you must impose discipline yourself first.
13. You cannot have liberty ………. discipline.
14. We think more …………. liberty than ……… responsibility.
15. History teaches us the need ……………. a disciplined living.
Answer:
1. of
2. of
3. in, for
4. over
5. to
6. out
7. into
8. in
9. to
10. on
11. by
12. on
13. without
14. of, of
15. of.

III. 1. Dr A.P.J. Abdul Kalam has three visions …….. India.
2. Indians have never tried to enforce their way of life ………. others.
3. India must stand up …………. the world.
4. India is first ……….. milk production.
5. We are ………. the top five nations of the world in terms of GDP.
6. Her lips constantly moved ………… an inaudible prayer.
7. Do we realize that self-respect comes ……… self-reliance ?
8. I came here the day ……………. yesterday.
9. Everything in this store is ………….. sale.
10. We expect the government to do everything ………… us.
11. Let’s do what our country needs ………….. us.
12. Our conscience is mortgaged …………. money.
13. Discipline is unavoidable …………… a modern man.
14. India got its first vision ………… Freedom in 1857.
15. The earth is a treasure we hold ………… trust for our descendants.
Answer:
1. for
2. on
3. to
4. in
5. among
6. in
7. with
8. before
9. for
10. for
11. from
12. to
13. for
14. of
15. in.

IV. 1. India has made wonderful success ……….. many fields.
2. Kalam refers to our obsession …………. foreign goods.
3. We are in the habit of finding fault ………… the government.
4. Major Som Nath Sharma was honoured ……………. the Param Vir Chakra.
5. Trust …………… God and do what is right.
6. I wear a ring ………… my finger.
7. We believe …………… freedom to think.
8. A man becomes a gentleman only ……….. overcoming his weakness.
9. Globalisation leads to a better understanding ……….. nations.
10. Man has always been …………. the fear of wars.
11. The United Nations was founded ………….. the name of common people.
12. They have to survive …………. very little income.
13. Half of the human race is living ………. poverty.
14. Internal wars result ………. unfair distribution of power and wealth in a country.
15. Som Nath Sharma’s right hand was ……… plaster.
Answer:
1. in
2. with
3. with
4. with
5. in
6. on
7. in
8. by / after
9. among
10. in
11. in
12. on
13. in
14. from
15. in.

V. 1. Major Thapa and his men inflicted heavy losses …….. the enemy.
2. Abdul Hamid was enrolled ………… the grenadiers Infantry Regiment in 1954.
3. He was badly wounded ………… a highly explosive shell.
4. Vikram Batra soon rose ………… the rank of Captain.
5. He was given the task ……….. recapturing Point 5140.
6. They hurled two grenades ………….. the machine gun post.
7. Captain Vikram alone killed three enemy soldiers ……… close combat.
8. Major Som Nath’s company was sent to Badgam in Kashmir …………… a fighting patrol.
9. Water is the basis ……….. all life.
10. By cutting ………….. our forests, we have disturbed the water cycle.
11. Water bodies add ………… the beauty of nature.
12. Eyes reflect the state ………….. human mind.
13. Many people had fled ……….. Hiroshima.
14. It was built …………. the delta of the river Ota.
15. Absolute silence reigned …………. the whole city.
Answer:
1. on
2. into
3. by
4. to
5. of
6. at
7. in
8. on
9. of
10. down
11. to
12. of
13. from
14. on
15. in.

VI. 1. A new war would leave nothing worthy ……….. mention.
2. Force is not a solution ……….. man’s problems.
3. Too much has been destroyed …………. this war.
4. They were deeply moved ………. seeing those photographs.
5. They were writhing ……….. agony because of the unbearable pain of their burns.
6. Trees went ………. in flames.
7. There was left nothing ………….. burn.
8. Barb continued writing ………….. Malcolm.
9. An emergency operation was performed ………… Malcolm.
10. Malcolm called …………. to Barb in a weak tone.
11. He received from Barb a proposal ………….. marriage.
12. They went …………. a hiking trip to Balu Pass.
13. Malcolm thought there was no time ………… fear.
14. His one knee-cap was torn …………. .
15. What he saw ……………. the mirror made him sick.
Answer:
1. of
2. to
3. in
4. on
5. in
6. up
7. to
8. to
9. on
10. out
11. for
12. on
13. for
14. off
15. in.

Exercises From Grammar Book (Fully Solved)

Exercise 1
Fill in the blanks with suitable prepositions.

1. The show will be over ………. 5 p.m.
2. His interview will be held ……….. Monday, 30th October.
3. Two boys quarrelled ………. the teacher had gone.
4. The new session starts ………. April.
5. They have been living here ……….. 2009.
6. We were at Shimla ……… Monday ………. Friday.
7. The hockey match was played ………. 1 p.m. ……….. 2.20 p.m.
8. They met only ……….. thirty minutes.
9. The train arrives ……….. 11 o’clock sharp.
10. Our shop opens ………. weekdays only.
Answer:
1. by
2. on
3. after
4. in
5. since
6. from, to
7. from, to
8. for
9. at
10. on.

Exercise 2
Fill in the blanks with suitable prepositions.

1. He stays ……….. Karol Bagh.
2. He spent his early life ……….. India.
3. He made his son sit ……… him and his friend on the bike.
4. Manav was sitting ……….. his mother.
5. He distributed the toffees ……….. all the children.
6. He was hiding ……….. a big board.
7. We stood ……….. the Sun Temple for a while to admire its beauty.
8. My cat was resting ……….. the table.
9. A big statue of Mahatma Gandhi has been placed ……… the Gandhi Museum.
10. The papers were scattered ………. the floor.
Answer:
1. at
2. in
3. between
4. beside
5. among
6. behind
7. in front of
8. under
9. in front of
10. on.

Exercise 3
Fill in the blanks with suitable prepositions.

1. We were on our way ……….. school.
2. Winter vacations start ……….. 25th December.
3. The axe fell ……….. the canal.
4. They were running ……….. the beach.
5. Our school is ………. the temple.
6. The wheel went ……….. .
7. He will have to pay ……….. his nose.
8. We must face the challenge ………. courage.
9. You must learn the lesson ………. heart.
10. He threw the packet ……….. the wall.
Answer:
1. to
2. on
3. into
4. along
5. near
6. off
7. through
8. with
9. by
10. over.

Exercise 4
Fill in the blanks with appropriate prepositions.

1. I was surprised ………. the contents of the notice.
2. He sat ……….. the sofa.
3. She was looking forward ………. your proposal.
4. The cat jumped ……….. the table.
5. I fell ……….. love with the picturesque beauty of Darjeeling.
6. Sudha was not afraid ……….. challenges.
7. Gulmohars were scattered all ……….. the fields.
8. The rat ran ……….. its hole.
9. I saw the advertisement ……… the Sunday newspaper.
10. She was transferred out ……….. Mumbai.
Answer:
1. at
2. on
3. to
4. upon
5. in
6. of
7. over
8. into
9. in
10. from.

Exercise 5
Fill in the blanks with appropriate prepositions.

1. We walked ………. the park.
2. He lives ………. India.
3. My grandfather’s portrait hung ……….. the mantelpiece.
4. His cat was sitting ……….. the table.
5. Grandmother’s lips constantly moved ……….. prayers.
6. I was left alone …….. my village.
7. Her face was covered ………. her silver locks.
8. My school was attached ………. the village temple.
9. She used to sit ………. the temple till my school finished.
10. She hobbled ……….. the house with a stick in her hand.
Answer:
1. to / into
2. in
3. over
4. under
5. in
6. at / in
7. by
8. to
9. in
10. about.

Exercise 6
Fill in the blanks with appropriate prepositions.

1. I used to get up early ……….. the morning.
2. A brisk walk is best ……….. the heart.
3. Some books are ……… be tasted, while others are to be read ……… parts.
4. You should not take things ………. granted.
5. Some students were reading books ………. the library.
6. Books serve ………. delight and ornament.
7. He is good ………. studies.
8. We will be free ……….. the end of the day.
9. Francis Bacon wrote an essay ……….. the importance of studies.
Answer:
1. in
2. for
3. to, in
4. for
5. in
6. for
7. at
8. at / by
9. on.

Exercise 7
Fill in the blanks with appropriate prepositions.

1. The children climbed ……….. the wall.
2. We watched the match ……… a big screen.
3. He answered some ……….. the questions only.
4. Wasan was admitted ……….. the class.
5. We must pray ……….. God.
6. I have no interest ………. games.
7. Kalam had three visions ……….. India.
8. I believe ……….. equality.
9. Discipline is important ……….. success.
10. He was worried ……….. the results of the competition.
Answer:
1. up
2. on
3. of
4. to
5. to
6. in
7. for
8. in
9. for
10. about.

Exercise 8
Fill in the blanks with appropriate prepositions.

1. He was moving ……….. the stage.
2. Kalam would not rest ………. he achieved his aim.
3. The students stood ……… the Taj for some time to admire its beauty.
4. We remained seated ………. the entire performance.
5. Self-respect comes ……….. self-reliance.
6. I could see only his head ………. the water.
7. I parked my car exactly ……….. the bridge.
8. I waited for him ……….. 5.00 p.m. and then left for my walk.
9. Cubs were jostling ……….. each other.
10. We should wash our hands ………. the meals.
Answer:
1. towards
2. till
3. in front of
4. through
5. from
6. above
7. under
8. till
9. with
10. before.

Exercise 9
Fill in the blanks with appropriate prepositions.

1. I have been waiting for you ……… 11.00 a.m.
2. He has been visiting our place ………. many weeks now.
3. They have lived here ……….. 2015.
4. You must learn ……….. stand ………. yourself.
5. The meeting was held ……….. 2 p.m. to 5 p.m.
6. He got a deep cut ……….. his right hand.
7. Both the vehicles collided …….. each other.
8. The plane flew ……….. the fields.
9. Children generally quarrel ………. themselves over trifles.
10. India is ………. the top five nations of the world.
Answer:
9. 1. since
2. for
3. since
4. to, for
5. from
6. on
7. against
8. over
9. among
10. among.

Exercise 10
Fill in the blanks with appropriate prepositions.

1. His car knocked ………. the pillar and overturned.
2. The crowd pressed ……….. the gate.
3. Captain Vikram Batra stormed ………. the enemy.
4. Major Som Nath Sharma laid down his life ………. the nation.
5. Indian troops were airlifted ……….. Srinagar.
6. Flying Officer Nirmaljit exposed himself ……….. danger.
7. The aeroplane flew ……….. the clouds.
8. Indian soldiers displayed highest bravery ……….. the battle.
9. Captain Batra led his company ……….. victory.
10. Our soldiers inflicted heavy casualties ………. the enemy.
Answer:
10. 1. against
2. against
3. at
4. for
5. from / to
6. to
7. over / under
8. in
9. to
10. on.

Exercise 11
Fill in the blanks with appropriate prepositions.

1. We can see ……….. clear water.
2. We should leave the natural resources ……….. future generations.
3. Rivers have been flowing ……….. ages.
4. Forests are essential ……….. human life too.
5. Saving our environment is the need ……….. the hour.
6. Many parts of Punjab are on the verge ………. becoming a desert.
7. Many cities were developed ……… the banks of rivers.
8. Man has always longed ……….. the imaginary elixir of life.
9. We must take steps ………. preserve it.
10. People should be educated ……….. the adverse effects of globalization.
Answer:
1. through
2. for
3. for
4. for
5. of
6. of
7. on
8. for
9. to
10. about.

Exercise 12
Fill in the blanks with appropriate prepositions.

1. U.S. forces dropped atomic bombs …….. Hiroshima and Nagasaki.
2. A soldier renders selfless service ………. his nation.
3. Violence is not a solution ……… any problem.
4. Man must learn ………. love.
5. Many animals hunt ………. night.
6. I requested the commander ……….. explain the situation of the patients.
7. I waited ……….. the doctor.
8. ……. attending to the patients, he gave them medicines also.
9. General Fitch took ……….. his glasses after reading the telegram.
10. The nurse was attending ………. the sick people.
Answer:
1. on
2. to
3. to
4. to
5. at
6. to
7. for
8. While
9. off
10. on.

Exercise 13
Fill in the blanks with appropriate prepositions.

1. Malcolm was walking ………. a creek.
2. He saw the grizzly ……… a distance of a few yards.
3. The grizzly pounced ……….. Barb.
4. Malcolm was standing ……… the grizzly and Barb.
5. She kept slipping ……….. the ice.
6. Grizzly grabbed him ………. both hands.
7. She squeezed him ………. her chest.
8. Barb burst ……….. tears.
9. There was no time ……….. fear.
10. Barb did not give ………. on Malcolm.
Answer:
1. along
2. at
3. on
4. between
5. on
6. with
7. against
8. into
9. for
10. up.

Exercise 14
Fill in the blanks with appropriate prepositions.

1. Nobody warned us ………. the dangers of pollution.
2. He never thought I would be ready ……….. the challenge.
3. The new law prohibits us ……….. drinking while driving.
4. Will you wait ………… your turn ?
5. The teacher handed ……….. the answer sheets ……… the students.
6. The Prime Minister was very keen ……… reforms.
7. BMW has recalled many cars because ………. some technical fault.
8. The policeman was walking ………. the street at night.
9. People of India know how ……….. welcome a guest.
10. Swami Vivekananda influenced millions of people ………. the world.
Answer:
1. aganist
2. for
3. from
4. for
5. ovet, to
6. on
7. of
8. on/about
9. to
10. around.

Exercise 15
Fill in the blanks with appropriate prepositions.

1. He was looking ……….. the board.
2. The haze prevented me ……… seeing the train.
3. She complained ……….. headache.
4. I could not agree ………. his proposal.
5. There is no exception ………. the rules of traffic.
6. They fought ……….. the last round.
7. Swami’s father was shocked ………. his behaviour.
8. I insist ………. his speaking the truth.
9. He was sorry ………. the delay.
10. My friend is a professor ………. profession.
Answer:
1. at
2. from
3. of
4. to
5. to
6. till
7. at
8. on
9. for
10. by.

Exercise 16
Fill in the blanks with appropriate prepositions.

1. Children are generally fond ………. laddoos.
2. A drowning man catches ……….. a straw.
3. We are now ………. good terms with each other.
4. He has not come to my house ……….. many days.
5. It is difficult ……….. convince him.
6. He knew that he was ……….. fault.
7. Maharana Pratap fought tooth and nail ……….. the Mughals.
8. Shivaji fought ……… his motherland.
9. He fell a victim ……….. drinking. 10. Her voice is familiar ………. me.
Answer:
1. of
2. at
3. on
4. for
5. to
6. at
7. against
8. for
9. to
10. to.

Exercise 17
Fill in the blanks with appropriate prepositions.

1. The child was clinging ……….. his mother.
2. He wrote a letter ……….. his friend.
3. She closed the door ……….. him.
4. Always be kind ……….. children.
5. I am pleased ……….. her.
6. Write ……….. ink.
7. I don’t care a fig ……….. him.
8. He ran short ……….. money.
9. We haven’t met ………. last week.
10. Her efforts were rewarded ………. success.
Answer:
1. to
2. to
3. upon / after
4. to
5. with
6. in
7. for
8. of
9. since
10. with.

Exercise 18
Fill in the blanks with appropriate prepositions.

1. This book is superior ……….. that.
2. I congratulated her ……….. her success.
3. He will deliver the mail ………. you.
4. The postman delivered the letter ……….. my home.
5. The man was leaning ……….. the wall.
6. I will get ………. the difficulties.
7. I wished him a speedy recovery ……….. illness.
8. She takes pride ………. her beauty.
9. My office is opposite ……….. the post office.
10. I never exempted him ……….. class.
Answer:
1. to
2. on
3. to
4. at
5. against
6. over
7. from
8. in
9. to
10. from.

Exercise 19
Fill in the blanks with appropriate prepositions.

1. She belongs ……….. a poor family.
2. Beware ………. stray dogs.
3. One should never boast ……… one’s knowledge.
4. He appealed ……….. the public for keeping the area clean.
5. My bed is made ……… teak wood.
6. I was not angry ……….. her.
7. Mr. Verma is sure ……….. his success.
8. He was anxious ……….. his son’s safety.
9. He believes ……….. fair dealing.
10. I solved the problem ………. any difficulty.
Answer:
1. to
2. of
3. of
4. to
5. of
6. with
7. of
8. about
9. in
10. without.

Exercise 20
Fill in the blanks with appropriate prepositions.

1. We go to office ……….. our official car.
2. He works in the factory ……… 8.30 a.m. ………. 4.30. p.m.
3. The boy hid himself ………. the tree.
4. He was found sleeping ……….. the class.
5. His mobile fell ……….. the water tank.
6. Sweets were distributed ………. the street children.
7. There is a cinema hall in front ………. his office.
8. The river is flowing ……… the danger mark.
9. Always hope ……… the best.
10. Always be positive ………. life.
Answer:
1. by
2. from, to
3. behind
4. in
5. into
6. among
7. of
8. above
9. for
10. in.

Punjab State Board PSEB 11th Class English Book Solutions English Message Writing Exercise Questions and Answers, Notes.

PSEB 11th Class English Message Writing

A messsage is a piece of information, news, request, etc. sent to somebody in writing, speech, by telephone, etc. Telephone is the quickest means of sending a message. Sometimes it so happens that a message is received on telephone, but the person for whom the message is meant is not at hand. So someone else has to receive it. And if he himself has to go out, he has to leave in writing the message for the person for whom it is meant.

Always Remember :

1. Address need not be given in a written message.
2. Salutation should be very brief (e.g. Dear Uncle).
3. Date and time of penning down the message should be given.
4. Mention Clearly mention the name of the person from whom the message was received.
5. Only important points should be written.
6. The language used should be very clear and comprehensible.
7. Nothing should be added of your own.

A specimen of message writing

Read the following telephonic conversation between Mrs Gupta and Surinder. Mrs Gupta is about to leave home and won’t be able to meet her son, Sanjay. She leaves a message for him. Write this message in your answer sheet in not more than 50 words. Put your message in a box.

The telephone rings and Mrs Gupta lifts the receiver.
Mrs Gupta : Hello ?
Suriner : Hello, could I speak to Sanjay ? This is Surinder.
Mrs Gupta : He’s not at home, dear. He has gone out with one of his friends.
Suriner : Oh, what a pity ! Aunty, we have arranged a cricket match this morning and we want him to play on our side. He’s such a good player. Please tell him to reach Kotla Grounds as soon as he comes. We shall be waiting for him.
Mrs Gupta : I won’t be able to meet him, but I’ll leave your message.
Suriner : Thanks, Aunty. Please don’t forget.
Mrs Gupta : Oh, never. Rest assured.

Examination-Style Examples of Messages

1. Read the following notes from the notepad of Shri M.A. Khan, the Principal of Samson Public School, Srinagar. He asks his assistant to draft a message on his behalf for Mr Q.M. Sagar, the Vice-Principal of the school. Using the information from the notepad, draft the message.

• Request attend urgent meeting
• Meeting in my office – 10 a.m. – today
• Agenda – planning for a two-week excursion to Gangotri
• Duration – 2 weeks – summer vacation
• Inform – class teachers of X, XI & XII : – should attend meeting

Answer:
Message

8 April 20 ……
8.30 a.m.

Mr QM. Sgyar
( Vice-Principal)

You’ are requested to attend an urgent meeting along with the clars teachers of X, XI and XII in my office* at 10 a,.m. today to discuss the agenda regarding planning for a tun-ureeh excursion, to gangotri, during summer vacation, Teachers are reguasted to take their seats by 9.50 a,.m. sharp.

M.A. Khan
(Principal)

2. You are Miss Shahida. You received a telephone call from Mr Vivek who informs you to tell Mr Rashid, your brother, that a cricket match is arranged this morning and his participation is a must. You leave a message for Rashid. Write this message in not more than 50 words. Put your message in a box.
Answer:
Message From Vivek

16 March 20 ……..
7.30 a,.m.
Rashid,

There mas a, callfor you,from Mr Vivek. He monied to tellyou, that a, cricket match has been arranged this morning and your participation, in it is a, must.

Shahida

3. Read the given sample and complete the message suitably:
You wish to inform your mother, who hasn’t come home from duty, that you are going to your friend’s house to prepare a class project with her / him. Inform her about this, requesting her to phone you up as soon as she gets home, and also tell her about your time to return.
Answer:
Message

14 September 20 ……
5.30 p.m.
Mom,

I am gouty to my friend Naseewis house to prepare a, class projest with huso. Please ring me up at my mobile number as soon as yon get homefrom duty. It’ll take me a, couple of hours before I am able to finish the project. However, l hope to be bask, before 8.00 p.m.

Anwar

4. Read the following notes from the notepad of Shri Ramnath, Principal of Secondary School, Ram Nagar. He asks his assistant to draft a message on his behalf for the class representatives and the staff secretary. Using the information from the notepad, draft the message in not more than 50 words. Put the message in a box.

• Growing tendency of not attending the school regularly.
• Sending applications with one or the other excuse.
• Indiscipline created there by
• All the class representatives and the staff secretary to attend the meeting.

Answer:
Message from the Principal
(For all Class Representatives and the Staff Secretary)

18 May 20 …..
11.30 a.m.

A growing tendency of net attending the school regularly has been noticed among me students and some members of the staff Applications for leave are sent wide one excuse or the other. It leads to the creation of indiscipline. To check this tendency, a, meeting will be held in the Principal’s office today in the recess period. All class representatives and the staff secretary should make it a point to attend the meeting.

For Principal
(P.A. to Principal)

5. You are Sonal, who has arrived at the New Delhi railway station to board the Shatabdi Express to Ludhiana. You hear the following announcement. Write a message to be sent to your father through your driver. Use not more than 50 words. Put the message in a box.

This is for the attention of all the passengers of the Shatabdi Express bound for Ludhiana scheduled to arrive at 6.55 a.m. The Shatabdi Express has derailed 20 kilometres from here. Please note that the Howrah Express that leaves the New Delhi railway station at 8.30 a.m, will carry all the passengers to their destination. You are requested not to buy a new ticket. Your ticket for the Shatabdi Express holds valid and at par for the Howrah Express. Additional coaches are being attached to the Howrah Express to accommodate passengers of the Shatabdi Express.
Answer:
Message

15 June 20 ……
6:40 a,m.
Respected Father

I shall be, going by the, Howrah, Express instead of the, Shatabdi Express. When, I resulted, the, New Delhi, railway station, to board, the, Shatabdi Express, it was announced, that the, train had, derailed, 20 kilometres from, here,. Ail the, Shatabdi passengers bound for Ludhiana, are now being accommodated in the Howrah, Express that will leone at 8.30 hrs. Till then, I shall be waiting at the station itself. And I am sending the driver bash.

Your Coving daughter
Sonal

6. Mr Ujwal, the Managing Director of Ceramics India Ltd., asks his P.A. to draft a message for Mr Ramesh, the P.R.O. of his company. Use the following information in the M.D.’s notepad to draft the message. Put the message in a box. Your answer Should be in not more than 50 words.

• Two dignitaries arriving from Delhi on 4th April.
• Accommodation to be booked in a five-star hotel.
• Show them around the factory.
• Book return tickets for the 6th of April.

CERAMICS INDIA LTD.
UJWAL JAIN (MANAGING DIRECTOR)
MESSAGE FOR MR RAMESH (P.R.O.)

27 March 20……..

Two dignitaries are* arriving prom, Delhi an/ 4th April to visit our factory. Please/ arrange/ to booh suitable/ accommodationfor them/ ire a five/-star hotel of ike/ city. Also shour them/ around the/ factory at any time’ convenient to them/. They will be staying here/for two days. Also, booh their return/ tickets for the/ 6th of April.

Nitish
(P.A. to M.D.)

7. Read the following notes from the notepad of Mr Ram Nath, Principal, Secondary School, Ram Nagar. He asks his Office Superintendent to draft a message on his behalf for the Staff Secretary and the class representatives. Using the information from the notepad, draft the message in not more than 50 words. Put the message in a box.

• Growing cases of bunking / missing class without any solid reason.
• Most truants found in parks, cinema halls or wandering on the roads.
• Indiscipline on the rise.
• Strict measures needed.
• All class representatives and the staff secretary to attend the meeting in my office at 10.30 a.m. tomorrow.

Answer:

MESSAGE
FOR ALL CLASS REPRESENTATIVES AND THE STAFF SECRETARY

18 May 20 ……….
11 : 30 am

A growing tendency of bunking / missing classes has been noticed among the students. Most of such truants are seen Cohering in parks, cinema/ halls, etc. It leads to the creation of indiscipline. To check this tendency, a/meeting will beheld in the Principal’s office tomorrour at 1030 am. All class representatives and the staff secretary should make it a point to attend the meeting.

Far Principal
(Office Superintendent)

Exercise From Grammar Book (Fully Solved)

Read the following telephonic conversation and write the message.
Kunaal : Hello, can I speak to Jasmine ?
Saina : Hello, Jasmine is not at home. I am her friend.
Kunaal : Would you please give my message to her ?
Saina : I will. Please tell me what do you want me to tell her ?
Kunaal : Kindly tell her we shall be visiting her office tomorrow at 11.00 for accounts inspection. She should keep her records ready.
Saina : I will convey your message to her.
Answer:
MESSAGE

ZS August 20 ……..
Jasmine,

Mr Kunaal rang up to inform you, that they unit be inciting your office tomorrow out 11.00 a.m. for accounts inspection. You have to keep- your records ready.

Saina.

2. Read the following telephonic conversation and write the message.

Mrs. Gupta : Hello, am I speaking to Seema ?
Maid : Good morning, madam. Memsahib is not at home. I am her maid.
Mrs. Gupta : Will you convey my message to her ?
Maid : Please tell me, madam.
Mrs. Gupta : Please tell her that my son is getting engaged on this Saturday. The marriage ceremony is going to be solemnized at Hotel Taj, Mumbai, on coming Monday at 7.00 p.m. She, along with her family, is invited to attend the ceremony. Her attendance will be highly appreciated.
Maid : I will convey your message.
Mrs. Gupta : Thanks.
Answer:
MESSAGE

14 July 20 ……….
Memsahib,

There was a call from Mrs (Jupta. Her souls engagement is taking place on this Saturday. Marriage ceremony will be solemnuced at Hotel Tag, Mumbai, on the coming Monday at 7.00 p.m. You are inmted to attend true ceremony with family. Your attendance will be highly appreciated.

Maid.

MESSAGE-WRITING TASKS FOR PRACTICE

1. You are Nanny / Namit. In her absence, your sister Reema’s boss makes a Telephone call. He tells you to pass on the following message to Reema which you note down on the notepad.

• Extension of her leave not approved
• Must report to office on Monday morning
• Important contract has to be signed
• Documents have to be ready by lunch-time on Monday.

You have to leave for a party, so you decide to leave the message. Write down the message for Reena in not more than 50 words.

2. Mr Vikram Batra, the Managing Director of Oswal Mills, asks his P.A. to draft a message for Mr Rajan Jain, the RR.O. of his company. Use the following information to draft the message. Put the message in a box. Your answer should be in not more than 50 words.

• Two dignitaries arriving from Mumbai on 14th August.
• Accommodation to be booked in Hayat Regency.
• Show them around the mill.
• Book return tickets for the 16th August.

3. Read the following telephonic conversation between Kusha and Armaan. Armaan will not be able to meet Moksh. He leaves a message for him. Write this message, using not more than 50 words. Put the’ message in a box.

Kusha : Hello ! Hello ! I am Kusha from Chandigarh. Can I speak to Moksh ? I am his sister.
Armaan : Hello ! Kusha, I am Armaan, Moksh’s colleague. Moksh is on leave today. Can I take a message ?
Kusha : Yes, Armaan. I am coming to Delhi tomorrow. Ask him to pick me up at the airport. I have an interview for the post of Scientist at NPL on the day after tomorrow.
Armaan : Which flight are you coming on ?
Kusha : It is the Jetline flight which arrives there at 7:15 in the morning. I am also bringing along that big box which contains his books. I hope it won’t be any trouble for you.
Armaan : Not at all. I will leave a message on his table. Okay, Kusha.
Kusha : Thank you Armaan. Bye.

Punjab State Board PSEB 11th Class English Book Solutions English Advertisement Writing Exercise Questions and Answers, Notes.

PSEB 11th Class English Advertisement Writing

An advertisement is a message carried in one of the various forms of ‘media’ such as newspapers and magazines, radio and television, and the posters and neon signs that we see in the marketplaces. The purpose of most advertisements is to persuade a particular audience or a group of people to buy the product or service offered by the advertiser.

There are, of course, many kinds of advertisements each with a different purpose. Some advertisements are simply informative. The ‘small ads’ columns in the local newspapers, for example, may contain information of items for sale. They include a simple description of the item, but do not normally use the techniques of persuasive advertising. Some advertisements may try to promote a cause or an idea. For example, there are posters carrying messages such as ‘Big Benefits from Small Savings’ or ‘Protect Your Environment’.

The following points should be kept in mind while drafting an advertisement :

1. It shouldn’t be too cluttered.
2. It should be simple and to the point.
3. It should be worded beautifully and tastefully.
4. The name of the advertiser and of the advertised product should be given in bold / capital letters.
5. A business advertisement should never be too lengthy. It should be as brief as possible.
6. The modern reader has neither the time nor the patience to go through lengthy write-ups. Therefore, an advertisement should be short, crisp and pithy.

Examination-Style Examples of Advertisements

(1) You want to sell a typewriter. Write a suitable advertisement to be published in the ‘For Sale’ column of a newspaper.
Answer:
FOR SALE

A Godrej refrigerator, 2005 model, excellent condition. Intending buyers may call at the office of SHARMA ELECTRICALS, Model Town, Ludhiana, between 3 p,m. and 5 p.m. on any working day.

(2) You are Manoj / Abha of 354, Upkar Nagar, Ludhiana. You want to sell the first floor of your newly constructed house. Draft an advertisement in not more than 50 words for publication in ‘The Indian Express’ under their classified column, marked ‘Property for Sale’. Include all relevant details like location, type of accommodation and contact address including telephone number, etc.
Answer:
PROPERTY FOR SALE

The first floor of our newly constructed house in Upkar Nagar is available for sale, as detailed below:
A two-room set with marble flooring and attached bathrooms – a spacious kitchen, a sitting room and a lobby. The balcony overlooks a beautiful public park. Stairs leading to the floor are quite separate, not connected with any other set. For further details, please contact : Manoj Kumar 354, Upkar Nagar, Ludhiana, Phone 5743713.

(3) You are Prashant / Purnima of 202, Laxmi Nagar, Moga. You lost your briefcase while travelling by bus from Moga to Ludhiana. The briefcase contains some valuable documents, including your Board Certificate for All India Secondary School Examination. Draft a notification in not more than 50 words to be published in ‘The Indian Express’ under their column ‘Lost and Found’. Include all necessary details about the lost briefcase to establish your claim.
Answer:
LOST AND FOUND

While travelling by bus from Moga to Ludhiana, I lost my briefcase which contains some valuable documents. It has also my Board Certificate for All India Secondary School Examination. The briefcase is light brown in colour and is of the VIP make. The finder is requested to return it to my address at my cost.

Prashant
202, Laxmi Nagar,
Moga.

(4) You need a stenographer for your office. Write a brief and to-the-point advertisement for the ‘Situations Vacant’ column of a local newspaper.
Answer:
SITUATIONS VACANT

Wanted an experienced stenographer for our Delhi office. Candidate must be a graduate who can handle office correspondence independently. Salary according to qualifications and experience. Apply within 7 days to P.O. Box No. 15020, Amritsar – 110006.

(5) You have recently started a centre for personality development for schoolchildren. Draft an advertisement to be published in a local daily about it, giving all relevant details.
Answer:
NEW AGE CENTRE FOR PERSONALITY DEVELOPMENT

An ideal centre to help boys and girls of all age groups to develop their personality through interactive activities. Special emphasis is given on developing a logical and healthy attitude in the solution of problems. Equal importance is given to the development of body and mind. Duration of course:
6 months. Consolidated fees : Rs 5000/-.

(6) You are Ashok / Ashita of Model Town, Patiala. You have got a foreign assignment and would be going abroad soon. You have a television set and a refrigerator to sell. Draft a suitable advertisement to be published in the classified columns of ‘Hindustan Times’, Delhi.
Answer:
FOR SALE

A Videocon television set and a Kelvinator refrigerator, both of 2013 model, in excellent condition. Owner going abroad shortly. Intending buyers may please contact-

Ashita
545, Model Town, Patiala.

(7) You are the General Manager of Brakes & Brakes India Limited. You want to appoint two sales representatives for your company. Give an advertisement to be published in the classified columns of a local daily.
Answer:
WANTED SALES REPRESENTATIVES

Brakes and Brakes (India) Limited require two young, efficient and hard-working sales representatives to work for the company. Candidates should have an impressive personality and should be able to converse in Hindi and English fluently. Salary and other perks to be commensurate with the candidates abilities. Applications with complete curriculum vitae should reach the General Manager by the 30th of this month.

Brakes and Brakes (India) Ltd
Focal Point, Ludhiana.

(8) Ravi Menon of 54/7, Vasant Kunj, wishes to let out his house. He drafts an advertisement for publication in the ‘To Let’ section of a newspaper, giving the location, type of accommodation, rent expected and contact address. Write the advertisement in not more than 50 words.
Answer:
TO LET

A newly built spacious house in Urban Estate Gokul, is available on rent. It is a three-room set with attached toilet for each room. Provision for car parking also exists. Beautiful lawn in the front and also in the rear of the nouse. Expected rent. Rs 4500/- per month.

Contact : Ravi Menon, 54/7, Vasant Kunj – between 6 p.m. and 9 p.m. – any day of the week.

(9) You are the General Manager of a leading industrial concern. You need a Chartered Accountant for your office. Draft an advertisement in not more than 50 words to be published in ‘Times of India’, New Delhi, under the classified columns.
Answer:
SITUATIONS VACANT

Wanted a Chartered Accountant for our Delhi office. Candidates must have an experience of minimum 5 years in auditing. Salary no bar for deserving candidates. Apply within 7 days to the General Manager, Rogues & Crooks Ltd., Deep Nagar, Ludhiana.

Exercise From Grammar Book (Fully Solved)

(1) Draft an advertisement for a Washing Machine.
Answer:
SAMINA WASHING MACHINE

Get rid of your stress and troublesome hours in a few minutes with Samina’s range of best washing machines. Seamless features as 350-degree Turbo-Jet Technology. One-Key spin, Fuzzy Logic and many others. It will surely take the burden off your shoulders. Available in three models to suit every budget-
→ Fully Automatic Top Load – 10400/-
→ Fully Automatic Front Load – 12490/-
→ Semi Automatic – 8890/-

(2) Draft an advertisement for a Furniture Exhibition.
Answer:
The First Indian International Furniture Exhibition in Delhi
All World Class Furniture On Display
Venue – Pragati Maidan, New Delhi.
Phase I – 18-21 March 20_ _
Phase II – 28-31 March 20_ _
Book Stalls Now

(3) Write an advertisement for the Mysore Silk Saree Centre, offering festival discounts.
Answer:
MYSORE SILK SAREE CENTRE

Spoil yourself with fabulous silk sarees available at Mysore Silk Saree Centre. Sarees of all leading brands available. Reasonable prices. Go east, go west, only we have the best. Special 40% festival discount offer on Dussehra and Diwali. So what are you waiting for ? Visit today.

Address
434, Booth Market, Mysore (Karnataka)
# 0821 – 1234567, 9888123456

(4) Draft an advertisement for a new HD TV Showroom.
Answer:
Navrang Electrovision
Now
Open In Your City Your Long Wait is Over

All HD LED TV Brands – Panasonic, LG, Sony, Toshiba, Videocon, etc. available with us:
All Leading Brands
Under One Roof.
VISIT TODAY
Address
425, Samrala Road, Ludhiana.

(5) Draft an advertisement for ‘The Leather Shop – an all-leather store’.
Answer:
THE LEATHER SHOP
An All-Leather Store in Your City
Real Leather, Not Rexin
Guarantee of Genuineness
Leather Belts, Leather Shoes, Leather Purses and Wallets,
Jackets Also Available Here.
Kindly Visit Soon.
Very Reasonable Prices.

Address
136, Gol Market, Jamalpur, Ludhiana.
Phone Nos. 0161-2670008, 9000007890

(6) Draft an advertisement for admission to various engineering and management courses being offered by an institute of engineering and management.
Answer:
POTHOHAN INSTITUTE OF ENGINEERING AND MANAGEMENT
(Approved by AICTE and Affilliated to Thapar University of Engineering and Management)

REGISTRATION FOR ADMISSION TO ENGINEERING & MANAGEMENT COURSES OPEN NOW

MANAGEMENT COURSES
1. B.B.A (all streams)
2. M.B.A (all streams)
3. PGDBM
4. D.B.M

ENGINEERING COURSES
1. Civil Engineering
2. Electrical Engineering
3. B. Tech., M. Tech.
4. Mechanical Engineering
5. Computer Science & Engineering
6. Electronics & Telecommunication

www.piem.com

(7) Draft an advertisement for high-speed Eco-Bikes – an alternative means of transport.
Answer:
‘Lapero’
India’s First Eco-Bike
Now Launched
Say Bye-Bye to Pollution

Features:

• Korean Tyres
• Alloy Rims
• High-end Battery
• 100 Km Per Charge
• 6-Speed Gear Box
• Digital Display
• Disc Brakes

Price : 55600/-

(8) Draft an advertisement for an electric car.
Answer:
‘Chanta Figore’
India’s First Electric Car
Now Available at Its Exclusive Showrooms

Features:

• Electronic Engine
• Top Speed-135 kmph
• 29.8 kw Electric Motor
• 155 km per charge
• Kerb Weight- 1516 kgs.
• Single-Speed Gearbox

(9) Draft an advertisement for ‘Swachh Bharat Mission in rural areas.
Answer:

(10) Draft an advertisement for a store of affordable Ayurvedic Products.
Answer:
Arihant Ayurveda Store

• All Types of Ayurvedic Products Available
• All Leading Brands Like Patanjali, Baidyanath, Dabur, Deendayal.
• Very affordable rates.
• A Qualified Ayurvedacharya Available for Consultation
• Say ‘Yes’ to Ayurveda and ‘No’ to Harmful Chemicals

Advertisement-Writing Tasks for Practice

1. Write out a paragraph advertisement, for release in a family magazine, to promote a new toothpaste being made by the company you are working for.
2. You want to sell your television set. Draft a suitable advertisement in about 50 words to be published in the ‘For Sale’ column of a newspaper.
3. You want to seek a suitable match for your sister who is an M.A. in English and is working as a college lecturer. Draft a suitable advertisement for the Matrimonial column of a local daily.
4. On transfer to a far-flung area, you want to dispose of some of your household items. Draft a suitable advertisement in about 50 words for insertion in the ‘For Sale’ column of a newspaper.
5. You are an athletics coach just returned from the U.S.A. You require a job. You are XYZ. Your phone number is 6663232, Moga. Write out an advertisement for a newspaper.
6. You have lost your pet dog. Draft a suitable advertisement in about 50 words to be published in the ‘Lost and Found’ column of a newspaper.

Punjab State Board PSEB 11th Class English Book Solutions English Grammar Use of Words as Different Parts of Speech Exercise Questions and Answers, Notes.

PSEB 11th Class English Grammar Use of Words as Different Parts of Speech

Use of the Same Word as Noun, Verb and Adjective (From Textbook)

1. All – (Noun) I lost my all in this business.
– (Adjective) All the boys reached the playground.
2. Above – (Noun) These orders have come from above.
– (Adjective) Read the above passage.
3. Attack – (Noun) We are ready to face any attack.
– (Verb) He attacked his enemy.
4. Arm – (Noun) It is my left arm.
– (Verb) Pakistan has armed herself with dangerous weapons
5. Address – (Noun) I don’t know your present address.
– (Verb) The Principal addressed the students.

6. Answer – (Noun) Give a short answer to this question.
– (Verb) He answered the question correctly.
7. Air – (Noun) Fresh air is useful for health.
– (Verb) The mattress needs to be aired.
– (Adjective) She is an air hostess.
8. Back – (Noun) I have pain in my back.
– (Verb) He backed my proposal.
– (Adjective) He came through the back door.
9. Book – (Noun) I have read this book.
– (Verb) Have you booked your passage to London ?
10. Brave – (Noun) Fortune favours the brave.
– (Verb) We braved all the dangers.
– (Adjective) He is a brave soldier.
11. Bell – (Noun) The peon is ringing the bell.
– (Verb) Who will bell the cat ?
12. Beat – (Noun) I heard the beat of a drum.
– (Verb) Why did you beat my brother ?
13. Conduct – (Noun) His conduct was quite satisfactory.
– (Verb) Who conducted this meeting ?
14. Chance – (Noun) He has no chance of success.
– (Verb) I chanced to meet an old friend at Delhi.
– (Adjective) It was just a chance meeting.
15. Change – (Noun) Change is the law of nature.
– (Verb) He has changed his programme.
16. Care – (Noun) You should take care of your health.
– (Verb) I do not care for what he says.
17. Cost – (Noun) The cost of living is very high these days.
– (Verb) How much did this book cost you ?
– (Adjective) What is the cost price of this machine ?
18. Cure – (Noun) There is no cure for cancer.
– (Verb) This medicine will cure you.
19. Dead – (Noun) It is useless to grieve over the dead.
– (Adjective) I saw a dead man lying on the road.
20. Down – (Noun) I have seen many ups and downs in my life.
– (Verb) He downed his opponent with a sliding trick.
– (Adjective) The down trains are mostly late.
21. Death – (Noun) Death does not spare anyone.
– (Adjective) The arrival of television dealt a death blow to mass cinema audiences.
22. Danger – (Noun) Life here is full of dangers.
– (Adjective) The river was flowing above the danger mark.
23. Dry – (Adjective) The weather is dry today.
– (Verb) This lake dries up in summer.
24. Dirty – (Adjective) Your hands are dirty.
– (Verb) Do not dirty your clothes.
25. Enough – (Noun) We have enough of food with us.
– (Verb) The poor cannot get enough food.
26. End – (Noun) There was no end to our difficulties.
– (Verb) Communal riots must end now.
27. Eye – (Noun) We see with our eyes.
– (Verb) He eyed me with anger.
28. Finish – (Noun) Our army fought to a finish.
– (Verb) Finish your work quickly.
29. Face – (Noun) Wash your face.
– (Verb) He has faced many difficulties in his life.
– (Adjective) Rani uses face cream to look pretty.
30. Fall – (Noun) Pride hath a fall.
– (Verb) Prices will fall if we produce more.
31. Fire – (Noun) The police opened fire on the crowd.
– (Verb) The hunter fired at the tiger.
32. Fine – (Noun) The Principal did not remit his fine.
– (Verb) The latecomers were fined.
– (Adjective) It is very fine today.
33. Fight – (Noun) There was a fight between Ram and Sham.
– (Verb) We should fight against the dowry system.

34. Floor – (Noun) He lives on the second floor of the house.
– (Verb) No one can floor Sham in debate.
35. Ground – (Noun) Our plans fell to the ground.
– (Verb) The teacher grounded his pupils in algebra.
– (Adjective) He lives on the ground floor.
36. Hand – (Noun) Wash your hands.
– (Verb) He has handed over the charge.
37. Honour – (Noun) I have a great honour for my teachers.
– (Verb) Patriots are always honoured.
37. Head – (Noun) Who is the head of your family ?
– (Verb) The procession was headed by the police band.
– (Adjective) Atma Ram is our head clerk.
38. House – (Noun) I want a house to live in.
– (Verb) Our college is housed in a rented building.
– (Adjective) The house owner was a very noble person.
39. Help – (Noun) I thank you for your help.
– (Verb) We should help the poor.
40. Heat – (Noun) Fire gives us heat.
– (Verb) Electricity heats our rooms.
41. Hit – (Noun) It was a fine hit.
– (Verb) He hit upon a plan.
– (Adjective) His uncle is on the hit list of the extremists.
42. Import – (Noun) The report calls for a ban on the import of hazardous waste.
– (Verb) We import many things from America.
43. Idle – (Noun) The idle fail in their life.
– (Verb) Do not idle away your precious time.
– (Adjective) Sohan is an idle boy.
44. Leave – (Noun) I applied to the Principal for leave.
– (Verb) He will leave for Delhi tomorrow.
45. Lead – (Noun) Gandhiji gave us a lead in the struggle for freedom.
– (Verb) Where does this road lead to ?
– (Adjective) It is a lead pencil.
46. Look – (Noun) I am afraid of his angry looks.
– (Verb) Look at the blackboard.
47. Lock – (Noun) He has lost his lock.
– (Verb) Lock the room.
48. Long – (Noun) Tell me the long and short of this matter.
– (Verb) He longs to see his parents.
– (Adjective) He had to cover a long distance.
49. Man – (Noun) We shall fight to the last man.
– (Verb) Who will man the ship ?
50. Mind – (Noun) Please keep it in your mind.
– (Verb) Mind your own business, please.
51. Milk – (Noun) I take milk every morning.
– (Verb) Milk the cow.
– (Adjective) I want some milk powder.
52. Mean – (Noun) I always follow the golden mean.
– (Verb) I mean what I say.
– (Adjective) He is a mean person.
53. Need – (Noun) I am in need of money.
– (Verb) I need your help at this time.
54. Open – (Noun) Always play in the open.
– (Verb) Open the door.
– (Adjective) It is an open space.
55. Offer – (Noun) He jumped at my offer of help.
– (Verb) I offered him a bunch of flowers.
56. Order – (Noun) We must obey the orders of our seniors.
– (Verb) I ordered a bunch of flowers.
– (Adjective) I have lost my order book.
57. Post – (Noun) I am satisfied with my present post.
– (Verb) Post this letter, please.
– (Adjective) His father works in the local post office
58. Place – (Noun) This is a new place for me.
– (Verb) He placed the whole matter before me.
59. Poor – (Noun) The rich should help the poor.
– (Verb) She is a poor woman.
60. Reply – (Noun) He has sent me no reply.
– (Verb) He did not reply my letter.
61. Round – (Noun) Let us have a round of cards.
– (Verb) He rounded his lips in anger.
– (Adjective) The earth is round.
62. Result – (Noun) His result has been declared today.
– (Verb) His carelessness will result in his failure.
– (Adjective) This is my result card.
63. Respect – (Noun) We should pay respect to our elders.
– (Verb) We should respect our teachers.
64. Rule – (Noun) All of us must obey the rule of the road.
– (Verb) He ruled out my suggestion.
65. Right – (Noun) We must fight for our rights.
– (Verb) Every wrong should be righted.
– (Adjective) This is my right hand.
66. Reach – (Noun) It is beyond my reach.
– (Verb) We reached the station in time.
67. Risk – (Noun) He ran the risk of his life.
– (Verb) He risked his life and saved the drowning boy.
68. Ring – (Noun) He will buy a ring for his wife.
– (Verb) The peon rings the bell.
69. Silence – (Noun) There was perfect silence in the classroom.
– (Verb) The teacher silenced the boys.
70. Stand – (Noun) He was criticized for his tough stand on immigration
– (Verb) Will you please stand up ?
71. Second – (Noun) I shall come back in a second.
– (Verb) I will second your proposal.
– (Adjective) February is the second month of the year.

72. Store – (Noun) He has a rich store of knowledge.
– (Verb) He stored huge stocks of sugar.
73. Station – (Noun) We walked to the railway station.
– (Verb) The army has been stationed on the border.
74. Study – (Noun) She was sitting in her study and reading a book.
– (Verb) The officer has studied the file.
75. Score – (Noun) What is the score of the Indian cricket team ?
– (Verb) Virat Kohli scored fifty runs.
76. Smoke – (Noun) Smoke is coming out of the chimney.
– (Verb) I smoke very seldom.
– (Adjective) The enemies used a smoke bomb to hide themselves.
77. Safe – (Noun) We keep our ornaments in a safe.
– (Adjective) We reached home safe and sound.
78. Search – (Noun) I am in search of a good job.
– (Verb) I am searching for my dog.
– (Adjective) The search party has arrived.
79. Sound – (Noun) We heard the sound of his footsteps.
– (Verb) He sounded the warning.
80. Sight – (Noun) It was a beautiful sight.
– (Verb) I sighted a snake in the garden.
81. Tear – (Noun) Mohan shed tears when he failed.
– (Verb) The child tore my exercise book.
82. Turn – (Noun) Now it is my turn.
– (Verb) Turn on the tap, please.
83. Time – (Noun) What is the time by your watch ?
– (Verb) The train is timed to reach here at 4 p.m.
84. Table – (Noun) This is my office table.
– (Verb) He tabled the agenda for the meeting.
85. Use – (Noun) It is no use going there.
– (Verb) – We use firewood in winter.
86. Up – (Noun) He has seen many ups and downs in his life.
– (Verb) – The up trains are running late today.
87. Visit – (Noun) He went on a visit to Germany.
– (Verb) – I will visit Shimla soon.
88. Water – (Noun) We cannot live without water.
– (Verb) – The gardener is watering the plants.
89. Well – (Noun) We have a well in our garden.
– (Verb) – Tears welled up in her eyes.
– (Adjective) – All is well that ends well.
90. Work – (Noun) I have a lot of work to do.
– (Verb) – The farmers are working in the fields.
91. Waste – (Noun) You will have to feel sorry for this waste of time.
– (Verb) – Do not waste your time.
– (Adjective) – War reduced the country to a waste land.

Exercises from Grammar Book (Fully Solved)

Exercise

Use the following words as Nouns and as Verbs and also as Adjectives (if applicable).
I. aim 2. pen 3. attempt 4. break 5. calm 6. deal 7. touch 8. promise 9. rain 10. pause 11. water 12. vote 13. man 14. dance 15. fix 16. gun 17. head 18. eye 19. light 20. rest 21. smell 22. ring 23. fly 24. oil.
Answer:
1. aim – (Noun) Her aim is to become a doctor.
– (Verb) The hunter aimed at the lion.
2. pen – (Noun) My uncle gifted me a Parker pen.
– (Verb) I penned a loving letter to my younger sister.
3. attempt – (Noun) I passed the entrance test in the first attempt.
– (Verb) I attempted all the questions well.

4. break – (Noun) I went to Shimla during our summer break.
– (Verb) It was she who broke the silence.
5. calm – (Noun) A perfect calm prevailed during his speech.
– (Verb) My friend’s presence had a calming effect on me.
6. deal – (Noun) I believe in fair deal with all my friends.
– (Verb) Mr. Verma deals in ready-made garments.
7. touch – (Noun) Midas’s golden touch could turn anything into gold.
– (Verb) I touched her gently on the hand.
8. promise – (Noun) It is always my endeavour to keep my promise.
– (Verb) I promised to repay the loan in a month.
9. rain – (Noun) The rain in winter months is not welcome.
– (Verb) It rained cats and dogs yesterday.
10. pause – (Noun) The pauses during the speech make it more effective.
– (Verb) She paused for a moment before answering.
11. water – (Noun) Water is truly the elixir of life.
– (Verb) I water the plants of my garden daily.
12. vote – (Noun) Each vote is valuable in an election.
– (Verb) People must vote fearlessly in any election.
13. man – (Noun) Man is mortal.
– (Verb) The border posts are manned 24 hours a day.
14. dance – (Noun) Dance is in Madhuri Dixit’s blood.
– (Verb) A peacock steals your heart when dancing.
15. fix – (Noun) I was in a fix what to do.
– (Verb) Can you fix my computer ?
16. gun – (Noun) The gun is every soldiers constant companion.
– (Verb) Our two soldiers were gunned down by the hooligans.
17. head – (Noun) We must always keep our heads high.
– (Verb) We headed deep into the forest.
18. eye – (Noun) We see with our eyes.
– (Verb) I eyed the stranger with suspicion.
19. light – (Noun) The sun gives us light and heat.
– (Verb) Our way was lit by a full moon.
– (Adj.) It gets light at about 5 o’clock.
20. rest – (Noun) We stopped for a well-deserved rest.
– (Verb) We rested for about two hours before setting out agian.
21. smell – (Noun) Everybody likes the smell of flowers.
– (Verb) The rose smells very sweet.
22. ring – (Noun) I gave her a golden ring as a parting gift.
– (Verb) Don’t forget to ring me up after reaching home.

23. fly – (Noun) The flies were buzzing about in the sweets shop.
– (Verb) I am flying to Mumbai tomorrow.
24. oil – (Noun) I bought the engine oil from the nearest petrol.
– (Verb) Oiling the machine regularly increases its life.

Punjab State Board PSEB 12th Class English Book Solutions English Grammar Translation Exercise Questions and Answers, Notes.

PSEB 12th Class English Grammar Translation

Some Common Proverbs

Exercise 1 (Solved)

Translate the following sentences into English:

Answer:
1. Every dog has his day.
2. He is completely a yes-man.
3. He has his own axe to grind.
4. No new taxes have been imposed upon us.
5. People (or subjects) have revolted against the king.

Exercise 2 (Solved)

Translate the following sentences into English:

Answer:
1. His poverty is an open secret.
2. He is at the root of this mischief.
3. My prosperity. is an eye-sore to him.
4. Ram and Sham are sworn enemies.
5. Where there is a will, there is a way.

Exercise 1 (For Practice)

Translate the following sentences into Hindi/Punjabi:
1. He works hard from dawn to dusk.
2. Most of our politicians are very corrupt.
3. Selfishness is the order of the day.
4. You must reduce your flab¹.
5. Spoken English is gaining importance these days.
6. Spoken English does not mean repeating in the parrot-fashion some crammed sentences.
7. Those who speak English fluently get easily noticed by others.
8. He is the only bread-earner² of the family.
9. Shaleenta is a very good Kathak dancer.
10. He is working as an executive³ in a bank.
11. My colleague in my college is a very good singer.
12. I feel pleased to meet you.
13. What is the nature of your job?
Hints:
1. ਸੀਟਾਧਾ, ਮੋਟਾਪਾ 2. ਹੇਜ਼ੀ ਸਾਰੇ ਭਾਗ, ਰੋਜ਼ੀ ਕਮਾਉਣ ਵਾਲਾ, 3. ਜਿਵੇਕ, ਨਿਦੇਸ਼ਕ ।

Note : Some of the sentences are very easy. It should not be difficult for many of you to translate them in simple Hindi and Punjabi. Hereafter we shall give a few more exercises with meanings in Hindi and Punjabi for your convenience.

Exercise 2 (For Practice)

Translate the following sentences into Hindi/Punjabi:
1. Gandhiji is known for his successful efforts¹ to liberate² India.
2. The lady is very mean in money matters³.
3. She did not allow her husband to spend much on himself.
4. This story tells us about the greed of well-to-do⁴ people.
5. Guru Gobind Singh was a great son of India who led India to sublime⁵ heights of glory⁶.
6. Why is this enormous⁷ increase in population taking place ?
7. Drug-addiction⁸ is a major social evil of modern times.
8. Nations become great by self-confidence⁹.
9. Mankind has undoubtedly progressed since medieval¹⁰ times.
10. Vidyasagar was a very generous¹¹ and charitable¹² man.
Hints:

Exercise 3 (For Practice)

Translate the following sentences into Hindi/Punjabi:
1. Everyone knows the events¹ of Buddha’s life.
2. Newton lived to a ripe age² and earned a great renown³.
3. Books are a wonderful blessing⁴.
4. Ishwara Chandra realised that he must go to England in oder to complete his scientific education.
5. Guru Teg Bahadur was the youngest son of Guru Hargobind Sahib.
6. Science is an angel⁵ in peace but a devil⁶ in war.
7. Wars of today are more dreadful⁷ than those of yesterday.
8. He stumbled⁸ at every step.
9. Silence prevailed⁹ all round the hospital.
10. It has been raining continuously¹⁰ for three hours.
11. It has been drizzling¹¹ for an hour.
Hints:

Exercise 4 (For Practice)

Translate the following sentences into Hindi/Punjabi:
1. This story is a typical example¹ of the storywriter’s work as a story.
2. He failed to find a man before whom he could express the feelings of his heart².
3. He wants to share his grief³ with somebody.
4. This story points out⁴ the truth that poverty dehumanises⁵ a man.
5. I am bereft⁶ of money at this time.
6. I want to know your opinion about etiquette⁷.
7. The teacher punished the students for being noisy in the class.
8. Some shopkeepers fleeces⁸ their customers.
9. A spendthrift lacks balance and perspective⁹.
10. He was reluctant¹⁰ to take the examination.
Hints:

Exercise 5 (For Practice)

Translate the following sentences into Hindi/Punjabi :
1. I have lost the novel¹ that you gave me.
2. She dressed in an elegant² manner.
3. We eat so that we may live.
4. God’s will be done³.
5. Prevention is better than cure⁴.
6. A bird in the hand is worth two in the bush⁵.
7. He is on the wrong side of sixty⁶.
8. We should not look down upon⁷ the poor.
9. I am at a loss to know⁸ what to do.
Hints:

Exercise 6 (For Practice)

Translate the following sentences into Hindi/Punjabi:
1. Trees give shade for the benefit of others.
2. Machines have become the slaves¹ of modern man.
3. A child should be trained to love the reading of books.
4. Politicians worship the rising sun².
5. Our body is like a machine.
6. Newton was a very kind-hearted³ man.
7. He never lost his temper⁴.
8. One should never read anybody’s letter without permission.
9. Children explode crackers on Diwali.
10. The life of modern cities is tiresome⁵.
Hints:

Punjab State Board PSEB 12th Class English Book Solutions English Grammar Use of Non-finites (Infinitives, Gerunds and Participles) Exercise Questions and Answers, Notes.

PSEB 12th Class English Grammar Use of Non-finites (Infinitives, Gerunds and Participles)

We divide verbs into two types. One of the types of verb is the finite verb. A verb that changes its form according to person, tense, mood is called a Finite Verb.

Read the examples given below:
Neeru runs fast.
They run fast.
She is running fast.
We have run fast.

The verbs used in the above example are finite verbs because they change according to person, number and tense.

Now have a look at the sentences given below:

1. She wants to be prosperous.
2. They wanted to be famous. .
3. You wanted to be rich and famous.
4. She made me realise my mistake.

The verbs be and realise in the above sentences do not change according to tense, number and person. Such verbs are called non-finite verbs.

Now read the following sentences:
Rashmi eats to live.
Reshma eats to live.
Rajan eats to live.
A girl eats to live.
These girls eat to live.
You eat to live.
They eat to live.

The verbs used in the above sentences/examples are finite verbs because they change according to person, number and tense.

In the above examples containing eat and to live, eat is governed by person, number and tense. So it is finite verb.

In all other sentences to live is not governed by person and tense. That is an infinite verb.

Three kinds of verbs are there in the infinite verb.

The Infinitive

1. In simple language infinitive means the use of to before the verb.
Examples : I want to go. I want to hit you.
I shall watch Rekha dance.
He made me leave the room.

There are some verbs before which we do not use to. These verbs are make, observe, bid, notice, make, feel, need, dare, see, hear.

2. I need to warn you.
Do you need to leave this place just now ?
He dares me to kill the mongoose ?
If need and dare are used as main verb, to has to be used after these verbs.

3. We do not use to before did, may, do, shall, might, can, could.
He could ride a car.
We shall laugh,
I might leave for Jalandhar.

4. There are some verbs which take an infinitive with the verb.
Promise, refuse, wish, want, dare, fail, decide, agree, hope, desire, expect, ready, glad, happy.
Sentences : I promise to help you. He refused to obey me. I wish to see you tonight.

We use infinitive after some adjectives:
Ready, glad, happy, eager, easy, anxious, hard, able etc.
I am ready to leave for Gurdaspur.
I am glad to see my friend.
I am happy to meet you.
I am eager to know the result on my examination.
It is easy to solve this problem.
I am anxious to see my father.
It is hard to solve this riddle.
He was able to get the job.

Forms of Infinitive

The Infinitive has two forms:

(i) Bare Infinitive
Or
Infinitive without to.
run, fight

(ii) to + Infinitive
to repair
to go

Use of To + Infinitive

We use to + infinitive in the following ways:
1. As a verb
(a) To walk is a good exercise
To smoke is very harmful.

(b) I want to learn French. (Object of the transitive verb want)
I know how to cook pasta. (Object)

(c) The best thing is to help yourself. (Complement to the linking verb is)
Her target was to become an actress. (Complement to the linking verb was)

It is easier to say than to do. (After the dummy subject ‘it’)

2. As an adverb to modify a verb or an adjective:

He went to see the Chairman. (modifies the verb went)
They stood up to protest. (modifies the verb stood)
She is anxious to resign. (modifies the adjective anxious)
He is hard to please. (modifies the adjective hard)

3. As an adjective to qualify a noun:
It is time to depart (qualifies the noun time)
We have nothing to offer. (qualifies nothing)

4. As an object complement or an object to a preposition.
He is about to retire.
We saw him run.
This shop is about to shut.
I felt something fall on my shoulder.

5. As an adjunct :
The captain was the last to abandon the ship.
Kindly give me some water to drink.

6. As part of too + adjective/adverb + infinitive.
He is too weak to walk.
He doesn’t have money enough to buy a flat.

7. As an absolute to modify the whole sentence.
To be candid with you, you are unfit for the job.
To be brief, you have cheated all of us.

Use the Bare Infinitive

8. We do not use to (bare infinitive) before some verbs like watch, bid, see, let, make, help, hear, feel, behold etc.
I shall watch you write an apology.
I heard her sing.
Mummy helped her daughter do her homework.
The Chairman made me wait.
I like you to be with me.
Let me know the truth.

9. With had better, had rather.
You had better stay where you are.
You had rather patch up with your friend.

10. With some prepositions like except, but, than etc.
He does nothing but play.
I would rather give up.
He does nothing except crib.
I would rather die than beg.

11. Passive form of the infinitive (to + be + past participle)
Women like to be flattered by men.
It is an insult to be maltreated like that.

Exercise 1

Fill in the blanks using the verbs within brackets (with or without to):

1. He made me ………… (to laugh)
2. He is too weak ………… (to walk)
3- Raju let me ………… his car. (to drive)
4. He must ………… them from going, (to prevent)
5. You had better ………… (to go)
6. The mango is ………… (eat)
7. You are too young ………… (to understand)
8. There is nothing ………… (to say)
9. We watched them ………… (to work)
10. Let him ………… this work (to do)
Answer:
1. laugh
2. to walk
3. drive
4. prevent
5. go
6. to eat
7. to understand
8. to be said
9. work
10. do.

Exercise 2

Fill up the blanks with infinitive:

1. There is no one ………… this claim, (challenge)
2. He did not let me ………… my work in peace, (do)
3. He was sure ………… a scholarship, (get)
4. The teacher asked the students ………… silence, (maintain)
5. The doctor advised me ………… regular exercise, (to take)
6. They did nothing but ………… (to think)
7. You had rather ………… there, (to go)
8. Everyone wants ………… peacefully, (to live)
9. My father allowed me ………… (to go)
10. She expects ………… (to succeed)
Answer:
1. to challenge
2. do
3. to get
4. to maintain
5. to take
6. think
7. go
8. to live
9. to go
10. to succeed.

The Gerund

A gerund is that form of the verb which ends in V-ing and has the force of a noun. That is why a gerund is called a verbal noun.

Use of Gerund

1. As a Subject
Running is good for health.
Swimming is useful for reducing weight.
Seeing is believing
Reading makes a man complete.
Writing makes a man perfect.

2. As an object
She likes painting.
Rekha loves dancing.

3. As a complement to a Linking Verb
My first love is singing.
My favourite sport is swimming.

4. As an object of preposition
I am tired of sitting all day long.
I am fond of fishing.
He is addicted to gambling.

5. As part of a Noun Phrase
Watching snowfall is soothing to the eyes.
It is foolish catching fire.

Note : Addicted to, look forward to are followed by gerund -ing.
He is addicted to drinking.
He is looking forward to meeting his friend.

The Participle

A participle is that form of verb which partakes of the nature both of a verb and of an adjective.

Kinds of Participle

Participle is of two kinds:

Use of Participle:

1. As an adjective :
I will buy a talking clock.
This house has no running water.

2. As an object complement:
I found the conditions soothing.
She found the baby weeping.

3. As an adverb :
She went away smiling.
The player left the ground abusing.
The girl stood whistling.

4. As part of an Adjective Phrase:
The lady doctor living on the ground floor is very charming.
The man managing the event is my uncle.

5. As a Participle Phrase :
Placing his hand on the Gita, he swore that he would speak the truth.
Taking her bag, the lady walked out of the Mall.

Difference between a Gerund and a Participle:
Throwing a grenade at the C.R.P.F. picket, the terrorist ran away. (Present Participle)
Throwing stones at other people’s houses is an anti-social act. (Gerund)
Swimming in the fast flowing water, Atul saved a drowning boy. (Present Participle)
Jogging is a good exercise. (Gerund)

Use of Past Participle:

1. As an Adjective:
The injured man was carried to the trauma centre.
He is a gone case.

2. As an Object Complement:
I found the door shut.
The murder of the leader left us shocked.

3. As an Adverb:
He left the gym totally exhausted.
Nervous, he kept on moving to and fro.

4. As part of a Participle Phrase:
I saw a dog crushed under a truck.
The landlady found her house burgled from the front and the back.

Exercise 1

Do as directed:

Question 1.
………… (hear) a noise, I turned round. (Fill up the blank with a participle)
Answer:
Hearing a noise, I turned round.

Question 2.
I saw a storm ………… (approach). (Fill up the blank with a participle)
Answer:
I saw a storm approaching.

Question 3.
(Hunt) deer is not allowed in this area. (Fill up the blank with a gerund)
Answer:
Hunting deer is not allowed in this area.

Question 4.
Children love ………… make mud houses. (Fill up the blank with a gerund)
Answer:
Children love making mud houses.

Question 5.
………… (toil) is the lot of mankind. (Fill up the blank with an infinitive)
Answer:
To toil or toiling is the lot of mankind.

Exercise 2

Do as directed:

Question 1.
Combine the following sentences using an infinitive:
(i) He went to Amritsar.
(ii) He wanted to visit the Golden Temple.
Answer:
He went to Amritsar to visit the Golden Temple.

Question 2.
Combine the following sentences using the participle:
(i) I speak the truth.
(ii) I am not afraid of speaking of it.
Answer:
I am not afraid of speaking the truth.

Question 3.
A ……….. candle fell off the table, (burn) (Fill up the blank with a participle)
Answer:
A burning candle fell off the table.

Question 4.
He left the tap (run). (Fill up the blank with a participle)
Answer:
He left the tap running.

Question 5.
Combine the following sentences using a participle :
He had resolved on a certain course.
He acted with vigour.
Answer:
Having resolved on a course, he acted with vigour.

Question 6.
Combine the following sentences using a participle:
They had no fodder.
They could give the cow nothing to eat.
Answer:
Having no fodder, they could give the cow nothing to eat.

Question 7.
Success is not merely ………….. (win) applause. (Fill up the blank with a gerund)
Answer:
Success is not merely winning applause.

Question 8.
………….. (amass) wealth often ruins the health. (Fill up the blank with a gerund)
Answer:
Amassing wealth often ruins the health.

Exercise 3

Do as directed:

Question 1.
The ability …………. (laugh) is peculiar to mankind. (Fill the blank with an infinitive)
Answer:
The ability to laugh is peculiar to mankind.

Question 2.
Can you hope (count) the stars ? (Fill up the blank with an infinitive)
Answer:
Can you hope to count the stars ?

Question 3.
Combine the following sentences using a participle:
(i) I call a spade a spade.
(ii) I am not afraid of it.
Answer:
I am not afraid of calling a spade a spade.

Question 4.
The man seems …………. (worry) (Fill up the blank with a participle)
Answer:
The man seems worried.

Question 5.
We had a drink of the ………….. (sparkle) water. (Fill up the blank with a participle)
Answer:
We had a drink of the sparkling water.

6. Combine the following sentences using a participle:
(i) The stable door was open.
(ii) The horse was stolen.
Answer:
The stable door having been opened, the horse was stolen.

Question 7.
Combine the following sentences using a participle:
(i) We met a man.
(ii) He was carrying a log of wood.
Answer:
We met a man carrying a log of wood.

Question 8.
We were prevented from …………. (enter) the house. (Fill up the blank by using a gerund)
Answer:
We were prevented from entering the house.

Question 9.
We heard her …………… (sing) at the function. (Fill up the blank with a gerund)
Answer:
We heard her singing at the function.

Exercise 4 (Textual)

Do as directed:

Question 1.
He is slow, ……………. (forgive) (Fill up the blank with an infinitive)
Answer:
He is slow to forgive.

Question 2.
I am sorry …………… (hear) this. (Fill up the blank with an infinitive)
Answer:
I am sorry to hear this.

Question 3.
Combine the following sentences using an infinitive:
(i) He collects old stamps even at great expense.
(ii) It is his hobby.
Answer:
It is his hobby to collect old stamps even at great expense.

Question 4.
…………… (Run) water is not always fit for drinking. (Fill up the blank with a participle)
Answer:
Running water is not always fit for drinking.

Question 5.
………… (carry) by the wind, seeds are scattered far and wide. (Fill up the blank with a participle)
Answer:
Having been carried by the wind, seeds are scattered far and wide.

Question 6.
Combine the following sentences using a participle:
(i) He was dissatisfied.
(ii) He resigned his job.
Answer:
Having been dissatisfied, he resigned his job.

Question 7.
Combine the following sentences using a participle :
(i) We met a girl.
(ii) She was carrying a basket of flowers.
Answer:
We met a girl carrying a basket of flowers.

Question 8.
He is fond of ……….. (swim) (Fill up the blank with a gerund)
Answer:
He is fond of swimming.

Question 9.
Are you afraid of his …………… (hear) you ? (Fill up the blank with a gerund)
Answer:
Are you afraid of his hearing you ?

Question 10.
I have come ……………. (see) you. (Fill up the blank with an infinitive)
Answer:
I have come to see you.

Exercise 5 (Textual)

Question 1.
Combine the following sentences using an infinitive:
(i) He has five children.
(ii) He must provide for them.
Answer:
He must provide for them.

Question 2.
He has five children to provide for.
(i) He wants to earn his livelihood.
(ii) He works hard for this reason.
Answer:
He works hard to earn his livelihood.

Question 3.
(i) I saw him (enter) the house. (Fill up the blank with a participle.)
Answer:
I saw him entering the house.

Question 4.
He played a …………. (lose) game. (Fill up the blank with a participle)
Answer:
He played a losing game.

Question 5.
Combine the following sentences using a participle:
(i) He staggered back.
(it) He sank to the ground.
Answer:
He staggered back sinking to the ground.

Question 6.
She is very keen to …………. modelling, (take up) (Fill up the blank with an infinitive)
Answer:
She is very keen to take up modelling.

Question 7.
What she hates most is ……………. (smoke) (Fill up the blank with a gerund)
Answer:
What she hates most is smoking.

Question 8.
He objected to …………. money on cosmetics, (spend) (Fill up the blank with a gerund)
Answer:
He objected to spending money on cosmetics.

Question 9.
It is a penal offence ……………. bribe a public servant. (Fill up the blank with an infinitive)
Answer:
It is a penal offence to bribe a public servant.

Question 10.
The boys are anxious ………….. (learn) (Fill up the blank with an infinitive)
Answer:
to learn.

Exercise 6 (Textual)

Question 1.
Combine the following sentences using participles:
(i) The strikers held a meeting.
(ii) They wished to discuss the terms of the employers.
Answer:
The strikers held a meeting wishing to discuss the terms of the employers.

Question 2.
(i) The robber took out a knife.
(ii) He wanted to frighten the old man.
Answer:
The robber took out a knife wanting to frighten the old man.

Question 3.
…………. (consider) the facts, he received scant justice. (Fill up the blank with a participle)
Answer:
Considering the facts, he received scant justice.

Question 4.
Combine the following sentences using a participle:
(i) He walked away.
(ii) He was whistling.
Answer:
He walked away whistling.

Question 5.
My hair needs …………….. (cut) (Fill up the blanks with a gerund)
Answer:
My hair needs cutting.

Question 6.
I saw him …………….. (cross) the road. (Fill up the blank with a gerund)
Answer:
I saw him crossing the road.

Exercise 7 (Textual)

Combine each of the following pairs of sentences into one sentence by using infinitives:
Question 1.
I am learning Sanskrit. I want to study the Gita.
Answer:
I am learning Sanskrit to study the Gita.

Question 2.
He labours hard. He wants to succeed.
Answer:
He labours hard to succeed.

Question 3.
I am learning English. I want to study the works of Shakespeare.
Answer:
I am learning English to study the works of Shakespeare.

Question 4.
Children are going to the field. They want to play.
Answer:
Children are going to the field to play.

Question 5.
I have come here. I would like to see you.
Answer:
I have come here to see you.

Exercise 8 (Textual)

Fill up the blanks with one of these: (Infinitive, Gerund, Participle)

Question 1.
He has a …………… look, (surprise)
Answer:
surprised (Participle)

Question 2.
My father is a …………… man. (retire)
Answer:
retired (Participle)

Question 3.
They promised …………… me. (help)
Answer:
ro help (Infinitive)

Question 4.
…………… is pleasant, (play)
Answer:
Playing (Gerund)

Question 5.
…………… on the footpath is safe, (walk)
Answer:
Walking on the footpath is safe. (Gerund)

Question 6.
He gave me a pen …………… with, (write)
Answer:
He gave me a pen to write with. (Infinitive)

Question 7.
He gave up …………… (drink)
Answer:
He gave up drinking. (Gerund)

Miscellaneous Exercises

Infinitive

Exercise 1

Fill in the blanks with a infinitive:

1. …………… (waste) time is a folly.
2. …………… (err) is human.
3. I find it sensible …………… (remain) silent.
4. She seems …………… (be) happy.
5. …………… (toil) is the lot of mankind.
6. You need …………… (do) a lot.
7. I gave him a chance …………… (reconsider) his stand.
8. You are advised not …………… (mislead) your friends.
9. The game was about …………… (begin).
10. Let him leave the room.
11. Make him realise his mistake.
Answer:
1. To waste
2. To err
3. to remain
4. to be
5. To toil
6. to do
7. to reconsider
8. to mislead
9. to begin
10. To is not to be used in these sentences.
11. To is not to be used in these sentences.

Exercise 2

Use the following pair of sentences by using an infinitive:
1. The poor man had live children.
He must provide for them.
2. Turn to the left.
You will find my house.
3. My teacher will learn about my success.
He will be delighted.
4. This box is very big.
He can’t lift it.
5. I had no money.
I could buy no clothes.
Answer:
1. The poor man had ro provide tor five children.
2. Turn to the left for my house.
Or
Turn to the left to find rny house.
3. My teacher will be delighted to learn about my success.
4. This box is too big to lift.
5. I had no money to buy clothes.

Gerund

Exercise 1

Complete the following sentences with correct use oi gerund form of the verb:

1. He is good ar …………… (dance).
2. He is crazy about …………… (sing).
3. He doesn’t like …………… (play; cards.
4. I am afraid of …………… (swim) in the canal.
5. I am always interested in …………… (make) ftiends.
6. He is scared of …………… (travel! by air.
7. I suggest …………… (do) sonic m ire sums.
8. They insisted on …………… (cook) the dinner at home.
9. I thanked him for …………… (fix) my door.
10. I praised him for …………… (help) me in distress.
Answer:
1. dancing
2. playing
3. playing
4. swimming
5. making
6. travelling
7. doing
8. cooking
9. fixing
10. helping.

Participle

Exercise 1

Fill in the blanks with Present Participle:

1. The man …………… (drive) the car is my uncle.
2. Lorries …………… (come) over the bridge have to be careful of the wind.
3. Who was the girl …………… (wear) the red dress ?
4. Students …………… (submit) their essays late will lose ten marks.
5. The …………… (bud) flowers looked very lovely.
6. The …………… (run) bus rammed against the wall.
7. …………… (hear) the noise, he rushed to the spot.
8. They told us an …………… (amuse) account of their journey.
9. I saw the lion …………… (approach) us.
10. He watched them …………… (fight) over trigger.
Answer:
1. driving
2. coming
3. wearing
4. submitting
5. budding
6. running
7. Hearing
8. amusing
9. approaching
10. fighting.

Punjab State Board PSEB 12th Class English Book Solutions English Letter Writing Business Letters Exercise Questions and Answers, Notes.

PSEB 12th Class English Letter Writing Business Letters

1. Applications for Different Jobs

1. Write an application for the post of a Steno-typist.

14, Civil Lines,
Amritsar.
August 6, 20 ………….

The Advertiser,
Post Box No. 313,
The Tribune,
Chandigarh.
Sir,

This is in response to your advertisement in ‘The Tribune’ dated 4th August 20 for the post of a Steno-typist in your office. I beg to offer myself as a candidate for the same. As regards my qualifications, they are detailed below:
I passed my BA. Examination in 20 ………. from D.A.V. College, Amritsar. I was placed in the second division. After my graduation, I joined a commercial college where I learnt both short-hand and type-writing. My speed in short-hand is 75 w.p.m. and my speed in type-writing is 50 w.p.m. I have been working as a steno-typist with Sehgal & Co., Amritsar for the last five years. Besides short-hand and type-writing, I am also well conversant with other official work and can speak fluently English, Hindi and Punjabi.

I am still in the service of the same firm. I am seeking better opportunities which my present employer, with his limited business, cannot supply. He has no objection to my seeking a better job somewhere else.

I am a youngman of 25 with a sound physique and an impressive personality.

I enclose a certificate from my present employer in regard to my character, experience and ability. Should you appoint me to the post, it would be my sincerest effort to give you every satisfaction.

Yours faithfully,
H.M.L. Sood

2. Reply to above.

Messrs Datta & Co.,
130, Park Lane,
New Delhi-15.
August 11, 20……

Mr. H.M.L. Sood,
14 Civil Lines,
Amritsar.

Dear Sir,

We have your application of the 6th August, for the post of a steno-typist in our office. We are glad to inform you that we have decided to appoint you for the post.

Your appointment will be on a temporary basis for three months. If your work and conduct are found satisfactory, you will be made permanent. Your salary will be Rs 12000/- per month during the temporary period and Rs 12450/- per month with a yearly increment of Rs 450/- on your being made permanent. Your promotion depends entirely on your hard work, honesty and sincerity.

If the appointment is acceptable to you, please let us know by return of post the date when you can join.

Yours faithfully,
R.M. Sinha,
Manager

3. Write an application for the job of an Asstt. Manager in a large firm – Messrs Ghooki and Sons – selling electrical appliances. Give details of your qualifications and experience and mention the minimum salary acceptable to you.

510, Church Street,
Chandigarh.
July, 28, 20……….

Messrs Ghooki and Sons,
R-120, Connaught Place,
New Delhi-1.
Dear Sirs,

In response to your advertisement in ‘The Times of India’ dated July 26, 20…. for the post of an Asstt. Manager in your firm engaged in selling electrical appliances, I beg to offer my services for the same. As desired by you, I give below a few particulars about myself.

I passed my M.A. (Economics) from St. Stephen’s College, New Delhi in 20… After my Post-Graduation, I passed M.B.A. course in 20…. from Punjab University, Chandigarh. I was placed in the first division. Since then I have been working in Mumbai Electricals in the capacity of an Asstt. Manager. I am 30 years of age. I have an experience of six years. I can plan and execute sales independently. I have an unblemished record and my present employer wants me to stay on. But I wish to come to my home town that is, Delhi.

At present I am drawing ? 25000/- p.m. and have a free furnished accommodation. I can join your concern at a minimum salary of ^ 35500/- plus a free furnished accommodation and a car at the company’s expense.

I am prepared to attend an interview only at your expense. Copies of my certificates and testimonials are enclosed herewith for your kind perusal.

Yours faithfully,
Shankar Chauhan

4. Reply to Application No. 3.

Messrs Ghooki & Sons,
R-120, Connaught Place,
New Delhi-I.
July 31, 20 ………..

Sh. Shankar Chauhan,
510, Church Street,
Chandigarh.
Dear Sir,

In reply to your application dated 28th July, for the post of an Asstt. Manager, we are glad to inform you that we are ready to offer you the job at the salary demanded by you. We shall also give you free furnished accommodation and a car at company’s expense. However, you will have to sign a contract to serve in our firm for a minimum period of 4 years.

If the above terms are acceptable to you, kindly make it convenient to call at our office on 10th August, 20 ………… at 10 A.M. to settle other details of your appointment.

Yours faithfully,
R.P. Ghooki,
Managing Director

5. Write an application for the post of a P.A. to the Technical Manager of Calico Textiles. The post requires knowledge of short-hand typing and textiles of all varieties. Quote references.

14, Patel Road,
New Delhi-33.
May 26, 20 ……….

The Technical Manager,
Calico Textiles,
Amritsar.
Dear Sir,

In response to your advertisement in ‘The Times of India dated May 22, 20…. for the post of a P.A., I beg to offer myself as a candidate for the same.

I passed my Higher Secondary Examination in the first division. I passed B. Com. Final in 20 with Hons, in Secretarial Practice. I was immediately employed by Mohindra Textiles, New Delhi and have been working there to the satisfaction of my superiors. I am seeking better opportunities which my present employers with their limited range of business cannot supply. They have no objection to my seeking a better job somewhere.

I am a young man of 26 with a pleasing personality. I am capable of doing hard work. I enclose a certificate from my present employers in regard to my character, experience and ability.

Should you appoint me to the post, it would be my sincerest effort to give you every satisfaction. Once again I assure you that I will do my duty honestly and conscientiously.

Yours faithfully,
Sunil Sharma

6. Reply to above.

The Technical Manager,
Calico Textiles,
Amritsar.
May 30, 20 …….

Mr. Sunil Sharma,
14, Patel Road,
New Delhi-33.
Dear Sir,

In reply to your application for the post of a P.A. dated May 26, 20 …………. I should be glad if you call at my office on Monday morning next so that I may discuss details concerning the post for which you have applied.

The initial salary offered is Rs 12400/- per month, the engagement being terminable at one month’s notice on either side.

Yours faithfully,
S.M. Kakkar

2. Placing Orders

7. Placing Order for Steel Furniture.

Messrs Raja Sodhi &C Co.,
(Steel Furniture Dealers)
Prasad Road,
Ludhiana.
October 3, 20 ……….

Ref. No. 602/…. RS
The Manager,
Messrs Mahesh & Co.,
Rani Jhansi Road,
New Delhi-11.
Dear Sir,

We are in receipt of your quotation October, 1, 20… and thank you for the same. Please arrange to supply us the following goods as early as possible:
12 Nos…. Steel Almirah, size 84⊄⊄ at Rs 700 Per Piece.
12 Nos…. Manager’s Table, size 60⊄⊄ at Rs 400 Per Piece.
Sales and any other tax, if required, will be paid extra.

As desired by you, we are enclosing herewith our cheque No. STB/610073 dated October 3, 20….. for Rs 10,000/- only as advance and balance will be paid as soon as the goods are delivered.

In this connection, please note that if you make your terms liberal, we confirm, we shall be able to give you a good turnover per year. Please see what you can do.
Thanking you,

Yours faithfully,
Ram Chand
Manager

Enel. : One cheque.
No. : STB/610073.
Date : October 3, 20 …..
Amount : Rs 10,000/- only.
Back : State Bank of India, New Delhi-1.

8. Reply to above.

Messrs Mahesh & Co.,
Rani Jhansi Road,
New Delhi-11.
October 10, 20 …….

The Manager,
Messrs Raja Sodhi & Co.
(Steel Furniture Dealers)
Prasad Road,
Ludhiana.
Dear Sir,

We thank you for your order of the 3rd October, 20… and are pleased to state that the goods required have been despatched by passenger train this morning.

We trust that the goods will arrive in excellent condition and their quality will induce your good self to send further orders.

Yours faithfully,
K. Mahesh,
Manager

Enel. : Invoice
R/R No. : Nd/16003
Dated : October 10, 20….

9. Placing Order for Books.

R. Chand & Co.,
(Booksellers)
College Road,
Patiala.
July 18, 20 ……..

Messrs Malhotra Publishers,
Railway Road,
Jalandhar City.
Dear Sirs,

We are in receipt of your letter No. B-605/78 K dated 8th July, 20 alongwith a list of your publications. We thank you for the same.
Please supply us immediately:
40 Copies AS YOU LIKE IT by Prof. Ashok @ Rs 19/- each.
40 Copies MBD English Guide, TDC, III @ Rs 45/- each.
30 Copies MBD Economics Refresher B.Com. II, @ Rs 26/- each.
40 Copies MBD History Refresher TDC 11 @ Rs 12/- each 25 Copies
MBD Refresher English Guide, B.Com. 1, @ Rs 16/- each.

As desired by you, we are enclosing herewith our cheque No. SKB/K 10032 dated July 18, 20…… for Rs 1500/- only as advance and the balance will be paid against delivery.

Our next order will be forwarded as and when necessary.

Yours faithfully,
Ravinder Ghai,
Proprietor

Enel. : One cheque.
No. : SKB/K10032.
Date : July 18, 20 …….
Amount : Rs 1500/-
Bank : State Bank of India,
Patiala.

10. Acknowledging a Big Order.

Messrs Malhotra Publishers,
Railway Road,
Jalandhar City.
July 12, 20……

Ref. : MBD/MA-16 TR.
Messrs Bose & Co.,
University Road,
New Delhi-16.
Dear Sirs,

We thank you for your order No. 818. dated July 6, 20 ….. for 300 copies of MBD ENGLISH LITERATURE SERIES, “AS YOU LIKE IT” by Prof. Ashok @ Rs 90/- each copy. The order has been booked for execution and the books will be despatched by goods train on 14th July.

This is the first occasion we have the pleasure to execute your order. We heartily welcome you to our list of will-wishers and patrons. We assure you that you will always find our books satisfactory and hope this order will lead to an enduring connection with you.

Yours faithfully,
Balwant Sharma
Manager

11. Placing Order for Sports Goods.

“SPORTS CORNER”
14, Lodhi Market,
New Delhi-21.
July 6, 20 ……

Ref. No. Sc/506.
Messrs Kohli & Co.,
Sports Goods Manufacturers,
Industrial Estate,
Jalandhar City.
Dear Sirs,

I am in receipt of your quotation dated July 2, 20 …… and thank you for the same. Please supply us immediately the following items:
6 dozen Cricket balls @ Rs 120/- per dozen.
20 Cricket Bats (Durrani) @ Rs 75/- per bat.
50 pairs of Batting Gloves @ Rs 10/- per pair,
8 dozen Hockey balls (Ashok) @ Rs 100/- per dozen.
5 dozen Hockey Sticks (Champion) @ Rs 400/- per dozen.
Sales and any other tax, if leviable, will be paid extra.

As desired by you. I am enclosing herewith my cheque No. PB/c 70078 dated July 6, 20… for Rs 2,500/- only as advance and balance will be paid against delivery.
Thanking you,

Yours faithfully,
J.L. Mehta
Manager

Enel. : One cheque.
No. : PB/c 70078
Date : July 6, 20…
Amount : Rs 2,500/- only.
Bank : Punjab National Bank, New Delhi-1.

12. Reply to Letter No. 11.

Messrs Kohli & Co.,
Sports Goods Manufacturers,
Industrial Estate,
Jalandhar City.
July 9, 20 …….

The Manager,
“Sports Corner”,
14, Lodhi Market,
New Delhi-21.
Dear Sirs,

We are indebted to you for your order of the 6th July, 20 ……. and pleased to state that the goods required have been despatched by passenger train this morning.

We trust that the goods will arrive in excellent condition and their quality will induce you to send further orders.

Yours faithfully,
Satish Kohli
Sales Manager

Enel. : Invoice
R/R No. : JK/622532
Date : July 2, 20 ……..

13. Placing order for Servicing of Typewriting Machines.

Michael Limited,
(Commission Agents & Gen. Order Suppliers)
150, Park Road,
Kolkata-500025.
August 12, 20 ………

Ref. No. 1832/ML-630
Messrs Baliram & Co.
18, Church Lane,
New Delhi-32.
Dear Sirs,

Thank you for your quotation dated August 8, 20… for servicing of our different Typewriting Machines.

We do hereby accept your quotation under reference and request you to start work from the next week.

Regarding payment, though you did not mention anything in your quotation, we propose to make payments bi-monthly (i.e. 6 times in a year). Hence you are to submit your bill after completion of servicing of every two months.

Thanking you,

Yours faithfully,
R.S. Singh Purchase
Manager

14. Asking for details of Order.

Renu Hosiery Goods,
Industrial Estate,
Jalandhar City.
October 6, 20 …….

Messrs Dimple Readymade Garments,
106, Main Market,
New Delhi-13.
Dear Sirs,

We thank you for your Order No. 512 October 4, 20… for 5 dozen All-wool Pullovers, Catalogue No. 12 A, @ Rs 80/- each. We should like to inform you that you did not write the specifications in regard to size and colour. Surely, you would like to make your own choice and do not want us to send you size and colour of our selection.

We shall be glad if you kindly let us know your choice.

Yours faithfully,
V.K. Nanda
Sales Manager

3. Complaints And Adjustments

15. Complaint for Non-execution of an Order.

Upkar Traders,
6-Gurudwara Road,
Amritsar.
December 10, 20 …….

Ref. No. UT/103/………
Messrs Aggarwal & Sons,
15/162-E, Rampur Road,
Agra.
Dear Sirs,

Please refer to our order No. UT/503/ ………. dated November 2, 20 ……… for 200 Tins of Ghee (Lotus Brand).

We note with regret that the goods ordered were not delivered till this day, in spite of the fact that a prompt delivery was guaranteed and the order obtained on the strength of guarantee. The reasons are, however, beyond our knowledge.

This is not the first time a delay in delivery has occurred. Any way, we shall like to point out that business on these conditions can’t be continued for long.

We hope that this letter will cause you to settle the problem finally. Your immediate reply is expected.

Thanking you,

Yours faithfully,
Rajinder K. Singh
Purchase Officer

16. A Negative Reply to Letter No. 15.

Messrs Aggarwal & Sons,
15/162-E, Rampura Road,
Agra.
December 13, 20 ……..

Ref. No. A/s-603213-G,
The Purchase Officer,
Upkar Traders,
6-Gurudwara Road,
Amritsar.
Dear Sir,

We are in receipt of your letter No. UT/1003/ ……….. dated December 10, 20 ……….. and thank you for the same.

Please note that since there are some technical difficulties in our factory, we are not in a position to execute the order this time. We request you to procure the same from some other source.

We hope that you will realise that it is due to circumstances beyond our control. We do sincerely hope that you will not mind anything. We shall inform you as soon as the position becomes normal.

We are extremely sorry for this but are helpless. Thanking you in anticipation and expecting your best cooperation always.

Yours faithfully,
R.N. Aggarwal
Proprietor

17. An Affirmative Reply.

Messrs Aggarwal & Sons,
15/162-E, Rampura Road,
Agra.
December 13, 20 ………

Ref. No. A/s-603213-G,
The Purchase Officer,
Upkar Traders,
6-Gurudwara Road,
Amritsar.
Dear Sir,

We are in receipt of your letter No. UT/1003/ ……….. dated December 10,20 …………. and thank you for the same.

We are really sorry for the delay in executing your order due to a strike by the workers in our factory. Now the things are settled and we shall send the goods within two’ or three days.

We hope you will realise that the delay is due to circumstances beyond our control. We shall try our best to execute all your future orders in time.

Yours faithfully,
R.H. Aggarwal
Proprietor

18. Complaint for Express Bill.

District Sports Officer,
Govinda Stadium,
Amritsar.
July 10, 20……….

Ref. No. D50/6-CR.
M/s R.P. Kalra & Co.,
Sports Goods Manufacturers,
16, Industrial Estate,
Jalandhar City.
Dear Sir,

We are in receipt of the hockey sticks sent under cover of your invoice No. RPC/340 ……… dated June, 8, 20 ………. and thank you for the same.

In this connection, kindly note that in your invoice, you have charged Rs 26/- per stick while you quoted Rs 24/- per stick.

Please rectify the mistake and either send us the excess amount charged by M.O. or issue us a C.N. for the same. Prompt action is expected.

Yours sincerely,
Swami Talwar
D.S.O.

19. Reply to Above.

M/S R.P. Kalra & Co.,
Sports Goods Manufacturers,
16, Industrial Estate,
Jalandhar city.
July 13, 20 ……..

Ref. No. RPK/32-
The District Sports Officer,
Govind Stadium,
Amritsar.
Dear Sir,

We are in receipt of your letter No. DSO/6-CR dated July 10, 20…. and note the contents with thanks. The mistake took place due to the inadvertence of our billing clerk for which we are extremely sorry.

Enclosed please find our Credit Note No. RPK/6-88 dated July 13, 20…….. for the excess amount which may be adjusted with your next order.
Thanking you in anticipation and assuring you of our best co-operation and service. We remain,

Yours faithfully,
K.L. Sharma
Sales Manager.

20. Complaint for sending wrong books.

“Book-Shop”
University Campus,
Patiala.
July 26, 20…….

Ref. No. BS/102 ……….
Messrs Manjit Bros.,
Educational Publishers,
Jalandhar City.
Dear Sirs,

I am in receipt of the parcel sent under cover of your invoice No. MB 4078 dated July 24, 20…….. (for Rs 5200/-) and thank you for the same.

But on opening the parcel, I find that you have sent us the Punjabi Edition intead of Hindi Edition.

I presume due to the inadvertence of your packer, this mistake has taken place. I am sending the parcel back. I request you to replace the same immediately. I do hope that you shall do the needful as soon as my letter reaches you.

Yours faithfully,
K.C. Sachdeva
Manager

21. Reply to Above Letter of Complaint.

Messrs Manjit Bros.,
Educational Publishers,
Jalandhar City.
July 28, 20…….

Ref. No. MB/607……
The Manager,
“Book-Shop”
University Campus,
Patiala.
Dear Sir,
We are in receipt of your letter No. BS/102 ……….. dated July 26,20 …… and noted the contents with thanks.

We are sending you the Hindi Edition by the next available Passenger Train. On receipt of the parcel, please do drop a line to us.

We are extremely sorry for the inconvenience caused to you due to this. We assure you that necessary care will be taken at the time of execution of all your future orders. Please never mind for this.

Thanking you in anticipation and assuring you of our best services always.

Yours faithfully,
D.K. Joshi
(Partner)
For Manjit Bros.

22. Complaint for Defective Goods.

Rehman & Sons,
Shawl Dealers,
Manik Road,
Amritsar.
November 10, 20 ……..

The Manager,
Messrs Bal Chand Lai Chand,
The Mall,
Jalandhar.
Dear Sir,

We thank you for the prompt execution of our Order No. RS/265-D, dated October 21, 20 ……

Unfortunately, on opening the case, we find twenty shawls torn which are unsaleable. There are six shawls whose finish is decidedly bad. In no way they appear like high-priced goods, a fact which is very much against our business ethics.

We are unable to offer such shawls to our customers and therefore returning them in the hope that you will let us have goods of a better quality.

Yours faithfully,
Kadir Rahman
(Partner)

23. Reply to Above.

Messrs Bal Chand Lai Chand,
The Mall,
Jalandhar.
November 18, 20 ……..

Ref. No. BCLC/……….
Messrs Rehman & Sons,
Shawl Dealers,
Manik Road,
Amritsar.
Dear Sirs,

We are in receipt of your letter dated November 10, 20……. complaining about the defective goods supplied by us. We are extremely sorry that we have not been able to supply goods to your satisfaction. Generally, our men thoroughly check the goods before they are despatched to our clients, but as some of our shawls have been found defective and inferior, we are ready to replace them at our cost.

We shall forward you a fresh supply of shawls within a few days and we trust that these will be to your full satisfaction.

Yours faithfully,
H.D. Sharma
Manager

24. You recently travelled on an old public transport bus which broke down a couple of times on the way. Write a letter to the manager of the company giving details of the poor condition of the bus and the harassment that it caused to the passengers. Also make a few suggestions to improve the service

To
The Manager,
Malhi Bus Service Private Limited,
Jalandhar Bus Stop.
Jalandhar.
Sir,

I wish to bring to your kind notice that I had a very bitter experience while travelling by one of the buses of your company BTK 2534 plying between Batala and Jalandhar on 12th January, 2012, I was in a hurry to reach Jalandhar. After buying a ticket for my journey, I boarded the bus. The bus left Batala at ten o’clock. As I took my seat, I found the seat cover torn. Some of the windows had no panes. The floor or deck of the bus was in a wretched shape. The iron plates were broken and twisted. Technically it could be called more of scrap than a roadworthy bus. It appeared to be a very aged bus.

After leaving Batala, the bus began to slow down after covering about two kilometres. Then it broke down and stopped near Achal Sahib. The driver and the conductor tried to repair it but it showed no sign of getting re-started. I got the impression that the bus was not at all roadworthy even when it was going to start from the Batala bus stop. Probably the conductor and the driver were in league with each other to dupe the passengers. After struggling to start, the bus showed signs of moving. But it began to run at a slower speed. All the passengers felt uncertain about the bus reaching the destination. We had already wasted 45 minutes at Achal Sahib.

Thereafter the bus came to a grinding halt at Baba Bakala. All the passengers got down. They asked the conductor to return their fare as they wanted to take other buses to reach Jalandhar. The conductor refused to refund the fare. He wanted the passengers to wait for the bus to re-start. Another forty-five minutes were wasted. Someone brought a mechanic.

He tried his level best to start the bus. The bus moved at a very slow speed. It broke down again at Beas. We had a similar experience at Subhanpur. It was 4.30 p.m.. by the time we reached Jalandhar. A journey of one and a half hours was completed within six hours.

It is a reflection on your competence as the manager of the company. You are requested to remove such a useless bus from plying. It is nothing short of deliberate cheating. You should ask the driver and the conductor why they sent such a bus to transport passengers. The public expects a proper value of their money.

Yours truly,
Gurtej Singh Dhillon
and ten others.

4. Inquiries, Quotations & Replies

25. Letter of Inquiry concerning the Status of the Firm.

Raman Manufacturing Co. Ltd.
Industrial Estate,
Jalandhar City.
December 4, 20……

Mr. D.R Azad,
5/31, Nehru Road,
Delhi.
Dear Sir,

We have been requested by M/s Singh Bros., Civil Lines, Delhi, to forward twenty pieces of Steel Almirahs for which they propose to pay by means of a three months’ bill. As the amount involved is more than Rs 10,000/-, we are naturally a bit reluctant to allow credit without some assurance as to their financial standing.

We shall feel obliged if you give us the detailed information regarding their financial status and reputation. Please do inform us whether you consider it proper to grant the said credit to them.

We thank you in advance for any other information which you can give us.

Yours faithfully,
K.R. Mani
Sales Manager

26. A favourable Reply to the above letter.

5/31, Nehru Road,
Delhi.
December 10, 20 …….

The Sales Manager,
Messrs Raman Manufacturing Co. Ltd.
Industrial Estate,
Jalandhar City.
Dear Sir,

I am pleased to be of service to you in ascertaining the financial status and reputation of M/s Singh Bros, Civil Lines, Delhi, about whom you enquired on December 4, 20……

I am glad to inform you that the said firm enjoys the fullest respect and reputation in local commercial circles. The firm is known to be possessed of a considerable amount of capital.

There seems to be no reason for withholding credit to the extent you mention, and you should have no hesitation in doing business with the firm on the terms suggested.

Yours faithfully,
D.P. Azad

27. An Unfavourable Reply.

5/31-Nehru Road,
Delhi.
December, 10, 20……….

The Sales Manager,
Messrs Raman Manufacturing Co. Ltd.
Jalandhar City.
Dear Sir,

In reply to your inquiry of the 4th December concerning M/s Singh Bros., Civil Lines, Delhi, I could recommend a policy of caution. Though the said firm is an excellent business organisation with a wide circle of customers, yet their operation hardly warrants an allowance of credit to the extent of Rs 10,000/- You should hesitate to accept the conditions they suggest. As far as I can see, payment of half this sum i.e. Rs 5000/- in cash would be advisable.

I hope that you will recognise the importance of keeping the information strictly private.

Yours faithfully,
D.R Azad

28. Asking for Quotation of Pencils.

Pandit Brothers,
Gandhi Road,
Ludhiana.
April 10, 20 ……..

Messrs Jain Bros.,
Pencil Manufacturers,
10, Industrial Area,
New Delhi-28.
Dear Sirs,

We are very much interested to purchase various brands of pencils manufactured by you, in large quantities regularly every month.

We shall be glad if you send us the details of the pencils manufactured by you quoting prices and other terms.

We assure you that we shall be able to give you good sales provided your terms and conditions suit us.

We hope you will facilitate business by quoting us the lowest possible rates. Your immediate action will be highly appreciated.

Yours faithfully,
S.M. Pandit

29. Reply to Above.

Messrs Jain Bros.,
Pencil Manufacturers,
10, Industrial Area,
New Delhi-28.
April 14, 20 ………

Ref. No. JB/16032-D.
Mr. S.M. Pandit,
Pandit Brothers,
Gandhi Road,
Ludhiana.
Dear Sir,

We are in receipt of your letter dated April 10, 20 ………. and thank you for the same. We are sending herewith our catalogue containing quotations for quantities from our existing stocks. You will also find the terms of payment indicated therein. But packing charges will be extra.

We like to add here that we shall be very glad to offer you a special discount of 2½% over and above our usual trade terms if your monthly requirement is 200 grosses (assorted).

Please let us have your standing order as early as possible which will always receive our most careful and prompt attention.

Yours faithfully,
P.K. Jain
Managing Director

Punjab State Board PSEB 12th Class English Book Solutions English Letter Writing Letters to Editor Exercise Questions and Answers, Notes.

PSEB 12th Class English Letter Writing Letters to Editor

1. Write a letter to the Editor of a newspaper describing the insanitary conditions prevailing in your town.

17, G.T. Road,
Gobindgarh.
November 8, 20 ……..

The Editor,
The Tribune,
Chandigarh.
Sir,

Through the columns of your esteemed paper, I want to draw the attention of the municipal committee authorities to the insanitary conditions of the bazaars and streets of Gobindgarh.

Sweepers do not sweep the streets and clouds of dust rise and enter ears, noses and throats of the people. That is why all kinds of diseases are on the increase. Whenever people move out of the houses, a thick layer of dust settles on their clothes. Why are the roads not sprinkled with water ? Are the authorities waiting for the next monsoon .? There are a large number of pits in the streets where water is allowed to stand. This stagnant water is a favourite breeding place for the mosquitoes. This water should be removed and the pits should be filled with dust otherwise an epidemic will spread in the town.

It is high time that the authorities should wake up and discharge their duties efficiently in the interest of public health.

Yours truly,
Mohan Dass

Word-notes : Insanitary – स्वास्थ्य बिगाड़ने वाली, ਸਿਹਤ ਵਿਗਾਤਨ ਵਾਲੀ Sweep – झाडू लगना, ਸਾਤੂ ਦੇਟਾ Layer – तह, ਤਹਿ Pits – गड़दे, ਵੋਏ Stagnant – standing. Epidemic – छूत की बीमारी, ਵੂਤੇ ਚੀ ਬਿਮਾਰੀ High time – proper time.

2. Write a letter to the Editor of a newspaper commenting on the rise in prices.

43, Railway Colony,
Amritsar.
May 4, 20……

To
The Editor,
The Indian Express,
New Delhi.
Sir,

I shall feel grateful to you if you publish the following few lines in your esteemed paper.

The rise in prices of essential articles has crushed every heart and living has become very costly these days. The labourers, middle class persons and the salaried people are groaning under their burden. The purchasing power of the rupee is fast sinking. The prices of the essential articles are rising sky high. The housewife finds it extremely difficult to manage the family budget. A vast majority of people in our country find it difficult to make their both ends meet. In certain families, people go without food at night. Milk has become a luxury. Fruit is no longer a part of our diet. The general health of the children in our country is suffering a setback.

It is high time that the government should take efficient steps to control the prices as early as possible. It should punish the black-marketeers and profiteers. Price-lists of all goods should be displayed at all shopping centres. A person who charges a higher rate should be severely punished. Both customers and shopkeepers should be punished for buying and selling things at higher prices. The rise in prices must be checked. At least the prices of essential articles of daily use must be brought down to a very reasonable level.

Yours truly,
Renuka

Word-notes : Groaning – crying. Sinking – decreasing. Displayed – put for show. Essential – अनिवार्य, ਜ਼ਰੂਰੀ.

3. Write a letter to the Editor of a newspaper about the need of parks and gardens in different localities of your city.

13, Kartar Singh Sarabha Nagar,
Ludhiana.
April 25, 20…….

To
The Editor,
The Tribune,
Chandigarh.
Sir,

I shall feel highly obliged if you publish the following few lines in your esteemed daily.

It is well-known to authorities that Ludhiana has become a very crowded and congested city. Population of the town is increasing very rapidly. Bihari labourers are living in wretched conditions. Open fields and beautiful gardens have been replaced by residential houses and shanties. Most streets of the city have grown into slums. Some congested houses do not get sunlight. Parks are needed for providing fresh air to the people. It is rightly remarked that parks and gardens are the lungs of a city.

They give us pure and fresh air and thus keep our body healthy. The young and the old, women and children can take exercise and relax in these parks and gardens. The city dwellers will really have a sense of relief as they will be relieved from their prison-houses for some time. It is, therefore, requested that the authorities should seriously consider this aspect, and provide public parks in those parts of the city which are very badly congested.
Thanking you,

Yours faithfully,
Surinder Kaur

Word-notes : Shanties – झोंपड़ियां, ਲੋਂਗੀਆ Congested – भीड़ वाला, ਭੀਤ ਭਰਿਆ

4. Write a letter to the Editor of a newspaper on evils of street-begging.

H-222, Sarojini Nagar,
Jalandhar.
May 27, 20 ……….

To
The Editor,
The Tribune,
Chandigarh.
Sir,

Through your esteemed paper, I wish to draw the attention of the authorities and the public to the evils of street-begging in our country.

Street-begging is a regular nuisance. It is a blot on the fair name of our country. Begging is an evil. But in India, it has become an organised profession. Almost at every step you are greeted by a beggar. Beggars gather round you in buses, in trains, in the bazaars and near the places of worship. Our national prestige suffers a good deal when beggars greet foreigners at every turn of the road.

Unfortunately, in our country giving alms is believed to be a part of religion. The most painful thing is that most of these beggars are able-bodied. They can do manual labour, if they so desire. But they find it more easy to live on other people’s hard-earned money. This easy- got money, they spend on drinking and gambling. Then the tragedy is that these beggars encourage even their children to beg. Thus, this so-called charity leads to so many vices.

The need of the hour is that this social evil must be put to an end. Healthy beggars should be made to work. The disabled, the crippled and the blind beggars, however, should be maintained at the state expense. They should be kept in some suitable buildings outside the city where they should be fed at Government expense. Not only begging should be declared illegal by an Act of Parliament but public opinion should also be educated against it. Those who give alms to the strong and able-bodied men and women and children should be punished.

Yours truly,
Ashok Kumar

Word-notes : Nuisance – a harmful thing. Blot – ugly mark. Prestige – honour, Alms – ਭਿਖਿਆ , भिक्षा Charity – ਦਨ, दान, Manual – physical. Vices – evils. Public opinion – ਲੋਗਸਤ , लोकमत.

5. Write a letter to the Editor of a newspaper complaining against reckless driving.

833, Ummatan Mohalla,
Nawanshahar.
July 6, 20…….

To
The Editor,
The Tribune,
Chandigarh.
Sir,

I shall feel grateful if you allow me some space in your esteemed paper. I want to draw the attention of the authorities concerned towards reckless driving in Nawanshahar.

This city has wide roads and chances of accidents are comparatively few here. But probably, this very fact encourages reckless driving. It has become a fashion. Motor-drivers drive at a terrific speed. Trucks carrying sand, stones or bricks are seen moving at a very fast speed. Cars pass by you whizzing even at turnings. Young college boys drive motor cycles and scooters without caring for their own or anybody else’s life. It is a positive danger to school-going children, to old men and women who cannot cross the road quickly.

This rash driving is a regular nuisance and is responsible for fatal accidents which take place daily. Not a single day passes when we do not hear of some accident. Only yesterday, a young man who was on the left side was run over by a motor cycle. The motor-cycle driver drove away at such a great speed that nobody could even take down the number of his motor cycle. Speed limits are not strictly enforced. Many a time the defaulters bribe the policeman on duty and get off.

The dire need is that a speed limit should be fixed and the offenders be severely dealt with.

Yours truly,
Rajesh Khanna

Word-notes : Reckless – rash. Terrific speed – great speed. Fatal – that which results in death. Dire – great. Offenders – defaulters.

6. Write a letter to the Editor of a newspaper complaining against the use of loudspeakers at night.

14, Model Town,
Ludhiana.
March 1, 20….

To
The Editor,
The Tribune,
Chandigarh.
Sir,

I shall be’thankful to you if you publish this letter in your esteemed paper.
The loudspeaker, like most of the inventions of science, is both a blessing and a curse. It is useful at a public meeting. Its use as a handy instrument for commercial propaganda can be tolerated during the day. But its use at night is intolerable. It causes a lot of inconvenience to the sick people. They cannot relax peacefully.

Some people and institutions use the loudspeaker at night as well as in the early hours of the morning. Their noise makes it difficult for one to study or to do any serious work in one’s home. The examinations are fast approaching. It will be greatly appreciated if the use of loudspeakers is banned after 8.30 p.m.
Thanking you,

Yours truly,
Dharmendra

Word-notes : Commercial – business. Approaching – coming near. Banned – forbidden.

7. Write a letter to the Editor of a newspaper criticising the evil of drinking.

202, Urban Estate,
Phagwara.
January 22, 20 …………

To
The Editor,
The Indian Express,
Chandigarh.
Sir,

Through your correspondence columns I like to draw the attention of the public towards a great social evil. This is the evil of drinking. It has become very common these days. The number of wine addicts is on the increase. People begin drinking in small doses. In course of time, they become hardened drunkards.

Drinking wine is harmful for the mental and physical growth of a person. In some cases, it leads to the financial ruin of the drunkard. Under the influence of wine, men begin to behave like beasts and animals. Wine leads to crime. Robberies, rapes and murders are often committed under the influence of drink. Excessive drinking ruins a mans health.

A drunkard begins to spend too much money on wine. The family depending on a drunkard begins to suffer. A drunkard cannot give proper education to his children for lack of money. The members of his family have to go without basic facilities of a comfortable life. Thus wine drinking weakens a society financially and morally. We should try to discourage the use of wine from our society.

Yours truly,
Subhash Kapoor

8. Write a letter to the editor of a daily newspaper complaining of open manholes near a children school.

12/3, Hari Nagar,
Hoshiarpur.
December 15, 20……

To
The Editor,
The Tribune,
Chandigarh.
Sir,

Recently we have had a series of accidents in our locality due to the manholes. In the nights and during the rainy season, when visibility is poor, there have been many cases of elderly people stumbling over these manholes. The other day, a lady did stumble over a manhole but luckily escaped from getting seriously hurt. As there is a children’s school nearby, we are in constant fear that a child may slip and fall into a manhole. When it rains, the road is flooded, as is the case with the other roads, and the open manholes are a death trap to the unwary and the playful children.

The streets are not well-lit and often the manhole covers are stolen. The hazards caused by open manholes are too dangerous for any citizen to keep quiet in the matter.

Will the authorities concerned either devise manhole covers which do not attract thieves or pay prompt attention by sending the officials concerned regularly to check and ensure ‘ that these death traps are promptly covered ? Otherwise, it will be only a question of time before serious accidents occur involving loss of lives of innocent children.

Yours faithfully,
Ravinder

9. Write a letter to a daily newspaper complaining of eve-teasing near the bus-stops.

11-B,
Harbans Nagar,
Jalandhar.
April 15, 20…….

To
The Editor,
The Tribune,
Chandigarh.
Sir,

I shall be thankful if you kindly allow me the hospitality of your esteemed columns to air my views on eve-teasing near the bus-stops.

I am at pains to report the irresponsible behaviour of youths and even some middle-aged gentlemen, near our bus-stops. Not only do we have to put up with obscene posters, but also have to see our young women harassed and teased while they wait for a bus.

The other day, two young ladies were standing at a distance from the bus-stop, obviously to avoid these roadside Romeos. As soon as the bus came, they started running to get into the already over-crowded bus. These ’Romeos’ who were standing nearby, made such vulgar comments about them, that I, a sixty-five year old man, had to blush and close my ears.

These incidents have become quite common. I wish to suggest that the Mahila Samaj should post a voluntary welfare worker near these bus-stops during the rush-hours and, if necessary, take the help of the women police also. Unless this menace is curbed, our young girls and women coming from sheltered background, will get intimidated and might, in due course, refuse to do even their ordinary daily shopping. Instead of helping the cause of emancipation of women, we seem to be going back to the Dark Ages by tolerating the unseemly behaviour of these men and their like who indulge in eve-teasing.

Kindly take such steps as are possible to put an end to such acts of intimidation of women, which alone can help preserve our rich traditions and culture which have always held our women in deep reverence.

Yours faithfully,
Raman Kapoor

10. Write a letter to the Editor of a daily newspaper expressing your views criticising some of the unhygienic habits of Indians such as urinating and spitting at the public places.

141, Civil Lines,
Ludhiana.
June 24, 20 ……..

To
The Editor,
The Indian Express,
Chandigarh.
Sir,

I shall be thankful if you kindly let me express my views about some of the unhygienic habits of our countrymen at all the public places.

People in our country keep on spitting afid urinating at all the public places. Spitting in public places is not just disgusting, it is extremely damaging for the public health. Diseases like tuberculosis (TB) are likely to spread in a society that tolerates them than in those that do not. India is the country with the largest number of TB cases in the world. We should take our cue from the Mumbai Municipal Corporation which has started imposing fines on those individuals who spit at the public places. Tuberculosis which is spread by spitting coughing, caused nearly 10,000 deaths in Mumbai last year.

There are various cleanliness and sanitation bylaws. Under these we can find defaulters/ offenders. Spitting in public calls for a rupee 50 fine, as also urinating. Sometimes people are seen defecating in parks and gardens. Another unhygienic habit of Indians is the habit of chewing pan, tobacco or pan masala, all of which requires spitting.

This leads to spraying of saliva in the public places. Sometimes rich people spit out a huge gob of pan by rolling down the windows of their cars. Some cleanliness drive must be launched in cities and towns and people should be warned against spitting and urinating in public places.

Yours trully,
Neeru Bajwa

11. Write a letter to the editor of a newspaper pointing out the growing incidence of drug menace in India.

72, Canal Park,
Dhariwal.
April 22, 20 ………

To
The Editor,
The Indian Express,
Chandigarh.
Sir,

Kindly allow me to express through columns of your esteemed paper my views about the growing incidence of drug menace in the country.

Drug addiction is a major social evil of modern times. Drugs are used by certain persons, to experience a floating sensation, feeling ease and relaxation. The use of drugs means physical dependence upon a drug. This includes the development of tolerance and withdrawal. An addict who develops tolerance requires more and more of the drug to take him to the world of fantasies. The habit of drug taking occurs at school, college and university or at a trip or at some social gatherings.

Youngsters who are very very shy, have emotional problems, inferiority complex and lack of strength and stamina. They fall easy victims to this habit. Lack of parental care and supervision, lack of religious and moral education, media and pop culture, hatred for authority, broken homes and truancy lead to the use of drugs. Drug addicts use narcotics such as heroin, smack, morphine and stimulants like cocaine and depressants.

Addiction to drugs is looming large as a social problem evading all solutions. In India, drug addiction is increasing. Intoxicating drugs are very harmful for health. The government has taken a number of steps to check the growing menace. The basic factor responsible for proneness to drugs is the sickening, emotional and psychological surroundings that leave the victim with no other option than to seek momentary escape from the mental tension. If the young ones are assured of proper love and care, these problems will not arise.

Yours truly,
Kulbhushan

12. Write a letter to the editor of a newspaper expressing your views against drunken driving.

15, The Mall,
Hoshiarpur.
March 20, 20……..

To
The Editor,
The Tribune,
Chandigarh.
Sir,

Kindly permit me to express my views about drunken driving on our roads in the columns of your newspaper.

We often hear it said that we should not drink and drive. But not many drivers or people on the wheels follow this advice. It is reported that more than 86,000 drunken driving accidents occur everyday across India. The absence of a compulsory alcohol test makes it difficult to get an exact figure. The number of such accidents is increasing. There is no law against drunken driving in the country. Under the old act of 1856, a drunken driver is charged with only a fine of Rs 2000 or imprisonment for six months and Rs 3000 or imprisonment for two years if found guilty a second time.

Laws against drunken driving should be made more stringent. Parties of drunken youngsters should be checked. They should be sent to jail for at least two weeks as measure to deter others from drunken driving. In Canada, a person driving under the influence of alcohol is barred from the roads and is also jailed. Stiffer penalties and fine should be imposed on those who are found guilty of drunken driving. Laws have to be made stiffer to act as a deterrent. The rich people should not be let off mildly if they are found guilty of drunken driving.

Yours truly,
Neeru Bajwa

13. Write a letter to the Editor of a newspaper expressing your views about underage driving of cars on the roads of Punjab cities.

25, Model Town,
Ludhiana.
October 22, 20 ………

To
The Editor,
The Tribune,
Chandigarh.
Sir,

Kindly allow me to express my views against the underage driving in the readers’ columns of your newspaper.

There are many vehicles on the roads nowadays. Fond parents indulge their underage or minor children to drive the cars. They take pride in seeing their minor children driving their cars. They are generally the kids of rich people driving cars without licences. According to reports published in newspapers, about 2097 minors were prosecuted in 2011. The number of challans issued last year registered a 16% increase over 2014. With the rise in the number of vehicles and an increased exposure to the outside world, it is feared that more and more children will want to drive. Principals of schools should advise children not to drive vehicles belonging to their parents when they are below eighteen. It might be a good idea for Principals to talk to parents.

A minor driving should be made to pay a fine of Rs 1000/- and Rs 500/- in case of motor-bike or scooter. The owner of the car whose car is driven by a minor should be made to pay Rs 1500/- as fine and he should be kept in jail for three days. Such a measure must be implemented because minors are often responsible for rash driving and accidents.

Yours truly,
Kamini Bajwa

14. Write a letter to the Editor of a newspaper expressing your views about lavish spending on marriages and other social events.

113, Tagore Nagar,
Hoshiarpur.
April 24, 20 ……….

To
The Editor,
The Indian Express,
Chandigarh.
Sir,

I shall be thankful if you kindly allow me to express my views regarding lavish and wasteful spending on marriages and other social events.

It is felt by most right thinking people that marriages and other social functions should be made frugal affairs. The number of dishes served at weddings should be restricted. They should not be allowed to exceed five or six. We should take our cue from Pakistan where only one dish is allowed at marriages and social gatherings. Marriages should not become event- filled as some have become. Ceremonies like mehndi, sangeet, cocktail parties and receptions should be clubbed into one or two. The number of guests or baratis should also be fixed and no violation should be tolerated.

The rich people may be able to spend a lot of money on these events. There is a lot of heart burning among those who cannot afford to spend so much on these functions. An awareness campaign should be launched by spirited social workers to advise the people to make marriages and other social functions frugal matters.

There are many people living under the poverty line in the country. By spending lavishly on marriages and other social events, we give the impression that we have no sympathy for their misery. We should try to reduce the misery of the poor people by making marriages and other social events simple affairs.

Yours truly,
Namrata Mann

15. Write a letter to the editor of a daily newspaper criticising the raising of age limit for consensual sex from 16 to 18 years.

15, Civil Lines,
Hoshiarpur.
May, 23, 20……….

To
The Editor,
The Tribune,
Chandigarh.

Kindly allow me to express my views about the raising of age limit for consensual sex from 16 to 18 years.

I understand that the worthy law-makers of our country are contemplating raising the age limit for consensual sex from 16 to 18 years for growing boys and girls. Even some of the courts and lawyers have expressed their views about this raising of age limit. They argue that with the advent of cable television, internet and rapid changes in biological factors, children are getting exposed to knowledge about sex and marital relations at an early age.

This results in boys and girls of young age being smitten with cupid at an early age before they attain majority or reach the zone of socially acceptable behaviour of responsibility. Parents are always opposed to such alliances between boys and girls. And the boys and girls in certain cases resort to elopement.

It is observed that even boys in some cases become victims as they do not understand the consequences of their actions and are sometimes lured by the girl. Such cases should act as an eye-opener for the worthy law-makers of our country. They should try to understand that gone are the days when a child of 16 to 17 years of age was ignorant about sex. Law-makers must have second thoughts before fixing the age of consensual sex.

Yours truly,
Unmukt Malhotra.

16. Write a letter to the editor of a daily newspaper pointing out some of the advantages and disadvantages of using mobile phones.

12, Basti Tankan Wali,
Ferozepur.
May 23, 20……..

To
The Editor,
The Tribune,
Chandigarh.
Sir,

Kindly allow me to express my views about the advantages and disadvantages of using mobile phones very frequently.

It is true that mobile phones have several advantages. There are disadvantages and demerits as well. It is undoubtedly correct that mobile phones are playing a vital role in every aspect of our life. Many users pass their leisure by playing games or watching songs and movies on mobile phones and having long chats with their friends. But there are some demerits as well.

Most of the economies of several countries are under depression due to several reasons and frequent use of mobile phone is one of them. Mobile phone is destroying our emerging students because of its unproductive use and this is a big drain on every economy. Teenage students spend most of their time by keeping themselves busy in messaging and music. They use mobile phones during school timings. Young students become intimate through mobile phones. They start taking pictures of each other. Then they try to blackmail each other leading to so many moral and social complications.

The mobile radiation is a health hazard. Mobile phones use electromagnetic radiation which may be harmful to human health. Recendy WH.O. confirmed that mobile phone may represent a long term health risk. It was said that mobile phone radiation is a carcinogenic health risk. Heavy users of mobile phones can have a risk of brain cancer. We should take practical steps to reduce exposure to radiation. We should reduce the number of calls. The wise approach is to follow the precautionary principle of reducing the exposure as best as we can.

Yours truly,
Narendra

17. Write a letter to the editor of a daily newspaper, emphasising the need for moral values in our curriculum (the group of subjects studied in a school, college, etc.).

To
The Editor,
The Indian Express,
Chandigarh,
Sir,

Kindly allow me to express my views in the correspondence columns of your newspaper. It is lamented by all right thinking people that the modern society is suffering from a moral vacuum. There is no place for moral values in our society. In olden days religious and moral values were highly valued. They were the basis of Indian culture. We took pride in valuing them. But the modern society has left all the moral values under the bad influence of materialism. Most of the social ills in our society are there because morality has been divorced from life. It has been thrown in the background.

There is an urgent need for re-building our society on moral values. For this purpose, we should introduce moral education as a necessary part of our syllabus in each subject. It is not that we should preach dogmatism or communalism. We should try to inject moral values in the minds of students. Students should be taught the value of truth, honesty, justice, good moral character, brotherhood and love for our fellow-men.

Corrupt practices, as are prevalent in our society, should be brought to the notice of our students. There should be lectures on moral values in all classes. Some weightage should be given to such subjects in each question paper. This could go a long way in inspiring our students to assimilate some of the moral values.

Yours truly,
Preeti Mann

18. Write a letter to the editor of a daily newspaper expressing your views about vulgarity in Punjabi songs.

212, Urban Estate,
Khanna.
July 15, 20 …….

To
The Editor,
The Indian Express,
Chandigarh.
Sir,

Kindly allow me to express my views about vulgarity in Punjabi songs through the correspondence columns of your newspaper.
All well-wishers of Punjab and Punjabi culture feel that Punjabi songs being heard in Punjab’s homes, villages, towns and public places are vulgar and lewd. They cater to a low taste. They glorify drinking, drugs and violence. They do not encourage good values of life. One feels embarrassed while listening to such songs. Punjabis say that Punjabi music became vulgar with Hey Jamah. It allowed to trigger vulgarity. It might be difficult to check the spread of vulgarity as this has become the vogue. Government should try to induct refinement and decency in the cultural fare by Punjabi singers. Song writers should be directed to give some refinement in their songs.

Yours truly,
Rani Ahuja

19. Write a letter to the editor of a newspaper expressing your views about the evil of aduleration in most of the eatables and domestic needs of daily use.

120, Jhoolna Mahal,
Gurdaspur,
May 21, 20………

To
The Editor,
The Indian Express,
Chandigarh.
Sir,

Kindly allow me to express my views about adulteration practised by sellers of food stuffs and eatables through this letter in your newspaper.
Adulteration of eatables and daily needs of people are easy targets in our country. Adulteration means making a commodity inferior in quality by mixing it with something inferior.

It enables a man to earn a fast buck. Like corruption, adulteration has come to stay because of an unholy nexus between the merchants, officials and politicians. We have a law against adulteration but it is rarely enforced. Many precious lives are lost because of the greed of adulterators. Illicit wine takes the lives of many people every year in different states of the country. There is hardly any item in the Indian market which is not adulterated. Research has shown that even fruits, vegetables and cereals, pulses sold in the market contain high levels of toxic metals like lead, nickel and chromium.

Chemicals are used to make vegetables look greener. It is shameful that our Health Ministry is sleeping. Some strong steps need to be taken so that adulterators get deterrent punishment immediately after the crime. TV tells us that day after day milk, ghee, medicines, khoya, paneer, fruits and vegetables are adulterated in one way or another. Health Ministry should recommend immediate punishment for this crime.

Yours truly,
Raj Chibber

Punjab State Board PSEB 12th Class English Book Solutions English Application Writing Exercise Questions and Answers, Notes.

PSEB 12th Class English Application Writing

1. Write an application to the Secretary, Board of Secondary Education, S.A.S. Nagar, Mohali, asking for a duplicate copy of your marks sheet.

To
The Secretary,
Board of Secondary Education,
S.A.S. Nagar, Mohali.
Sir,

With due respect I beg to state that I passed my secondary examination in 20…. I was a regular student on the rolls of D.A.V. Senior Secondary School, Jalandhar. My roll number was 87316. I got first division by securing 66 per cent marks. Unfortunately, I have lost the original marks sheet. I am in need of a duplicate copy of marks sheet.

I am enclosing a postal order for Rs 25/- as the fee for issuance of a duplicate marks sheet. Kindly send the duplicate copy of my marks sheet at the earliest.
Date : May 14, 20 …….

Yours faithfully,
Harbans Singh
H.No. 221-A,
Kajori Mohalla,
Jalandhar.

2. The Punjab School Education Board has entered your name wrongly on your certificate. Write an application to the Secretary of the Board giving your correct name and requesting him to make necessary changes in the Board records.

333, Jhoolna Mahal,
Gurdaspur.
June 23, 20……

To
The Secretary,
The Punjab School Education Board,
S.A.S. Nagar, Mohali.
Sir,

With due respect I like to bring the following facts to your kind notice for prompt action.

I, Raminder Singh Bajwa, son of S. Krishan Singh Bajwa, resident of Gurdaspur qualified my Matriculation Examination in March 2002. I appeared under roll number 233222. I wrote my name correctly in the admission form. I regret to bring to your kind notice that my name has been wrongly registered as Rajinder Singh in place of Raminder Singh Bajwa in the certificate issued to me. This has involved me in unnecessary and unavoidable correspondence with the Board authorities. Your office clerks insist on writing my name as Rajinder Singh Bajwa as they do not like to take the trouble of correcting their own error. For this lapse and negligence of the Board clerks, I have failed to get my matriculation certificate in my correct name.

I am enclosing an affidavit affirming that I am Raminder Singh Bajwa and not Rajinder Singh Bajwa. You are requested to personally look into the matter and get the error corrected. You are also requested to arrange to issue a fresh certificate with my correct name as Raminder Singh Bajwa.
Thanking you,

Yours faithfully,
Raminder Singh Bajwa

3. Write a letter/application to D.C. of your district requesting him to open a dispensary in your town.

To
The Deputy Commissioner,
Gurdaspur.
Sir,

We, the residents of Fatehgarh Churian, Tehsil Batala, lay down the following for your kind perusal and prompt action.

Fatehgarh Churian is a small town situated at a distance of about 15 kilometres from Batala and 25 kilometres from Gurdaspur. It is a growing town and its present population is about ten thousand people. It has no government hospital or dispensary worth the name. In case of an emergency, the. residents of the town have to go to Gurdaspur or Batala for hospitalisation. It becomes very difficult to provide first aid to a needy person. Recently, five women and three children who had been suffering from cholera died for want of medical attention.

We, the residents of the town, shall be grateful to you if you kindly arrange to open a dispensary. A town-dweller who has settled in Canada has agreed to pay the price of two acres of land and the cost of the construction of the building.

Thanking you in anticipation,

Yours faithfully,
Naresh Malik
and ten others,
Fatehgarh Churian.

4. Write a letter/application to the Postmaster of your town for the change of address.

12, Model Town,
Jalandhar City.
April 18, 20….

To
The Postmaster,
Central Town,
Jalandhar.
Sir,

I want to bring to your kind notice that I have shifted my residence from K-44, Central Town to 12-Model Town.

I had to shift my belongings in a hurry. I could not inform my friends and relatives. It is possible that some of my letters might be written at the previous address. It is, therefore, requested that my letters may kindly be redirected on my new address. Thanking you,

Yours faithfully,
Rajesh Kumar

5. Write a letter to the Postmaster General for providing a post office in your village.

Village Sanghar,
P.O. Dhariwal,
District Gurdaspur.
May 15, 20 ……..

To
The Postmaster General,
Ambala.
Sir,

I, on behalf of the inhabitants of village Sanghar, would like to bring to your kind notice that there is no post office in our village which is growing everyday.

The villagers have to face many hardships because there is no post office within a radius of three kilometres. This village has a number of young men working in the defence services. Villagers have to go a long way for sending their telegrams, parcels and letters. Moreover, sometimes the most urgent messages and telegrams do not reach our village in time. The result is that villagers are put to a lot of inconvenience and hardship.

Under such circumstances, it becomes imperative to open a post office in our village. I, therefore, request you earnestly to provide a post office in our village. We are ready to donate land for the post office building.

Thanking you,

Yours faithfully,
Sarpanch,Village Sanghar,
Post Office Dhariwal,
District Gurdaspur

6. You are Ramnik Singh. Write an application to the Manager of a firm for the post of a clerk.

22, Putli Ghar,
Amritsar.
April 22, 20 ………

To
The Manager,
Bird and Company, Amritsar.
Sir,

This is in response to your advertisement in ‘The Tribune’ dated 24th March, 20…… for the post of a clerk in your office. I beg to offer myself as a candidate for the same. As regards my qualifications, they are detailed below:

I passed my B.A. I examination in 20…….. as a regular student of D.A.V. College, Jalandhar in the second division. Then I joined a commercial college where I learnt both typewriting and shorthand. I can type in English, Hindi and Punjabi. My speed in shorthand is 80 w.p.m. My speed in typewriting in three languages is 50 w.p.m. I have been working as a clerk for the last two years. I am well-conversant with other official works and can speak English, Hindi and Punjabi fluently.

I am still in the service of the same firm. I seek better prospects which my present employer, with his limited business, cannot supply. He has no objection to my seeking a better job somewhere else.

I am a hardworking young man with a sound body and good experience. I enclose a certificate from my present employer about my character, experience and ability. Should you appoint me to the post, it would be my sincerest effort to give you every satisfaction.

Yours faithfully,
Ramnik Singh

7. Imagine you are Rohan. Write a letter to the Secretary, the Punjab School Education Board, Mohali requesting him to declare your result.

22, Urban Estate,
Batala.
March 29, 20…….

To
The Secretary,
The Punjab School Education Board,
Mohali.
Sir,

With due respect I beg to say that I appeared in the 10 + 2 examination of the Punjab School Education Board in March 20 ……. with roll number 231212 as a regular student of Government College, Gurdaspur. Even after the passing of a year, my result has not been declared. In the absence of the result I could not get admission in any of the educational institutions. I have suffered because of the failure of the Board to declare my result. This is despite the fact that I wrote three registered letters on the subject.

Kindly do me the favour of declaring my result without further delay. Thanking you in anticipation,

Yours faithfully,
Rohan

8. Write a letter/application to the Postmaster General, concerning a lost money order.

15, Netaji Road,
Amritsar.
25th August, 20……

To
The Postmaster General,
Ambala.
Sir,

About a month ago, I sent a money order for Rs 200 to my brother Dr. R. L. Kapoor, 15-Park Road, Jodhpore. This should ordinarily have reached him by September 1st at the latest. But so far he has not received it. I have made enquiries several times at the local post office, where I booked the money order, but they are unable to tell me what has become of it.

I have been highly inconvenienced through what appears to be the carelessness of the postal authorities. I should be much obliged if you look into the matter immediately. The number of the money order receipt is 1221; it is dated Sept. 1, 20 …….. and stamped Amritsar, P.O.

Yours faithfully,
Gunwant Singh

9. Write a letter to the Postmaster requesting him to enquire why a parcel sent to you has not been delivered as yet.

124, Model Town,
Gurdaspur.
June 10, 20…….

To
The Postmaster,
Gurdaspur.
Sir,

I want to bring to your notice the non-delivery of a parcel of books to me.

M/s Shyam Lai and Co., New Delhi, sent me a parcel of books on 4th April. It was posted at Chanakyapuri Post Office. It was a registered parcel bearing Registration No. 01396. It was to be delivered to me by the 9th of April. But even after a lapse of almost two months the parcel has not been delivered to me. It contained some very important books which I had ordered because I am preparing for the ensuing UPSC exam. The delay has upset all my plans and will certainly affect my career badly.

I request you to trace the parcel and deliver it to me as early as possible.
Expecting an early action.

Yours faithfully,
Rakesh Puri

10. Write a letter/application to the Commissioner of Jalandhar Division requesting him to get a sports stadium built at Gurdaspur.

110, Jail Road,
Gurdaspur.
June 20, 20 ……

To
The Commissioner,
Jalandhar Division,
Jalandhar.
Sir,

I like to bring to your notice that ours is a growing town with several facilities. The only thing lacking here is a sports stadium.

The residents of this town feel that we should have a sports stadium. The reason for this is simple. The state wrestling championship has been won by a wrestler from this town. Even at the district level wrestling competition, the wrestlers of Gurdaspur have done very well. Besides, we have several district and state level athletes. Nitish Kumar and Harjinder Singh represented the state at a swimming competition. Some of the first class sportsmen have joined the Navy and the Indian army. Some of the girls have also distinguished themselves in some of the disciplines.
A rich landlord has agreed to donate three acres of land for the purpose.

I also know that you are a lover of sports. I am sure that you would leave no stone unturned to give a practical shape to this proposal.

Yours faithfully,
Makhan Singh and ten other sports lovers.

11. Write a letter to the President of the Municipal Committee of your town about the unsatisfactory position of street lighting on the streets and lanes of your town.

202, Murgai Mohalla,
Dinanagar.
July 25, 20…….

To
The President,
Municipal Committee,
Dinanagar.
Sir,

With due respect, I like to bring to your kind notice the extremely unsatisfactory position of street lighting in the narrow lanes and streets of Murgai Mohalla.

Murgai Mohalla is a neglected area so far as street lighting is concerned. As night falls, people are put to a lot of inconvenience because of absence of tubes and lights on the poles meant for that purpose. Absence of street lighting encourages anti-social people. The instances of eve-teasing are on the increase. Young women and girls who happen to return home soon after sunset are victims of molestation and groping. Rowdies take advantage of the prevailing darkness.

Petty thefts are also on the increase. On two or three occasions, two old women fractured their legs as they fell into some of the pits in the lanes. It is impossible to go out of one’s home even in an emergency because of the pitch dark streets. We brought the matter to the notice of the local Municipal Commissioner who promised to do something. Unfortunately he is lying in a hospital at Amritsar. You are requested to see the situation for yourself and get adequate tubes and bulbs fitted in the area.

Yours faithfully.
Chetan Rathore

12. Write a letter to the Director, Doordarshan Kendra, suggesting some improvements in the TV Programmes.

18, Chopra House,
Gali Khazanchian,
Kapurthala.
May 28, 20……..

To
The Director,
Doordarshan Kendra,
Jalandhar.
Sir,

I wish to draw your attention to the deteriorating quality of television programmes telecast from your Kendra. They are cheap, dull, boring and not at all entertaining or informative. They do not cater to the needs of youth and housewives.

Most of the programmes are meant for the rural people who do not have any inclination to watch such dull and boring programmes.

The Kendra should start some programmes to attract young men and women who have some artistic genius in them. They should be. given opportunities to show their talent.

Even the plays telecast from your Kendra are sub-standard. They have no originality about them. The incharge of your plays should be asked to monitor plays telecast from Pakistan Television. They are realistic and have the human element in them. The plays telecast by Jalandhar Doordarshan are of a very poor quality. They hardly have any theme. Your station.must take the cue from PTV.

There must be a programme on general awareness for the benefit of those Punjab students who want to have a career outside the state. Some quiz programmes should be telecast. Young men and women with better performance should be encouraged through prizes.

A panel of distinguished artists, singers and educationists should be asked to monitor the quality of programmes from your station. I think these measures will go a long way in improving the programmes telecast by your Kendra.

Yours faithfully,
Akanksha Chopra

13. Suppose you are Sandeep. Write a letter to the Postmaster of your town complaining against the irregular delivery of your letters.

222, Urban Estate,
Jalandhar.
July 22, 20 ………..

To
The Postmaster,
Jalandhar.
Dear Sir,

I regret to bring to your kind notice that the delivery of letters in our area is very very irregular. The postman, Mr. Joginder Singh, is not performing his duty properly. He is very careless in delivering the letters. He is irregular also. On some Saturdays he does not deliver the letters. On days before other holidays, he fails to deliver the letters.

Sometimes he hands over letters to children asking them to deliver them to the addresses. His attitude to his duty is casual and irresponsible. This careless approach to duty often leads to the loss of some important letters. If urgent letters are not delivered in time, they cannot be responded to in time. I have warned him on several occasions but he does not seem to be bothered.

He is very rude, hie does not know how to respond politely to the people. You are requested to ask for his explanation about his irresponsible and casual attitude to the delivery of letters. It would be appreciated if he is suspended or warned to mend himself.

Yours faithfully,
Sandeep

Applications for Different Jobs

14. Write an application for the post of a teacher in a school.

12, Canal Park,
Dhariwal.
April 15, 20 …….

To
The Principal,
Khalsa Higher Secondary School,
Burj Sahib (Dhariwal).
Sir,

With reference to your advertisement in the ‘Daily Tribune’ of April 14, 20……. for the post of a science teacher in your school, I beg to offer myself as a candidate for the post.

As regards my qualifications, I may state that I passed the B.Sc. (Non-medical) from the G.N.D.U. as a regular student of Baring Union Christian College, Batala in 20…. securing 65% marks in the first division. Thereafter, I did my B.Ed. training from the Janata Teachers Training College, Nakodar in first division in the year 20…. At present, I am working as a science teacher in a village high school.

I have a special aptitude for teaching. During my college days, I took keen interest in extra-curricular activities. I was a member of the College Dramatic Society and also an active member of the College Music Club.

I can teach very proficiently. A testimonial from my present Principal speaks volumes for my ability and performance. If I am given a chance to serve in your school, I promise to give a good account of myself as a science teacher.
Thanking you,

Yours faithfully,
Raj an Tandon

15. Write an application to your Principal requesting him to condone your deficiency/ shortage in lectures. Give reasons.

To
The Principal,
Baring Union Christian College,
Batala.
Sir,

With due respect I beg to state that I am a student of + 2 class of your college. During the month of November, I fell seriously ill and was admitted in a local nursing home for treatment. I had to remain in the nursing home for four weeks. So, I could not attend my classes. As a result, I have fallen short of the required percentage of lectures to be eligible to appear in the annual examination. I may add that I did very well in my September House Examination. I secured more than 65% marks in all the subjects. I am sure that I shall do very well in my final examination also. Kindly condone my shortage of lectures and enable me to appear in the final examination.
Thanking you,

Yours faithfully,
Swaroop Krishan Bhalla
Roll No : 1202
10 + 2

Dated : March 10, 20……

16. Write an application to the Principal of your school / college for remission of fine.

To
The Principal,
A.B. College,
Pathankot.
Sir,

Most respectfully I beg to state that I have been fined Rs 100 for reaching the college late on three consecutive days. I request you to remit the fine as I got late due to unavoidable circumstances.

My mother met with an accident. I had to take her to a local nursing home. For the three days on which I got late, there was none at home to take her to the nursing home. Hence it was not possible for me to neglect the treatment of my mother. To support my reason, I am enclosing a certificate from the doctor that I used to bring my mother to the clinic for treatment at 10 o’ clock in the morning for the three days in question.

I have placed the facts before you. I was late no doubt, but I was helpless. So, I request you to remit my fine. I shall be thankful to you for this act of kindness.

Yours faithfully,
Saurabh Kumar
Roll Number 1203
XII B

Dated : April 19, 20……

17. Write an application for the post of a steno-typist.

14, Civil Lines,
Jalandhar City.
August 6, 20…..

To
The Advertiser,
Post Box No. 313,
The Tribune,
Chandigarh.
Sir,

This is in response to your advertisement in “The Tribune” dated 4th August, 20….. for the post of a steno-typist in your office. I beg to offer myself as a candidate for the same. As regards my qualifications, they are detailed below:

I passed my B.A. Examination in 20….. from D.A.V. College, Jalandhar in the second division. After my graduation, I joined a commercial college where I learnt both shorthand and type-writing. My speed in shorthand is 75 w.p.m. and my speed in type-writing is 50 w.p.m. I have been working as a stenotypist with Sehgal & Co., Jalandhar for the last five years. Besides shorthand and type-writing, I am also well conversant with other official work and can speak English, Hindi and Punjabi fluently.

I am still in the service of the same firm. I am seeking better opportunities which my present employer, with his limited, business, cannot supply. He has no objection to my seeking a better job somewhere else.

I am a young man of 25 with a sound body and a good appearance. I enclose a certificate from my present employer about my character, experience and ability.

Should you appoint me to the post, it would be my sincerest effort to give you every satisfaction.

Yours faithfully,
H.M.L. Sood

18. Write a letter to your Principal for a testimonial / character certificate.

2652, Sector 37 C,
Chandigarh.
August 10, 20 …..

To
The Principal,
Government College,
Gurdaspur.
Sir,

Respectfully I request you to kindly issue me a character certificate. I am required to produce it when applying for the post of a teacher.

I was a student of the college from 2001 to 2004 and passed the B.A. examination in April, 2004 securing first division marks with distinction in Mathematics. I was a member of the Students’ Central Association and the President of the Debating and Speakers Club. I was President of the English Speech Society. Throughout my college career, I remained in the good books of my worthy teachers.

My Roll No. in BA. final was 1305 and I was under the tutorship of Prof. Akash Malhotra who will testify to the truth of the facts stated above.
Kindly issue the character-cum-merit certificate at the earliest and oblige.
Thanking you in anticipation,

Yours faithfully,
Arvind Bhandari

19. Write an application to the Principal of your school / college complaining against the poor quality of food being supplied to you at the school hostel.

To
The Principal,
Government Higher Secondary School,
Bhatinda.
Sir,

I regret to inform you that the food served in the hostel is the most unhygienic and of poor quality. Of course, the Kitchen Committee is there to monitor the quality of food served but the Mess Manager is more than a match for the Kitchen Committee.

Pure milk and curd are never served in the hostel. There is the Government dairy where pure milk is readily available but the Mess Manager buys it from Gujjars who are known for diluting milk with water.

The cooked food is no better. The vegetables that are cooked are often stale and rotten. They are cooked in sub-standard or inferior quality of edible oil. Most of the boarders suffer from cough and bad throat. The Mess Manager buys sub-standard quality of rice and wheat atta. We find small pieces of stone and some foreign matter in chapaties and cooked rice. Very often the boarders complain of dysentery and other stomach disorders.

It is high time that things are set right by improving the quality of food served in the hostel. The Mess Manager must be taken to task. He must be told that the quality of food supplied by him to the boarders is telling upon their health. He should be warned to improve things immediately otherwise he should be asked to leave.

Yours faithfully,
Rajesh Kohli
XI
Govt. School Hostel
Bhatinda.

20. Write a letter to the Superintendent of Police pointing out the increase in the incidents of thefts in your locality.

15, Garden Colony,
Patti.
February 22, 20 ……..

To
The Superintendent of Police,
Patti.
Dear Sir,

I am writing this to invite your kind attention to the rising incidence of thefts in our locality, Garden Colony. This area has become a paradise for thieves and burglars. This locality is continuing to develop. Some of the roads are deserted especially in the afternoon. Hardly a day passes without an incident of theft. The worrisome point is that these incidents occur in the broad daylight when women-folk in their homes retire for rest after their household work.

Since it is a developing colony, labourers and strangers are seen moving around in a suspicious manner. Of course, there are a couple of policemen on guard near the entrance of the colony but they rarely patrol the colony. The S.H.O of the area has been kept informed about the incidents of thefts but there is no improvement in the situation. The residents of the locality are beginning to lose faith in the junior officials. Hence your personal attention is required to the problem facing the colony.
Thanking you,

Yours faithfully,
P.K. Khanna