Bhagya

Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 12 Exponents and Powers Ex 12.2 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 8 Maths Chapter 12 Exponents and Powers Ex 12.2

1. Express the following numbers in standard form:

Question (i)
0.0000000000085
Solution:
= \(\frac{85}{10000000000000}\)
= \(\frac{85}{10^{13}}\)
= \(\frac{8.5 \times 10}{10^{13}}\)
= 8.5 × 10 × 10^-13
= 8.5 × 10^-12

Question (ii)
0.00000000000942
Solution:
= \(\frac{942}{100000000000000}\)
= \(\frac{942}{10^{14}}\)
= \(\frac{9.42 \times 10^{2}}{10^{14}}\)
= 9.42 × 10² × 10^-14
= 9.42 × 10^-12

Question (iii)
6020000000000000
Solution:
= 602 × 10000000000000
= 602 × 10¹³
= 6.02 × 10² × 10¹³
= 6.02 × 10¹⁵

Question (iv)
0.00000000837
Solution:
= \(\frac{837}{100000000000}\)
= \(\frac{837}{10^{11}}\)
= \(\frac{8.37 \times 10^{2}}{10^{11}}\)
= 8.37 × 10² × 10^-11
= 8.37 × 10^2+(-11)
= 8.37 × 10^-9

Question (v)
31860000000
Solution:
= 3186 × 10000000
= 3186 × 10⁷
= 3.186 × 10³ × 10⁷
= 3.186 × 10^{3 + 7}
= 3.186 × 10¹⁰

2. Express the following numbers in usual form:

Question (i)
3.02 × 10^-6
Solution:
= 302 × 10^-2 × 10^-6
= 302 × 10^-8
= \(\frac{302}{100000000}\)
= 0.00000302

Question (ii)
4.5 × 10⁴
Solution:
= \(\frac {45}{10}\) × 10000
= 45 × 1000
= 45000

Question (iii)
3 × 10^-8
Solution:
= \(\frac{3}{100000000}\)
= 0.00000003

Question (iv)
1.0001 × 10⁹
Solution:
= \(\frac{10001}{10000}\) × 1000000000
= 10001 × 100000
= 1000100000

Question (v)
5.8 × 10¹²
Solution:
= \(\frac {58}{10}\) × 100000000000
= 58 × 10000000000
= 5800000000000

Question (vi)
3.61492 × 10⁶
Solution:
= \(\frac{361492}{100000}\) × 1000000
= 361492 x 10
= 3614920

3. Express the number appearing in the following statements in standard form:

Question (i)
1 micron is equal to \(\frac{1}{1000000}\) m.
Solution:
1 micron = \(\frac{1}{1000000}\)m
= \(\frac{1}{10^{6}}\)m
∴ 1 micron = 1 × 10^-6 m

Question (ii)
Charge of an electron is 0.000,000,000,000,000,000,16 coulomb.
Solution:
Charge of an electron
= 0.000,000,000,000,000,000,16 coulomb
= \(\frac{16}{100000000000000000000}\) coulomb
= \(\frac{1.6 \times 10}{10^{20}}\) coulomb
= \(\frac{1.6}{10^{19}}\) coulomb
= 1.6 × 10^-19 coulomb
∴ Charge of an electron = 1.6 × 10^-19 coulomb

Question (iii)
Size of a bacteria is 0.0000005 m.
Solution:
Size of a bacteria = 0.0000005 m
= \(\frac{5}{10000000}\)m
= \(\frac{5}{10^{7}}\)m
= 5 × 10^-7 m
∴ Size of a bacteria = 5 × 10^-7 m

Question (iv)
Size of a plant cell is 0.00001275 m.
Solution:
Size of a plant cell = 0.00001275 m
= \(\frac{1275}{100000000}\)m
= \(\frac{1275}{10^{8}}\)m
= \(\frac{1.275 \times 10^{3}}{10^{8}}\) m
= 1.275 × 10^3-8
= 1.275 × 10^-5
∴ Size of a plant cell
= 1.275 × 10^-5 m

Question (v)
Thickness of a thick paper is 0.07 mm.
Solution:
Thickness of a thick paper = 0.07 mm
= \(\frac {7}{100}\) mm
= \(\frac{7}{10^{2}}\) mm
= 7 × 10^-2 mm
∴ Thickness of a thick paper
= 7 × 10^-2 mm

4. In a stack there are 5 books each of thickness 20 mm and 5 paper sheets each of thickness 0.016 mm. What is the total thickness of the stack.
Solution:
Thickness of a book = 20 mm
∴ Thickness of 5 books = (5 × 20) mm
= 100 mm
Thickness of a paper sheet = 0.016 mm
∴ Thickness of 5 paper sheets
= (5 × 0.016) mm
= 0.08 mm
∴ Total thickness of a stack = Thickness of books + Thickness of paper sheets
= (100 + 0.08) mm
= 100.08 mm
In standard form 100.08 is written as 1.0008 × 10².
Thus, the total thickness of the stack is 1.0008 × 10² mm.

Punjab State Board PSEB 10th Class Maths Book Solutions Chapter Statistics Ex 14.1 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 10 Maths Chapter 14 Statistics Ex 14.1

Question 1.
A survey was conducted by a group of students as a part of their enviroment awareness programme, in which they collected the following data regarding the number of plants in 20 houses in a locality. Find the mean number of plants per house.

Which method did you use for finding the mean, and why?
Solution:
Since, number of plants and houses are small in their values; so we must use direct method

Mean X = \(\overline{\mathrm{X}}=\frac{\Sigma f_{i} x_{i}}{\Sigma f_{i}}\)

\(\frac{162}{20}\) = 8.1

Hence, mean number of plants per house is 8.1.

Question 2.
Consider the following distribution of daily wages of 50 workers of a factory.

Find the mean daily wages of the workers of the factory by using an appropriate method.
Solution:

From given data,
Assumed Mean (a) = 150
and width of the class (h) = 20
∴ \(\bar{u}=\frac{\Sigma f_{i} u_{i}}{\Sigma f_{i}}\)
= \(\frac{-12}{50}\) = – 0.24

Using formula, Mean \((\overline{\mathrm{X}})=a+h \bar{u}\)
= 150 + (20) (- 0.24)
= 150 – 4.8 = 145.2
Hence,mean daily wages of the workers of factory is ₹ 145.20.

Question 3.
The following distribution shows the daily pocket allowance of children of a locality. The mean pocket allowance is 18. Find the missing frequency f.

Solution;

From the above data,
Assumed mean (a) = 18
Using formula, Mean \((\overline{\mathrm{X}})=a+\frac{\sum f_{i} d_{i}}{\Sigma f_{i}}\)

\(\bar{X}=18+\frac{2 f-40}{44+f}\)
But. Mean of data \((\bar{x})\) = 18 …(Given)
∴ 18 = 18 + \(\frac{2 f-40}{44+f}\)
or \(\frac{2 f-40}{44+f}\) = 18 – 18 = 0
or 2f – 40 = 0
or 2f = 40
or f = \(\frac{40}{2}\) = 20
Hence, missing frequency f is 20.

Question 4.
Thirty women were examined in a hospital by a doctor and the number of heart beats per minute were recorded and summarised as follows. Find the mean heart beats per minute for these women, choosing a suitable method.

Solution:

From above data,
Assumed Mean (a) = 75.5
Width of Class (h) = 3
∴ \(\bar{u}=\frac{\sum f_{i} u_{i}}{\sum f_{i}}\)
= \(\frac{4}{30}\) (approx.)
Using formula,
Mean \((\overline{\mathrm{X}})=a+h \bar{u}\)
= 75.5 + 3 (0.13) = 75.5 + 0.39
\(\overline{\mathrm{X}}\) = 75.89
Hence, mean heart beats per minute for women is 75.89.

Question 5.
In a retail market, fruit vendors were selling mangoes kept in packing boxes. These boxes contained varying number mangoes. The following was the distribution of mangoes according to the number of boxes.

Find the mean number of mangoes kept in a packing box. Which method of finding the mean did you choose?
Solution:
Since, values of number of mamgoes and number of boxes are large numerically. So, we must use step-deviation method.

From above data,
Assumed Mean (a) = 57
Width of class (h) = 3
∴ \(\bar{u}=\frac{\sum f_{i} u_{i}}{\sum f_{i}}\)

\(\bar{u}=\frac{25}{400}\) = 0.0625

Using formula, Mean \((\overline{\mathrm{X}})=a+h \bar{u}\)
\(\bar{X}\) = 57 + 3(0.0625)
= 57 + 0.1875 = 57.1875 = 57.19.
Hence, mean number of mangoes kept in a packing box is 57.19.

Question 6.
The table below shows the daily expenditure on food of 25 households in a locality.

Find the mean daily expenditure on food by a suitable method.

Solution:

From above data,
Assumed mean (a) = 225
Width of class (h) = 50
∴ \(\bar{u}=\frac{\Sigma f_{i} u_{i}}{\Sigma f_{i}}\)

\(\bar{u}=-\frac{7}{25}\) = 0.28
Using formula,
Mean \((\overline{\mathrm{X}})=a+h \bar{u}\)
\(\bar{X}\) = 225 + 50 (- 0.28)
\(\bar{X}\) = 225 – 14
\(\bar{X}\) = 211
Hence, mean daily expenditure on food is ₹ 211.

Question 7.
To find out the concentration of SO₂ in the air (in parts per million, e., ppm), the data was collected for 30 localities in a certain city and is presented below:

Find the mean concentration of SO₂ in the air.
Solution:

From above data,
Assumed mean (a) = 0.10
Width of class (h) = 0.04
∴ \(\bar{u}=\frac{\Sigma f_{i} u_{i}}{\Sigma f_{i}}\)

\(\bar{u}=-\frac{1}{30}\) = – 0.33(approx.)
Using formula, Mean \((\overline{\mathrm{X}})=a+h \bar{u}\)
\(\bar{X}\) = 0.10 + 0.04 (- 0.33)
= 0.10 – 0.0013 = 0.0987 (approx.)
Hence, mean concentration of SO₂ in the air is 0.0987 ppm.

Question 8.
A class teacher has the folloing absentee record of 40 students of a class for the whole term. Find the mean number of days a student was absent.

Solution:

From above data,
Assumed Mean (a) = 17
Using formula, Mean(\(\bar{X}\)) = a + \(\frac{\Sigma f_{i} d_{i}}{\Sigma f_{i}}\)
\(\bar{X}\) = 17 + \(-\frac{181}{40}\)
= 17 – 4.52 = 12.48.
Hence, mean 12.48 number of days a student was absent.

Question 9.
The following table gives the literacy rate (in percentage) of 35 citIes. Find the mean literacy rate.

Solution:

From the above data,
Assumed Mean (a) = 70
Width of class (h) = 10
∴ \(\bar{u}=\frac{\Sigma f_{i} u_{i}}{\Sigma f_{i}}=\frac{-2}{35}\) = – 0.057

Using formula, Mean \((\overline{\mathrm{X}})=a+h \bar{u}\)
\(\bar{X}\) = 70 + 10 (- 0.057)
= 70 – 0.57 = 69.43
Hence, mean literacy rate is 69.43%.

Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 12 Exponents and Powers Ex 12.1 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 8 Maths Chapter 12 Exponents and Powers Ex 12.1

1. Evaluate:

Question (i)
3^-2
Solution:
= \(\frac{1}{3^{2}}\)
= \(\frac{1}{3 \times 3}\)
= \(\frac {1}{9}\)

Question (ii)
(-4)^-2
Solution:
= \(\frac{1}{(-4)^{2}}\)
= \(\frac{1}{(-4) \times(-4)}\)
= \(\frac {1}{9}\)

Question (iii)
(\(\frac {1}{2}\))^-5
Solution:
= \(\frac{1}{2} \times \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2} \times \frac{1}{2}\)
= \(\frac{1}{\frac{1}{32}}\)
= 32

2. Simplify and express the result in power notation with positive exponent:

Question (i)
(-4)⁵ ÷ (-4)⁸
Solution:
= (-4)^{5 – 8} (∵ a^m ÷ aⁿ = a^m-n)
= (-4)^{– 3}
= \(\frac{1}{(-4)^{3}}\)

Question (ii)
\(\left(\frac{1}{2^{3}}\right)\)²
Solution:
= \(\frac{(1)^{2}}{\left(2^{3}\right)^{2}}\) [∵ \(\left(\frac{a}{b}\right)^{m}=\frac{a^{m}}{b^{m}}\)]
= \(\frac{1}{2^{3 \times 2}}\) [∵ (a^m)ⁿ = a^mn
= \(\frac{1}{2^{6}}\)

Question (iii)
(-3)⁴ × (\(\frac {5}{3}\))⁴
Solution:
= [(-3) × \(\frac {5}{3}\)]⁴ [∵ a^m × b^m = (ab)^m]
= [(-1) × 5]⁴
= (-1)⁴ × 5⁴
= 1 × 5⁴
= 5⁴

Question (iv)
(3^-7 ÷ 3^-10) × 3^-5
Solution:
= (3^(-7)-(-10)) × 3^-5 (∵ a^m ÷ aⁿ = a^m-n)
= (3^-7+10 × 3^-5
= 3³ × 3^-5
= 3³ + (-5) (∵ a^m × aⁿ = a^m+n)
= 3^-2
= \(\frac{1}{3^{2}}\)

Question (v)
2^-3 × (-7)^-3
Solution:
= [2 × (-7)]^-3 [∵ a^m × b^m = (ab)^m
= (-14)^-3
= \(\frac{1}{(-14)^{3}}\)

3. Find the value of:

Question (i)
(3⁰ + 4^-1) × 2²
Solution:
= (1 + \(\frac {1}{4}\)) × 2²
= (\(1 \frac{1}{4}\)) × 2²
= (\(\frac {5}{4}\)) × 4
= 5

Question (ii)
(2^{– 1} × 4^-1) ÷ 2²
Solution:
= (\(\frac {1}{2}\) × \(\frac {1}{4}\)) ÷ \(\frac{1}{2^{2}}\)
= (\(\frac {1}{8}\)) ÷ \(\frac {1}{4}\)
= \(\frac {1}{8}\) × \(\frac {4}{1}\)
= \(\frac {1}{2}\)

Question (iii)
(\(\frac {1}{2}\))^{– 2} + (\(\frac {1}{3}\))^{– 2} + (\(\frac {1}{4}\))^{– 2}
Solution:
=\(\frac{1}{\left(\frac{1}{2}\right)^{2}}+\frac{1}{\left(\frac{1}{3}\right)^{2}}+\frac{1}{\left(\frac{1}{4}\right)^{2}}\)
= \(\frac{1}{\frac{1}{4}}+\frac{1}{\frac{1}{9}}+\frac{1}{\frac{1}{16}}\)
= 4 + 9 + 16
= 29

Question (iv)
(3^{– 1} + 4^{– 1} + 5^{– 1})⁰
Solution:
(3^-1 + 4^-1 + 5^-1)⁰
∴ [3^-1 + 4^-1 + 5^-1]⁰ = 1
[∵ a⁰ = 1]

Question (v)
\(\left\{\left(\frac{-2}{3}\right)^{-2}\right\}\)²
Solution:
= \(\left(\frac{-2}{3}\right)^{(-2) \times 2}\) [∵ (a^m)^m = a^mn]
= (\(\frac {-2}{3}\))^-4
= \(\frac{(-2)^{-4}}{(3)^{-4}}\) [∵ \(\left(\frac{a}{b}\right)^{m}=\frac{a^{m}}{b^{m}}\)]
= \(\frac{3^{4}}{(-2)^{4}}\)
= \(\frac{3 \times 3 \times 3 \times 3}{(-2) \times(-2) \times(-2) \times(-2)}\)
= \(\frac {81}{16}\)

4. Evaluate:

Question (i)
\(\frac{8^{-1} \times 5^{3}}{2^{-4}}\)
Solution:
= \(\frac{2^{4} \times 5^{3}}{8}\)
= \(\frac{2^{4} \times 5^{3}}{2^{3}}\)
= 2^4-3 × 5³
= 2 × 125
= 250

Question (ii)
(5^-1 × 2^-1) × 6^-1
Solution:
= (\(\frac {1}{5}\) × \(\frac {1}{2}\)) × \(\frac {1}{6}\)
= (\(\frac {1}{10}\)) × \(\frac {1}{6}\)
= \(\frac {1}{60}\)

Another method:
= 5^-1 × 2^-1 × 6^-1
= (5 × 2 × 6)^-1
= (60)^-1
= \(\frac {1}{60}\)

5. Find the value of m for which 5^m ÷ 5^-3 = 5⁵.
Solution:
∴ 5^m-(-3) = 5⁵.
∴ 5^{m + 3} = 5⁵
∴ m + 3 = 5 (∵ a^m = aⁿ then m = n)
∴ m = 5 – 3
∴ m = 2
Thus, value of m is 2.

6. Evaluate:

Question (i)
\(\left\{\left(\frac{1}{3}\right)^{-1}-\left(\frac{1}{4}\right)^{-1}\right\}^{-1}\)
Solution:
= {\(\frac{3}{1}-\frac{4}{1}\)}^-1 (∵ a^-m = \(\frac {1}{a^m}\))
= {3 – 4}^-1
= {-1}^-1
= \(\frac {1}{-1}\)
= -1

Question (ii)
(\(\frac {5}{8}\))^-7 × (\(\frac {8}{5}\))^-4
Solution:
(\(\frac {5}{8}\))^-7 × (\(\frac {5}{8}\))⁴
(∵ a^-m = \(\frac{1}{a^{m}}\))
= (\(\frac {5}{8}\))^-7+4 (∵ a^m × aⁿ = a^m+n)
= (\(\frac {5}{8}\))^-3
= (\(\frac {8}{5}\))³
= \(\frac{8 \times 8 \times 8}{5 \times 5 \times 5}\)
= \(\frac {512}{125}\)

7. Simplify:

Question (i)
\(\frac{25 \times t^{-4}}{5^{-3} \times 10 \times t^{-8}}\) (t ≠ 0)
Solution:

Question (ii)
\(\frac{3^{-5} \times 10^{-5} \times 125}{5^{-7} \times 6^{-5}}\)
Solution:

Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 4 Practical Geometry Ex 4.5 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 8 Maths Chapter 4 Practical Geometry Ex 4.5

Draw the following.

Question 1.
The square READ with RE = 5.1 cm.
Solution:

Steps of construction :

• Draw a line segment RE = 5.1 cm.
• At E, draw \(\overrightarrow{\mathrm{EM}}\), such that ∠REM = 90°.
• With E as centre and radius =5.1 cm, draw an arc intersecting \(\overrightarrow{\mathrm{EM}}\) at A.
• With R as centre and radius = 5.1 cm, draw an arc.
• With A as centre and radius = 5.1 cm, draw an arc which intersect the previous arc at D.
• Draw \(\overline{\mathrm{RD}}\) and \(\overline{\mathrm{AD}}\).

Thus, READ is the required square.

Question 2.
A rhombus whose diagonals are 5.2 cm and 6.4 cm long.
Solution:

[Note : The diagonals of a rhombus bisect each other at right angle.]
Here, in rhombus XYZW, YW 6.4 cm.
∴ OW = OY = 3.2 cm

Steps of construction:

• Draw a line segment XZ = 5.2 cm.
• Draw \(\overleftrightarrow{\mathrm{AB}}\), the perpendicular bisector of \(\overline{\mathrm{XZ}}\), which intersect \(\overline{\mathrm{XZ}}\) at O.
• With O as centre and radius = 3.2 cm, draw an arc intersecting \(\overleftrightarrow{\mathrm{AB}}\) above \(\overline{\mathrm{XZ}}\) at W.
• With O as centre and radius = 3.2 cm, draw another arc which intersects \(\overleftrightarrow{\mathrm{AB}}\) below \(\overline{\mathrm{XZ}}\) at Y.
• Draw \(\overline{\mathrm{XY}}\), \(\overline{\mathrm{YZ}}\), \(\overline{\mathrm{ZW}}\) and \(\overline{\mathrm{XW}}\).

Thus, XYZW is the required rhombus.

Question 3.
A rectangle with adjacent sides of lengths 5 cm and 4 cm.
Solution:

[Note: Each angle of a rectangle is a right angle.]
Steps of construction :

• Draw a line segment AB = 5 cm.
• At A, draw \(\overrightarrow{\mathrm{AX}}\), such that ∠XAB = 90°.
• With A as centre and radius = 4 cm, draw an arc intersecting \(\overrightarrow{\mathrm{AX}}\) at D.
• With B as centre and radius = 4 cm, draw an arc.
• With D as centre and radius = 5 cm, draw an arc which intersects the previous arc at C.
• Draw \(\overline{\mathrm{BC}}\) and \(\overline{\mathrm{CD}}\).

Thus, ABCD is the required rectangle.

Question 4.
A parallelogram OKAY where OK = 5.5 cm and KA = 4.2 cm. Is it unique ?
Solution:

A parallelogram cannot be constructed as sufficient measurements are not given. It is not unique as angles may vary in parallelogram drawn by given measurements.

Punjab State Board PSEB 10th Class Maths Book Solutions Chapter 13 Surface Areas and Volumes Ex 13.5 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 10 Maths Chapter 13 Surface Areas and Volumes Ex 13.5

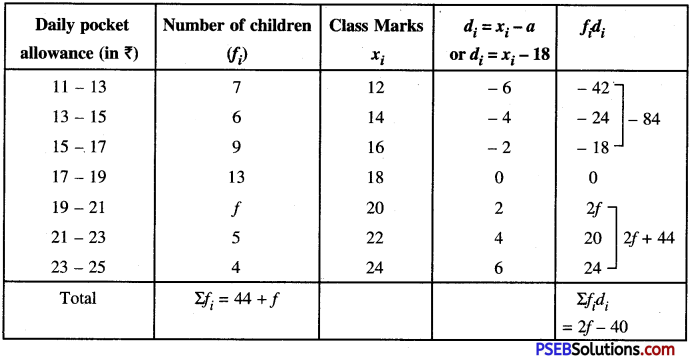
Question 1.
A copper wire 3 mm in diameter is wound about a cylinder whose length is 12 cm, and diameter 10 cm, so as to cover the curved surface of the cylinder. Find the length and mass of the wire, assuming the density of copper to 8.88 g per cm³.
Solution:
Diametcr of wire (d) = 3 mm
∴ Radius of wire (r) = \(\frac{3}{2}\) mm = \(\frac{3}{20}\) cm

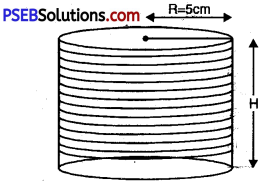
Diameter of cylinder = 10 cm
Radius of cylinder (R) = 5 cm
Height of cylinder (H) = 12 cm
Circumference of cylinder = length of wire used in one turn
2πR = length of wire used in one turn
2 × \(\frac{22}{7}\) × 5 = length of wire used in one turn
\(\frac{220}{7}\) = length of wire used in one turn
Number of turn used = \(\frac{\text { Height of cylinder }}{\text { Diameter of wire }}\)
= \(\frac{12 \mathrm{~cm}}{3 \mathrm{~mm}}=\frac{12}{3} \times 10\) [1 mm = \(\frac{1}{10}\) cm]
= \(\frac{120}{3}\) = 40

∴ length of wire used = Number of turns × length of wire used in one turn
H = 40 × \(\frac{220}{7}\) = 1257.14 cm
Volume of wire used = πr²H
= \(\frac{22}{7} \times \frac{3}{20} \times \frac{3}{20} \times 1257.14\) = 88.89 cm³
Mass of 1 cm³ = 8.88 gm
Mass of 88.89 cm³ = 8.88 × 88.89 = 789.41 gm
Hence, length of wire is 1257.14 cm
and mass of wire is 789.41 gm.

Question 2.
A right triangle, whose sides are 3 cm and 4 cm (other than hypotenuse) is made to revolve about its hypotenuse. Find the volume and surface area the double cone so formed. (Choose value of t as found
appropriate.)
Solution:
Let ∆ABC be the right triangle right angled at A whose sides AB and AC measure 3 cm and 4 cm respectively.
The length of the side BC (hypotenuse) = \(\sqrt{3^{2}+4^{2}}=\sqrt{9+16}\) = 5 cm
Here, AO (or A’O) is the radius of the common base of the double cone formed revolving the right triangle about BC.
Height of the cone BAA’ is BO and slant height is 3 cm.
Height of the cone CAA’ is CO and slant height is 4 cm.
Now, ∆AOB ~ ∆CAB (AA similarity)

∴ \(\frac{\mathrm{AO}}{4}=\frac{3}{5}\)

⇒ AO = \(\frac{4 \times 3}{5}=\frac{12}{5}\) cm

Also \(\frac{\mathrm{BO}}{3}=\frac{3}{5}\)

⇒ BO = \(\frac{\mathrm{BO}}{3}=\frac{3}{5}\) cm
Thus CO = BC – OB
= 5 – \(\frac{9}{5}\) = \(\frac{16}{5}\) cm
Now, Volume of double cone = [Volume of Cone ABA’] + [Volume of cone ACA’]

∴ Volume of double cone = 30 cm³.
Also, surface area of double cone = [Surface area of Cone ABA’] + [surface area of Cone ACA’]
= π .AO.AB + π. AO.A’C
= \(\left(\frac{22}{7} \times \frac{12}{5} \times 3\right)+\left(\frac{22}{7} \times \frac{12}{5} \times 4\right)\)

= \(\left(\frac{792}{35} \times \frac{1056}{35}\right)\)
= \(\frac{1848}{35}\) = 52.75 cm²

Question 3.
A cistern, Internally measuring 150 cm × 120 cm × 110 cm, has 129600 cm3 of water in it. Porous bricks are placed in the water until the cistern is full to the brim. Each brick absorbs one-seventeenth of its own volume of water. How many bricks can be put in without the water overflowing, each brick being 22.5 cm × 7.5 cm × 6.5 cm?
Solution:
Volume of one brick = 22.5 × 7.5 × 6.5 cm³ = 1096.87 cm³
Volume of cistern = 150 × 120 × 110 cm³ = 1980000 cm³
Let number of bricks used = n
Total volume of n bricks = n (volume of one brick) = n [1096.871] cm³
Volume of water = 129600 cm³
Volume of water available for bricks = 1980000 – 129600 = 1850400 cm³

Each bricks absorb \(\frac{1}{17}\)th of its volume in water
Volume of water available for bricks = \(\frac{17}{16}\) × volume of water available for bricks
= \(\frac{17}{10}\) × 1850400
Volume of water available for bricks = 1966050 cm³
Total volume of n bricks = Volume of water available for bricks
n[1096.87] cm³ = 1966050 cm³

n = \(\frac{1966050}{1096.87}\)
n = 1792.42
Hence, Number of bricks used = 1792.

Question 4.
In one fortnight of a given month, there was a rainfall of 10 cm in a river valley. If the area of the valley is 97280 km², show that the total rainfall was approximately equivalent to the addition to the normal water of three rivers each 1072 km long, 75 m wide and 3 m deep.
Solution:
Area of valley = 97280 km²
Rainfall in valley = 10 cm
∴ Volume of total rainfall = 97280 × \(\frac{10}{100}\) × \(\frac{1}{1000}\) km³
= 9.728 km³

Question 5.
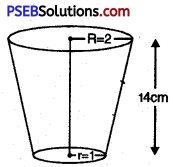
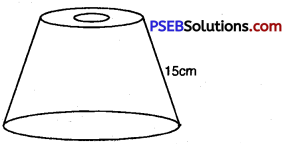
An oil funnel made of tin sheet consists of a cylindrical portion 10 cm long attached to a frust.un of a cone. if the total height is 22 cm, diameter of the cylindrical portion is 8 cm and the diameter of the top of the funnel is 18 cm, find the area of the tin sheet required to make the funnel.

Solution:
Diameter of top of funnel = 18 cm
∴ Radius of top of funnel (R) = \(\frac{18}{2}\) cm = 9 cm
Diameter of bottom of funnel = 8 cm
Radius of bottom of funnel (r) = 4 cm
Height of cylindrical portion (h) = 10 cm
Height of frustum (H) = (22 – 10) = 12 cm
Slant height of frustum (l)
= \sqrt{\mathrm{H}^{2}+(\mathrm{R}-r)^{2}}\(\)

= \(\sqrt{(12)^{2}+(9-4)^{2}}\)

= \(\sqrt{144+(5)^{2}}\)

= \(\sqrt{144+25}\) = \(\sqrt{169}\)
Slant height of frustum (l) = 13 cm
Area of metal sheet required curved
surface area of cylindrical base + curved surface of frustum = 2πrh + πL [R + r]
= 2 × \(\frac{22}{7}\) × 4 × 10 + \(\frac{212}{7}\) × 13 [9 + 4] cm²
= 251.42 + 531.14 = 782.56 cm²

Question 6.
Derive the formula for the curved surface area and total surface area of a frustum of a cone, given to you in Section 13.5, using the symbols as explamed.
Solution:
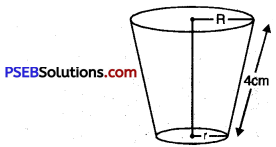
A frustum of a right circular cone has two unequal flat circular bases and a curved surface. Let ACDB be the frustum of the cone obtained by removing the portion VCÐ. The line-segment OP joining the centres of two bases is called the height of the frustum. Each of the line-segments AC and BD of frustum ACDB is called its slant height.

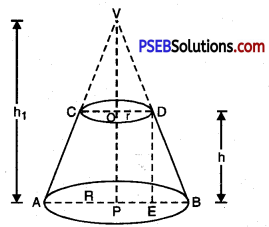
Let R and r be the radii of the circular end faces (R > r) of the frustum ACDB of the cone (V, AB). We complete the conical part VCD. Let h and l be the vertical height and slant height respectively. Then OP = h and AC = BD = l.

The frustum of the right circular cone can be viewed as the difference of the two right circular VAB and VCD.
Let the height of the cone VAB be h₁ and its slant height l₁ i.e. VP = h₁ and VA = VB = l₁.

Now from right ∆DEB, we have
DB² = DE² + BE²

⇒ l² = h² + (R – r)²
⇒ l = \(\sqrt{h^{2}+(\mathrm{R}-r)^{2}}\)
Again ∆VOD ~ ∆VPB

⇒ \(\frac{V D}{V B}=\frac{O D}{P B}\)

⇒ \(\frac{l_{1}-l}{l_{1}}=\frac{r}{R}\)

⇒ 1 – \(\frac{l}{l_{1}}=\frac{r}{\mathrm{R}}\)

⇒ \(\frac{l}{l_{1}}=1-\frac{r}{\mathrm{R}}=\frac{\mathrm{R}-r}{\mathrm{R}}\)

⇒ l₁ = \(\frac{l \mathrm{R}}{\mathrm{R}-r}\)

⇒ Now, l₁ – l₁ = \(\frac{l \mathrm{R}}{\mathrm{R}-r}-l=\frac{l r}{\mathrm{R}-r}\)

Curved surface area of the frustum of cone = πRl₁ – πr(l₁ – l)
[Curved surface area of a cone = π × r × 1]

= πR. \(\frac{l R}{R-r}\) – πr. \(\frac{l r}{\mathrm{R}-r}\)

= πl (\(\frac{\mathrm{R}^{2}-r^{2}}{\mathrm{R}-r}\)) = \(\frac{\pi l(\mathrm{R}-r)(\mathrm{R}+r)}{(\mathrm{R}-r)}\)
= πl (R + r) sq. units.
∴ Curved snafaue area of the frustum of right circular cone = πl (R + r) sq. units,
where l = \(\sqrt{h^{2}+(\mathrm{R}-r)^{2}}\)
and total surface area of frustum of right circular cone = Curved surface area + Area of base + Area of top
= πl (R + r) + πR² + πr²
∴ π [R² + r² + l (R + r)] sq. units.

Question 7.
Derive the formula for the volume of the frustum of a cone, given to you in section 13.5, using the symbols as explained.
Solution:
A frustum of a right circular cone has two unequal flat circular bases and a curved surface. Let ACDB be the frustum of the cone obtained by removing the portion VCD. The line-segment OP joining the centres of two bases is called the height of the frustum. Each of the line-segments AC and BD of frustum ACDB is called its slant height.

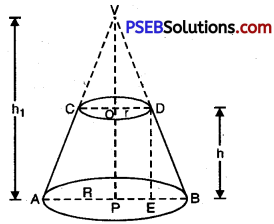
Ler R and r be the radii of the circular end faces (R > r) of the fnistum ACDB of the cone (V, AB). We complete the conical part VCD. Let h and l be the vertical height and slant height respectively. Then OP = h and AC = BD = l. The frustum of the right circular cone can be viewed as the difference of the two right circular VAB and VCD.
Let theheight of the cone VAB be h₁ and its slant height l₁ i.e.VP = h₁ and VA = VB = l₁.
∴ The height of the cone VCD = VP – OP = h₁ – h
Since right ∆s VOD and VPB are similar
⇒ \(\frac{\mathrm{VO}}{\mathrm{VP}}=\frac{\mathrm{OD}}{\mathrm{PB}}=\frac{h_{1}-h}{h_{1}}=\frac{r}{\mathrm{R}}\)

⇒ 1 – \(\frac{h}{h_{1}}=\frac{r}{\mathrm{R}}\)

⇒ \(\frac{h}{h_{1}}=1-\frac{r}{\mathrm{R}}=\frac{\mathrm{R}-r}{\mathrm{R}}\)

⇒ h₁ = \(\frac{h \mathrm{R}}{\mathrm{R}-r}\)

⇒ Height of the cone VCD = \(\frac{h \mathrm{R}}{\mathrm{R}-r}-h=\frac{h \mathrm{R}-h \mathrm{R}+h r}{\mathrm{R}-r}=\frac{h r}{\mathrm{R}-r}\)

Volume of the frustrum ACDB of the cone (V,AB) = Volume of the cone (V, AB) – Volume of the cone (V, CD)
= \(\)

= \(\frac{\pi}{3}\left(\mathrm{R}^{2} \cdot \frac{h \mathrm{R}}{\mathrm{R}-r}-r^{2} \cdot \frac{h r}{\mathrm{R}-r}\right)\)

= \(\frac{\pi h}{3}\left(\frac{\mathrm{R}^{3}-r^{3}}{\mathrm{R}-r}\right)\)

= \(\frac{1}{3} \pi h \times \frac{(\mathrm{R}-r)\left(\mathrm{R}^{2}+\mathrm{R} r+r^{2}\right)}{(\mathrm{R}-r)}\)

= \(\frac{1}{3}\) πh (R² + Rr + r²)
Hence, volume of the frustum of cone is \(\frac{1}{3}\) πh (R² + Rr + r²).

Again if A₁ and A₂ (A₁ > A₂) are the surface areas of the two circular bases, then A₁ = πR² andA₂ = πr²

Now volume of the frustum of cone,

= \(\frac{1}{3}\) πh (R² + r² + Rr)

= \(\frac{h}{3}\) (πR² + πr² + \(\sqrt{\pi \mathrm{R}^{2}} \sqrt{\pi r^{2}}\))

= \(\frac{h}{3}\) (A₁ + A₂ + \(\sqrt{A_{1} A_{2}}\))

Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 4 Practical Geometry Ex 4.4 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 8 Maths Chapter 4 Practical Geometry Ex 4.4

1. Construct the following quadrilaterals:

Question (i).
Quadrilateral DEAR
DE = 4 cm
EA = 5 cm
AR = 4.5 cm
∠E = 60°
∠A = 90°
Solution:

Steps of construction:

• Draw a line segment DE = 4 cm.
• At E, draw \(\overrightarrow{\mathrm{EM}}\) such that ∠DEM = 60°.
• With E as centre and radius = 5 cm, draw an arc intersecting \(\overrightarrow{\mathrm{EM}}\) at A.
• At A, draw \(\overrightarrow{\mathrm{AN}}\) such that ∠EAN = 90°.
• With A as centre and radius = 4.5 cm, draw an arc intersecting \(\overrightarrow{\mathrm{AN}}\) at R.
• Draw \(\overline{\mathrm{DR}}\).

Thus, DEAR is the required quadrilateral.

Question (ii).
Quadrilateral TRUE
TR = 3.5 cm
RU = 3 cm
UE = 4 cm
∠R = 75°
∠U = 120°
Solution:

Steps of construction :

• Draw a line segment TR = 3.5 cm.
• At R, draw a \(\overrightarrow{\mathrm{RM}}\) such that ∠TRM = 75°.
• With R as centre and radius = 3 cm, draw an arc intersecting \(\overrightarrow{\mathrm{RM}}\) at U.
• At U, draw \(\overrightarrow{\mathrm{UN}}\) such that ∠RUN = 120°.
• With U as centre and radius = 4 cm, draw an arc intersecting \(\overrightarrow{\mathrm{UN}}\) at E.
• Draw \(\overline{\mathrm{ET}}\).

Thus, TRUE is the required quadrilateral.

Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 4 Practical Geometry Ex 4.3 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 8 Maths Chapter 4 Practical Geometry Ex 4.3

1. Construct the following quadrilaterals:

Question (i).
Quadrilateral MORE
MO = 6 cm
OR = 4.5 cm
∠M = 60°
∠O = 105°
∠R = 105°
Solution:

• Draw a line segment MO = 6 cm.
• At M, draw \(\overrightarrow{\mathrm{MA}}\), such that ∠OMA = 60°
• At O, draw \(\overrightarrow{\mathrm{OB}}\) such that ∠MOB = 105°.
• With O as centre and radius = 4.5 cm, draw an arc intersecting \(\overrightarrow{\mathrm{OB}}\) at R.
• At R, draw \(\overrightarrow{\mathrm{RC}}\) such that ∠ORC = 105°.
• Locate E at intersection of \(\overrightarrow{\mathrm{RC}}\) and \(\overrightarrow{\mathrm{MA}}\).

Thus, MORE is the required quadrilateral.

Question (ii).
Quadrilateral PLAN
PL = 4 cm
LA = 6.5 cm
∠P = 90°
∠A = 110°
∠N = 85°
Solution:

[Note : In □ PLAN, m∠P = 90°, m∠A = 110° and m∠N = 85°)
∴ m∠L = 360°- (m∠P + m∠A + m∠N)
= 360° – (90° + 110° + 85°)
= 360° – 285°
= 75°

Steps of construction:

• Draw a line segment AL = 6.5 cm.
• At A, draw \(\overrightarrow{\mathrm{AX}}\) such that ∠XAL = 110°. (Use protractor)
• At L, draw \(\overrightarrow{\mathrm{LY}}\) such that ∠YLA = 75°. (Use protractor)
• With L as centre and radius = 4 cm, draw an arc intersecting \(\overrightarrow{\mathrm{LY}}\) at P.
• At P, draw \(\overrightarrow{\mathrm{PZ}}\) such that ∠ZPL = 90°. (∵ ∠ ZPY = 90°)
• Locate N at intersection of \(\overrightarrow{\mathrm{AX}}\) and \(\overrightarrow{\mathrm{PZ}}\).

Thus, PLAN is the required quadrilateral.

Question (iii).
Parallelogram HEAR
HE = 5 cm
EA = 6 cm
∠R = 85°
Solution:

[Note : □ HEAR is a parallelogram.
Opposite sides of parallelogram are of equal lengths.]
HE = 5 cm, ∴ AR = 5 cm, EA = 6 cm, ∴ HR = 6 cm
Adjacent angles of a parallelogram arc supplementary.
m∠R = 85° (given)
∴ m∠H = 180° – 85° = 95°
Opposite angles of a parallelogram are of equal measures,
m ∠ R = 85°
∴ m ∠ E = 85°

Steps of construction:

• Draw a line segment HE = 5 cm.
• At H, draw \(\overrightarrow{\mathrm{HX}}\), such that ∠ XHE = 95°. (Use protractor)
• With H as centre and radius = 6 cm, draw an arc intersecting \(\overrightarrow{\mathrm{HX}}\) at R.
• At E, draw \(\overrightarrow{\mathrm{EY}}\) such that ∠ HEY = 85°. (Use protractor)
• With E as centre and radius = 6 cm, draw an arc intersecting \(\overrightarrow{\mathrm{EY}}\) at A.
• Draw \(\overline{\mathrm{AR}}\).

Thus, HEAR is the required parallelogram.

Question (iv).
Rectangle OKAY
OK = 7 cm
KA = 5 cm
Solution:

[Note: Here, OKAY is a rectangle. Opposite sides of a rectangle are of equal lengths.]
OK = 7 cm, ∴ AY = 7 cm and KA = 5 cm, ∴ OY = 5 cm
Moreover, all angles of a rectangle are right angles.

Steps of construction:

• Draw a line segment OK = 7 cm.
• At O, draw \(\overrightarrow{\mathrm{OM}}\) such that ∠ MOK = 90°.
• With O as centre and radius = 5 cm, draw an arc intersecting \(\overrightarrow{\mathrm{OM}}\) at Y.
• At K, draw \(\overrightarrow{\mathrm{KN}}\) such that ∠ NKO = 90°.
• With K as centre and radius = 5 cm, draw an arc intersecting \(\overrightarrow{\mathrm{KN}}\) at A.
• Draw \(\overline{\mathrm{AY}}\).

Thus, OKAY is the required rectangle.

Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 13 Direct and Inverse Proportions InText Questions and Answers.

PSEB 8th Class Maths Solutions Chapter 13 Direct and Inverse Proportions InText Questions

Try These : [Textbook Page No. 204]

1. Observe the following tables and find if x and y are directly proportional.

Question (i)

X	20	17	14	11	8	5	2
y	40	34	28	22	16	10	4

Solution:
\(\begin{aligned}
&\frac{20}{40}=\frac{1}{2}, \frac{17}{34}=\frac{1}{2}, \frac{14}{28}=\frac{1}{2}, \frac{11}{22}=\frac{1}{2}, \frac{8}{16}=\frac{1}{2} \\
&\frac{5}{10}=\frac{1}{2}, \frac{2}{4}=\frac{1}{2}
\end{aligned}\)
The value of \(\frac{\text {x}}{\text {y}}\) is same for different values of x and y. So these values x and y are directly proportional.

Question (ii)

X	6	10	14	18	22	26	30
y	4	8	12	16	20	24	28

Solution:
\(\begin{aligned}
&\frac{6}{4}=\frac{3}{2}, \frac{10}{8}=\frac{5}{4}, \frac{14}{12}=\frac{7}{6}, \frac{18}{16}=\frac{9}{8}, \frac{22}{20}=\frac{11}{10}, \\
&\frac{26}{24}=\frac{13}{12}, \frac{30}{28}=\frac{15}{14}
\end{aligned}\)
The values of \(\frac{\text {x}}{\text {y}}\) are different for different values of x and y respectively. So these values of x and y are not directly proportional.

Question (iii)

X	5	8	12	15	18	20
y	15	24	36	60	72	100

Solution:
The values of \(\frac{\text {x}}{\text {y}}\) are different for different values of x and y respectively.
So these values of x and y are not directly proportional.

2. Principal = ₹ 1000, Rate = 8 % per annum. Fill in the following table and find which type of interest (simple or compound) changes in direct proportion with time period.

Time Period	1 year	2 years	3 years
Simple Interest (in ₹)
Compound Interest (in ₹)

Solution:
Simple interest : SI = \(\frac{P \times R \times T}{100}\)
For calculation:
P = ₹ 1000, R = 8 %, T = …………….

Time (T) →	1 year: T = 1	2 years : T = 2	3 years : T = 3
Simple interest SI = \(\frac{P \times R \times T}{100}\)	₹ \(\frac{1000 \times 8 \times 1}{100}\) = ₹ 80	₹ \(\frac{1000 \times 8 \times 2}{100}\) = ₹ 160	₹ \(\frac{1000 \times 8 \times 3}{100}\) = ₹ 240
\(\frac{\text { SI }}{\text { T }}\)	\(\frac {80}{1}\) = 80	\(\frac {160}{2}\) = 80	\(\frac {240}{3}\) = 80

Here, the ratio of simple interest with time period is same for every year.
Hence, simple interest changes in direct proportion with time period.
Compound interest:
For calculation:
P = ₹ 1000, R = 8 %, T = ……………

Time →	1 year : n = 1
A = P(1 + \(\frac {R}{100}\))n CI = A – P	A = 1000(1 + \(\frac {8}{100}\))1 = 1000 × \(\frac {108}{100}\) = 1080 ∴ CI = 1080 – 1000 = ₹ 80
\(\frac{\text { CI }}{\text { T }}\)	\(\frac {80}{1}\)

Time →	2 years : n = 2
A = P(1 + \(\frac {R}{100}\))n CI = A – P	A = 1000(1 + \(\frac {8}{100}\))2 = 1000 × \(\frac {108}{100}\) × \(\frac {108}{100}\) = ₹ 1166.40 ∴ CI = ₹ 1166.40 – 1000 = ₹ 166.40
\(\frac{\text { CI }}{\text { T }}\)	\(\frac {166.40}{2}\)

Time →	3 years : n = 3
A = P(1 + \(\frac {R}{100}\))n CI = A – P	A = 1000(1 + \(\frac {8}{100}\))3 = 1000 × \(\frac {108}{100}\) × \(\frac {108}{100}\) × \(\frac {108}{100}\) = ₹ 1259.712 ∴ CI = ₹ 1259.712 – ₹ 1000 = ₹ 259.712
\(\frac{\text { CI }}{\text { T }}\)	\(\frac {259.712}{3}\)

Here, the ratio of CI and T is not same.
Thus, compound interest is not proportional with time period.

Think, Discuss and Write: [Textbook Page No. 204]

1. If we fix time period and the rate of interest, simple interest changes proportionally with principal. Would there be a similar relationship for compound interest? Why?
Solution:
Time period (T) and rate of interest (R) are fixed, then
Simple interest = \(\frac {PRT}{100}\) = P × Constant
So simple interest depends on principal. Simple interest changes proportionally with principal.
Now, compound interest = P(1 + \(\frac {R}{100}\))^T – P
i.e., A – P
= P [(1 + \(\frac {R}{100}\)^T – 1]
= P × Constant
So compound interest depends on principal.
If principal increases or decreases, then compound interest will also increases or decreases.
Thus, compound interest changes with principal.

Think, Discuss and Write : [Textbook Page No. 209]

1. Take a few problems discussed so far under ‘direct variation’. Do you think that they can be solved by ‘unitary method’?
Solution:
Yes, each problem can be solved by unitary method.
e.g. Question 4 of Exercise: 13.1
Number of bottles filled in 6 hours = 840
∴ The number of bottles filled in 1 hour = \(\frac {840}{6}\) = 140
The number of bottles filled in 5 hours = 140 × 5 = 700
Thus, 700 bottles will it fill in five hours.

Try These : [Textbook Page No. 211]

1. Observe the following tables and find which pair of variables (here x and y) are in inverse proportion.

Question (i)

X	50	40	30	20
y	5	6	7	8

Solution:
x₁ = 50 and y₁ = 5
∴ x₁y₁ = 50 × 5
∴ x₁y₁ = 250

x₂ = 40 and y₂ = 6
∴ x₂y₂ = 40 × 6
∴ x₂y₂ = 240

x₃ = 30 and y₃ = 7
∴ x₃y₃ = 30 × 7
∴ x₃y₃ = 210

x₄ = 20 and y₄ = 8
∴ x₄y₄ = 20 × 8
∴ x₄y₄ = 160
Now 250 ≠ 240 ≠ 210 ≠ 160
∴ x₁y₁ ≠ x₂y₂ ≠ x₃y₃ ≠ x₄y₄
∴ x and y are not in inverse proportion.

Question (ii)

X	100	200	300	400
y	60	30	20	15

Solution:
x₁ = 100 and y₁ = 60
∴ x₁y₁ = 100 × 60
∴ x₁y₁ = 6000

x₂ = 200 and y₂ = 30
∴ x₂y₂ = 200 × 30
∴ x₂y₂ = 6000

x₃ = 300 and y₃ = 20
∴ x₃y₃ = 300 × 20
∴ x₃y₃ = 6000

x₄ = 400 and y₄ = 15
∴ x₄y₄ = 400 × 15
∴ x₄y₄ = 6000
Now x₁y₁ = x₂y₂ = x₃y₃ = x₄y₄
∴ x and y are in inverse proportion.

Question (iii)

X	90	60	45	30	20	5
y	10	15	20	25	30	35

Solution:
x₁ = 90 and y₁ = 10
∴ x₁y₁ = 90 × 10
∴ x₁y₁ = 900

x₂ = 60 and y₂ = 15
∴ x₂y₂ = 60 × 15
∴ x₂y₂ = 900

x₃ = 45 and y₃ = 20
∴ x₃y₃ = 45 × 20
∴ x₃y₃ = 900

x₄ = 30 and y₄ = 25
∴ x₄y₄ = 30 × 25
∴ x₄y₄ = 750

x₅ = 20 and y₅ = 30
∴ x₅y₅ = 20 × 30
∴ x₅y₅ = 600

x₆ = 30 and y₆ = 35
∴ x₆y₆ = 5 × 35
∴ x₆y₆ = 175

Now x₁y₁ = x₂y₂ = x₃y₃ ≠ x₄y₄ ≠ x₅y₅ ≠ x₆y₆
∴ x and y are in inverse proportion.

Punjab State Board PSEB 10th Class Maths Book Solutions Chapter 13 Surface Areas and Volumes Ex 13.4 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 10 Maths Chapter 13 Surface Areas and Volumes Ex 13.4

Question 1.
A drinking glass is in the shape of a frustum of a cone of height 14 cm. The diameters of its two circular ends are 4 cm and 2 cm. Find the capacity of the glass.
Solution:

Radius of upper end (R) = 2 cm
Radius of lower end (r) = 1 cm
Height of glass (H) = 14 cm
Glass is in the shape of frustum
Volume of frustum = \(\frac{1}{3}\) π [R² + r² +Rr]H

= \(\frac{1}{3}\) × \(\frac{22}{7}\) [(2)² + (1)² + 2 × 1] 14

= \(\frac{1}{3}\) × \(\frac{22}{7}\) [4 + 1 + 2] 14

= \(\frac{1}{3}\) × \(\frac{22}{7}\) × 7 × 14

= \(\frac{22 \times 14}{3}\)
Hence, Volume of glass = 102.67 cm³.

Question 2.
The slant height of a frustum of a cone is 4 cm and the perimeters (circumference) of its circular ends are 18 cm and 6 cm. Find the curved surface area of the frustum.
Solution:
Slant height of frustum = 4 cm

Let radius of upper end and lower ends are R and r
Circumference of upper end = 18 cm
2πR = 18
R = \(\frac{18}{2 \pi}=\frac{9}{\pi}\) cm
Circumference of lower end = 6 cm
2πr = 6 cm
r = \(\frac{6}{2 \pi}=\frac{3}{\pi}\) cm
Curved surface area of frustum = π [R + r] l
= π \(\left[\frac{9}{\pi}+\frac{3}{\pi}\right]\) 4
= π \(\left[\frac{9+3}{\pi}\right]\) 4
= 12 × 4 = 48 cm²
Hence, Curved surface area of frustum = 48 cm².

Question 3.
A fez, the cap used by the Turks, is shaded like the frustum of a cone. If its radius on the open side is 10 cm, radius at the upper base is 4 cm and its slant height is 15 cm, find the area of material used for making it.

Solution:

Radius of lower end of frustum (R) = 10 cm
Radius of upper end of frustum (r) = 4 cm
Slant height of frustum (l) = 15 cm
Curved surface area of frustum = πL [R+ r]
= \(\frac{22}{7}\) × 15 [10 + 4]
= \(\frac{22}{7}\) × 15 × 14
= 22 × 15 × 2 = 660 cm²
Area of the closed side = πr² = \(\frac{22}{7}\) × (4)²
= \(\frac{22}{7}\) × 4 × 4 = \(\frac{352}{7}\) cm²
Total area of the material used = Curved surface area of frustum + Area of the closed side
= 660 + 50.28 = 710.28 cm²
Hence, Total material used = 710.28 cm².

Question 4.
A container opened from the top is made up of a metal sheet ¡s in the form of a frustum of a cone of height 16 cm with radii of its lower and upper ends as 8 cm and 20 cm, respectively. Find the cost of the milk which can completely fill the container, at the rate, of ₹ 20 per litre. Also fmd the cost of metal sheet used to make the container, If it costs ₹ 8 per 100 cm². (Take π = 3:14.)
Solution:

Radius of upper end of container (R) = 20 cm
Radius of lower end of container (r) = 8 cm
Height of container (H) = 16 cm
Slant height (l) = \(\sqrt{\mathrm{H}^{2}+(\mathrm{R}-r)^{2}}\)
= \(\sqrt{(16)^{2}+(20-8)^{2}}\) = \(\sqrt{256+144}\)
Slant height (l) = \(\sqrt{400}=\sqrt{20 \times 20}\) = 20 cm

Capacity of the container = \(\frac{1}{3}\) πH[R² + r² + Rr]
= \(\frac{1}{3}\) × 3.14 × 16 [(20)² + (8)² + 20 × 8]
= \(\frac{3.14 \times 16}{3}\) [400 + 64 + 100]
= 3.14 × 16 × 624 = 10449.92 cm³
Milk in the container = 10449.92 cm³
= \(\frac{10449.92}{1000}\) litres [∵ 1 cm³ = \(\frac{1}{1000}\) litres]
∴ Milk in the container = 10.45 litres
Cost of 1 it. milk = ₹ 20
∴ Cost of 10.45 litre = ₹ 20 × 10.45
Total cost of milk = ₹ 209
Curved surface area of frustum = πL [R + r]
= 3.14 × 20[20 + 8]
= 3.14 × 20 × 28 cm² = 1758.4 cm²
Area of base of container = πr²
= 3.14 × (8)π = 3.14 × 64 = 200.96 cm²

Total metal used to make coniainer = curved surface area of frustum + area of base
= (1758. 4 + 200.96) cm² = 1959.36 cm²
Cost of 100 cm² metal sheet used = ₹ 8
Cost of 1 cm² metal sheet used = ₹ \(\frac{8}{100}\)
Cost of 1959.36 cm² metal sheet used = ₹ \(\frac{8}{100}\) × 1959.36
= ₹ 156.748 = ₹ 156.75
Hence, Total cost of sheet used = ₹ 156.75
and Total cost of milk is ₹ 209.

Question 5.
A metallic right circular cone 20 cm high and whose vertical angle Ls 600 is cut into two parts at the middle of its height by a plane parallel to Its base. If the frustum so obtained be drawn into a wire of diameter \(\frac{4}{4}\) cm, find the length of the wire.
Solution:
Vertical angle of cone = 60°
Altitude of cone divide vertical angle ∠EOF = 30°

In ∆ODB,
\(\frac{\mathrm{BD}}{\mathrm{OD}}\) = tan 30°

\(\frac{r}{10}=\frac{1}{\sqrt{3}}\)

r = \(\frac{10}{\sqrt{3}}\) cm

In ∆OEF,

\(\frac{E F}{O E}\) = tan 30°

\(\frac{\mathrm{R}}{20}=\frac{1}{\sqrt{3}}\)

R = \(\frac{20}{\sqrt{3}}\) cm

Volume of frustum = \(\frac{\pi}{3}\)h[R² + r² + Rr]
= \(\frac{22}{73} \times \frac{10}{3}\left[\left(\frac{20}{\sqrt{3}}\right)^{2}+\left(\frac{10}{\sqrt{3}}\right)^{2}+\frac{20}{\sqrt{3}} \times \frac{10}{\sqrt{3}}\right]\)

= \(\frac{22}{7} \times \frac{10}{3}\left[\frac{400}{3}+\frac{100}{3}+\frac{200}{3}\right]\)

= \(\frac{22}{7} \times \frac{10}{3}\left[\frac{400+100+200}{3}\right]\)

Volume of frustum = \(\frac{22}{7} \times 10 \times \frac{700}{9}\) cm³

= \(\frac{22}{7} \times \frac{7000}{9}\) cm³
Frustum is made into wiie, which is in shape of cylinder having diameter \(\frac{1}{16}\) cm
∴ Radius of cylinderical wire (r₁) = \(\frac{1}{2} \times \frac{1}{16} \mathrm{~cm}=\frac{1}{32} \mathrm{~cm}\)

Let height of cylinder so formed be H cm
On recasting volume remain same
Volume of frustum = Volume of cylindrical wire

\(\frac{22}{7} \times \frac{7000}{9}\) = πr₁²H

\(\frac{22}{7} \times \frac{7000}{9}=\frac{22}{7} \times\left(\frac{1}{32}\right)^{2} \times \mathrm{H}\)

H = \(\frac{\frac{22}{7} \times \frac{7000}{9}}{\frac{22}{7} \times \frac{1}{32} \times \frac{1}{32}}\)

= \(\frac{7000}{9}\) × 32 × 32

H = 796444.44 cm

H = \(\) = 7964.44 m
Hence, Length of cylindrical wire (H) = 7964.44 m

Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 13 Direct and Inverse Proportions Ex 13.2 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 8 Maths Chapter 13 Direct and Inverse Proportions Ex 13.2

1. Which of the following are in inverse proportion?

Question (i)
The number of workers on a job and the time to complete the job.
Solution:
If the number of workers on a job increases, then time to complete the job decreases. So, it is the case of inverse proportion.

Question (ii)
The time taken for a journey and the distance travelled in a uniform speed.
Solution:
Here, the speed is uniform.
∴ The time taken for a journey is directly proportional to the speed. So, it is not the case of inverse proportion.

Question (iii)
Area of cultivated land and the crop harvested.
Solution:
For more area of cultivated land, more crops would be harvested. So, it is not the case of inverse proportion.

Question (iv)
The time taken for a fixed journey and the speed of the vehicle.
Solution:
If speed of vehicle is more, then time to cover the fixed journey would be less. So, it is the case of inverse proportion.

Question (v)
The population of a country and the area of land per person.
Solution:
For more population, less area per person would be in the country. So, it is a case of inverse proportion.

2. In a Television game show, the prize money of ₹ 1,00,000 is to be divided equally amongst the winners. Complete the following table and find whether the prize money given to an individual winner is directly or inversely proportional to the number of winners?

Number of winners	1	2	4	5	8	10	20
Prize for each winner (in ₹)	1,00,000	50,000	…	…	…	…	…

Solution:
Here, more the number of winners, less is the prize money for each winner.
∴ This is a case of inverse proportion.

Now, the table is as follows:

Number of winners	1	2	4	5	8	10	20
Prize for each winner (in ₹)	1,00,000	50,000	25,000	20,000	12,500	10,000	5,000

3. Rehman is making a wheel using spokes. He wants to fix equal spokes in such a way that the angles between any pair of consecutive spokes are equal. Help him by completing the following table:

Number of spokes	4	6	8	10	12
Angle between a pair of consecutive spokes	90°	60°	…	…	…

Question (i)
Are the number of spokes and the angles formed between the pairs of consecutive spokes in inverse proportion?
Solution:
Here, more the number of spokes, less the measure of angle between a pair of consecutive spokes.
∴ This is a case of inverse proportion.
Here,

Now, the table is as follows:

Number of spokes	4	6	8	10	12
Angle between a pair of consecutive spokes
	90°	60°	45°	36°	30°

Question (ii)
Calculate the angle between a pair of consecutive spokes on a wheel with 15 spokes.
Solution:
Let the measure of angle be x°.
∴ 15 × x° = 4 × 90°
∴ x° = \(\frac{4 \times 90^{\circ}}{15}\) = 24°
Hence, this angle should be 24°.

Question (iii)
How many spokes would be needed, if the angle between a pair of consecutive spokes is 40°?
Solution:
Let the number of spokes be n.
∴ n × 40° = 4 × 90°
∴ n = \(\frac{4 \times 90^{\circ}}{40^{\circ}}\) = 9
Thus, 9 spokes would be needed.

4. If a box of sweets is divided among 24 children, they will get 5 sweets each. How many would each get, if the number of the children is reduced by 4?
Solution:

Number of children x	Number of sweets y
x1 = 24	y1 = 5
x2 = 24 – 4 = 20	y2 = (?)

Here, if the number of children decreases, then the number of sweets received by each child will increase.
∴ This is a case of inverse proportion.
x₁ × y₁ = x₂ × y₂
∴ 24 × 5 = 20 × y₂
∴ y₂ = \(\frac{24 \times 5}{20}\) = 6
Thus, each child will get 6 sweets.

5. A farmer has enough food to feed 20 animals in his cattle for 6 days. How long would the food last if there were 10 more animals in his cattle?
Solution:

Number of animals x	Number of days y
x1 = 20	y1 = 6
x2 = 20 + 10 = 20	y2 = (?)

Here, the number of animals increases, so the number of days to feed them will decrease.
∴ This is a case of inverse proportion.
∴ x₁ × y₁ = x₂ × y₂
∴ 20 × 6 = 30 × y₂
∴ y₂ = \(\frac{20 \times 6}{30}\) = 4
Thus, the food will last for 4 days.

6. A contractor estimates that 3 persons could rewire Jasminder’s house in 4 days. If, he uses 4 persons instead of three, how long should they take to complete the job?
Solution:

Number of persons x	Number of days y
x1 = 42	y1 = 63
x2 = (?)	y1 = 63

Here, more the number of persons, less will be the time required to complete the job.
∴ This is a case of inverse proportion.
∴ x₁ × y₁ = x₂ × y₂
∴ 3 × 4 = 4 × y₂
∴ y₂ = \(\frac{3 \times 4}{4}\) = 3
Thus, 3 days will be required to complete the job.

7. A batch of bottles were packed in 25 boxes with 12 bottles in each box. If the same batch is packed using 20 bottles in each box, how many boxes would be filled?

Solution:

Number of bottles in a box x	Number of boxes y
x1 = 12	y1 = 25
x2 = 20	y2 = (?)

Here, more the number of bottles in a box, less would be the number of boxes.
∴ This is a case of inverse proportion.
∴ x₁ × y₁ = x₂ × y₂
∴ 3 × 4 = 4 × y₂
∴ y₂ = \(\frac{3 \times 4}{4}\) = 3
Thus, 15 boxes would be filled.

8. A factory requires 42 machines to produce a given number of articles in 63 days. How many machines would be required to produce the same number of articles in 54 days?
Solution:

Number of machines x	Number of days y
x1 = 42	y1 = 63
x2 = (?)	y2 = 54

Here, if the number of days will be less, the number of machines required will be more.
∴ This is a case of inverse proportion.
∴ x₁ × y₁ = x₂ × y₂
∴ 42 × 63 = x₂ × 54
∴ x₂ = \(\frac{42 \times 63}{54}\) = 49
Thus, 49 machines would be required.

9. A car takes 2 hours to reach a destination by travelling at the speed of 60 km/h. How long will it take when the car travels at the speed of 80 km/h?
Solution:

Speed (km/h) x	Number of hours y
x1 = 60	y1 = 2
x2 = 80	y2 = (?)

Here, if the speed of car increases, then the time taken to cover the same distance will decrease.
∴ This is a case of inverse proportion.
∴ x₁ × y₁ = x₂ × y₂
∴ 60 × 2 = 80 × y₂
∴ y₂ = \(\frac{60 \times 2}{80}=\frac{3}{2}=1 \frac{1}{2}\) h
Thus, car would take 1\(\frac {1}{2}\) hours.

10. Two persons could fit new windows in a house in 3 days.

Question (i)
One of the persons fell ill before the work started. How long would the job take now?
Solution:

Number of persons x	Number of days y
x1 = 2	y1 = 3
x2 = 2 – 1 = 1	y2 = (?)

Here, less the number of persons, more would be the number of days to complete the job.
∴ This is a case of inverse proportion.
∴ x₁ × y₁ = x₂ × y₂
∴ 2 × 2 = 1 × y₂
∴ y₂ = \(\frac{2 \times 3}{1}\) = 6
Thus, it would take 6 days to complete the job.

Question (ii)
How many persons would be needed to fit the windows in one day?
Solution:

Number of days x	Number of persons y
x1 = 3	y1 = 2
x2 = 1	y2 = (?)

Here, less the number of days, more will be the number of persons needed.
∴ This is a case of inverse proportion.
∴ y₂ = ? and x₂ = 1
∴ x₁ × y₁ = x₂ × y₂
∴ 3 × 2 = 1 × y₂
∴ y₂ = \(\frac{3 \times 2}{1}\) = 6
Thus, 6 persons would be needed.

11. A school has 8 periods a day each of 45 minutes duration. How long would each period be, if the school has 9 periods a day, assuming the number of school hours to be the same?
Solution:

Number of periods x	Length of each period (in minute) y
x1 = 8	y1 = 45
x2 = 9	y2 = (?)

Here, the number of periods is more, then the length of each period will be less.
∴ This is a case of inverse proportion.
∴ x₁ × y₁ = x₂ × y₂
∴ 8 × 45 = 9 × y₂
∴ y₂ = \(\frac{8 \times 45}{9}\) = 40
Thus, each period would be of 40 minutes.