Bhagya

Punjab State Board PSEB 6th Class English Book Solutions English Grammar Verbs Exercise Questions and Answers, Notes.

PSEB 6th Class English Grammar Verbs

A Verb is an action word. It tells us something about a person or thing.
Verb एक क्रिया शब्द है। यह हमें किसी व्यक्ति या वस्तु के बारे में कुछ बताता है।

A Verb tells us-
1. What a person or thing is; as-

• The rose is red.
• The mouse was dead.
• Camels are useful animals.

2. What a person or thing has; as-

• A week has seven days.
• Monkeys have long tails.
• They had a good day yesterday.

3. What a person or thing does; as-

• She made a doll.
• He is writing a poem.
• The sun rises in the east.

Parts of a Verb क्रिया के भाग

A Verb in English can have two parts:
1. The Main (Primary) (मुख्य) Verb.
2. The Auxiliary (Helping) (सहायक) Verb.

1. The Main Verb tells us ‘what happened or ‘what the situation is’. The main verb can have four different forms:
(i) V₁ (go) or the root form.
(ii) V₂ (went) or the past form.
(iii) V₃ (gone) or the past participle form.
(iv) V₁-ing (going) or the present participle form.

2. The Auxiliary Verb helps the Main Verb to complete its meaning. It helps the Main Verb to form a Tense, or to form Negatives and Questions.
सहायक क्रिया Main verb (मख्य क्रिया) के अर्थ को पूरा करने में सहायता करती है। यह काल (Tense), नकारात्मक कथनों अथवा प्रश्नों के निर्माण में मुख्य क्रिया की सहायता करती है।

The following verbs are often used as Auxiliary Verbs:

• is, am, are, was, were.
• has, have, had.
• do, does, did.
• will, would, shall, should.
• can, could, may, might, must, etc.

Exercises (Solved)

I. Underline the Verbs in the following sentences:

1. I live in Delhi.
2. The lion roars.
3. It is raining outside.
4. I have two brothers.
5. Rina gave me a flower.
6. A cobbler mends shoes.
7. I will give you my book.
8. The driver was cleaning the car.
Hints:
1. live
2. roars
3. is raining
4. have
5. gave
6. mends
7. will give
8. was cleaning

II. Fill in the blanks with appropriate Auxiliaries:

1. …………… I come in, sir ?
2. I ………….. finished my work.
3. You …………… pay your debts.
4. We …………… help our friends.
5. I ………….. solve this question.
6. You ……………. sit on this bench.
7. Anyone ……………. make mistakes.
8. He ………….. not telling the truth.
Hints:
1. May
2. have
3. must
4. shall
5. can
6. can
7. can
8. is

Three Forms (Conjuction) of the verb

Exercises (Solved)

I. Conjugate the following Verbs:

build, fall, rise, speak, teach, weep, ruin, look, sleep, learn, study, throw, weave, forget, destroy
Answer:

Present	Past	Past Participle
build	built	built
fall	fell	fallen
rise	rose	risen
speak	spoke	spoken
teach	taught	taught
weep	wept	wept
ruin	ruined	ruined
look	looked	looked
sleep	slept	slept
learn	learnt	learnt
study	studied	studied
throw	threw	thrown
weave	wove	woven
forget	forgot	forgotten
destroy	destroyed	destroyed

II. Give the present participle form of the following Verbs:

1. sit
2. die
3. sink
4. fight
5. catch
6. tie
7. run
8. bite
9. shine
10. apply
11. hit
12. live
13. swim
14. writer
15. begin
Hints:
1. sitting
2. dying
3. sinking
4. fighting
5. catching
6. tying
7. running
8. biting
9. shining
10. applying
11. hitting
12. living
13. swimming
14. writing
15. beginning.

Note : 1. Verb की Present participle form verb के साथ ‘ing’ जोड़ने से बनती है।
2. कुछ verbs में ‘ing’ जोड़ते समय उनके अंतिम अक्षर अक्षरों में कुछ फेरबदल भी करना पड़ता है।

Punjab State Board PSEB 6th Class English Book Solutions English Grammar Preposition Exercise Questions and Answers, Notes.

PSEB 6th Class English Grammar Preposition

A Preposition is a word used before a Noun or Pronoun to indicate place, direction, source; as-
on, in, at, under, upon, into, from.
In the following pictures, study the different positions of the cat.

The italicized words show the position of the cat. We call them prepositions. Some other Propositions of common use are-
of, near, to, after, with, during, off, down, by, above, before, between, at, from, for, among, below, without

Use of Some Prepositions

1. On, Upon.
On indicates position of rest on something.
Upon indicates motion.

• The books are on the table.
• The cat jumped upon the wall.

2. In, Into.
In expresses position of rest inside something.
Into indicates motion towards the inside of something.

• Meera was sitting in her room.
• Neha went Into the room.

3. At, In, On
At is used for a point of time.
On is used with days and dates.
In is used with months and years.

• My brother came here at eight.
• I met my friend at 5 o’clock.
• India got freedom in 1947.
• I shall go to Mumbai on Monday.
• His birthday falls on 15 Nov.
• He went to Ludhiana in May.

Note some other uses of ‘at’ and ‘in’.

• At dawn / noon / night
• In the morning
• In the evening
• In the afternoon

4. At, In
At is used with names of small villages and towns.
In is used with names of big cities and states.

• I was bom at Rampur.
• My uncle lives in Delhi.

5. Between, Among.
‘Between’ is used for two persons, places or things.
‘Among’ is used for more than two persons, places or things.

• The two brothers divided their property between themselves.
• The three brothers quarrelled among themselves.

Exercises (Solved)

I. Underline the prepositions in the following sentences:

1. She sat beside me.
2. Rani is afraid of her teacher.
3. The bird flew over the trees.
4. The dog ran after the mouse.
5. The sky and clouds are above us.
6. The river flows under the bridge.
7. December comes after November.
8. There is a big well behind his house.
9. She brought a beautiful dress for me.
10. I went with my friend to see a movie.
Hints:
2. of
3. over
4. after
5. above
6. under
7. after
8. behind
9. for
10. with, to.

II. Fill in the blanks with a suitable preposition from the box:

of, on, for, near, into, at, by, after, from, with
1. I am fond …………… music.
2. Look …………… the blackboard.
3. I am waiting …………… the bus.
4. The policeman ran …………… the thief.
5. Keep the books …………… the shelf.
6. What is Ludhiana famous …………… ?
7. We are proud …………… our country.
8. My ball has fallen …………… the well.
9. Are you coming …………… road or rail ?
10. I went …………… my Mends for a picnic.
11. There is a temple …………… the hospital.
12. Tony cleaned the floor …………… a broom.
13. The girls are waiting …………… the station.
14. My Mend’s house is far …………… our house.
15. She lives …………… her parents.

Hints:
1. of
2. at
3. for
4. after
5. on
6. for
7. of
8. into
9. by
10. with
11. near
12. with
13. at
14. from
15. with.

III. Write what you see in this picture. Use a suitable preposition in each one of your sentences; as-

Mrs. Raj and Mrs. Rani are sitting on a bench.
Tony is trying to get at the apple.
Some birds are flying in the sky.
A crow is sitting on the branch of a tree.
A dog is running after a ball.
Neha is reading a book under a tree.
Sonu and Anu are waiting for the fancy ball to come down.

Punjab State Board PSEB 6th Class English Book Solutions English Grammar Articles Exercise Questions and Answers, Notes.

PSEB 6th Class English Grammar Articles

‘A’, “an’, ‘the’ are called Articles.
An Article is a word that determines or limits the noun that follows it.
An Article is always used with a Noun. So it can also be called an Adjective.
Article वह शब्द है जो अपने बाद प्रयोग किए गए Noun को निरिचत अथवा सीमित करता है।
Article का प्रयोग हमेशा Noun के साथ ही किया जाता है। इसलिए इसे Adjective भी कहा जा सकता है।

Use of ‘a’, ‘an’

You have already learnt that ‘an’ is used before words beginning with a vowel sound and ‘a’ before words beginning with a consonant sound.

Look at these pictures and name the objects using appropriate articles. Two have been done for you:

Exercise (Solved)

Put ‘a’ or ‘an’ for each:

1. …………. ox
2. ………… kite
3. ………… unit
4. ………… cart
5. ………… M.A.
6. ……….. hour
7. …………. table
8. ………. inkpot
9. ……….. monkey
10. ……….. elephant
11. ……….. honest man
12. ………. useful thing
13. ……….. European lady
14. ……….. one-eyed man.
Hints:
1. an
2. a
3. a
4. a
5. an
6. an
7. a
8. an
9. a
10. an
11. an
12. a
13. a
14. a.

Use of ‘the’

We use “the’ to talk of some specific (विशिष्ट) person, animal, place or thing. We use the’ in the following cases also:

1. Before the names of rivers and seas–
the Ganges, the Sutlej, the Jamuna; the Indian Ocean, the Arabian sea.

2. Before the names of magazines, newspapers and holy books–
the India Today, the Observer, the Tribune, the Times of India; the Bible, the Quran, the Gita.

3. Before the names of races or people–
the Hindus, the Punjabis, the English.

4. Before superlatives–
the hardest, the best, the eldest

5. Before the names of natural objects–
the sun, the moon, the earth.

6. Before the names of mountain ranges–
the Himalayas, the Vindhyas, the Alps

7. Before the names of historical places–
the Jallianwalla Bagh; the Red Fort, the Taj Mahal

8. Before a noun that is modified-
She is the girl who stands first in the class.

Exercises (Solved)

I. Fill in the blanks with the articles ‘a’, ‘an’ or ‘the’:

1. I waited for ……………. hour.
2. ………….. ant is …………… insect.
3. …………. Red Fort is in Delhi.
4. Jack and Jill went up …………… hill.
Speak truth. Don’t tell …………. lie.
6. She rode on …………… elephant at the zoo.
7. ………….. earth is covered with land and water.
8. Bible is ……………. sacred book of Christians.
9. In ………….. sky at night we can see …………… stars and ……………. moon.
10. He took …………… banana, …………… orange and ………….. apple for breakfast.
11. ………….. Sun shines in the east.
12. He is …………….. engineer.
Hints:
1. an
2. An, an
3. The
4. the
5. the, a
6. an
7. The
8. The, a, the
9. the, the, the
10. a, an, an.
11. The
12. an

II. Fill in the blanks with suitable articles (a/an/the):

1. Rohit wrote …………. essay.
2. I saw …………… one eyed man.
3. ………….. sun rises in the east.
4. watch tells us …………. time.
5. accident is ……………. ugly sight.
6. She went home in …………….. morning.
7. …………….. boy standing there is my friend.
8. Mohit saw ……………… old man crossing the road.
9. In the north of our country are ……………. Himalayas.
10. ………….. Ganges, ……………… Yamuna and Saraswati meet at Sangam.
Hints:
1. an
2. a
3. The
4. The, the
5. An, an
6. the
7. The
8. an
9. the
10. The, the, the

III. Rewrite each sentence correctly:

1. He is an European.
2. I heard loud noise.
3. Look at blackboard.
4. Rajan is honest man.
5. I go for the walk in evening.
6. Gardener is watering plants.
7. I gave him an one-rupee coin.
8. An umbrella is an useful thing.
9. He was a best judge of horses.
10. She is a tallest girl in our class.
Answer:
1. He is a European.
2. I heard a loud noise.
3. Look at the blackboard.
4. Rajan is an honest man.
5. I go for a walk in the evening.
6. The gardener is watering the plants.
7. I gave him a one-rupee coin.
8. An umbrella is a useful thing.
9. He was the best judge of horses.
10. She is the tallest girl in our class.

Punjab State Board PSEB 6th Class English Book Solutions English Grammar Adjectives Exercise Questions and Answers, Notes.

PSEB 6th Class English Grammar Adjectives

An Adjective is a defining word. It is used with a Noun to tell us something more about that noun; as-
Adjective एक व्याख्यात्मक शब्द है। इसका प्रयोग किसी संज्ञा (Noun) के साथ उसके बारे में कुछ और बताने के लिए किया जाता है।

1. He is a tall boy.
2. She is a rich lady.
3. I have many books.

We can use Adjectives to compare (तुलना करना) the qualities of Nouns; as-

1. Chintu is a fat boy.
2. Mintu is fatter than Chintu.
3. Pintu is the fattest of the three.

The words fat, fatter and fattest show the degrees of a quality. We call them Degrees of Comparison.

Degrees Of Comparison

There can be three Degrees of Comparison:

1. Positive Degree.
2. Comparative Degree.
3. Superlative Degree.

1. Positive Degree : The ordinary form (साधारण रूप) of the adjective is called Positive Degree; as-

• Meena is a tall girl.
• June is a hot month.
• Dhawan is a good player.

2. Comparative Degree : When two things of the same class are compared, we use the Comparative Degree; as-

• Anita is taller than Payal.
• May is hotter than April.
• Virat is a better player than Murli.

3. Superlative Degree : When one thing is compared with all others of the same class, we use the Superlative Degree; as-

• Anita is the tallest of the three.
• June is the hottest month of the year.
• Virat is the best player in our cricket team.

Note that we usually use-
“the’ before a Superlative Degree.
“than’ after a Comparative Degree.

Forming Degrees Of Comparison

1. By adding -er and -est:

Positive	Comparative	Superlative
tall	taller	tallest
fast	faster	fastest
long	longer	longest
kind	kinder	kindest
slow	slower	slowest
poor	poorer	poorest
short	shorter	shortest
small	smaller	smallest
old	older	oldest
young	younger	youngest

2. By adding -r and -st:

Positive	Comparative	Superlative
late	later	latest
fine	finer	finest
nice	nicer	nicest
wise	wiser	wisest
large	larger	largest
brave	braver	bravest
gentle	gentler	gentlest

3. By adding -ier and -iest in place of the final ‘-y’:

Positive	Comparative	Superlative
dirty	dirtier	dirtiest
noisy	noisier	noisiest
funny	funnier	funniest
heavy	heavier	heaviest
happy	happier	happiest
pretty	prettier	prettiest
greedy	greedier	greediest

4. By doubling the final consonant before adding -er and -est:

Positive	Comparative	Superlative
fat	fatter	fattest
big	bigger	biggest
hot	hotter	hottest
sad	sadder	saddest
thin	thinner	thinnest
wet	wetter	wettest

5. By adding more and most:

Positive	Comparative	Superlative
honest	more honest	most honest
popular	more popular	most popular
beautiful	more beautiful	most beautiful
wonderful	more wonderful	most wonderful
interesting	more interesting	most interesting

6. By using a different word:

Positive	Comparative	Superlative
good	better	best
little	less	least
old	elder	eldest
bad	worse	worst
much / many	more	most

Exercises (Solved)

I. Fill in the blanks with ‘than’ or ‘the’:

1. June is hotter ————– April.
2. This is ———— best book I have.
3. Riding is ———- best kind of exercise.
4. She is more intelligent ———– her sister.
5. A wise enemy is better ———— a foolish friend.
6. Shakespeare was ———— greatest dramatist of England.
Hints:
1. than
2. the
3. the
4. than
5. than
6. the.

II. Complete the following table for Degrees of Comparison:

Positive	Comparative	Superlative
dim	dimmer	dimmest
lazy	lazier	laziest
cool	cooler	coolest
dull	duller	dullest
hard	harder	hardest
wide	wider	widest
good	better	best.
quick	quicker	quickest
clever	cleverer	cleverest
happy	happier	happiest
narrow	narrower	narrowest
greedy	greedier	greediest
naughty	naughtier	naughtiest
important	more important	most important

III. Fill in each blank with the correct form of the given Adjective:

1. Soni is ———– than Neha. (pretty)
2. My bag is ———— than his. (heavy)
3. Today is ———— than yeserday. (cold)
4. Raj has ———– friends than I have. (much)
5. A hare runs ———— than a tortoise. (fast)
6. Rosy is the ———– girl in our town. (beautiful)
7. I am not ———– happy than you are. (little)
8. She is the ————– student in our class. (good)
9. This is the ———— thing I have ever seen. (bad)
Hints:
1. prettier
2. heavier
3. colder
4. more
5. faster
6. most beautiful
7. less
8. best
9. worst.

IV. Point out the Adjective and its Degree of Comparison in each sentence:

1. This is an easy question.
2. Ashoka was a great king.
3. She is wiser than her brother.
4. Mumbai is hotter than Shimla.
5. Neeru was wearing a red frock.
6. Manu got more marks than Rajan.
7. Which is the longest river of India ?
8. Who is the best player of your team?
Hints:
1. easy – positive
2. great – positive
3. wiser – comparative
4. hotter – comparative
5. red – positive
6. more – comparative
7. longest – superlative
8. best – superlative.

V. Supply the proper form of the given Adjectives:

1. Hot : May is ———– than April.
2. Tall : Megha is ————- than her sister.
3. Old : My uncle is ———- than my father.
4. Rich : He is the ————- man in our town.
5. Large : Name the ———— city in the world.
6. Dry : Rajasthan is the part ———- of India.
7. Good : This pen is ————- than any other pen.
8. Sharp : Your knife is sharp, but mine is ———-

Hints:
1. hotter
2. taller
3. older
4. richest
5. largest
6. driest
7. better
8. sharper.

Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 5 Data Handling InText Questions and Answers.

PSEB 8th Class Maths Solutions Chapter 5 Data Handling InText Questions

Textual (Textbook Page No. 69 – 70)

1. A Pictograph : Pictorial representation of data using symbols.

Question (i).
How many cars were produced in the month of July ?
Solution:
250 cars were produced in the month of July.

Question (ii).
In which month were maximum number of cars produced?
Solution:
Maximum number of cars were produced in the month of September.

2. A Bar Graph : A display of information using bars of uniform width, their heights being proportional to the respective values.

Question (i).
What is the information given by the bar graph ?
Solution:
Here, the bar graph gives information about number of students of class VIII in different academic years.

Question (ii).
In which year is the increase in the number of students maximum ?
Solution:
In year 2004-05, the increase in the number of students is maximum.

Question (iii).
In which year is the number of students maximum ?
Solution:
In year 2007 – 08, the number of students is maximum.

Question (iv).
State whether true or false:
‘The number of students during 2005 – 06 is twice that of 2003 – 04.’
Solution :
False, the number of students during 2005 – 06 is not twice that of 2003 – 04 but more than twice.

3. Double Bar Graph : A bar graph showing two sets of data simultaneously. It is useful for the comparison of the data.

Question (i).
What is the information given by the double bar graph?
Solution :
Here, the double bar graph provides information about marks obtained by a student in different subjects and comparison of his marks in year 2005 – 06 and 2006 – 07.

Question (ii).
In which subject has the performance improved the most?
Solution :
In the subject Maths, the performance has improved the most.

Question (iii).
In which subject has the performance deteriorated?
Solution :
In the subject English, the performance has deteriorated.

Question (iv).
In which subject is the performance at par?
Solution:
In the subject Hindi, the performance is at par.

Think, Discuss and Write (Textbook Page No. 71)

Question 1.
If we change the position of any of the bars of a bar graph, would it change the information being conveyed? Why?
Solution:
If the height of a bar remains unchanged, then changing of its position does not change the information being conveyed.

Try These (Textbook Page No. 71)

Question 1.
Draw an appropriate graph to represent the given information:

Solution:
To represent the given data by a bar graph, draw two axes perpendicular to each other. Let us represent ‘Months’ on \(\overrightarrow{\mathrm{OX}}\) and ‘Number of watches sold’ \(\overrightarrow{\mathrm{OY}}\) on OY. Let us make rectangles of same width. The heights of the rectangles are proportional to the number of watches, using a suitable scale :
Here, scale is 1 cm = 500 watches
Since, 500 watches = 1 cm
1000 watches = 2 cm
1500 watches = 3 cm
2000 watches = 4 cm
2500 watches = 5 cm

Question 2.

Children who prefer	School A	School B	School C
Walking	40	55	15
Cycling	45	25	35

Solution:
Since, a comparison of two activities is to be represented, therefore a double graph is drawn by taking the ‘Schools’ along X-axis and ‘Number of children’ along Y-axis, using a scale of 1 cm = 5 children.

Question 3.
Percentage wins in ODI by 8 top cricket teams.

Teams	From Champions Trophy to World Cup ’06	Last 10 ODI in ’07
South Africa	75%	78%
Australia	61 %	40%
Sri Lanka	54%	38%
New Zealand	47%	50%
England	46%	50%
Pakistan	45%	44%
West Indies	44%	30%
India	43%	56%

Solution:
To compare the percentage win in ODI achieved by various teams, we represent the data by a double bar graph. We represent the ‘Team’s Names’ along the X-axis and their ‘percentage win’ along Y-axis, using the scale 1 cm – 5%.

Try These (Textbook Page No. 72)

1. A group of students were asked to say which animal they would like most to have as a pet. The results are given below:
dog, cat, cat, fish, cat, rabbit, dog, cat, rabbit, dog, cat, dog, dog, dog, cat, cow, fish, rabbit, dog, cat, dog, cat, cat, dog, rabbit, cat, fish, dog.
Make a frequency distribution table for the same.
Solution:
Using tally-marks, we have

Try These (Textbook Page No. 73-74)

1. Study the following frequency distribution table and answer the questions given below.
Frequency Distribution of Daily Income of 550 Workers of a factory:

Class Interval (Daily Income in ₹)	Frequency (Number of workers)
100 – 125	45
125 – 150	25
150 – 175	55
175 – 200	125
200 – 225	140
225 – 250	55
250 – 275	35
275 – 300	50
300 – 325	20
Total	550

Question (i).
What is the size of the class intervals ?
Solution:
Class size = [Upper class limit] – [Lower class limit]
= 125 – 100
= 25

Question (ii).
Which class has the highest frequency ?
Solution:
The class 200 – 225 is having the highest frequency (140).

Question (iii).
Which class has the lowest frequency ?
Solution :
The class 300 – 325 has the lowest frequency (20).

Question (iv).
What is the upper limit of the class interval 250 – 275?
Solution:
The upper limit of the class interval 250 – 275 is 275.

Question (v).
Which two classes have the same frequency ?
Solution :
The classes 150 – 175 and 225 – 250 are having the same frequency (55).

2. Construct a frequency distribution table for the data on weights (in kg) of 20 students of a class using intervals 30 – 35, 35 – 40 and so on.
40, 38, 33, 48, 60, 53, 31, 46, 34, 36, 49, 41, 55, 49, 65, 42, 44, 47, 38, 39
Solution:
Lowest observation = 31
Highest observation = 65
Class intervals :
30-35, 35-40, 40-45, ……..
The frequency distribution table for above data can be prepared as follows :

Try These (Textbook Page No. 75 – 76)

1. Observe the histogram and answer questions given below:

Question (i).
What information is being given by the histogram ?
Solution:
This histogram represents the heights (in cms) of girls of class VII.

Question (ii).
Which group contains maximum girls ?
Solution:
The group 140- 145 contains maximum number of girls (7).

Question (iii).
How many girls have a height of 145 cms and more?
Solution:
7 girls have a height of 145 cm and more (4 + 2 + 1 = 7).

Question (iv).
If we divide the girls into the following three categories, how many would there be in each?
150 cm and more-Group A
140 cm to less
than 150 cm – Group B
Less than 140 cm – Group C
Solution:
Number of girls in
Group A : 150 cm and more = 2 + 1 = 3
Group B : 140 cm and less than 150 cm = 7 + 4 = 11
Group C : Less them 140 cm = 3 + 2 + 1 = 6

Try These (Textbook Page No. 78)

1. Each of the following pie charts gives you a different piece of information !; about your class. Find the fraction of the circle representing each of these information:

Solution:
(i) Fraction of the circle representing the ‘girls’ 50 % = \(\frac {50}{100}\) = \(\frac {1}{2}\)
Fraction of the circle representing the ‘boys’ 50% = \(\frac {50}{100}\) = \(\frac {1}{2}\)

(ii) Fraction of the circle representing ‘walk’ 40 % = \(\frac {40}{100}\) = \(\frac {2}{5}\)
Fraction of the circle representing ‘bus or car’ 40 % = \(\frac {40}{100}\) = \(\frac {2}{5}\)
Fraction of the circle representing ‘cycle’ 20 % = \(\frac {20}{100}\) = \(\frac {1}{5}\)

(iii) Fraction of the circle representing those who love mathematics = (100 – 15)%
= 85 %
= \(\frac {85}{100}\) = \(\frac {17}{20}\)
Fraction of the circle representing those who hate mathematics = 15%
= \(\frac {15}{100}\) = \(\frac {3}{20}\)

2. Answer the following questions based on the pie chart given:

(i) Which type of programmes are viewed the most?
(ii) Which two types of programmes have number of viewers equal to those watching sports channels ?
Solution:
From the given pie chart,

Type of viewers	Percentage
Sports viewers	25 %
News viewers	15 %
Information viewers	10 %
Entertainment viewers	50%

(i) The entertainment programmes are viewed the most (50 %).
(ii) The news and informative programmes have the number of viewers equal to those watching sports channels (15 % + 10 % = 25 %).

Try These (Textbook Page No. 81)

1. Draw a pie chart of the data given below : The time spent by a child during a day.
Sleep – 8 hours
School – 6 hours
Homework – 4 hours
Play – 4 hours
Others – 2 hours
Solution:
First we find the central angle corresponding to each of the given activities.

Activity	Duration of the activity in a day out of 24 hours	Central angle corresponding to the activity
Sleep	8 hours	\(\frac {8}{24}\) × 360° = 120°
School	6 hours	\(\frac {6}{24}\) × 360° = 90°
Home­ work	4 hours	\(\frac {4}{24}\) × 360° = 60°
Play	4 hours	\(\frac {4}{24}\) × 360° = 60°
Others	2 hours	\(\frac {2}{24}\) × 360° = 30°

The required pie chart is given below.
[Note: Dividing a circle into sectors with corresponding angle (with protractor) you get the required pie chart.]

Think, Discuss and Write (Textbook Page No. 81)

Which form of graph would be appropriate to display the following data.

Question 1.
Production of food grains of a state.

Solution :
A bar graph will be an appropriate representation to display given data.

Question 2.

Favourite food	Number of people
North Indian	30
South Indian	40
Gujarati	25
Others	25
Total	120

Solution :
A pie chart (circle graph) will be an appropriate representation to display given data.

Question 3.
The daily income of a group of a factory workers:

Daily income (in Rupees)	Number of workers (in a factory)
75 – 100	45
100 – 125	35
125 – 150	55
150 – 175	30

Solution :
A histogram will be an appropriate representation to display given data.

Try These (Textbook Page No. 83 – 84)

Question 1.
If you try to start a scooter, what are the possible outcomes ?
Solution:
It may start.
It may not start.

Question 2.
When a die is thrown, what are the six possible outcomes?
Solution:
When a die is thrown, the possible outcomes are: 1, 2, 3, 4, 5 or 6 on the upper face of the die.

Question 3.
When you spin the wheel shown, what are the possible outcomes ? (Fig 5.9)
List them.
(Outcome here means the sector at which the pointer stops.)

Solution:
When we spin the wheel shown, the possible outcomes are A, B or C.
[Note: The sector at which the pointer stops is outcome.]

Question 4.
You have a pot with five identical balls of different colours and you are to pull out (draw) a ball without looking at it; list the outcomes you would get (Fig 5.10).

Solution:
When we draw a ball from a bag with five identical balls of different colours, the possible outcomes are : W, R, B, G or Y.

Think, Discuss and Write (Textbook Page No. 84)

In throwing a die:

1. Does the first player have a greater chance of getting a six?
Solution:
No.

2. Would the player who played after him have a lesser chance of getting a six?
Solution:
No.

3. Suppose the second player got a six. Does it mean that the third player would not have a chance of getting a six ?
Solution:
No.

Try These (Textbook Page No. 86)

Suppose you spin the wheel:

1. ( i ) List the number of outcomes of getting a green sector and not getting a green sector on this wheel.
Solution:
Number of outcomes of getting a green sector = 5
Number of outcomes of not getting a green sector = 3

(ii) Find the probability of getting a green sector.
Solution :
∴ The total number of outcomes = 8
Number of outcomes of getting a green sector = 5
∴ Probability of getting a green sector = \(\frac {5}{8}\)

(iii) Find the probability of not getting a green sector.
Solution:
∴ The total number of outcomes = 8
Number of outcomes of not getting a green sector = 3
∴ Probability of not getting a green sector = \(\frac {3}{8}\)

Punjab State Board PSEB 10th Class Maths Book Solutions Chapter 15 Probability Ex 15.2 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 10 Maths Chapter 15 Probability Ex 15.2

Question 1.
Two customers Shyam and Ekta are visiting a particular shop in the same week (Tuesday to Saturday). Each is equally likely to visit the shop on any day as on another day. What is the probability that both will visit the shop on
(i) the same day?
(ii) consecutive days?
(iii) different days?
Solution:
When Shyam and Ekta visit a particular shop in the same week. Possible outcomes are:
S = {(T, T) (T, W) (T, Th) (T, F) (T, S) (W, T) (W, W) (W, Th) (W, F) (W, S) (Th, T) (Th, W) (Th, Th) (Th, F) (Th, S) (F, T) (F, W) (F, Th) (F, F) (F, S) (S, T) (S, W) (S, Th) (S, F) (S, S)}

Here T stands For Tuesday
W stands For Wednesday
Th stands For Thursday
F stands For Friday
S stands For Saturday
n(S) = 25
(i) Let A is event that Shyam and Ekta visit the shop on the same day
A = {(T, T) (W, W) (Th, Th) (F, F) (S, S)}
n(A) = 5
Probability that both will visit the shop on same day = \(\frac{5}{25}\)
∴ P(A) = \(\frac{1}{5}\).

(ii) Let B is event that both will visit consecutive days particular shop
B = {(T, W) (W, T) (W, Th) (Th, W) (Th, F) (F, Th) (F, S) (F, S)}
n(B) = 8
∴ Probability that both will visit particular shop on consecutive days = \(\frac{8}{25}\).

(iii) Probability that both will visit the shop on different days = 1 – Probability that both will visit the shop
on same day.
= 1 – \(\frac{1}{5}\) [∵ P \((\bar{A})\) = 1 – P(A)]
= \(\frac{5-1}{5}\)
P \((\bar{A})\) = \(\frac{4}{5}\).

Question 2.
A die, is numbered in such a way that its faces show the numbers 1, 2, 2, 3, 3, 6. It is thrown two times and the total score in two throws is noted. Complete the following table which gives a few values of the total score on the two throws:

What is the probability that the total score is
(i) even?
(ii) 6?
(iii) at least 6?
Solution:
The complete table is

Number of all possible out comes = 6 × 6 = 36
(i) Let A is event of getting total as even
A = {2, 4, 4, 4, 4, 4, 4, 4, 4, 6, 6, 6, 6, 8, 8, 8, 8, 12}
n (A) = 18
∴ Probability of getting an even number = \(\frac{18}{36}=\frac{1}{2}\)
P (Even Number) = \(\frac{1}{2}\).

(ii) Let B is event of getting sum as 6 B = {6, 6, 6, 6)
n(B) = 4
Probability of getting an even number = \(\frac{4}{36}\)
∴ P(B) = \(\frac{1}{9}\).

(iii) Let C is event of getting sum at least 6
C = (6, 6, 6, 6, 7, 7, 8, 8, 8, 8, 9, 9, 9, 9, 12}
n(C) = 15
∴ Probability of getting at least 6 = \(\frac{15}{36}=\frac{5}{12}\)
∴ P(C) = \(\frac{5}{12}\).

Question 3.
A bag contains 5 red balls and some blue balls. lithe probability of drawing a blue ball is double that of a red ball, determine the number of blue balls in the bag.
Solution:
Number of red balls = 5
Let number of blue balls = x
∴ Total number of balls = 5 + x
According to question,
Probability of drawing blue ball = 2 Probability of Red ball
\(\frac{x}{5+x}=2\left[\frac{5}{5+x}\right]\)
x = 10
∴ Number of blue balls = 10.

Question 4.
A box contains 12 balls out of which x are black. If one ball is drawn at random from the box, what is the probability that it will be a black ball?
If 6 more black balls are put in the box, the probability of drawing a black ball is now double of what it was before. Find x.
Solution:
Total number of balls in bag = 12
Number of black balls x
∴ Probability of getting black ball = \(\frac{x}{12}\)
If 6 more balls put in the box then total number of balls in the box = 12 + 6 = 18
Number of black balls = x + 6
Probability of getting black ball = \(\frac{x+6}{18}\)
According to Question,
Probability of drawing black ball = 2
Probability of drawing blackball in first case
\(\frac{x+6}{18}=\frac{2 x}{12}\)

\(\frac{x+6}{3}=\frac{2 x}{2}\)

\(\frac{x+6}{3}\) = x
x + 6 = 3x
6 = 3x – x
6 = 2x
x = 3
∴ Number of black balls = 3.

Question 5.
A jar contains 24 marbles, some are green and others are blue. If a marble is drawn at random from the jar, the probability that it is green is \(\frac{2}{3}\). Find the number of blue marbles in the jar.
Solution:
Total number of marbles in jar =24
Let number of green marbles = x
∴ Number of blue marbles = 24 – x
P (Green marbles) = \(\frac{x}{24}\)
When a marble is drawn
Probability of drawing green marble = \(\frac{2}{3}\) (Given)
\(\frac{x}{24}=\frac{2}{3}\)
x = \(\frac{24 \times 2}{3}\)
x = 16

∴ Number of green marbles = 16
∴ Number of blue marbles = 24 – x = 24 – 16 = 8.

Punjab State Board PSEB 10th Class Maths Book Solutions Chapter 15 Probability Ex 15.1 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 10 Maths Chapter 15 Probability Ex 15.1

Question 1.
Complete the following statements:

(i) Probability of an event E + Probability of the event ‘not E’ = _________.
Solution:
Probability of an event E +
Probability of the event ‘not E’ = 1

(ii) The probability of an event that cannot happen is ___________. Such an event is called _________.
Solution:
The probability of an event that cannot happen is 0. Such an event is called impossible event.

(iii) The probability of an event that is certain to happen is _________. Such an event is called ________.
Solution:
The probability of an event that is certain to happen is 1. Such event is called sure event.

(iv) The sum of the probabilities of all the elementary events of an experiment is __________.
Solution:
The sum of the probabilities of all the elementary events of an experiment is 1.

(v) The probability of an event is greater than or equal to _________ and less than or equal to _________.
Solution:
The probability of an event is greater than or equal to 0 and less than or equal to 1.

Question 2.
Which of the following experiments have equally likely outcomes? Explain.

(i) A driver attempts to start a car. The car starts or does not start.
Solution:
When a dnver attempts to start a car the car starts normally. Only when there is some defects the car does not start. So the outcome is not equally likely.

(ii) A player attempts to shoot a basketball. She/he shoots or misses the shot.
Solution:
When a player attempts to shoot a basketball the outcome in this situation is not equally likely because the outcome depends on many factors such as the training of the player, quality of the gun used etc.

(iii) A trial is made to answer a true – false question. The answer is right or wrong.
Solution:
Since for a question there are two possibilities either right or wrong the outcome in this trial of true-false question is either true or false i.e. one out of the two and both have equal chances to happen. Hence, the two outcomes are equally likely.

(iv) A baby is born. It is a boy or a girl.
Solution:
A new baby (i.e. who took birth at a moment) can be either a boy or a girl and both the outcome have equally likely chances.

Question 3.
Why is tossing a coin considered to he a fair way of deciding which team should get the ball at the beginning of a football game?
Solution:
When a coin is tossed there are only two possibilities i.e. Head or tail both are equally likely to happen. Result of the toss of a fair coin is completely unpredictable.

Question 4.
Which of the following cannot be the probability of an event?
(A) \(\frac{2}{3}\)
(B) – 1.5
(C) 15 %
(D) 0.7
Solution:
As we know probability of event cannot be less than O and greater than 1
i.e. 0 ≤ P ≤ 1
∴ (B) – 1.5 is not possible.

Question 5.
If P(E) = 0.05, what is the probability of not E.
Solution. As we know P (E) + P \((\overline{\mathrm{E}})\) = 1
P\((\overline{\mathrm{E}})\) = 1 – P(E)
= 1 – 0.05 = 0.95.

Question 6.
A bag contains lemon flavoured candies only. Malini takes out one candy without looking into the bag. What is the probability that she takes out
(i) an orange flavoured candy?
(ii) a lemon flavoured candy?
Solution:
(i) Since bag contains only lemon flavoured candies
∴ There is no orange candies
∴ It is impossible event.
∴ Probability of getting orange flavoured = 0.

(ii) Since there are only lemon flavoured candies, it is sure event
∴ Probability, of getting lemon flavoured candy = \(\frac{1}{1}\) = 1.

Question 7.
It is given that in a group of 3 students, the probability of 2 students not having the same birthday is 0.992. What is the probability that the 2 studenís have the same birthday?
Solution:
Let A is event that two students have same birthday
∴ \((\overline{\mathrm{A}})\) is event that 2 students not having same birthday is 0.992
∴ P \((\overline{\mathrm{A}})\) = 0.992
∴ P (A) = 1 – P (A) (P (A) + P \((\overline{\mathrm{A}})\) = 1)
= 1 – 0.992 = 0.008
∴ Probability that two students have saine birthday = 0.008.

Question 8.
A bag contains 3 red balls and 5 black balls. A ball is drawn at random from the bag. What is the probabifity that the ball drawn is
(i) red?
(ii) not red?
Solution:
Number of Red balls = 3
Number of Black balls = 5
Total number of balls = 3 + 5 = 8
One hail is drawn at random
(i) Probability of getting Red ball = \(\frac{\text { Number of favourable cases }}{\text { Total number of cases }}\)
P (Red ball) = \(\frac{3}{8}\).

(ii) Probability of getting not red ball = 1 – P (Red ball)
= 1 – \(\frac{3}{8}\) = \(\frac{3}{8}\) [P \((\overline{\mathrm{A}})\) = 1 – P(E)].

Question 9.
A box contaIns 5 red marbles, 8 white marbles and 4 green marbles. One marble is taken out of the box at random. What is the probability that the marble taken out will be
(i) red ?
(ii) white?
(iii) not green?
Solution:
Number of red marbles = 5
Number of white marbles = 8
Number of green marbles = 4
Total number of marbles = 5 + 8 + 4 = 17
Since, one marble is taken out
(i) There are 5 Red marbles
Probability of drawing Red marble = \(\frac{\text { Number of favourable cases }}{\text { Total number of cases }}\)
= \(\frac{5}{17}\)

(ii) Since there are 8 white marbles
Probability of drawing white marble = \(\frac{\text { Number of favourable cases }}{\text { Total number of cases }}\)
= \(\frac{8}{17}\)

(iii) There are 4 green bails
Probability of drawing green ball = \(\frac{\text { Number of favourable cases }}{\text { Total number of cases }}\)
= \(\frac{4}{17}\)

∴ Probability of not drawing green ball = 1 – Probability of green ball
= 1 – \(\frac{4}{17}\) = \(\frac{17-4}{17}\) = \(\frac{13}{17}\).

Question 10.
A piggy bank contains hundred 50p coins, fifty ₹ 1 coins, twenty ₹ 2 coins and ten ₹ 5 coins. If it is equally likely that one of the coins will fall out when the bank is turned upside down, what is the probability that the coin
(i) will be a 50 p coin?
(ii) will not be a ₹ 5 coin?
Solution;
Number of 50 coins = 100
Number of ₹ 1 coins = 50
Number of ₹ 2 coins =20
Number of ₹ 5 coins = 10
∴ Total number of coins = 100 + 50 + 20 + 10 = 180

(i) Since there are 100 ; 50’ p coin
Probability of getting 50p coin = \(\frac{\text { Number of favourable cases }}{\text { Total number of outcomes }}\)

= \(\frac{100}{180}\)

P (50 p coins) = \(\frac{5}{9}\).

(ii) Number of ₹ 5 coins = 10
∴ Probability of getting ₹ 5 coin = \(\frac{\text { Number of favourable cases }}{\text { Total number of cases }}\)

P (₹ 5 coins) = \(\frac{10}{180}\) = \(\frac{1}{18}\)
Probability of getting not ₹ 5 coin = 1 – P (₹ 5 coins)
= 1 – \(\frac{1}{18}\)
= \(\frac{18-1}{18}\) = \(\frac{17}{18}\).

Question 11.
Gopi buys a fish from a shop for his aquarium. The shopkeeper takes out one fish at random from a tank containing 5 male fish and 8 female fish. What is the probability that the fish taken out is a male fish?

Solution:
Number of male fish = 5
Number of female fish = 8
Total number of fish in the tank = 5 + 8 = 13
Probability of getting a male fish = \(\frac{\text { Number of favourable cases }}{\text { Total number of cases }}\)
P(Male fish) = \(\frac{5}{13}\)

Question 12.
A game of chance consists of spinning an arrow which comes to rest pointing at one of the numbers 1, 2, 3, 4, 5, 6, 7, 8 and these are equally likely outcomes. What is the probability that it will point at
(i) 8?
(ii) an odd number?
(iii) a number greater than 2?
(iv) a number less than 9?

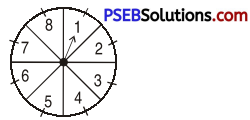
Solution:
(i) Total number of outcomes = {1, 2, 3, 4, 5, 6, 7, 8)
Probability of getting ‘8’ = \(\frac{1}{8}\)

(ii)Odd numbers are = {1, 3, 5, 7)
Probability of getting odd number = \(\frac{4}{8}=\frac{1}{2}\)

(iii) Numbers greater than 2 are {3, 4, 5, 6, 7, 8)
∴ Probability of getting number greater than 2 = \(\frac{6}{8}=\frac{3}{4}\)
P (number greater than 2) = \(\frac{3}{4}\).

(iv) Numbers less than 9 are: {1, 2, 3, 4, 5, 6, 7, 8)
∴ Probability of getting number less than 9 = \(\frac{8}{8}\)
P(a numher less than 9) = 1.

Question 13.
A die is thrown once. Find the probability of getting
(i) a prime number,
(ii) a number lying between 2 and 6;
(iii) an odd number.
Solution:
When dice is thrown number of possible outcomes
S = {1, 2, 3, 4, 5, 6)
(i) Prime numbers are {2, 3, 5)
∴ Probability of getting prime number = \(\frac{3}{6}=\frac{1}{2}\)

(ii) Numbers lying between 2 and 6 = {3, 4, 5}
Probability of getting number between 2 and 6 = \(\frac{3}{6}=\frac{1}{2}\).

(iii) The odd numbers are = {1, 3, 5}
Probability of getting an odd number = \(\frac{3}{6}=\frac{1}{2}\)
P (odd number) = \(\frac{1}{2}\).

Question 14.
One card is drawn from a well. shuffled deck of 52 cards. Find the probability of getting
(i) a king of red colour
(ii) a face card
(iii) a red face card
(iv) the jack of hearts
(v) a spade
(vi) the queen of diamonds.
Solution:
There are 52 cards in a pack
(i) There are two red kings i.e. king of heart and king of diamond
Probability of getting red king = \(\frac{2}{52}=\frac{1}{26}\)
P(Red king) = \(\frac{1}{26}\)

(ii) There are 12 face cards
i.e. 4 Jack, 4 Queens and 4 kings
Probability of getting face card = \(\frac{12}{52}\)
∴ P (A face card) = \(\frac{3}{13}\).

(iii) Since there are 6 Red face cards i.e 2 Jacks; 2 Queens and 2 Kings
∴ Probability of getting 6 Red face cards = \(\frac{6}{52}\)
P (Red face card) = \(\frac{3}{26}\).

(iv) There is only one Jack of Heart
∴ Probability of getting Jack of Heart = \(\frac{1}{52}\)
P (A Jack card) = \(\frac{1}{52}\)

(v) Since there are 13 spade cards
∴ Probability of getting a spade card = \(\frac{13}{52}\)
P (A spade card) = \(\frac{1}{4}\).

(vi) Since there is only one queen of diamonds
∴ Probability of getting queen of spade card = \(\frac{1}{52}\)
P (A queen of spade) = \(\frac{1}{52}\).

Question 15.
Five cards – the ten, jack, queen, king and ace of diamonds, are well-shuffled with their face downwards. One card is then picked up at random.
(i) What is the probability that the card is the queen?
(ii) if the queen is drawn and put aside, what is the probability that the second card picked up is (a) an ace? (b) a queen?
Solution:
Five cards are ten, jack, queen, king and ace
(i) Probability of getting queen = \(\frac{1}{5}\)
∴ P (A queen) = \(\frac{1}{5}\).

(ii) If the queen is drawn and put aside then there are 4 cards left – Ten, a Jack, a king and an ace.
(a) Probability of getting an ace = \(\frac{1}{4}\)
P (An Ace) = \(\frac{1}{4}\).
There’s no queen left

(b) Probability of getting a queen = \(\frac{0}{4}\) = 0
P (a queen) = 0.

Question 16.
12 defective pens are accidentally mixed with 132 good ones. It is not possible to just look at a pen and tell whether or not it is defective. One pen is taken out at random from this lot. Determine the probability that the pen taken out is a good one.
Solution:
Number of defective pens = 12
Number of good pens = 132
∴ Total number of pens = 12 + 132 = 144
Probability of getting good pen = \(\frac{132}{144}=\frac{11}{12}\)
P (a good pen) = \(\frac{11}{12}\).

Question 17.
(i) A lot of 20 bulbs contains 4 defective ones. One bulb is drawn at random from the lot. What is the probability that this bulb is defective?
(ii) Suppose the bulb drawn in
(i) is not defective and is not replaced. Now one bulb is defective and is not replaced. Now one bulb is drawn at random from the rest. What is the probability that this bulb is not defective?
Solution:
(i) Number of defective bulbs 4
Number of good bulbs (Not defective) = 16
Total number of bulbs = 4 + 16 = 20
Probability of getüng defective bulb = \(\frac{4}{20}=\frac{1}{5}\).

(ii) When a defective bulb drawn is not being replaced, we are left with 19 bulbs
Now probability of getting not defective bulb = \(\frac{15}{19}\)
∴ P (Not defective bulb) = \(\frac{15}{19}\)

Question 18.
A box contains 90 discs which are numbered from 1 to 90. If one disc is drawn at random from the box, find the probability that it bears
(i) a two-digit number
(ii) a perfect square number
(iii) a number divisible by 5.
Solution:
From 1 to 90 there are 90 numbers in all and 81 two – digit numbers from 10 to 90
(i) Probability of getting two digit number = \(\frac{81}{90}\)
∴ P (two digit number) = \(\frac{81}{90}=\frac{9}{10}\).

(ii) Perfect square numbers are (1, 4, 9, 16, 25, 36, 49, 64, 81 } there are 9 perfect square numbers between 1 to 90
Probability of getting perfect square = \(\frac{9}{90}=\frac{1}{10}\)
∴ P (Perfect square) = \(\frac{1}{10}\)

(iii) Numbers divisible by 5 are (5, 10, 15, 20, 25, 30, 35, 40, 45. 50, 55. 60, 65, 70, 75, 80, 85, 90}
There are 18 numbers divisible by 5
∴ Probability of number getting divisible by 5 = \(\frac{18}{90}=\frac{1}{5}\)
∴ Required probability = \(\frac{1}{5}\).

Question 19.
A child has a die whose six faces show the letters as given below:

The die is thrown. What is the probability of getting
(i) A ?
(ii) D?
Solution:
Number of faces of a die = 6
S = {A, B, C, D, E, A}
n(S) = 6
(i) Since there are two A’s
∴ Probability of getting A = \(\frac{2}{6}=\frac{1}{3}\)
P(A) = \(\frac{1}{3}\)

(ii) Since there is only one face with D
Probability of getting D = \(\frac{1}{6}\)
∴ P(D) = \(\frac{1}{6}\)

Question 20.
Suppose you drop a die at random on the rectangular region shown in Fig. What is the probability that it will land inside the circle with diameter 1 m?

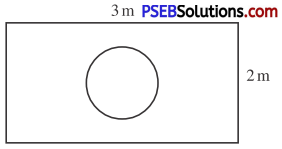
Solution:
Length of rectangle (l) = 3 m
Width of rectangle (b) = 2 m
∴ Area of rectangle = 3 m × 2 m = 6m²
Diameter of circle = 1 m
Radius of circle (R) = \(\frac{1}{2}\) m
∴ Area of circle = πR² = π(\(\frac{1}{6}\))²
= \(\frac{\pi}{4}\) m².

Probability of die to land on a circle = \(\frac{\text { Area of circle }}{\text { Area of rectangle }}\)
= \(\frac{\frac{\pi}{4} \mathrm{~m}^{2}}{6 \mathrm{~m}^{2}}=\frac{\pi}{24}\)
∴ Required Probability = \(\frac{\pi}{24}\).

Question 21.
A lot consists of 144 ball pens of which 20 are défective and the others are good. Nun will buy a pen if it is good, but will not buy it if it is defective. The shopkeeper draws one pen at random and gives it to her. What is the probability that
(i) She will buy it?
(ii) She will not buy it?
Solution:
Total number of Pens in lot = 144
Number of defective Pens = 20
∴ Number of good Pens = 144 – 20 = 124

(i) Let ‘A’ is event showing she buy the pen
∴ Probability that she buy a Pen = \(\frac{124}{144}\)
P(A) = \(\frac{31}{36}\)

(ii) \(\bar{A}\) is event showing that she will not buy the pen
P \((\bar{A})\) = 1 – P(A)
= 1 – \(\frac{31}{36}\) = \(\frac{36-31}{36}\)
∴ P (Not buy the pen) = \(\frac{5}{36}\).

Question 22.
Two dice, one blue and one grey are thrown at the same time. Write down all the possible outcomes
(i) Complete the following table:

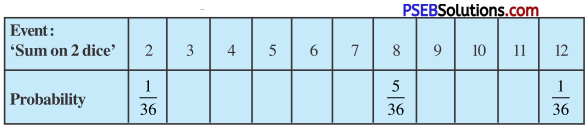
(ii) A student argues that ‘there are 11 possible outcomes 2, 3, 4, 5, 6, 7, 8, 9 10, 11 and 12. Therefore, each of them has a
probability \(\frac{1}{11}\) Do you agree with this argument ? Justify your answer.
Solution:
When two dices are thrown total number of possible outcomes
{(1, 1) (1, 2) (1, 3) (1, 4) (1, 5) (1, 6)
(2, 1) (2, 2) (2, 3) (2, 4) (2, 5) (2, 6)
(3, 1) (3, 2) (3, 3) (3, 4) (3, 5) (3, 6)
S (4, 1) (4, 2) (4, 3) (4, 4) (4,5) (4,6)
(5, 1) (5, 2) (5.3) 5, 4) (5, 5) (5, 6)
(6, 1) (6, 2) (6, 3) (6, 4) (6, 5) (6, 6)}
n(S) = 36
Let A is event of getting sum as 3
∴ A = {(1,2) (2, 1)}
n(A) = 2
∴ Probability of getting sum as 3 = \(\frac{2}{36}=\frac{1}{18}\)
P(A) = \(\frac{1}{18}\)

Let B is event of getting sum as 4 B = ((1, 3), (3, 1), (2, 2))
n(B) = 3
∴ P(B) = \(\frac{3}{36}=\frac{1}{12}\)

Let C is event of getting sum as 5.
C = {(1, 4) (4, 1) (2, 3) (3, 2)}
n(C) = 4
P(C) = \(\frac{4}{36}=\frac{1}{9}\)

Let D is event of getting sum as 6
D = {(1, 5) (5, 1)(2, 4) (4,2) (3, 3)}, n (D) = 5
∴ P(6) = \(\frac{5}{36}\)

Let E is event of getting sum as 7
E = {(1, 6) (6, 1) (2, 5) (5,2) (4, 3) (3, 4)}
∴ P (E) = P (Sum as 7) = \(\frac{6}{36}=\frac{1}{6}\)

Let F is event of getting sum as 8
F = {(2, 6) (6, 2) (3, 5) (4, 4) (5, 3)}
∴ n(F) = 5
P(F) = P(sum as 8) = \(\frac{5}{36}\)

Let G is event of getting sum as 9 when two dices are thrown
G = {(4, 5) (5, 4) (3, 6) (6, 3))
n(G) = 4
∴ P (G) P (Sum as 8) = \(\frac{4}{36}=\frac{1}{9}\)

Let H is event of getting sum as 10
H= {(6, 4) (4, 6) (5, 5)}
n(H) = 3
∴ P (H) = P (sum as 10) = \(\frac{3}{36}=\frac{1}{12}\)

Let I is event of getting sum as 11
I = ((5,6) (6, 5))
n(I) = 2
∴ P(D) = \(\frac{2}{36}=\frac{1}{18}\)

Let J is event of,getting sum as 12
J = {(6,6)}; n(J) = 1
∴ P(J) = \(\frac{1}{36}\)

(ii) No, here all 11 possible outcomes are not equally likely
∴ Three probabilites are different.

Question 23.
A game consists of tossing a one rupee coin 3 times and noting its outcome each time. Hanif wins if all the tosses give the same result i.e. three heads or three tails, and loses otherwise. Calculate the probability that Hanif will lose the game.
Solution:
When a coin tossed three times, then possible out comes are
S = {HHH, HHT HTH, THH, HTF, THT, TTH, TTT)
n(S) = 8
Let A is event of getting all the three same results i.e., {HHH, TTT}
∴ P(A) = \(\frac{2}{8}=\frac{1}{4}\)
Probability of lossing the game = 1 – P (A)
P \((\bar{A})\) = 1 – \(\frac{1}{4}\)
= \(\frac{4-1}{4}\) = \(\frac{3}{4}\)
∴ Probability of losing the game = \(\frac{3}{4}\).

Question 24.
A die is thrown twice. What is the probability that
(i) 5 will not come up either time?
(ii) 5 will come up at least once?
Solution:
When a die is thrown twice all possible outcomes are
S = {(1, 1) (1, 2) (1, 3) (1, 4) (1, 5) (1, 6) (2, 1) (2, 2) (2, 3) (2, 4) (2, 5) (2, 6) (3, 1) (3, 2) (3, 3) (3, 4) (3, 5) (3, 6) (4, 1) (4, 2) (4, 3) (4, 4) 5) (4, 6) (5, 1) (5, 2) (5, 3) (5,4) (5, 5) (5, 6) (6, 1) (6, 2) (6, 3) (6, 4) (6, 5) (6, 6)}
n(S) = 36
Ler A is event that 5 will come up either time
A = {(1, 5) (2, 5) (3, 5) (4, 5) (5, 5) (6, 5) (5, 1) (5, 2) (5, 3) (5, 4) (5, 6)}
n(A) = 11
∴ \((\bar{A})\) is event that 5 will not come up either time.
n\((\bar{A})\) = 36 – 11 = 25.

(i) ∴ Probability of not getting 5 up either time = \(\frac{25}{36}\)
P \((\bar{A})\) = \(\frac{25}{36}\)
Probability that 5 will come up at least once = \(\frac{11}{36}\)
∴ P(A) = \(\frac{11}{36}\).

Question 25.
Which of the following arguments are correct ? Give reasons for your answer:
(i) 1f two coins are tossed simultaneously there are three possible outcomes – two heads, two tails or one of each. Therefore, for each of these outcomes, the probability is \(\frac{1}{3}\):
(ii) If a die is thrown, there are two possible outcomes – an odd number or an even number. Therefore, the probability of getting an odd number is \(\frac{1}{2}\).
Solution:
(i) When two coins are tossed the possible outcomes are S = {HH, HT, TH, TT}
Probability of getting 2 Heads = \(\frac{1}{4}\)
P(HH) = \(\frac{1}{4}\)
Probability of getting two tails = \(\frac{1}{4}\)
P(TT) = \(\frac{1}{4}\)
Probability of getting one head and one tail = \(\frac{2}{4}=\frac{1}{2}\)
∴ (i) argument is incorrect.

(ii) When a die is thrown possible outcomes are S = (1, 2, 3, 4, 5, 6)
n(S) = 6
Odd numbers are 1, 3, 5
∴ Probability of getting odd number = \(\frac{3}{6}=\frac{1}{2}\)
Even numbers are 2, 4, 6
∴ Probability of getting even number = \(\frac{3}{6}=\frac{1}{2}\)
(ii) argument is correct.

Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 11 Mensuration Ex 11.4 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 8 Maths Chapter 11 Mensuration Ex 11.4

1. Given a cylindrical tank, in which situation will you find surface area and in which situation volume:

Question (a)
To find how much it can hold.
Solution:
To find how much a cylindrical tank can hold, we will find its volume.

Question (b)
Number of cement bags required to plaster it.
Solution:
To find number of cement bags required to plaster a cylindrical tank, we will find its surface area.

Question (c)
To find the number of smaller tanks that can be filled with water from it.
Solution:
To find the number of smaller tanks that can be filled with water from it, we will find volume of the tank.

2. Diameter of cylinder A is 7 cm and the height is 14 cm.

Diameter of cylinder B is 14 cm and height is 7 cm. Without doing any calculations can you suggest whose volume is greater? Verify it by finding the volume of both the cylinders. Check whether the cylinder with greater volume also has greater surface area?
Solution:
Radius of cylinder B is double them that of cylinder A.
∴ Volume of cylinder B should be more than that of cylinder A.

For cylinder A:
radius (r) = \(\frac{diameter}{2}\) = \(\frac{7}{2}\)
height (h) = 14 cm
Volume of cylinder A = πr²h
= \(\frac{22}{7}\) × (\(\frac{22}{7}\))² × 14
= \(\frac{22}{7}\) × \(\frac{7}{2}\) × \(\frac{7}{2}\) × 14
= 11 × 7 × 7
= 593 cm³

For cylinder B:

radius (r) = \(\frac{diameter}{2}\) = \(\frac{14}{2}\) = 7cm and
height (h) = 7 cm
Volume of cylinder B = πr²h
= \(\frac{22}{7}\) × 7² × 7
= \(\frac{22}{7}\) × 7 × 7 × 7
= 22 × 7 × 7
= 1078 cm³

Total surface area:
For cylinder A:

radius (r) = \(\frac{7}{2}\) cm
height (h) = 14 cm
Total surface area of cylinder A
= 2πr (r + h)
= 2 × \(\frac{22}{7}\) × \(\frac{7}{2}\)(\(\frac{7}{2}\) +14)
= 22(\(\frac{35}{2}\))
= 11 × 35
= 385 cm²

For cylinder B :
radius (r) = 7 cm, height (h) = 7 cm
Total surface area of cylinder B
= 2πr (r + h)
= 2 × \(\frac {22}{7}\) × 7(7 + 7)
= 44(14) = 616 cm²
So the surface area of cylinder B is greater than that of cylinder A. Hence, the cylinder with greater volume also has greater surface area.

3. Find the height of a cuboid whose base area is 180 cm² and volume is 900 cm³?
Solution:
Let the height of cuboid be h cm.
Now, = Area of base × Height
∴ 900 = 180 × h
∴ h = \(\frac {900}{180}\) = 5cm
Hence, height of cuboid is 5 cm.

4. A cuboid is of dimensions 60 cm × 54 cm × 30 cm. How many small cubes with side 6 cm can be placed in the given cuboid?
Solution:
Volume of a cuboid = 60 × 54 × 30 cm³
Volume of a cube = 6³ = 6 × 6 × 6 cm³
∴ Number of small cubes
= \(\frac{\text { Volume of cuboid }}{\text { Volume of one cube}}\)
= \(\frac{60 \times 54 \times 30}{6 \times 6 \times 6}\)
= 10 × 9 × 5 = 450
Hence, 450 cubes can be placed in the given cuboid.

5. Find the height of the cylinder whose volume is 1.54 m³ and diameter of the base is 140 cm?
Solution:
For given cylinder:
Volume = 1.54 m³
Radius(r) = \(\frac {diameter}{2}\) = \(\frac {140}{2}\) = 70cm = 0.7 m
Volume of cylinder = πr²h
∴ 1.54 = \(\frac {22}{7}\) × 0.7 × 0.7 × h
∴ h = \(\frac{1.54 \times 7}{22 \times 0.7 \times 0.7}\) = 1m
Hence, height of the cylinder is 1 m.

6. A milk tank is in the form of cylinder whose radius is 1.5 m and length is 7 m. Find the quantity of milk in litres that can be stored in the tank?
Solution:
For given cylindrical milk tank:
Radius (r) = 1.5 m = \(\frac {15}{10}\) m
Height (h) = 7m
Volume of cylindrical milk tank
= πr²h
= \(\frac {22}{7}\) × (\(\frac {15}{10}\))² × 7
= \(\frac {22}{7}\) × \(\frac {15}{10}\) × \(\frac {15}{10}\) × 7
= \(\frac{11 \times 3 \times 3}{2}=\frac{99}{2}\) = 49.5m³
= 49.5 m³
1 m³ = 1000 litres
∴ 49.5 m³ = 49.5 × 1000 = 49500 litres
Hence, 49,500 litres of milk can be stored in the tank.

7. If each edge of a cube is doubled:

Question (i)
How many times will its surface area increase?
Solution:
Let the edge of the original cube be x.
Then, its new edge (by doubling) = 2x
Original surface area of the cube
= 6 (side)²
= 6 (x)²
= 6x²
New surface area of the cube
= 6 (side)²
= 6 (2x)²
= 6 (2x × 2x)
= 6 (4x²) = 24X²
= \(\frac{\text { New surface area of the cube }}{\text { Original surface area of the cube }}\) = \(\frac{24 x^{2}}{6 x^{2}}\) = 4
Hence, surface area of a cube will increase 4 times.

Question (ii)
How many times will its volume increase ?
Solution:
Original volume of the cube
= (side)³
= (x)³
= x³
New volume of the cube = (side)³
= (2x)³
= (2x × 2x × 2x)
= 8x³
Now,
\(\frac{\text { New volume of the cube }}{\text { Original volume of the cube }}\) = \(\frac{8 x^{3}}{x^{3}}\) = 8
Hence, volume of the cube will increase 8 times.

8. Water is pouring into a cuboidal reservoir at the rate of 60 litres per minute. If the volume of reservoir is 108 m³, find the number of hours it will take to fill the reservoir.
Solution:
Volume of the cuboidal reservoir =108 m³ 1 m³ = 1000 litres
∴ 108 m³ = 108 × 1000 litres
= 1,08,000 litres
Water poured in a minute = 60 litres
∴ Water poured in an hour
(∵ 1 hour = 60 minutes) = 60 × 60
= 3600 litres
Time taken to fill reservoir = \(\frac{\text { volume of reservoir }}{\text { water poured in an hour }}\) = \(\frac {108000}{3600}\)
= 30 hours
Hence, 30 hours it will take to fill the reservoir.

Punjab State Board PSEB 6th Class English Book Solutions English Grammar Conjunction Exercise Questions and Answers, Notes.

PSEB 6th Class English Grammar Conjunction

A Conjunction is a word which joins two words, phrases or sentences; as-
Conjunction वह शब्द है जो दो शब्दों, शब्द-समूहों अथवा वाक्यों को आपस में जोडता है: जैसे-

1. I know that Raju is a good boy.
2. Ram and Sham are friends.
3. You will fail if you don’t work hard.
4. He wants coffee but I want milk.

Some other Conjunctions of common use are-
because, therefore, yet, for, though, otherwise, so, or

Now look at these sentences:

1. Deepa went to the shop.
She bought a book.
We can combine these two sentences by using ‘and’:
= Deepa went to the shop and bought a book.

2. Anil is thin.
His sister is fat.
= Anil is thin but his sister is fat.

3. He did not go for a walk today.
He is unwell.
= He did not go for a walk today because he is unwell.

4. They played well.
They could not win the match.
= Though they played well, they could not win the match.

Exercises (Solved)

1. Point out the Conjunction in each sentence:

1. He is slow but steady.
2. Nisha and Meera are friends.
3. We went out and had an ice-cream.
4. I missed the train because I was late.
5. Yesterday it rained but today it is sunny.
6. I remember his name but not his address.
7. He bought a radio because he loves music.
8. They went to the market and did some shopping.
Hints:
2. and
3. and
4. because
5. but
6. but.
7. because
8. and.

II. Join each pair of sentences using ‘but’:

1. Seema is tall.
Her brother is short.

2. A bird can fly.
A fish can’t fly.

3. Shimla is cold.
Jaipur is warm.

4. Varun worked hard.
He failed the test.

5. The girls saw a lion.
The lion did not see them.

6. A car has four wheels.
A cycle has two wheels.
Answer:
1. Seema is tall but her brother is short.
2. A bird can fly but a fish cannot.
3. Shimla is cold but Jaipur is warm.
4. Though Varun worked hard, he failed the test.
5. The girls saw a lion but the lion did not see them.
6. A car has four wheels but a cycle has two (wheels).

III. Fill in the blanks with a suitable Conjunction:

1. She bought nothing because she had no money.
2. He was sad ………….. he had failed.
3. June is warm ………….. January is cold.
4. Arun has a car ………….. he can’t drive.
5. Tom …………. Bob always play together.
6. Neha went to Agra …………. saw the Taj.
7. An elephant is big …………. an ant is small.
8. Go to the garden …………. get some flowers.
9. I went to see him …………. he was not at home.
10. I couldn’t make any tea ……….. there was no milk.
Hints:
2. because
3. but
4. but
5. and
6. and
7. but
8. and
9. but
10. because.

IV. Match the columns to make meaningful sentences:

1. He worked hard	but not tea.
2. Kapil likes coffee	yet he failed.
3. I went to see my mother	because she was ill.
4. No one answered the bell	and rested for a while.
5. Tanu and Manu came home	because everyone was out.

Answer:
1. He worked hard yet he failed.
2. Kapil likes coffee but not tea.
3. I went to see my mother because she was ill.
4. No one answered he bell because everyone was out.
5. Tanu and Manu came home and rested for a while.

V. Join each pair of sentences using a suitable Conjunction. You can use one from the box below:

so, if, but, though, therefore, yet, and, because, otherwise, or

1. You will win. You run fast.
2. He was poor. He was honest.
3. I helped him. He is my friend.
4. She was ill. She did not come.
5. He did not work hard. He failed.
6. Anu came early. Bonny came late.
7. Tell me the truth. I will punish you.
8. Is that story true ? Is that story false ?
9. Ravi ran fast. He couldn’t win the race.
10. Amit can read English. Amit can write English.

Answer:
1. You will win if you run fast.
2. He was poor yet he was honest.
3. I helped him because he is my friend.
4. She was ill, therefore, she did not come.
5. She did not work hard, so she failed.
6. Anu came early but Bonny came late.
7. Tell me the truth otherwise I will punish you.
8. Is that story true or false ?
9. Though Ravi ran fast, he could not win the race.
10. Amit can read and write English.

Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 5 Data Handling Ex 5.3 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 8 Maths Chapter 5 Data Handling Ex 5.3

1. List the outcomes you can see in these experiments:

Question (a).
Spinning a wheel

Solution :
Outcomes of spinning the given wheel are :
A, B, C or D.

Question (b).
Tossing two coins together
Solution:
Outcomes in tossing two coins together are : HH, HT, TT, TH
(H = Head, T = Tail)

2. When a die is thrown, list the outcomes of an event of getting

Question (i).
(a) a prime number.
(b) not a prime number.
Solution:
Possible outcomes are: 1, 2, 3, 4, 5 or 6
Prime numbers = 2, 3, 5
(a) Outcomes of getting a prime number are: 2, 3 or 5.
(b) Outcomes of getting not a prime number are : 1, 4 or 6.

(ii) (a) a number greater than 5.
(b) a number not greater than 5.
Solution:
(a) Outcomes of getting a number greater than 5 is 6.
(b) Outcomes of getting a number not greater than 5 are: 1, 2, 3, 4 or 5.

3. Find the:

Question (a).
Probability of the pointer stopping on D in (Question 1 – (a)) ?
Solution:
There are 5 sectors containing A, B, C and D.
Since, there is only 1 sector containing D.
∴ Favourable outcomes = 1
Number of total outcomes = 5
∴ Probability of the pointer stopping on D = \(\frac {1}{5}\)

Question (b).
Probability of getting an ace from a well shuffled deck of 52 playing cards ?
Solution:
Number of possible outcomes = 52
(Total number of cards in a deck = 52)
Since, there are 4 aces in a pack of 52 cards.
∴ Favourable outcomes = 4
∴ Probability of getting an ace = \(\frac{4}{52}=\frac{1}{13}\)

Question (c).
Probability of getting a red apple. (See figure below)

Solution:
Total number of apples = 7
∴ Possible number of ways = 7
Since, there are 4 red apples.
∴ Favourable outcomes = 4
∴ Probability of getting a red apple = \(\frac {4}{7}\)

4. Numbers 1 to 10 are written on ten separate slips (one number on one slip), kept in a box and mixed well. One slip is chosen from the box without looking into it. What is the probability of.

Question (i).
getting a number 6 ?
Solution :
We can get a slip written the number ‘6’ only once.
∴ Number of favourable outcome = 1
Probability of getting the number 6 = \(\frac {1}{10}\)

Question (ii).
getting a number less than 6 ?
Solution:
Numbers less than 6 are 1, 2, 3, 4 and 5. These are five numbers.
∴ Favourable outcomes = 5
Probability of getting the number less than 6 = \(\frac {5}{10}\) = \(\frac {1}{2}\)

Question (iii).
getting a number greater than 6 ?
Solution :
Numbers greater than 6 are 7, 8, 9 and 10. These are four numbers.
∴ Favourable outcomes = 4
Probability of getting the number greater than 6 = \(\frac {4}{10}\) = \(\frac {2}{5}\)

Question (iv).
getting a 1-digit number ?
Solution :
1-digit numbers are 1, 2, 3, 4, 5, 6, 7, 8 and 9. These are nine numbers.
∴ Favourable outcomes = 9
Probability of getting a 1-digit number = \(\frac {9}{10}\)

5. If you have a spinning wheel with 3 green sectors, 1 blue sector and 1 red sector, what is the probability of getting a green sector? What is the probability of getting a non-blue sector?
Solution:
There are 5 sectors in all.
(3 + 1 + 1 = 5)
∴ Number of total possible outcomes = 3
Probability of getting a green colour sector = \(\frac {3}{5}\)
There are 4 non-blue sectors. (5 – 1 = 4)
∴ Number of favourable outcomes = 4
Probability of getting a non-blue sector = \(\frac {4}{5}\)

6. Find the probabilities of the events given in Question 2.
Solution:
There are 6 outcomes.
(i) 2, 3 and 5 are prime numbers. These are three numbers.
∴ Favourable outcomes = 3
Probability of getting a prime number = \(\frac {3}{6}\) = \(\frac {1}{2}\)

(ii) 1, 4 and 6 are non-prime numbers. These are three numbers.
∴ Favourable outcomes = 3
Probability of getting a non-prime number = \(\frac {3}{6}\) = \(\frac {1}{2}\)

(iii) 6 is greater than 5. This is only one number.
∴ Favourable outcome = 1
Probability of a number greater than 5 = \(\frac {1}{6}\)

(iv) 1, 2, 3, 4 and 5 are not greater than 5. There are five numbers.
∴ Favourable outcomes = 5
Probability of a number which is not greater than 5 = \(\frac {5}{6}\)