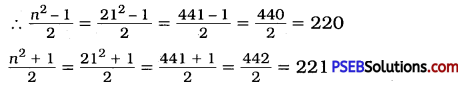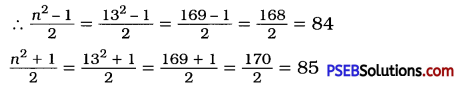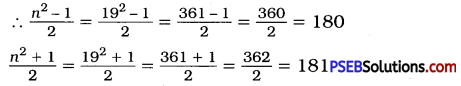Bhagya

Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 7 Cubes and Cube Roots InText Questions and Answers.

PSEB 8th Class Maths Solutions Chapter 7 Cubes and Cube Roots InText Questions

Try These (Textbook Page No. 111)

Find the one’s digit of the cube of each of the following numbers:
(i) 3331
(ii) 8888
(iii) 149
(iv) 1005
(v) 1024
(vi) 77
(vii) 5022
(viii) 53
Solution:

Sl. No.	Number	Number ending in	Units place digit of the cube
(i)	3331	1	1
(ii)	8888	8	2
(iii)	149	9	9
(iv)	1005	5	5
(v)	1024	4	4
(vi)	77	7	3
(vii)	5022	2	8
(viii)	53	3	7

Some interesting patterns: (Textbook Page No. 111)

Observe the following pattern of sums of odd numbers.

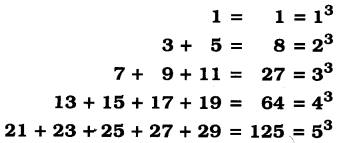
Try These (Textbook Page No. 111)

1. Express the following numbers as the sum of odd numbers using the above pattern ?
(a) 6³
(b) 8³
(c) 7³
Solution:
From above pattern, we can conclude n³ = [n(n – 1) + 1] + [n(n – 1) + 3] + [n(n – 1) + 5]… + n terms
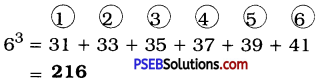
(a) 6³
Here, n = 6, n- 1=5
6 (6 – 1) + 1 → 6 × 5 + 1 → 31

OR
= [6(6 – 1) + 1] + [6(6 – 1) + 3] + [6(6 – 1) + 5] + [6(6 – 1) + 7] + [6(6 – 1) + 9] + [6(6 – 1) + 11]
= (6 × 5 + 1) + (6 × 5 + 3) + (6 × 5 + 5) + (6 × 5 + 7) + (6 × 5 + 9) + (6 × 5 + 11)
= (30 + 1) + (30 + 3) + (30 + 5) + (30 + 7) + (30 + 9) + (30 + 11)
= 31 +33 + 35 + 37 + 39 + 41
= 216

(b) 8³
Here, n = 8, n – 1 = 7
8 (8 – 1) + 1 → 8 × 7 + 1 → 57

OR
= [8(8 – 1) + 1] + (8(8 – 1) + 3] + [8(8 – 1) + 5] + [8(8 – 1) + 7] + [8(8 – 1) + 9] + [8(8 – 1) + 11] + [8(8 – 1) + 13] + [8(8 – 1) + 15]
= (8 × 7 + 1) + (8 × 7 + 3) + (8 × 7 + 5) + (8 × 7 + 7) + (8 × 7 + 9) + (8 × 7 + 11) + (8 × 7 + 13) + (8 × 7 + 15)
= (56 + 1) + (56 + 3) + (56 + 5) + (56 + 7) + (56 + 9) + (56 + 11) + (56 + 13) + (56 + 15)
= 57 + 59 + 61 + 63 + 65 + 67 + 69 + 71
= 512

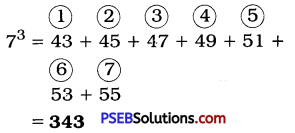
(c) 7³
Here, n = 7, n – 1 = 6
7 × 6 + 1 → 42 + 1 → 43

OR
= [7(7 – 1) + 1] + [7(7 – 1) + 3] + [7(7 – 1) + 5] + [7(7 – 1) + 7] + [7(7 – 1) + 9] + [7(7 – 1) + 11] + [7(7 – 1) + 13]
= (7 × 6 + 1) + (7 × 6 + 3) + (7 × 6 + 5) + (7 × 6 + 7) + (7 × 6 + 9) + (7 × 6 + 11) + (7 × 6 + 13)
= (42 + 1) + (42 + 3) + (42 + 5) + (42 + 7) + (42 + 9) + (42 + 11) + (42 + 13)
= 43 + 45 + 47 + 49 + 51 + 53 + 55
= 343

Consider the following pattern:
2³ – 1³ = 1 + 2 × 1 × 3
3³ – 2³ = 1 + 3 × 2 × 3
4³ – 3³ = 1 + 4 × 3 × 3
Using the above pattern, find the value of the following:
(i) 7³ – 6³
(ii) 12³– 11³
(iii) 20³ – 19³
(iv) 51³ – 50³
Solution:
From above pattern, we can conclude
n³ – (n – 1)³ = 1 + n × (n – 1) × 3
(i) 7³ – 6³ = 1 + 7 × 6 × 3
= 1 + 126
= 127

(ii) 12³ – 11³ = 1 + 12 × 11 × 3
= 1 + 396
= 397

(iii) 20³ – 19³ = 1 + 20 × 19 × 3
= 1 + 1140
= 1141

(iv) 51³ – 50³ = 1 + 51 × 50 × 3
= 1 + 7650
= 7651

Try These (Textbook Page No. 112)

1. Which of the following are perfect cubes?

Question (1).
400
Solution:
\(\begin{array}{l|l}
2 & 400 \\
\hline 2 & 200 \\
\hline 2 & 100 \\
\hline 2 & 50 \\
\hline 5 & 25 \\
\hline 5 & 5 \\
\hline & 1
\end{array}\)
400 = 2 × 2 × 2 × 2 × 5 × 5
Here, the prime factors 2 and 5 do not appear in triples.
∴ 2 × 5 × 5 is left over.
∴ 400 is not a perfect cube.

Question (2).
3375
Solution:
\(\begin{array}{l|l}
3 & 3375 \\
\hline 3 & 1125 \\
\hline 3 & 375 \\
\hline 5 & 125 \\
\hline 5 & 25 \\
\hline 5 & 5 \\
\hline & 1
\end{array}\)
3375 = 3 × 3 × 3 × 5 × 5 × 5
Here, the prime factors 3 and 5 appear in triples.
No factor is left over.
∴ 3375 is a perfect cube.
3375 = 3³ × 5³

Question (3).
8000
Solution:
\(\begin{array}{l|l}
2 & 8000 \\
\hline 2 & 4000 \\
\hline 2 & 2000 \\
\hline 2 & 1000 \\
\hline 2 & 500 \\
\hline 2 & 250 \\
\hline 5 & 125 \\
\hline 5 & 25 \\
\hline 5 & 5 \\
\hline & 1
\end{array}\)
8000 = 2 × 2 × 2 × 2 × 2 × 2 × 5 × 5 × 5
Here, the prime factors 2 and 5 appear in triples.
No factor is left over.
∴ 8000 is a perfect cube.
8000 = 2³ × 2³ × 5³

Question (4).
15625
Solution:
\(\begin{array}{l|l}
5 & 15625 \\
\hline 5 & 3125 \\
\hline 5 & 625 \\
\hline 5 & 125 \\
\hline 5 & 25 \\
\hline 5 & 5 \\
\hline & 1
\end{array}\)
15625 = 5 × 5 × 5 × 5 × 5 × 5
Here, the prime factor 5 appear in triples.
No factor is left over.
∴ 15625 is a perfect cube.
15625 = 5³ × 5³

Question (5).
9000
Solution:
\(\begin{array}{l|l}
2 & 9000 \\
\hline 2 & 4500 \\
\hline 2 & 2250 \\
\hline 3 & 1125 \\
\hline 3 & 375 \\
\hline 5 & 125 \\
\hline 5 & 25 \\
\hline 5 & 5 \\
\hline & 1
\end{array}\)
9000 = 2 × 2 × 2 × 3 × 3 × 5 × 5 × 5
Here, among the prime factors 2 and 5 appear in triples but 3 does not appear in triple.
3 × 3 is left over.
∴ 9000 is not a perfect cube.

Question (6).
6859
Solution:
\(\begin{array}{l|l}
19 & 6859 \\
\hline 19 & 361 \\
\hline 19 & 19 \\
\hline & 1
\end{array}\)
6859 = 19 × 19 × 19
Here, the prime factor 19 appears in triple.
No factor is left over.
∴ 6859 is a perfect cube.
6859 = 193

Question (7).
2025
Solution:
\(\begin{array}{l|l}
3 & 2025 \\
\hline 3 & 675 \\
\hline 3 & 225 \\
\hline 3 & 75 \\
\hline 5 & 25 \\
\hline 5 & 5 \\
\hline & 1
\end{array}\)
2025 = 3 × 3 × 3 × 3 × 5 × 5
Here, the prime factor 3 appears in triple, but 3 × 5 × 5 is left over.
∴ 2025 is not a perfect cube.

Question (8).
10648
Solution:
\(\begin{array}{r|l}
2 & 10648 \\
\hline 2 & 5324 \\
\hline 2 & 2662 \\
\hline 11 & 1331 \\
\hline 11 & 121 \\
\hline 11 & 11 \\
\hline & 1
\end{array}\)
10648 = 2 × 2 × 2 × 11 × 11 × 11
Here, the prime factors 2 and 11 appear in triples.
No factor is left over.
∴ 10648 is a perfect cube.
10648 = 2³ × 11³

Think, Discuss and Write (Textbook Page No. 113)

1. Check which of the following are perfect cubes:
(i) 2700
(ii) 16000
(iii) 64000
(iv) 900
(v) 125000
(vi) 36000
(vii) 21600
(viii) 10000
(ix) 27000000
(x) 1000
What pattern do you observe in these perfect cubes ?
Solution:
(i) 2700
The number is ending with two zeros. If a number ends with three zeros or a multiple of 3 zeros, it may be a perfect cube.
∴ 2700 is not a perfect cube.

(ii) 16000
The number is ending with three zeros.
So it may be a perfect cube.
But, 16 is not a perfect cube.
∴ 16000 is not a perfect cube.

(iii) 64000
The number is ending with three zeros.
So it may be a perfect cube.
64 is a perfect cube. (∵ 4³ = 64)
∴ 64000 is a perfect cube.

(iv) 900
The number is ending with two zeros.
So it is not a perfect cube.
∴ 900 is not a perfect cube.

(v) 125000
The number is ending with three zeros.
So it may be a perfect cube.
125 is a perfect cube. (∵ 5³ = 125)
∴ 125000 is a perfect cube.

(vi) 36000
The number is ending with three zeros.
So it may be a perfect cube.
But, 36 is not a perfect cube.
∴ 36000 is not a perfect cube.

(vii) 21600
The number is ending with two zeros.
So it is not a perfect cube.
∴ 21600 is not a perfect cube.

(viii) 10000
The number is ending with four zeros.
So it is not a perfect cube.
∴ 10000 is not a perfect cube.

(ix) 27000000
The number is ending with six zeros.
So it may be a perfect cube.
27 is a perfect cube. (∵ 3³ = 27)
∴ 27000000 is a perfect cube.

(x) 1000
The number is ending with three zeros.
So it may be a perfect cube.
1 is a perfect cube, (∵ 1³ = 1)
∴ 1000 is a perfect cube.

Think, Discuss and Write (Textbook Page No. 115)

1. State true or false for any integer m, m² < m³. Why ?
Solution:
It seems true, but not always true.
m × m = m² and m × m × m = m³
∴ m² < m³
e.g. if m = 1
∴ m² = 1² = 1 and m³ = 1³ = 1
∴ m² ≮  m³, but m² = m³
If m = (- 1)
∴ m² = (- 1)² = 1 and m³ = (- 1)³ = (- 1)
∴ m² ≮  m³, but m2 > m³
So the above statement is not always true.

Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 9 Algebraic Expressions and Identities Ex 9.4 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 8 Maths Chapter 9 Algebraic Expressions and Identities Ex 9.4

1. Multiply the binomials:

Question (i)
(2x + 5) and (4x – 3)
Solution:
= (2x + 5)(4x – 3)
= 2x(4x – 3) + 5 (4x – 3)
= 8x² – 6x + 20x – 15
= 8x² + 14x – 15

Question (ii)
(y – 8) and (3y – 4)
Solution:
= (y -8) (3y – 4)
= y (3y – 4) – 8 (3y – 4)
= 3y² – 4y – 24y + 32
= 3y² – 28y + 32

Question (iii)
(2.51 – 0.5m) and (2.51 + 0.5m)
Solution:
= (2.5l – 0.5m) (2.5l + 0.5m)
= 2.5l(2.5l + 0.5m) – 0.5m (2.5l + 0.5m)
= 6.25l² + 1.25lm – 1.25lm – 0.25m²
= 6.25l² – 0.25m²

Question (iv)
(a + 3b) and (x + 5)
Solution:
= (a + 3b) (x + 5)
= a (x + 5) + 3b (x + 5)
= ax + 5a + 3bx + 15b

Question (v)
(2pq + 3q²) and (3pq – 2q²)
Solution:
= (2pq + 3q²) (3pq – 2q²)
= 2pq (3pq – 2q²) + 3q² (3pq – 2q²)
= 6p²q² – 4pq³ + 9pq³ – 6q⁴
= 6p²q² + 5pq³ – 6q⁴

Question (vi)
(\(\frac {3}{4}\)a² + 3b²) and 4 (a² – \(\frac {2}{3}\)b²)
Solution:
= (\(\frac {3}{4}\)a² + 3b²) (4a² – \(\frac {8}{3}\)b²)
= \(\frac {3}{4}\)a⁴ (4a² – \(\frac {8}{3}\)b²) + 3b² (4a² – \(\frac {8}{3}\)b²)
= 3a₄ – 2a²b² + 12a²b² – 8b₄
= 3a⁴ + 10a²b² – 8b⁴

2. Find the product:

Question (i)
(5 – 2x) (3 + x)
Solution:
= 5 (3 + x) – 2x (3 + x)
= 15 + 5x – 6x – 2x²
= 15 – x – 2x²

Question (ii)
(x + 7y) (7x – y)
Solution:
= x(7x – y) + 7y (7x – y)
= 7x² – xy + 49xy – 7y²
= 7x² + 48xy – 7y²

Question (iii)
(a² + b) (a + b²)
Solution:
= a² (a + b²) + b (a + b²)
= a³ + a²b² + ab + b³

Question (iv)
(p² – q²) (2p + q)
Solution:
= p²(2p + q) – q²(2p + q)
= 2p³ + p²q – 2pq² – q³

3. Simplify:

Question (i)
(x² – 5) (x + 5) + 25
Solution:
= x² (x + 5) – 5 (x + 5) + 25
= x³ + 5x² – 5x – 25 + 25
= x³ + 5x² – 5x

Question (ii)
(a² + 5) (b³ + 3) + 5
Solution:
= a²(b³ + 3) + 5 (b³ + 3) + 5
= a²b³ + 3a² + 5b³ + 15 + 5
= a²b³ + 3a² + 5b³ + 20

Question (iii)
(t + s²)(t² – s)
Solution:
= t (t² – s) + s² (t² – s)
= t³ – st + s²t² – s³

Question (iv)
(a + b) (c – d) + (a – b) (c + d) + 2 (ac + bd)
Solution:
= a(c – d) + b(c – d) + a(c + d) – b(c + d) + 2 (ac + bd)
= ac – ad + bc – bd + ac + ad – bc – bd + 2ac + 2bd
= ac + ac + 2ac – ad + ad + bc – bc – bd – bd + 2 bd
= 4 ac

Question (v)
(x + y) (2x + y) + (x + 2y) (x – y)
Solution:
= x (2x + y) + y (2x + y) +x(x – y) + 2y (x – y)
= 2x² + xy + 2xy + y² + x² – xy + 2xy – 2y²
= 2x² + x² + xy + 2xy – xy + 2xy + y² – 2y²
= 3x² + 4xy – y²

Question (vi)
(x + y) (x² – xy + y²)
Solution:
= x (x² – xy + y²) + y (x² – xy + y²)
= x³ – x²y + xy² + x²y – xy² + y³
= x³ – x²y + x²y + xy² – xy² + y³
= x³ + y³

Question (vii)
(1.5x – 4y) (1.5x + 4y + 3) – 4.5x + 12y
Solution:
= 1.5x (1.5x + 4y + 3) – 4y (1.5x + 4y + 3) – 4.5x + 12y
= 2.25x² + 6xy + 4.5x – 6xy – 16y² – 12y – 4.5x + 12y
= 2.25x² + 6xy – 6xy + 4.5x – 4.5x – 16y² – 12y + 12y
= 2.25x² – 16y²

Question (viii)
(a + b + c) (a + b – c)
Solution:
= a (a + b – c) + b (a + b – c) + c (a + b – c)
= a² + ab – ac + ab + b² – bc + ac + bc – c²
= a² + ab + ab – ac + ac + b² – bc + bc – c²
= a² + 2ab + b² – c²
= a² + b² – c² + 2 ab

Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 7 Cubes and Cube Roots Ex 7.2 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 8 Maths Chapter 7 Cubes and Cube Roots Ex 7.2

1. Find the cube root of each of the following numbers by prime factorisation method.

Question (i).
64
Solution:
\(\begin{array}{l|l}
2 & 64 \\
\hline 2 & 32 \\
\hline 2 & 16 \\
\hline 2 & 8 \\
\hline 2 & 4 \\
\hline 2 & 2 \\
\hline & 1
\end{array}\)
By prime factorisation,
64 = 2 × 2 × 2 × 2 × 2 × 2
∴ \(\sqrt[3]{64}\) = 2 × 2
= 4
Thus, cube root of 64 is 4.

Question (ii).
512
Solution:
\(\begin{array}{l|l}
2 & 512 \\
\hline 2 & 256 \\
\hline 2 & 128 \\
\hline 2 & 64 \\
\hline 2 & 32 \\
\hline 2 & 16 \\
\hline 2 & 8 \\
\hline 2 & 4 \\
\hline 2 & 2 \\
\hline & 1
\end{array}\)
By prime factorisation,
512 = 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2
∴ \(\sqrt[3]{512}\) = 2 × 2 × 2
= 8
Thus, cube root of 512 is 8.

Question (iii).
10648
Solution:
\(\begin{array}{r|l}
2 & 10648 \\
\hline 2 & 5324 \\
\hline 2 & 2662 \\
\hline 11 & 1331 \\
\hline 11 & 121 \\
\hline 11 & 11 \\
\hline & 1
\end{array}\)
By prime factorisation,
10648 = 2 × 2 × 2 × 11 × 11 × 11
∴ \(\sqrt[3]{10648}\) = 2 × 11
= 22
Thus, cube root of 10648 is 22.

Question (iv).
27000
Solution:
\(\begin{array}{l|l}
2 & 27000 \\
\hline 2 & 13500 \\
\hline 2 & 6750 \\
\hline 3 & 3375 \\
\hline 3 & 1125 \\
\hline 3 & 375 \\
\hline 5 & 125 \\
\hline 5 & 25 \\
\hline 5 & 5 \\
\hline & 1
\end{array}\)
By prime factorisation
27000 = 2 × 2 × 2 × 3 × 3 × 3 × 5 × 5 × 5
∴ \(\sqrt[3]{27000}\) = 2 × 3 × 5
= 30
Thus, cube root of 27000 is 30.

Question (v).
15625
Solution:
\(\begin{array}{l|l}
5 & 15625 \\
\hline 5 & 3125 \\
\hline 5 & 625 \\
\hline 5 & 125 \\
\hline 5 & 25 \\
\hline 5 & 5 \\
\hline & 1
\end{array}\)
By prime factorisation,
15625 = 5 × 5 × 5 × 5 × 5 × 5
∴ \(\sqrt[3]{15625}\) = 5 × 5
= 25
Thus, cube root of 15625 is 25.

Question (vi).
13824
Solution:
\(\begin{array}{l|l}
2 & 13824 \\
\hline 2 & 6912 \\
\hline 2 & 3456 \\
\hline 2 & 1728 \\
\hline 2 & 864 \\
\hline 2 & 432 \\
\hline 2 & 216 \\
\hline 2 & 108 \\
\hline 2 & 54 \\
\hline 3 & 27 \\
\hline 3 & 9 \\
\hline 3 & 3 \\
\hline & 1
\end{array}\)
By prime factorisation,
13824 = 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 × 3 × 3 × 3
∴ \(\sqrt[3]{13824}\) = 2 × 2 × 2 × 3
= 24

Question (vii).
110592
Solution:
\(\begin{array}{l|l}
2 & 110592 \\
\hline 2 & 55296 \\
\hline 2 & 27648 \\
\hline 2 & 13824 \\
\hline 2 & 6912 \\
\hline 2 & 3456 \\
\hline 2 & 1728 \\
\hline 2 & 864 \\
\hline 2 & 432 \\
\hline 2 & 216 \\
\hline 2 & 108 \\
\hline 2 & 54 \\
\hline 3 & 27 \\
\hline 3 & 9 \\
\hline 3 & 3 \\
\hline & 1
\end{array}\)
By prime factorisation,
110592 = 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 × 3 × 3 × 3
∴ \(\sqrt[3]{110592}\) = 2 × 2 × 2 × 2 × 3
= 48
Thus, cube root of 110592 is 48.

Question (viii).
46656
Solution:
\(\begin{array}{l|l}
2 & 46656 \\
\hline 2 & 23328 \\
\hline 2 & 11664 \\
\hline 2 & 5832 \\
\hline 2 & 2916 \\
\hline 2 & 1458 \\
\hline 3 & 729 \\
\hline 3 & 243 \\
\hline 3 & 81 \\
\hline 3 & 27 \\
\hline 3 & 9 \\
\hline 3 & 3 \\
\hline & 1
\end{array}\)
By prime factorisation,
46656 = 2 × 2 × 2 × 2 × 2 × 2 × 3 × 3 × 3 × 3 × 3 × 3
∴ \(\sqrt[3]{46656}\) = 2 × 2 × 3 × 3
= 36
Thus, cube root of 46656 is 36.

Question (ix).
175616
Solution:
\(\begin{array}{l|l}
2 & 175616 \\
\hline 2 & 87808 \\
\hline 2 & 43904 \\
\hline 2 & 21952 \\
\hline 2 & 10976 \\
\hline 2 & 5488 \\
\hline 2 & 2744 \\
\hline 2 & 1372 \\
\hline 2 & 686 \\
\hline 7 & 343 \\
\hline 7 & 49 \\
\hline 7 & 7 \\
\hline & 1
\end{array}\)
By prime factorisation,
175616 = 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 × 7 × 7 × 7
∴ \(\sqrt[3]{175616}\) = 2 × 2 × 2 × 7
= 56
Thus, cube root of 175616 is 56.

Question (x).
91125
Solution:
\(\begin{array}{l|l}
3 & 91125 \\
\hline 3 & 30375 \\
\hline 3 & 10125 \\
\hline 3 & 3375 \\
\hline 3 & 1125 \\
\hline 3 & 375 \\
\hline 5 & 125 \\
\hline 5 & 25 \\
\hline 5 & 5 \\
\hline & 1
\end{array}\)
By prime factorisation,
91125 = 3 × 3 × 3 × 3 × 3 × 3 × 5 × 5 × 5
∴ \(\sqrt[3]{91125}\) =3 × 3 × 5
= 45
Thus, cube root of 91125 is 45.

2. State true or false.

Question (i).
Cube of any odd number is even.
Solution:
False, cube of any odd number is odd.

Question (ii).
A perfect cube does not end with two zeros.
Solution:
True, a perfect cube ending with zero will always have a triplet of zeros.

Question (iii).
If square of a number ends with 5, then its cube ends with 25.
Solution:
False, let us understand with an example.
15² = 15 × 15 = 225
15³ = 15 × 15 × 15 = 3375

Question (iv).
There is no perfect cube which ends with 8.
Solution:
False, let us understand with an example.
8 = 2³, 1728 = 12³

Question (v).
The cube of a two-digit number may be a three-digit number.
Solution:
False, let us take an example.
The smallest two-digit number is 10.
10³ = 1000
which is a four-digit number and not a three-digit number.

Question (vi).
The cube of a two-digit number may have seven or more digits.
Solution:
False, let us take an example.
The greatest two-digit number is 99.
99³ = 970299
which is a six-digit number.

Question (vii).
The cube of a single digit number may be a single digit number.
Solution:
True, let us take examples.
1³ = 1 and 2³ = 8

3. You are told that 1,331 is a perfect cube. Can you guess without factorisation what is its cube root? Similarly, guess the cube roots of 4913, 12167, 32768.
Solution:
Yes, we can guess without prime factorisation.
1331 : Separate given number into two groups.
1331 → 1 and 331
331 → Units place digit of 331 is 1.
∴ Unit place digit of cube root of 1331 = 1 (∵ 1³ = 1)
1 → 1³ = 1 and 2³ = 8
∴ Tens digit of cube root of 1331 = 1
Thus, the cube root of 1331 is 11.

(i) 4913 : Separate given number into two groups.
4913 → 4 and 913
913 → Units place digit of 913 is 3.
∴ Unit digit of cube root of 4913 = 7 (∵ 7³ = 343)
4 → 1³ = 1 and 2³ = 8
1 < 4 < 8 (∴ 1³ < 4 < 2³)
∴ The tens digit of cube root of 4913 = 1
Thus, the cube root of 4913 is 17.

(ii) 12167 : Separate given number into two groups.
12167 → 12 and 167
167 → Units place digit of 167 is 7.
∴ Unit digit of cube root of 12167 = 3
(∵ 3³ = 27)
12 → 2³ = 8 and 3³ = 27
8 < 12 < 27 (∵ 2³ < 12 < 3³)
∴ Tens place digit of cube root of 12167 = 2
Thus, the cube root of 12167 is 23.

(iii) 32768 : Separate given number into two groups.
32768 → 32 and 768
768 → Units place digit of 768 is 8.
∴ Unit digit of cube root of 32768 = 2 (∵ 2³ = 8)
32 → 3³ = 27 and 4³ = 64
27 < 32 < 64 (∵ 3³ < 32 < 4³)
∴ The tens digit of cube root of 32768 = 3
Thus, the cube root of 32768 is 32.

Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 9 Algebraic Expressions and Identities Ex 9.3 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 8 Maths Chapter 9 Algebraic Expressions and Identities Ex 9.3

1. Carry out the multiplication of the expressions in each of the following pairs:

Question (i)
4p, q + r
Solution:
= 4p × (q + r)
= (4p × q) + (4p × r)
= 4pq + 4pr

Question (ii)
ab, a – b
Solution:
= ab × (a-b)
= (ab × a) – (ab × b)
= a²b – ab²

Question (iii)
a + b, 7a²b²
Solution:
= (a + b) × 7a²b²
= (a × 7a²b²) + (b × 7a²b²)
= 7 a³b² + 7a²b³

Question (iv)
a² – 9, 4a
Solution:
= (a² – 9) × 4a
= (a² × 4a) – (9 × 4a)
= 4a³ – 36a

Question (v)
pq + qr + rp, 0
Solution:
= (pq + qr + rp) × 0
= 0

2. Complete the table:

First expression	Second expression	Product
1. a	b + c + d	……………
2. x + y- 5	5xy	……………
3. P	6p2 – 7p + 5	…………..
4. 4p2q- q2	p2 – q2	…………..
5. a + b + c	abc	………….

Solution:
(i) a × (b + c + d)
= (a × b) + (a × c) + (a × d)
= ab + ac + ad

(ii) (x + y – 5) × 5xy
= (x × 5xy) + (y × 5xy) + [(- 5) × 5xy]
= 5x²y + 5xy² – 25xy

(iii) p × (6p² – 7p + 5)
= (p × 6p²) + [p × (- 7p)] + (p × 5)
= 6p³ – 7p² + 5p

(iv) 4p²q² × (p² – q²)
= (4p²q² × p²) + [4p²q² × (-q²)]
= 4p⁴q² – 4p²q⁴

(v) (a + b + c) × abc
= (a × abc) + (b × abc) + (c × abc)
= a²bc + ab²c + abc²

3. Find the product:

Question (i)
(a²) × (2a²²) × (4a²⁶)
Solution:
= (1 × 2 × 4) × a² × a²² × a²⁶
= 8 × a⁵⁰
= 8a⁵⁰

Question (ii)
(\(\frac {2}{3}\)xy) × (\(\frac {-9}{10}\)x²y²)
Solution:
= \(\frac {2}{3}\) × (\(\frac {-9}{10}\)) × xy × x²y²
= \(\frac {-2}{3}\) × \(\frac {9}{10}\) × x³y³
= \(\frac {-3}{5}\)x³y³

Question (iii)
(\(\frac {-10}{3}\)pq³) × (\(\frac {6}{5}\)p³q)
Solution:
= [(\(\frac {-10}{3}\)) × \(\frac {6}{5}\)] × pq³ × p³q
= – \(\frac {10}{3}\) × \(\frac {6}{5}\) × p⁴q⁴
= – 4p⁴q⁴

Question (iv)
x × x² × x³ × x⁴
Solution:
= (1 × 1 × 1 × 1) × x × x² × x³ × x⁴
= (1) × x¹⁰
= x¹⁰

4.

Question (a)
Simplify 3x(4x – 5) + 3 and find its value for
(i) x = 3
(ii) x = \(\frac {1}{2}\)
Solution:
3x (4x- 5) + 3
= (3x × 4x) – (3x × 5) + 3
= 12x¹⁰ – 15x + 3

(i) When x = 3, then
12x² – 15x + 3
= 12 (3)² – 15(3) + 3
= 12 (9) – 15 (3) + 3
= 108 -45 + 3
= 111 – 45
= 66

(ii) x = \(\frac {1}{2}\), then
12x² – 15x + 3
= 12(\(\frac {1}{2}\))² – 15(\(\frac {1}{2}\)) + 3
= 12(\(\frac {1}{4}\)) – 15(\(\frac {1}{2}\)) + 3
= 3 – \(\frac {15}{2}\) + 3
= 6 – \(\frac {15}{2}\)
= \(\frac{12-15}{2}\)
= \(\frac {-3}{2}\)

Question (b)
Simplify a (a2 + a + 1) + 5 and find its values for
(i) a = 0 (ii) a = 1 (iii) a = (-1)
Solution:
a (a² + a + 1) + 5
= (a × a²) + (a × a) + (a × 1) + 5
= a³ + a² + a + 5

(i) When a = 0, then
a³ + a² + a + 5
= (-1)³ + (0)³ + (0) + 5
= 0 + 0 + 0 + 5
= 5

(ii) a = 1, then
a³ + a² + a + 5
= (1)³ + (1)² + (1) + 5
= 1 + 1 + 1 + 5
= 8

(iii) a = (-1), then
a³ + a² + a + 5
= (-1)³ + (-1)² + (-1) + 5
= (-1) + (1) + (-1) + 5
= 6 – 2 = 4

5.

Question (a)
Add : p(p – q), q(q – r) and r(r – p)
Solution:
[p(p-q)] + [q(q-r)] + [r(r-p)]
= p² – pq + q² – qr + r² – rp
= p² + q² + r² – pq – qr – rp

Question (b)
Add : 2x(z – x – y) and 2y(z – y – x)
Solution:
[2x (z – x – y)] + [2y (z – y – x)]
= 2xz – 2x² – 2xy + 2yz – 2y² – 2xy
= – 2x² – 2y² – 2xy – 2xy + 2yz + 2xz
= – 2x² – 2y² – 4xy + 2yz + 2xz

Question (c)
Subtract : 31(l – 4m + 5n) from 4l(10n – 3m + 2l)
Solution:
[4l(10n – 3m + 21)] – [3l(l – 4m + 5n)]
= [40ln – 12lm + 8l²] – [3l² – 12lm + 15ln]
= 40ln – 12lm + 8l² – 3l² + 12lm – 15ln
= 40ln – 15ln – 12lm + 12lm + 8l² – 3l²
= 25ln + 0lm + 5l²
= 25ln + 5l²

Question (d)
Subtract : 3a(a + b + c) – 2b(a – b + c) from 4c(- a + b + c)
Solution:
[4c (- a + b + c)] – [3a (a + b + c) – 2b (a – b + c)]
= [- 4ac + 4bc + 4c²]
– [3a² + 3ab + 3ac – 2ab + 2b² – 2bc]
= – 4ac + 4be + 4c² – 3a² – 3ab – 3ac + 2ab – 2b² + 2bc
= – 3a² – 2b² + 4c² – 3ab + 2ab + 4bc + 2bc – 4ac – 3ac
= – 3a² – 2b² + 4c² – ab + 6bc – 7ac

Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 7 Cubes and Cube Roots Ex 7.1 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 8 Maths Chapter 7 Cubes and Cube Roots Ex 7.1

1. Which of the following numbers are not perfect cubes ?

Question (i).
216
Solution:
216
\(\begin{array}{l|l}
2 & 216 \\
\hline 2 & 108 \\
\hline 2 & 54 \\
\hline 3 & 27 \\
\hline 3 & 9 \\
\hline 3 & 3 \\
\hline & 1
\end{array}\)
216 = 2 × 2× 2 × 3 × 3 × 3
Here, the prime factors 2 and 3 appear in a group of three (triples).
∴ 216 is a perfect cube.
216 = 2³ × 3³

Question (ii).
128
Solution:
\(\begin{array}{l|l}
2 & 128 \\
\hline 2 & 64 \\
\hline 2 & 32 \\
\hline 2 & 16 \\
\hline 2 & 8 \\
\hline 2 & 4 \\
\hline 2 & 2 \\
\hline & 1
\end{array}\)
128 = 2 × 2 × 2 × 2 × 2 × 2 × 2
Here, the prime factor 2 does not appear in a group of three.
∴ 128 is not a perfect cube.

Question (iii).
1000
Solution:
\(\begin{array}{l|l}
2 & 1000 \\
\hline 2 & 500 \\
\hline 2 & 250 \\
\hline 5 & 125 \\
\hline 5 & 25 \\
\hline 5 & 5 \\
\hline & 1
\end{array}\)
1000 = 2 × 2 × 2 × 5 × 5 × 5
Here, the prime factors 2 and 5 appear in a group of three.
∴ 1000 is a perfect cube.
1000 = 2³ × 5³

Question (iv).
100
Solution:
\(\begin{array}{l|l}
2 & 100 \\
\hline 2 & 50 \\
\hline 5 & 25 \\
\hline 5 & 5 \\
\hline & 1
\end{array}\)
100 = 2 × 2 × 5 × 5
Here, the prime factors 2 and 5 do not appear in a group of three.
∴ 100 is not a perfect cube.

Question (v).
46656
Solution:
\(\begin{array}{l|l}
2 & 46656 \\
\hline 2 & 23328 \\
\hline 2 & 11664 \\
\hline 2 & 5832 \\
\hline 2 & 2916 \\
\hline 2 & 1458 \\
\hline 3 & 729 \\
\hline 3 & 243 \\
\hline 3 & 81 \\
\hline 3 & 27 \\
\hline 3 & 9 \\
\hline 3 & 3 \\
\hline & 1
\end{array}\)
46656 = 2 × 2 × 2 × 2 × 2 × 2 × 3 × 3 × 3 × 3 × 3 × 3
Here, the prime factors 2 and 3 appear in a group of three.
∴ 46656 is a perfect cube.
46656 = 2³ × 2³ × 3³ × 3³

2. Find the smallest number by which each of the following numbers must be multiplied to obtain a perfect cube:

Question (i).
243
Solution:
\(\begin{array}{l|l}
3 & 243 \\
\hline 3 & 81 \\
\hline 3 & 27 \\
\hline 3 & 9 \\
\hline 3 & 3 \\
\hline & 1
\end{array}\)
243 = 3 × 3 × 3 × 3 × 3
Here, the prime factor 3 does not appear in a group of three.
∴ 243 is not a perfect cube.
(243) × 3 = (3 × 3 × 3 × 3 × 3) × 3
∴ 729 = 3³ × 3³
Thus, 3 is the smallest number by which 243 must be multiplied to get a perfect cube.

Question (ii).
256
Solution:
\(\begin{array}{l|l}
2 & 256 \\
\hline 2 & 128 \\
\hline 2 & 64 \\
\hline 2 & 32 \\
\hline 2 & 16 \\
\hline 2 & 8 \\
\hline 2 & 4 \\
\hline 2 & 2 \\
\hline & 1
\end{array}\)
256 = 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2
Here, the prime factor 2 does not appear in a group of three.
∴ 256 is not a perfect cube.
(256) × 2
= (2 × 2 × 2 × 2 × 2 × 2 × 2 × 2) × 2
∴ 512 = 2³ × 2³ × 2³
Thus, 2 is the smallest number by which 256 must be multiplied to get a perfect cube.

Question (iii).
72
Solution:
\(\begin{array}{l|l}
2 & 72 \\
\hline 2 & 36 \\
\hline 2 & 18 \\
\hline 3 & 9 \\
\hline 3 & 3 \\
\hline & 1
\end{array}\)
72 = 2 × 2 × 2 × 3 × 3
Here, the prime factor 3 does not appear in a group of three.
∴ 72 is not a perfect cube.
(72) × 3
= (2 × 2 × 2 × 3 × 3) × 3
∴ 216 = 2³ × 3³
Thus, 3 is the smallest number by which 72 must be multiplied to get a perfect cube.

Question (iv).
675
Solution:
\(\begin{array}{l|l}
3 & 675 \\
\hline 3 & 225 \\
\hline 3 & 75 \\
\hline 5 & 25 \\
\hline 5 & 5 \\
\hline & 1
\end{array}\)
∴ 675 = 3 × 3 × 3 × 5 × 5
Here, the prime factor 5 does not appear in a group of three.
∴ 675 is not a perfect cube.
(675) × 5
= (3 × 3 × 3 × 5 × 5) × 5
∴ 3375 = 3³ × 5³
Thus, 5 is the smallest number by which 675 must be multiplied to get a perfect cube.

Question (v).
100
Solution:
\(\begin{array}{l|l}
2 & 100 \\
\hline 2 & 50 \\
\hline 5 & 25 \\
\hline 5 & 5 \\
\hline & 1
\end{array}\)
100 = 2 × 2 × 5 × 5
Here, the prime factors 2 and 5 do not appear in a group of three.
∴ 100 is not a perfect cube.
(100) × 2 × 5 = (2 × 2 × 5 × 5) × 2 × 5
∴ 1000 = 2³ × 5³
Thus, 10 is the smallest number by which 100 must be multiplied to get a perfect cube.

3. Find the smallest number by which each of the following numbers must be divided to obtain a perfect cube:

Question (i).
81
Solution:
\(\begin{array}{l|l}
3 & 81 \\
\hline 3 & 27 \\
\hline 3 & 9 \\
\hline 3 & 3 \\
\hline & 1
\end{array}\)
81 = 3 × 3 × 3 × 3
Here, the prime factor 3 is left over after making triples of 3.
∴ 81 is not a perfect cube.
(81) ÷ 3 = (3 × 3 × 3 × 3) ÷ 3
∴ 27 = 3 × 3 × 3 = 33
Thus, 3 is the smallest number by which 81 must be divided to get a perfect cube.

Question (ii).
128
Solution:
\(\begin{array}{l|l}
2 & 128 \\
\hline 2 & 64 \\
\hline 2 & 32 \\
\hline 2 & 16 \\
\hline 2 & 8 \\
\hline 2 & 4 \\
\hline 2 & 2 \\
\hline & 1
\end{array}\)
128 = 2 × 2 × 2 × 2 × 2 × 2 × 2
Here, the prime factor 2 is left over after making triples of 2.
∴ 128 is not a perfect cube.
(128) ÷ 2
= (2 × 2 × 2 × 2 × 2 × 2 × 2) ÷ 2
∴ 64 = 2 × 2 × 2 × 2 × 2 × 2
= 2³ × 2³
Thus, 2 is the smallest number by which 128 must be divided to get a perfect cube.

Question (iii).
135
Solution:
\(\begin{array}{l|l}
3 & 135 \\
\hline 3 & 45 \\
\hline 3 & 15 \\
\hline 5 & 5 \\
\hline & 1
\end{array}\)
135 = 3 × 3 × 3 × 5
Here, the prime factor 5 is left over after making triples of 3.
∴ 135 is not a perfect cube.
(135) ÷ 5 = (3 × 3 × 3 × 5) ÷ 5
∴ 27 = 3 × 3 × 3
= 3³
Thus, 5 is the smallest number by which 135 must be divided to get a perfect cube.

Question (iv).
192
Solution:
\(\begin{array}{l|l}
2 & 192 \\
\hline 2 & 96 \\
\hline 2 & 48 \\
\hline 2 & 24 \\
\hline 2 & 12 \\
\hline 2 & 6 \\
\hline 3 & 3 \\
\hline & 1
\end{array}\)
192 = 2 × 2 × 2 × 2 × 2 × 2 × 3
Here, the prime factor 3 is left over after making triples of 2.
∴ 192 is not a perfect cube.
(192) ÷ 3
= (2 × 2 × 2 × 2 × 2 × 2 × 3) ÷ 3
∴ 64 = 2 × 2 × 2 × 2 × 2 × 2
= 2³ × 2³
Thus, 3 is the smallest number by which 192 must be divided to get a perfect cube.

Question (v).
704
Solution:
\(\begin{array}{l|l}
2 & 704 \\
\hline 2 & 352 \\
\hline 2 & 176 \\
\hline 2 & 88 \\
\hline 2 & 44 \\
\hline 2 & 22 \\
\hline 11 & 11 \\
\hline & 1
\end{array}\)
704 = 2 × 2 × 2 × 2 × 2 × 2 × 11
Here, the prime factor 11 is left over after making triples of 2.
∴ 704 is not a perfect cube.
(704) ÷ 11
= (2 × 2 × 2 × 2 × 2 × 2 × 11) ÷ 11
∴ 64 = 2 × 2 × 2 × 2 × 2 × 2
= 2³ × 2³
Thus, 11 is the smallest number by which 704 must be divided to get a perfect cube.

4. Parikshit makes a cuboid of plasticine of sides 5 cm, 2 cm, 5 cm. How many such cuboids will he need to form a cube ?
Solution:
Sides of the cuboid are 5 cm, 2 cm and 5 cm (Given).
∴ Volume of the cuboid = l × b × h
= 5 cm × 2 cm × 5 cm
To form it as a cube, its dimensions should be in the group of triplets.
Here, the prime factors 2 and 5 are not in group of three.
∴ Volume of the required cube
= (5 cm × 5 cm × 2 cm) × 5 cm × 2 cm × 2 cm
= (5³ × 2³) cm³
Thus, Parikshit needed 5 × 2 × 2 = 20 cuboids to form a cube.

Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 9 Algebraic Expressions and Identities Ex 9.2 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 8 Maths Chapter 9 Algebraic Expressions and Identities Ex 9.2

1. Find the product of the following pairs of monomials:

Question (i)
4, 7p
Solution:
= 4 × 7p
= 4 × 7 × p
= 28p

Question (ii)
– 4p, 7p
Solution:
= – 4p × 7p
= (-4 × 7) × p × p
= – 28p²

Question (iii)
– 4p, 7pq
Solution:
= (- 4p) × 7pq
= – 4 × 7 × p × pq
= – 28p²q

Question (iv)
4p³, – 3p
Solution:
= 4p³ × (- 3p)
= 4 × (- 3) × p³ × p
= – 12p⁴

Question (v)
4p, 0
Solution:
= 4p × 0
= 0

2. Find the areas of rectangles with the following pairs of monomials as their s lengths and breadths respectively:
(p, q); (10m, 5n); (20x², 5y²); (4x, 3x²); (3mn, 4np)
Solution:
Area of a rectangle = length × breadth
(i) length = p, breadth = q
∴ Area = length × breadth
= p × q = pq unit²

(ii) length = 10m, breadth = 5n
∴ Area = length × breadth
= 10m × 5n
= 50mn unit²

(iii) length = 20x², breadth = 5y²
∴ Area = length × breadth
= 20x² × 5y²
= 100x²y² unit²

(iv) length = 4x, breadth 3x²
∴ Area = length × breadth
= 4x × 3x²
= 12x³ unit²

(v) length = 3mn, breadth = 4np
∴ Area = length × breadth
= 3mn × 4np
= 12 mn²p unit²

3. Complete the table of products:
Solution:

First monomial → Second monomial ↓	2x	-5 y	3x2	-4xy	7x2y	– 9x2y2
2x	4x2	-10xy	6x3	-8x2y	14x3y	– 18x3y2
– 5 y	– 10xy	25y2	– 15x2y	20xy2	– 35x2y2	45x2y3
3x2	6x3	– 15x2y	9x4	– 12x3y	21x4y	– 27x4y2
– 4xy	– 8x2y	20xy2	-12x3y	16x2y2	– 28x3y2	36x3y3
7x2y	14x3y	– 35x2y2	21x4y	– 28x3y2	49x4y2	– 63x4y3
– 9x2y2	– 18x3y2	45x2y3	– 27x4y2	36x3y3	– 63x4y3	81x4y4

4. Obtain the volume of rectangular boxes with the following length, breadth and height respectively:

Question (i)
5a, 3a², 7a⁴
Solution:
Volume of rectangular box = length × breadth × height
length = 5a, breadth = 3a², height = 7a⁴
∴ Volume = length × breadth × height
= 5a × 3a² × 7a⁴
= (5 × 3 × 7) × a × a² × a⁴
= 105a⁷ cubic unit

Question (ii)
2p, 4q, 8r
Solution:
length = 2p, breadth = 4q, height = 8r
∴ Volume = length × breadth × height
= 2p × 4q × 8r
= (2 × 4 × 8) × p × q × r
= 64pqr cubic unit

Question (iii)
xy, 2x²y, 2xy²
Solution:
length = xy, breadth = 2x²y, height = 2 xy²
∴ Volume = length × breadth × height
= xy × 2x²y × 2xy²
= (1 × 2 × 2) × xy × x²y × xy²
= 4x⁴y⁴ cubic unit

Question (iv)
a, 2b, 3c
Solution:
length = a, breadth = 2b, height = 3c
∴ Volume = length × breadth × height
= a × 2b × 3c
= (1 × 2 × 3) × a × b × c
= 6abc cubic unit

5. Obtain the product of:

Question (i)
xy, yz, zx
Solution:
= xy × yz × zx
= [x × y) × (y × z) × (z × x)
= x × x × y × y × z × z
= x²y²z²

Question (ii)
a, -a², a³
Solution:
= (a) × (- a²) × (a³)
= – a × a² × a³
= – a⁶

Question (iii)
2, 4y, 8y², 16y³
Solution:
= 2 × 4y × 8y² × 16y³
= 2 × 4 × 8 × 16 × y × y² × y³
= 1024y⁶

Question (iv)
a, 2b, 3c, 6abc
Solution:
= a × 2b × 3c × 6abc
= 1 × 2 × 3 × 6 × a × b × c × abc
= 36 a²b²c²

Question (v)
m, – mn, mnp
Solution:
= (m) × (- mn) × (mnp)
= – (m × mn × mnp)
= – m³n²p

Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 6 Squares and Square Roots InText Questions and Answers.

PSEB 8th Class Maths Solutions Chapter 6 Squares and Square Roots InText Questions

Try These (Textbook Page No. 90)

1. Find the perfect square numbers between (i) 30 and 40 (ii) 50 and 60
Solution:
(i) Since,
1 × 1 = 1,
2 × 2 = 4,
3 × 3 = 9,
4 × 4 = 16,
5 × 5 = 25,
6 × 6 = 36,
7 × 7 = 49.
Thus, 36 is the only perfect square number between 30 and 40.

(ii) Since, 7 × 7 = 49 and 8 × 8 = 64.
∴ There is no perfect square number between 49 and 64.
Thus, there is no perfect square number between 50 and 60.

Try These (Textbook Page No. 90 – 91)

1. Can we say whether the following numbers are perfect squares? How do we know ?
(i) 1057
(ii) 23453
(iii) 7928
(iv) 222222
(v) 1069
(vi) 2061
Write five numbers which you can decide by looking at their units digit that they are not square numbers.
Solution:
(i) 1057
The ending digit is 7. All square numbers end with 0, 1, 4, 5, 6 or 9 at unit’s place.
∴ 1057 cannot be a perfect square.

(ii) 23453
The ending digit is 3. All square numbers end with 0, 1, 4, 5, 6 or 9 at unit’s place.
∴ 23453 cannot be a perfect square.

(iii) 7928
The ending digit is 8. All square numbers end with 0, 1, 4, 5, 6 or 9 at unit’s place.
∴ 7928 cannot be a perfect square.

(iv) 222222
The ending digit is 2. All square numbers end with 0, 1, 4, 5, 6 or s 9 at unit’s place.
∴ 222222 cannot be a perfect square.

(v) 1069
The ending digit is 9. All square jj numbers end with 0, 1, 4, 5, 6 or 9 at unit’s place.
∴ 1069 may or may not be a square number.
30 × 30 = 900, 31 × 31 = 961,
32 × 32 = 1024 and 33 × 33 = 1089.
e.g. No natural number between 1024 and 1089 is a perfect square.
∴ 1069 cannot be a perfect square.

(vi) 2061
The ending digit is 1. All square s numbers end with 0, 1, 4, 5, 6 or 9 at unit’s place.
∴ 2061 may or may not be a square number.
45 × 45 = 2025, 46 × 46 = 2116,
e.g. No natural number between 2025 and 2116 is a square number.
∴ 2061 is not a square number.

2. Write five numbers which you cannot decide just by looking at their unit’s digit (or units place) whether they are square numbers or not.
Solution:
Any natural number ending with 0, 1, 4, 5, 6 or 9 can be or cannot be a square number.
Five such numbers are :
719, 2431, 524, 215, 326
[Note: You can write many more numbers.]

Try These (Textbook Page No. 91)

1. Which of 1232, 772, 822, 1612, 1092 would end with digit 1 ?
Solution:
The square of those numbers end in 1 which end in either 1 or 9. Here, the square of 161 and 109 would end in 1.
OR
The number having 1 or 9 in units place has the digit 1 in units place of its square.

Try These (Textbook Page No. 91)

Which of the following numbers would have digit 6 at unit place ?
(i) 19²
(ii) 24²
(iii) 26²
(iv) 36²
(v) 34²
Solution:
The number having 4 or 6 in the units place has the digit 6 in units place of its square. Except 19² all other numbers have 6 in their units place.
OR
(i) 19²
Units place digit is 9.
∴ 19² would not have units digit as 6. (9 × 9 = 81)

(ii) 24²
Units place digit is 4.
∴ 24² would have units digit as 6. (4 × 4= 16)

(iii) 26²
Units place digit is 6.
∴ 26² would have 6 at units place. (6 × 6 = 36)

(iv) 36²
Units place digit is 6.
∴ 36² would have 6 at units place. (6 × 6 = 36)

(v) 34²
Units place digit is 4.
∴ 34² would have 6 at units place. (4 × 4 = 1²)

Try These (Textbook Page No. 92)

What will be the “one’s digit” in the square of the following numbers ?

Question (i).
1234
Solution:
1234
The ending digit is 4.
4 × 4 = 16
∴ (1234)² will have 6 as the one’s place.

Question (ii).
26387
Solution:
26387
The ending digit is 7.
7 × 7 = 49
∴ (26387)² will have 9 as the one’s place.

Question (iii).
52698
Solution:
52698
The ending digit is 8.
8 × 8 = 64
∴ (52698)² will have 4 as the one’s place.

Question (iv).
99880
Solution:
99880
The ending digit is 0.
0 × 0 = 0
∴ (99880)² will have 0 as the one’s place.

Question (v).
21222
Solution:
21222
The ending digit is 2.
2 × 2 = 4
∴ (21222)² will have 4 as the one’s place.

Question (vi).
9106
Solution:
9106
The ending digit is 6.
6 × 6 = 36
∴ (9106)² will have 6 as the one’s place.

Try These (Textbook Page No. 92)

1. The square of which of the following numbers would be an odd number/an even number? Why?

Question (i).
727
Solution:
727
Here, the ending digit is 7.
It is an odd number.
∴ Its square is also an odd number.

Question (ii).
158
Solution:
158
Here, the ending digit is 8.
It is an even number.
∴ Its square is also an even number.

Question (iii).
269
Solution:
269
Here, the ending digit is 9.
It is an odd number.
∴ Its square is also an odd number.

Question (iv).
1980
Solution:
1980
Here, the ending digit is 0.
It is an even number.
∴ Its square is also an even number.

2. What will be the number of zeros in the square of the following numbers?

Question (i).
60
Solution:
60
In 60, number of zero is 1.
∴ (60)² will have 2 zeros. (∵ 60² = 3600)

Question (ii).
400
Solution:
400
In 400, number of zeros are 2.
∴ (400)² will have 4 zeros.
(∵ 400² = 160000)

Try These (Textbook Page No. 94)

1. How many natural numbers lie between 9² and 10²? Between 11² and 12²?
Solution:
(a) We can find 2n natural numbers between two consecutive natural numbers, n² and (n + 1)².
Here, n = 9, n + 1 = 9 + 1 = 10.
∴ Natural numbers between 9² and 10² are 2n = 2 × 9 = 18.
Thus, 18 natural numbers lie between 9² and 10².

(b) We can find 2n natural numbers between two consecutive natural numbers, n² and (n + 1)².
Here, n = 11, n + 1 = 11 + 1 = 12.
∴ Natural numbers between 11² and 12² are 2n = 2 × 11 = 22.
Thus, 22 natural numbers lie between 112 and 122.

2. How many non square numbers lie between the following pairs of numbers:

Question (i).
100² and 101²
Solution:
100² and 101²
Here, n = 100 and n + 1 = 100 + 1
= 101
∴ non square numbers between 100² and 101² = 2 × n
= 2 × 100
= 200
Thus, 200 non square numbers lie between 100² and 101².

Question (ii).
90² and 91²
Solution:
90² and 91²
Here, n = 90 and n + 1 = 90 + 1 = 91.
∴ Natural numbers between 90² and 91²
= 2 × n
= 2 × 90
= 180.
Thus, 180 non square numbers lie between 90² and 91².

Question (iii).
1000² and 1001²
Solution:
1000² and 1001²
Here, n = 1000 and
n + 1 = 1000 + 1 = 1001.
∴ Natural numbers between 1000² and 1001²
= 2 × n
= 2 × 1000
= 2000.
Thus, 2000 non square numbers lie between 1000² and 1001².

Try These (Textbook Page No. 94)

Find whether each of the following numbers is a perfect square or not:

Question (i).
121
Solution:
121
121 – 1 = 120, 120 – 3 = 117
117-5 = 112, 112 – 7 = 105
105-9 = 96, 96 – 11 = 85
85 – 13 = 72, 72 – 15 = 57
57- 17 = 40, 40 – 19 = 21
21 – 21=0
e.g. 121 = 1 + 3 + 5 + 7 + 9 + 11 + 13 + 15 + 17 + 19 + 21
Thus, 121 is a perfect square.

Question (ii).
55
Solution:
55
55 – 1 = 54, 54 – 3 = 51
51 – 5 = 46, 46 – 7 = 39
39 – 9 = 30, 30 – 11 = 19
19 – 13 = 6, 6 – 15 = – 9
Thus, 55 cannot be expressed as the sum of successive odd numbers starting from 1.
∴ 55 is not a perfect square.

Question (iii).
81
Solution:
81
81 – 1 = 80, 80 – 3 = 77
77 – 5 = 72, 72 – 7 = 65
65 – 9 = 56, 56 – 11 = 45
32 – 15 = 17, 32 – 15 = 17
17 – 17 = 0
∴ 81 = 1 + 3 + 5 + 7 + 9 + 11 + 13 + 15 + 17
Thus, 81 is a perfect square.

Question (iv).
49
Solution:
49
49 – 1 = 48, 48 – 3 = 45
45 – 5 = 40, 40 – 7 = 33
33 – 9 = 24, 24 – 11 = 13
13 – 13 = 0
∴ 49 = 1 + 3 + 5 + 7 + 9 + 11 + 13
Thus, 49 is a perfect square.

Question (v).
69
Solution:
69
69 – 1 = 68, 68 – 3 = 65
65 – 5 = 60, 60 – 7 = 53
53-9 = 44, 44 – 11 = 33
33- 13 = 20, 20 – 15 = 5
5 – 17 = – 12
Thus, 69 cannot be expressed as the sum of successive odd numbers starting from 1.
∴ 69 is not a perfect square.

Try These (Textbook Page No. 95)

1. Express the following as the sum of two consecutive integers:

Question (i).
21²
Solution:
Remember : n² = \(\frac{n^{2}-1}{2}+\frac{n^{2}+1}{2}\)
21²
n = 21

∴ 21² = 220 + 221 = 441

Question (ii).
13²
Solution:
13²
n = 13

∴ 13² = 84 + 85 = 169

Question (iii).
11²
Solution:
11²
n = 11

∴ 11² = 60 + 61 = 121

Question (iv).
19²
Solution:
19²
n = 19

∴ 19² = 180 + 181 = 361

2. Do you think the reverse is also true, e.g. is the sum of any two consecutive positive integers is perfect square of a number? Give example to support your answer.
Solution:
No, the reverse is not always true.
(i) 3 + 4 = 7, 7 is not a perfect square.
(ii) 10 + 11 = 21, 21 is not a perfect square.
But,
(i) 4 + 5 = 9, 9 is a perfect square.
(ii) 12 + 13 = 25, 25 is a perfect square.

Try These (Textbook Page No. 95)

Write the square, making use of the above pattern:

Question (i).
111111²
Solution:
(111111)²
The given number is a six-digit number,
∴ middle number 6

Question (ii).
1111111²
Solution:
(1111111)²
The given number is a seven-digit number.
∴ middle number 7

Try These (Textbook Page No. 95)

Can you find the square of the following numbers using the above pattern ?
(i) 6666667²
(ii) 66666667²
Solution:
(i) Yes, 6666667² = 44444448888889
(ii) Yes, 66666667² = 4444444488888889

Try These (Textbook Page No. 97)

Find the squares of the following numbers containing 5 in unit’s place:

Question (i).
15
Solution:
[Note : A number with unit digit 5, e.g. a5
(a5)² = a(a + 1) × 100 + 25]
( i ) (15)² = 1 × (1 + 1) × 100 + 25
= 1 × 2 × 100 + 25
= 200 + 25
= 225
Hint:

Question (ii).
95
Solution:
(95)² = 9 (9 + 1) × 100 + 25
= 9 × 10 × 100 + 25
= 9000 + 25
= 9025

Question (iii).
105
Solution:
(105)² = 10 × (10 + 1) × 100 + 25
= 10 × 11 × 100 + 25
= 11000 + 25
= 11025
Hint:

Question (iv).
205
Solution:
(205)² = 20 × (20 + 1) × 100 + 25
= 20 × 21 × 100 + 25
= 42000 + 25
= 42025

Try These (Textbook Page No. 99)

Question (i).
11² = 121. What is the square root of 121 ?
Solution:
Square root of 121 is 11.

Question (ii).
14² = 196. What is the square root of 196 ?
Solution:
Square root of 196 is 14.

Think, Discuss and Write (Textbook Page No. 99)

Question (i).
(-1)² = 1. Is – 1, a square root of 1 ?
Solution:
[Note: There are two (positive as well as negative) integral square roots of a perfect square number.]
(- 1) × (- 1) = 1, (- 1)² = 1
∴ Square root of 1 can also be (-1).

Question (ii).
(-2)² = 4. Is -2, a square root of 4 ?
Solution:
(- 2) × (- 2) = 4, (-2)² = 4
∴ Square root of 4 can also be (-2).

Question (iii).
(-9)² = 81. Is – 9, a square root of 81 ?
Solution:
(-9) × (-9) = 81, (- 9)² = 81
∴ Square root of 81 can also be (-9).

Try These (Textbook Page No. 100)

By repeated subtraction of odd numbers starting from 1, find whether the following numbers are perfect squares or not? If the number is a perfect square then find its square root:

Question (i).
121
Solution:
Subtracting the successive odd numbers from 121 :
121 – 1 = 120, 120 – 3 = 117
117 – 5 = 112, 112 – 7 = 105
105 – 9 = 96, 96 – 11 = 85
85 – 13 = 72, 72 – 15 = 57
57 – 17 = 40, 40 – 19 = 21
21 – 21 = 0
∴ \(\sqrt{121}\) = 11
∴ 121 is a perfect square.

Question (ii).
55
Solution:
∵ 55 – 1 = 54, 54 – 3 = 51
51 – 5 = 46, 46 – 7 = 39
39 – 9 = 30, 30 – 11 = 19
19 – 13 = 6, 6 – 15 = – 9
55 does not reduced to 0 after subtracting odd numbers starting from 1.
∴ 55 is not a perfect square.

Question (iii).
36
Solution:
∵ 36 – 1 = 35, 35 – 3 = 32
32 – 5 = 27, 27 – 7 = 20
20 – 9 = 11, 11 – 11 = 0
∴ \(\sqrt{36}\) = 6
∴ 36 is a perfect square.

Question (iv).
49
Solution:
49 – 1 = 48, 48 – 3 = 45
45 – 5 = 40, 40 – 7 = 33
33 – 9 = 24, 24 – 11 = 13
13- 13 = 0
∴ \(\sqrt{49}\) = 7
∴ 49 is a perfect square.

Question (v).
90
Solution:
90 – 1 = 89, 89 – 3 = 86
86 – 5 = 81, 81 – 7 = 74
74 – 9 = 65, 65 – 11 = 54
54 – 13 = 41, 41 – 15 = 26
26 – 17 = 9, 9 – 19 = – 10
90 does not reduced to 0 after subtracting odd numbers starting from 1.
∴ 90 is not a perfect square.

Think, Discuss and Write (Textbook Page No. 103)

Can we say that if a perfect square is of n-digits, then its square root will have \(\frac{n}{2}\) digits if n is even or \(\frac{(n+1)}{2}\) if n is odd ?
Solution:
Yes, we can say that if a perfect square is of n-digits, then its square root will have
(a) \(\frac{n}{2}\) digits, if n is even.
(b) \(\frac{(n+1)}{2}\) digits, if n is odd.

Try These (Textbook Page No. 105)

Without calculating square roots, find the number of digits in the square root of the following numbers:

Question (i).
25600
Solution:
Here, the number of digits, n = 5 (an odd number.)
∴ Number of digits in the square root of 25600 = \(\frac{(n+1)}{2}\)
= \(\frac{5+1}{2}\)
= \(\frac {6}{2}\)
= 3

Question (ii).
100000000
Solution:
Here, the number of digits, n =9 (an odd number.)
∴ Number of digits in the square root of 100000000 = \(\frac{(n+1)}{2}\)
= \(\frac{9+1}{2}\)
= \(\frac {10}{2}\)
= 5

Question (iii).
36864
Solution:
Here, the number of digits, n = 5 (an odd number.)
∴ Number of digits in the square root of 36864 = \(\frac{(n+1)}{2}\)
= \(\frac{5+1}{2}\)
= \(\frac {6}{2}\)
= 3

Try these (Textbook Page No. 107)

Estimate the value of the following to the nearest whole number:

Question (i).
\(\sqrt{80}\)
Solution:
\(\sqrt{80}\)
10² = 100, 9² = 81, 8² = 64
∴ 80 is between 64 and 81.
∴ 64 < 80 < 81
∴ 8² < 80 < 9²
∴ 8 < \(\sqrt{80}\) < 9
Thus, \(\sqrt{80}\) lies between 8 and 9.
\(\sqrt{80}\) is much closer to 81 than 64.
∴ \(\sqrt{80}\) is approximately 9.

Question (ii).
\(\sqrt{1000}\)
Solution:
\(\sqrt{1000}\)
30² = 900, 31² = 961, 32² = 1024
∴ 1000 is between 961 and 1024.
∴ 961 < 1000 < 1024
∴ 31² < 1000 < 32²
∴ 31 < \(\sqrt{1000}\) < 32
Thus, \(\sqrt{1000}\) lies between 31 and 32.
\(\sqrt{1000}\) is much closer to 32 than 31.
∴ \(\sqrt{1000}\) is approximately 32.

Question (iii).
\(\sqrt{350}\)
Solution:
\(\sqrt{350}\)
18² = 324 and 19² = 361
∴ 350 is between 324 and 361.
∴ 324 < 350 < 361
∴ 18² < 350 < 19²
∴ 18 < \(\sqrt{350}\) < 19
Thus, \(\sqrt{350}\) lies between 18 and 19.
\(\sqrt{350}\) is much closer to 19 than 18.
∴ \(\sqrt{350}\) is approximately 19.

Question (iv).
\(\sqrt{500}\)
Solution:
\(\sqrt{500}\)
22² = 484 and 23² = 529
∴ 500 is between 484 and 529.
∴ 484 < 500 < 529
∴ 22² < 500 < 23²
∴ 22 < \(\sqrt{500}\) < 23
Thus, \(\sqrt{500}\) lies between 22 and 23.
\(\sqrt{500}\) is much closer to 22 than 23.
∴ \(\sqrt{500}\) is approximately 22.

Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 9 Algebraic Expressions and Identities Ex 9.1 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 8 Maths Chapter 9 Algebraic Expressions and Identities Ex 9.1

1. Identify the terms, their coefficients for each of the following expressions:

(i) 5xyz² – 3zy
(ii) 1 + x + x²
(iii) 4x²y² – 4x²y²z² + z²
(iv) 3 – pq + qr – rp
(v) \(\frac{x}{2}+\frac{y}{2}\) – xy
(vi) 0.3a – 0.6ab + 0.5b
Solution:

Terms	Coefficient of terms
(i) 5 xyz2 -3zy	5 – 3
(ii) 1 x x2 ‘	1 1 1
(iii) 4x2y2 – 4x2y2z2 z2	4 -4 1
(iv) 3 – pq qr – rp	3 – 1 1 – 1
(v) \(\frac {x}{2}\) \(\frac {y}{2}\) – xy	\(\frac {1}{2}\) \(\frac {1}{2}\) – 1
(vi) 0.3 a – 0.6ab 0.5b	0.3 – 0.6 0.5

2. Classify the following polynomials as monomials, binomials, trinomials. which polynomials do not fit in any of these three categories?
x + y, 1000, x + x² + x³ + x⁴, 7 + y + 5x, 2y – 3y², 2y – 3y² + 4y³, 5x – 4y + 3xy, 4z – 15z², ab + bc + cd + da, pqr, p²q + pq², 2p + 2q.
Solution:

Monomials	Binomials	Trinomials
1000 pqr	x + y 2y – 3y2 4z – 15y2 p2q +pq2 2p + 2q	7 + y + 5x 2y – 3y2 + 4y3 5x – 4y + 3xy

Following polynomials do not fit in any catagories:
x + x² + x³ + x⁴ [∵ Polynomial has 4 terms] ab + bc + cd + da [∵ Polynomial has 4 terms]

3. Add the following:

Question (i)
ab – bc, bc – ca, ca – ab
Solution:
To add, let us arrange like terms one below the other.

Question (ii)
a – b + ab, b – c + bc, c – a + ac
Solution:

Question (iii)
2p²q² – 3pq + 4, 5 + 7pq – 3p²q²
Solution:

Question (iv)
l² + m², m² + n², n² + l², 2lm + 2mn + 2nl
Solution:

4.

Question (a)
Subtract 4a – 7ab + 3b + 12 from 12a – 9ab + 5b – 3
Solution:

Question (b)
Subtract 3xy + 5yz – 7zx from 5xy – 2yz – 2zx + 10xyz
Solution:

Question (c)
Subtract 4p²q – 3pq + 5pq² – 8p + 7q – 10 from 18 – 3p – 11q + 5pq – 2pq² + 5p²q
Solution:

Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 10 Visualising Solid Shapes InText Questions and Answers.

PSEB 8th Class Maths Solutions Chapter 10 Visualising Solid Shapes InText Questions

Do This : [Textbook Page No. 153 – 154]

1. Match the following: (First one is done for you.)

Solution:
(A) → (ii) → (c),
(B) → (iii) → (g),
(C) → (i) → (b),
(D) → (iv) → ( h ),
(E) → (v) → (f),
(F) → (vii) → (d),
(G) → (vi ) → (e),
(H) → (viii) → (a).

Do This : [Textbook Page No. 154 – 155]

1. Match the following pictures (objects) with their shapes:

Solution:
(i) → (c),
(ii) → (d),
(iii) → (e),
(iv) → (b),
(v) → (a).

Do This : [Textbook Page No. 162-163]

1. Look at the following map of a city:

(a) Colour the map as follows: Blue – Water, Red – Fire Station, Orange – Library, Yellow – Schools, Green -Park, Pink – Community Centre, Purple – Hospital, Brown – Cemetery.
(b) Mark a Green ‘X’ at the intersection of 2nd street and Danim street. A Black ‘Y’ where the river meets the third street. A red ‘Z’ at the intersection of main street and 1st street.
(c) In magenta colour, draw a short street route from the college to the lake.

2. Draw a map of the route from your house to your school showing important landmarks.
[Note: Friends, do activity 1 and 2 by yourself. Enjoy drawing and colouring.]

Do This : [Textbook Page No. 165-166]

1. Tabulate the number of faces, edges and vertices for the following polyhedrons: (Here ‘V’ stands for number of vertices, ‘F’ stands for number of faces and ‘E’ stands for number of edges.)

Solid	F	V	E	F + V	E + 2
Cuboid
Triangular pyramid
Triangular prism
Pyramid with square base
Prism with square base

What do you infer from the last two columns ? In each case, do you find F + V = E + 2, i.e., F + V – E = 2? This relationship is called Euler’s formula. Infact this formula is true for any polyhedron.
Solution:

Solid	F	V	E	F + V	E + 2
Cuboid	6	8	12	6 + 8 =14	12 + 2 = 14
Triangular pyramid	4	4	6	4 + 4 = 8	6 + 2 = 8
Triangular prism	5	6	9	5 + 6 =11	9 + 2 =11
Pyramid with square base	5	5	8	5 + 5 = 10	8 + 2 = 10
Prism with square base	6	8	12	6 + 8 = 14	12 + 2 = 14

Yes, in each case Euler’s formula F + V = E + 2 or F + V – E = 2 is true.

Think, Discuss and Write : [Textbook Page No. 166]

1. What happens to F, V and E if some parts are sliced off from a solid ? (To start with, you may take a plasticine cube, cut a corner off and investigate.)
Solution:

Suppose ABCDEFGH is a cube. It has 6 faces, 8 vertices and 12 edges.
V = 8, F = 6 and E = 12
∴ V + F – E
= 8 + 6 – 12 = 2
So the Euler’s formula is verified.

Now, suppose we sliced off ∆ PQR from this cube, then F = 6 + 1 = 7
V = 8 – 1 + 3
= 7 + 3 = 10
E = 12 + 3 = 15
∴ V + F – E = 10 + 7 – 15 = 2
∴ Here also Euler’s formula is verified. Thus, if some parts are sliced off a solid, then the number of vertices, edges and faces will be changed but still the Euler’s formula is verified.

Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 10 Visualising Solid Shapes Ex 10.3 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 8 Maths Chapter 10 Visualising Solid Shapes Ex 10.3

1. Can a polyhedron have for its faces:

Question (i)
3 triangles?
Solution:
[Note: Polyhedron is a solid, which is made by polygonal regions and these polygonal regions are called its faces.]
No, a polyhedron cannot have 3 triangles for its faces because it have atleast 4 faces.

Question (ii)
4 triangles?
Solution:
Yes, a polyhedron can have 4 triangles for its faces as triangular pyramid.

Question (iii)
a square and four triangles?
Solution:
Yes, a polyhedron can have a square and four triangles for its faces as a pyramid with square base.

2 Is it possible to have a polyhedron with any given number of faces? (Hint: Think of a pyramid.)
Solution:
Yes, it can be possible only if the number of faces is greater than or equal to 4, because a polyhedron has atleast 4 faces.

3. Which are prisms among the following?

Solution:
Note: A prism is a polyhedron whose base and top are congruent polygons and lateral faces are rectangles.
(i) No, a nail is not a prism because its base and top are not congruent polygons.
(ii) Yes, an unsharpened pencil is a prism because its base and top are congruent polygons and faces are rectangles.
(iii) No, a table weight is not a prism because its top and base are not congruent polygons.
(iv) Yes, box is a prism because its base and top are congruent polygons and lateral faces are rectangles.

4.

Question (i)
How are prisms and cylinders alike?
Solution:
Top and base of a prism and of a cylinder are congruent and parallel to each other and a prism becomes a cylinder, if the number of sides of its base becomes larger and larger.

Question (ii)
How are pyramids and cones alike?
Solution:
The pyramids and cones are alike because their lateral faces meet at a vertex. Also, a pyramid becomes a cone if the number of sides of its base becomes larger and larger.

5. Is a square prism same as a cube? Explain.
Solution:
No, a square prism is not same as a cube. It can be a cuboid also.

6. Verify Euler’s formula for these solids:

Solution:
(i) For figure (i)
F = 7, V= 10 and E = 15
∴ F + V = 7 + 10 = 17
Now, F + V – E = 17 – 15 = 2
Thus, F + V – E = 2
Hence, Euler’s formula is verified.

(ii) For figure (ii)
F = 9, V = 9 and E = 16
∴ F + V = 9 + 9 = 18
Now, F + V – E = 18 – 16 = 2
Thus, F + V – E = 2
Hence, Euler’s formula is verified.

7. Using Euler’s formula find the unknown:

	(i)	(ii)	(iii)
Faces	?	5	20
Vertices	6	?	12
Edges	12	9	?

Solution:
(i) Here, F = ?, V = 6 and E = 12
Now, F + V – E = 2 (∵ Euler’s formula)
∴ F + 6 – 12 = 2
∴ F – 6 = 2
∴ F = 2 + 6
∴ F = 8

(ii) Here, F = 5, V = ? and E = 9
Now, F + V – E = 2 (∵ Euler’s formula)
∴ 5 + V – 9 = 2
∴ V – 4 = 2
∴ V = 2 + 4
∴ V = 6

(iii) Here, F = 20, V = 12 and E = ?
Now, F + V – E = 2 (∵ Euler’s formula)
∴ 20 + 12 – E = 2
∴ 32 – E = 2
∴ – E = 2 – 32
∴ – E = – 30
∴ E = 30

8. Can a polyhedron have 10 faces, 20 edges and 15 vertices?
Solution:
Here, F = 10, E = 20 and V = 15
By Euler’s formula, F + V – E = 2
LHS = F + V – E
= 10 + 15 – 20
= 25 – 20 = 5
Thus, F + V – E ≠ 2
Hence, such a polyhedron is not possible.