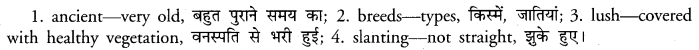Bhagya

Punjab State Board PSEB 5th Class English Book Solutions English Reading Comprehension Passages Exercise Questions and Answers, Notes.

PSEB 5th Class English Reading Comprehension Passages

Read the following passages/stanzas and answer the questions given below each:

(1) Once there was a shoemaker. He became so poor that he had nothing left to run his family but only enough leather for just one pair of shoes. So, in the evening, he cut out the leather with great care to make the shoes. But he was so disappointed that he left his work on the table to finish in the morning. He was almost in tears. Before he fell asleep, he prayed to God and asked to help him.

(A) Question 1.
What was the shoemaker’s problem?
(ਜੁੱਤੀਆਂ ਬਣਾਉਣ ਵਾਲੇ ਦੀ ਕੀ ਸਮੱਸਿਆ ਸੀ ?)
जूते बनाने वाले की क्या समस्या थी ?
Answer:
The shoemaker had become very poor. He had nothing left to run his family.
(ਜੁੱਤੀਆਂ ਬਣਾਉਣ ਵਾਲਾ ਬਹੁਤ ਹੀ ਗਰੀਬ ਹੋ ਗਿਆ ਸੀ । ਆਪਣਾ ਪਰਿਵਾਰ ਚਲਾਉਣ ਦੇ ਲਈ ਉਸ ਦੇ ਕੋਲ ਕੁਝ ਨਹੀਂ ਬਚਿਆ ਸੀ )
जूते बनाने वाला बहुत ही ग़रीब हो गया था। अपना परिवार चलाने के लिए उसके पास कुछ बाच था।

Question 2.
How much leather did he have ?
(ਉਸ ਕੋਲ ਕਿੰਨਾ ਚਮੜਾ ਸੀ?)
उसके पास कितना चमड़ा था ?
Answer:
He had leather only for one pair of shoes.
((ਉਸ ਦੇ ਕੋਲ ਸਿਰਫ਼ ਇਕ ਜੋੜੀ ਜੁੱਤੀਆਂ ਦਾ ਜੋੜਾ ਬਣਾਉਣ ਲਈ ਚਮੜਾ ਸੀ ।)
उसके पास केवल एक जोड़ी जूते बनाने का चमड़ा था।

Question 3.
What for did he pray to God ?
(ਉਸਨੇ ਪ੍ਰਮਾਤਮਾ ਅੱਗੇ ਕਿਸੇ ਵਾਸਤੇ ਅਰਦਾਸ ਕੀਤੀ ?)
उसने परमात्मा से किस बात की प्रार्थना की ?
Answer:
He prayed to God for help.
((ਉਸਨੇ ਪਰਮਾਤਮਾ ਅੱਗੇ ਸਹਾਇਤਾ ਲਈ ਅਰਦਾਸ ਕੀਤੀ ।)

(B) Write True / False for the following sentences:

(a) The shoemaker became a very rich.
(b) He asked God to help him.
(c) He cut out the leather carelessly.
(d) He was almost in tears.
Or
Give the opposite of the following words:
(a) poor ………….
(b) morning ………..
(c) nothing ……….
Or
Complete the following sentences:
(a) He left his work on the ………. to finish in the ……….
(b) He was almost in …………… .
Answer:
(a) False
(b) True
(c) False
(d) True
Or
(a) rich
(b) evening
(c) everything
Or
(a) He left his work on the table to finish it in the morning.
(b) He was almost in tears.

(2) In the morning, he found a wonderful pair of shoes on his table. He was surprised. He took the shoes in his hands and looked at them closely. They were so neatly made that there was not even one bad stitch on them. The pair of shoes was, indeed, a masterpiece.

Soon after, a customer came into the shop. He liked the shoes and paid more than the usual price. Now, the shoemaker was very happy as he had enough money to buy leather for two pairs of shoes.

(A) Question 1.
What did the shoemaker find in the morning ?
(ਜੁੱਤੀਆਂ ਬਣਾਉਣ ਵਾਲੇ ਨੂੰ ਸਵੇਰੇ ਕੀ ਲੱਭਿਆ ?)
जूते बनाने वाले ने सुबह क्या देखा ?
Answer:
The shoemaker found a wonderful pair of shoes in the morning.
(ਜੁੱਤੀਆਂ ਨਿਰਮਾਤਾ ਨੂੰ ਜੁੱਤੀਆਂ ਦਾ ਇਕ ਅਦਭੁੱਤ ਜੋੜਾ ਲੱਭਿਆ ॥
जूता निर्माता ने सुबह जूतों का एक अद्भुत जोड़ा देखा।

Question 2.
What made the pair of shoes a masterpiece ?
(ਜੁੱਤੀਆਂ ਦੀ ਜੋੜੀ ਨੂੰ ਇੱਕ ਮਾਸਟਰਪੀਸ ਕਿਸ ਚੀਜ਼ ਨੇ ਬਣਾਇਆ?)
कौन सी बात जूतों के जोड़े को शानदार कृति बनाती थी ?
Answer:
Fine stiching of the shoes made them a masterpiece.
((ਜੁੱਤੀਆਂ ਦੀ ਵਧਿਆ ਸਿਲਾਈ ਉਨ੍ਹਾਂ ਨੂੰ ਸ਼ਾਨਦਾਰ ਕ੍ਰਿਤੀ ਬਣਾਉਂਦੀ ਸੀ ।
जूतों की बढ़िया सिलाई उन्हें शानदार कृति बनाती थी।

Question 3.
What price did the shoemaker get for the shoes ?
(ਜੁੱਤੀ ਬਣਾਉਣ ਵਾਲੇ ਨੂੰ ਜੁੱਤੀ ਦੀ ਕੀ ਕੀਮਤ ਮਿਲੀ?)
जूता निर्माता को जूतों की कितनी कीमत मिली ?
Answer:
He got more than usual price for the shoes.
(ਉਸਨੂੰ ਜੁੱਤੀਆਂ ਦੀ ਸਾਧਾਰਨ ਤੋਂ ਵੀ ਜ਼ਿਆਦਾ ਕੀਮਤ ਮਿਲੀ । )
उसे जूतों की सामान्य से भी अधिक कीमत मिली।

(B) Write True / False for the following sentences:

(a) He found a wonderful pair of shoes.
(b) He was sad.
(c) The customer liked the shoes.
(d) He threw the shoes away.
Or
Give the opposite of the following words:
(a) bad …………
(b) found …………..
(c) after ………………
Or
Complete the following sentences:
(a) He took the shoes in his ………… and ……… at them.
(b) He had enough money to buy leather for ………..
Answer:
(a) True
(b) False
(c) True
(d) False.
Or
(a) He took the shoes in his hands and looked at them.
(b) He had enough money to buy leather for two pairs of shoes.
Or.
(a) good
(b) lost
(c) before.

(3) The woman said, “Those little men have made us rich. We should show our gratitude to them for their selfless service. I will make little shirts, coats, vests, trousers and a pair of socks for them.” “Ok, then I will make two little pairs of shoes for them,” said the shoemaker.

When everything was ready, they laid their presents on the table and hid themselves. At midnight, the elves came in. They were delighted to find the gifts. Both the elves dressed themselves in the new clothes. Then, they danced and sang happily.

(A) Question 1.
Why did the woman want to show gratitude to the little men ?
(ਔਰਤ ਬੌਨਿਆਂ ਦਾ ਅਹਿਸਾਨ ਕਿਉਂ ਜਤਾਉਣਾ ਚਾਹੁੰਦੀ ਸੀ ?)
औरत बौनों का आभार क्यों जताना चाहती थी ?
Answer:
The woman wanted to show gratitude to them for their selfless service.
((ਔਰਤ ਬੌਨਿਆਂ ਦੀ ਨਿਰਸਵਾਰਥ ਸੇਵਾ ਦੇ ਲਈ ਉਨ੍ਹਾਂ ਦਾ ਅਹਿਸਾਨ ਜਤਾਉਣਾ ਚਾਹੁੰਦੀ ਸੀ ॥)
औरत बौनों की नि:स्वार्थ सेवा के लिए उनका आभार जताना चाहती थी।

Question 2.
What did the shoemaker want to do for them ?
(ਜੁੱਤੀਆਂ ਦਾ ਨਿਰਮਾਤਾ ਉਨ੍ਹਾਂ ਦੇ ਲਈ ਕੀ ਕਰਨਾ ਚਾਹੁੰਦਾ ਸੀ ?)
जूता निर्माता उनके लिए क्या करना चाहता था ?
Answer:
The shoemaker wanted to make two little pairs of shoes for them.
(ਜੁੱਤੀਆਂ ਦਾ ਨਿਰਮਾਤਾ ਉਨ੍ਹਾਂ ਦੇ ਲਈ ਦੋ ਜੋੜੀਆਂ ਛੋਟੀਆਂ ਜੁੱਤੀਆਂ ਬਣਾਉਣਾ ਚਾਹੁੰਦਾ ਸੀ )
जूता निर्माता उनके लिए दो जोड़ी छोटे जूते बनाना चाहता था।

Question 3.
What made the little men feel happy ?
((ਬੌਨਿਆਂ ਨੂੰ ਕਿਸ ਗੱਲ ਦੇ ਕਾਰਨ ਖੁਸ਼ੀ ਹੋਈ ?))
बौनों को किस बात के कारण खुशी हुई ?
Answer:
The gifts for them on the table made the little men feel happy.
(ਬੌਨਿਆਂ ਨੂੰ ਆਪਣੇ ਲਈ ਤੋਹਫੇ ਦੇਖ ਕੇ ਖੁਸ਼ੀ ਹੋਈ ॥)
बौनों को मेज़ पर उनके लिए पड़े तोहफों को देख कर खुशी हुई।

(B) Write True / False for the following sentences:

(a) The little men had made them rich.
(b) They laid their presents on the chairs.
(c) The elves were happy to find the gifts.
(d) The elves came early in the morning.
Or
Give the opposites of the following words:
(a) selfless ………….
(b) little ………. .
(c) new …………
Or
Complete the following sentences:
(a) Both the elves dressed themselves in ………… .
(b) They laid their presents on the …….. and hid ………..
Answer:
(a) True
(b) False
(c) True
(d) False.
Or
(a) selfish
(b) big
(c) old.
Or
(a) Both the elves dressed themselves in the new clothes.
(b) They laid their presents on the table and hid themselves.

(4) Pala said, “Dear, look at the buildings. How tall they are ! Our village is now on the way to advancement”. Beero grumbled, “Advancement ! Don’t you realize that our health is at stake ?” “What are you saying ?” asked Pala. “You need to look around you,” said Beero. “People have beautiful homes with all kinds of facilities but they are not using them properly.”

(A) Question 1.
How does Pala feel about their village ?
(ਪਾਲਾ ਆਪਣੇ ਪਿੰਡ ਦੇ ਬਾਰੇ ਵਿਚ ਕਿਸ ਤਰ੍ਹਾਂ ਮਹਿਸੂਸ ਕਰਦਾ ਹੈ ?)
पाला अपने गांव के बारे में कैसा महसूस करता है ?
Answer:
Pala feels that their village is on the way to advancement.
(ਪਾਲਾ ਮਹਿਸੂਸ ਕਰਦਾ ਹੈ ਕਿ ਉਨ੍ਹਾਂ ਦਾ ਪਿੰਡ ਤਰੱਕੀ ਕਰ ਰਿਹਾ ਹੈ ॥)
पाला महसूस करता है कि उनका गांव तरक्की कर रहा है।

Question 2.
Does Beero agree with him ? How does she feel ?
(ਕੀ ਬੀਰੋ ਉਸ ਨਾਲ ਸਹਿਮਤ ਹੈ ? ਉਹ ਕਿਸ ਤਰ੍ਹਾਂ ਮਹਿਸੂਸ ਕਰਦੀ ਹੈ ?)
क्या बीरो उससे सहमत है ? वह कैसा महसूस करती है ?
Answer:
No, Beero does not agree with him. She feels that their health is at stake.
( ਨਹੀਂ ਬੀਰੋ ਉਸਦੇ ਨਾਲ ਸਹਿਮਤ ਨਹੀਂ ਹੈ । ਉਹ ਮਹਿਸੂਸ ਕਰਦੀ ਹੈ ਕਿ ਉਨ੍ਹਾਂ ਦੀ ਸਿਹਤ ਖ਼ਤਰੇ ਵਿਚ ਹੈ ॥)
नहीं, बीरो उससे सहमत नहीं है। वह महसूस करती है कि उनका स्वास्थ्य खतरे में है।

Question 3.
What kind of homes do the people have in the village ?
(ਪਿੰਡ ਵਿਚ ਲੋਕਾਂ ਦੇ ਕੋਲ ਕਿਸ ਤਰ੍ਹਾਂ ਦੇ ਘਰ ਹਨ ?)
गांव में लोगों के पास कैसे घर हैं ?
Answer:
They have beautiful homes with all kinds of facilities.
(ਉਨ੍ਹਾਂ ਦੇ ਕੋਲ ਸੁੰਦਰ ਘਰ ਹਨ, ਜਿਨ੍ਹਾਂ ਵਿਚ ਹਰ ਤਰ੍ਹਾਂ ਦੀਆਂ ਸੁਵਿਧਾਵਾਂ ਹਨ )
उनके पास सुंदर घर हैं जिनमें सभी प्रकार की सुविधाएं हैं।

(B) Write True / False for the following sentences:

(a) The buildings were tall.
(b) People had beautiful homes.
(c) Their health was not/at stake.
(d) Beero grumbled at the word advancement used for the village.
Or
Give the opposites of the following words:
(a) tall …………. .
(b) beautiful ……… .
(c) now ………. .
Or
Complete the following sentences:
(a) People are not using their homes ………………
(b) The buildings in the village are ………..
Answer:
(a) True
(b) True
(c) False
(d) True
Or
(a) tiny / small
(b) ugly
(c) there.
Or
(a) People are not using their homes properly.
(b) The buildings in the village are very tall.

(5) “What do you want to say?” asked Pala. Beero responded, “They are not using their toilets.” “What !” exclaimed Pala. Beero continued, “They defecate in the open, near my home. This place stinks ! I am fed up of this unpleasant odour. They are not even afraid of the danger they are going to face.”

(A) Question 1.
What wrong thing are the people doing ?
(ਲੋਕ ਕੀ ਗ਼ਲਤ ਕੈਮ ਕਰ ਰਹੇ ਹਨ ?)
लोग क्या ग़लत काम कर रहे हैं ?
Answer:
They are not using their toilets and defecate in the open.
(ਉਹ ਆਪਣੇ ਟਾਇਲੈਟ ਦਾ ਪ੍ਰਯੋਗ ਨਾ ਕਰਕੇ ਖੁੱਲ੍ਹੇ ਸ਼ੌਚ ਕਰਦੇ ਹਨ ॥)
वे अपने टॉयलट्स का प्रयोग न करके खुले में शौच करते हैं।

Question 2.
Why does the place stink ?
(ਉਸ ਜਗ੍ਹਾ ਤੇ ਬਦਬੂ ਕਿਉਂ ਆਉਂਦੀ ਹੈ ?)
उस स्थान से दुर्गंध क्यों आती है ?
Answer:
The place stinks because the people defecate near it.
(ਉਸ ਸਥਾਨ ਤੋਂ ਬਦਬੂ ਇਸ ਲਈ ਆਉਂਦੀ ਹੈ, ਕਿਉਂਕਿ ਲੋਕ ਉਸਦੇ ਨੇੜੇ ਸ਼ੌਚ ਕਰਦੇ ਹਨ ॥)
उस स्थान से दुर्गंध इसलिए आती है क्योंकि लोग उसके निकट शौच करते हैं।

Question 3.
What is Beero fed up of ?
(ਬੀਰੋ ਕਿਸ ਗੱਲ ਤੋਂ ਦੁਖੀ ਹੈ ?)
बीरो किस बात से दुःखी है ?
Answer:
Beero is fed up of unpleasant odour.
(ਬੀਰੋ ਬਦਬੂ ਤੋਂ ਦੁਖੀ ਹੈ ॥)
बीरो दुर्गंध से दुःखी है।

(B) Write True / False for the following sentences:

(a) Beero did not respond to Pala’s question
(b) People were using their toilets.
(c) They defecated in the open.
(d) People were afraid of the danger.
Or
Give the opposite of the following words:
(a) near …………. .
(b) unpleasant ………. .
(c) open ………. .
Or
Complete the following sentences:
(a) They are not afraid of the ……… they are going to …………
(b) People defecate in the ………….. near ………….. home.
Answer:
(a) False
(b) False
(c) True
(d) False.
Or
(a) far
(b) pleasant
(c) covered / hidden.
Or
(a) They are not afraid of the danger they are going to face.
(b) People defecate in the open near Beero’s home.

(6) Grandmother and I.
We often sigh,
At the big adult world.
An alien world.

(A) Question 1.
Name the poem.
(ਕਵਿਤਾ ਦਾ ਨਾਂ ਦੱਸੋ )
कविता का नाम बताओ?
Answer:
The name of the poem is ‘Child and Grandma’.
(ਕਵਿਤਾ ਦਾ ਨਾਂ ‘Child and Grandma’ ਹੈ)
कविता का नाम ‘Child and Grandma’ हैं।

Question 2.
What do Grandmother and the child sigh at ?
(ਦਾਦੀ ਮਾਂ ਅਤੇ ਬੱਚਾ ਆਹ ਕਿਉਂ ਭਰਦੇ ਹਨ ?)
दादी माँ और बच्चा आह क्यों भरते हैं ?
Answer:
They sigh at the big adult world.
(ਉਹ ਬਾਲਗਾਂ ਦੇ ਵੱਡੇ ਸੰਸਾਰ ’ਤੇ ਆਹ ਭਰਦੇ ਹਨ । )
वे वयस्कों के बड़े संसार पार आह भरते हैं।

Question 3.
What do they call the big adult world ?
(ਉਹ ਬਾਲਗਾਂ ਦੇ ਵੱਡੇ ਸੰਸਾਰ ਨੂੰ ਕੀ ਕਹਿ ਕੇ ਬੁਲਾਉਂਦੇ ਹਨ ?)
वे वयस्कों के बड़े संसार को क्या कह कर पुकारते हैं ?
Answer:
They call it an alien world.
(ਉਹ ਇਸਨੂੰ alien world ਕਹਿ ਕੇ ਬੁਲਾਉਂਦੇ ਹਨ ॥)
वे इसे alien world कह कर पुकारते हैं।

(B) Complete the following lines:

(a) We often ………….
(b) The child is with its ………
Answer:
(a) We often sigh.
(b) The child is with its grandmother.

(7) I caress her wrinkled skin.
In her I find my kin.
We are so much alike.
We think so alike.

(A) Question 1.
Whose skin is wrinkled ?
(ਝੁਰੜੀਆਂ ਵਾਲੀ ਚਮੜੀ ਕਿਸ ਦੀ ਹੈ ?))
झुरियों वाली त्वचा किसकी है?
Answer:
Grandma’s skin is wrinkled.
(ਦਾਦੀ ਮਾਂ ਦੀ ਚਮੜੀ ਝੁਰੜੀਆਂ ਵਾਲੀ ਹੈ ।)

Question 2.
How are the child and its grandmother alike ?
(ਬੱਚਾ ਅਤੇ ਦਾਦੀ ਮਾਂ ਕਿਸ ਤਰ੍ਹਾਂ ਇਕੋ ਜਿਹੇ ਹਨ ?)
बच्चा और दादी माँ किस तरह एक जैसे हैं ?
Answer:
Both feel oneness with each other. They think alike.
(ਦੋਵੇਂ ਇਕ-ਦੂਸਰੇ ਦੇ ਨਾਲ ਜੁੜਿਆ ਹੋਇਆ ਅਨੁਭਵ ਕਰਦੇ ਹਨ । ਉਹ ਇਕੋ ਜਿਹਾ ਸੋਚਦੇ ਹਨ ॥)
दोनों एक-दूसरे से जुड़ा हुआ अनुभव करते हैं। वे एक जैसा सोचते हैं।

Question 3.
Name the poem.
(ਕਵਿਤਾ ਦਾ ਨਾਂ ਲਿਖੋ !)
कविता का नाम बताओ?
Answer:
The name of the poem is ‘Child and Grandma’.
(ਕਵਿਤਾ ਦਾ ਨਾ ‘Child and Grandma’ ਹੈ ।)

(B) Complete the following sentences:

(a) In her I find ………………….
(b) I caress her ……………
Answer:
(a) In her I find my kin.
(b) I caress her wrinkled skin.

(8) Milkha Singh is one of India’s greatest personalities. He is a famous athlete. He is also known as the Flying Sikh. He was born in a Sikh Rathore Rajput family in Punjab in undivided India (now in Pakistan). The partition of India happened when he was still a teenager. He witnessed the killing of his parents. The last words of his father were “Bhaag Milkha Bhaag”. It means “Run Milkha Run”. This was a last-minute plea to Milkha to run away and save his life.

(A) Question 1.
Who is Milkha Singh ?
(ਮਿਲਖਾ ਸਿੰਘ ਕੌਣ ਹੈ?)
मिल्खा सिंह कैन हैं। ?
Answer:
Milkha Singh is one of India’s greatest personalities. He is a famous althlete.
(ਮਿਲਖਾ ਸਿੰਘ ਭਾਰਤ ਦੀਆਂ ਸਭ ਤੋਂ ਮਹਾਨ ਸ਼ਖ਼ਸੀਅਤਾਂ ਵਿਚੋਂ ਇਕ ਹੈ । ਉਹ ਪ੍ਰਸਿੱਧ ਥਲੀਟ ਹੈ।)
मिल्खा सिंह भारत के सबसे महान् व्यक्तियों में से एक है। वह प्रसिद्ध खिलाड़ी हैं।

Question 2.
What were the last words of his father ?
(ਉਸਦੇ ਪਿਤਾ ਦੇ ਆਖਰੀ ਸ਼ਬਦ ਕੀ ਸਨ?)
उनके पिता के अंतिम शब्द थे ?
Answer:
The last words of his father were “Bhaag Milkha Bhaag”.
((ਉਨ੍ਹਾਂ ਦੇ ਪਿਤਾ ਜੀ ਦੇ ਅੰਤਿਮ ਸ਼ਬਦ ਸਨ, “ਦੌੜ ਮਿਲਖਾ ਦੌੜ’’ ॥ )
उनके पिता के अंतिम शब्द थे “भाग मिल्खा भाग”।

Question 3.
How old was Milkha Singh at the time of the partition of India ?
(ਭਾਰਤ ਦੀ ਵੰਡ ਦੇ ਸਮੇਂ ਮਿਲਖਾ ਸਿੰਘ ਦੀ ਕਿੰਨੀ ਉਮਰ ਸੀ ?)
भारत-विभाजन के समय मिल्खा सिंह की आयु कितनी थी ?
Answer:
At that time Milkha Singh was still a teenager.
((ਉਸ ਸਮੇਂ ਮਿਲਖਾ ਸਿੰਘ ਇਕ ਕਿਸ਼ੋਰ ਸਨ ॥)
उस समय मिल्खा सिंह अभी एक किशोर थे।

(B) Write True / False for the following sentences:

(a) Milkha Singh is a famous leader.
(b) He witnessed the killing of his parents.
(c) His mother asked Milkha to save her.
(d) Milkha Singh was born in a Sikh family.
Or
Give the opposites of the following words:
(a) known …………. .
(b) last …………
(c) save ………..
Or
Complete the following sentences:
(a) He witnessed the killing of ……………
(b) Milkha Singh is also known as ………….
Answer:
(a) False
(b) True
(c) False
(d) True
Or
(a) unknown
(b) first
(c) waste / kill.
Or
(a) He witnessed the killing of his parents.
(b) Milkha Singh is also known as the Flying Sikh.

(9) Milkha’s school was at a distance of 10 km. from his home. He had the habit of running to cover this distance every day. This habit helped him to become a good runner. After partition, he came to India with his elder sister. Later on, he tried to join the army. He got selected in his fourth attempt in 1951 and was introduced to athletics.

(A) Question 1.
How far was Milkha’s school from his home ?
मिल्खा का स्कूल उनके घर से कितनी दूर था ?
Answer:
Milkha’s school was at a distance of 10 km from his home.
मिल्खा का स्कूल उनके घर से 10 कि०मी० की दूरी पर था।

Question 2.
What made him a good runner ?
(ਕਿਸ ਗੱਲ ਨੇ ਉਨ੍ਹਾਂ ਨੂੰ ਇਕ ਚੰਗਾ ਦੌੜਾਕ ਬਣਾਇਆ ?))
किस बात ने उन्हें एक अच्छा धावक बनाया ?
Answer:
His habit of running to school everyday made him a good runner.
(ਮਿਲਖਾ ਦੀ ਹਰ ਰੋਜ਼ ਸਕੂਲ ਤੱਕ 10 ਕਿ. ਮੀ. ਦੀ ਦੂਰੀ ਤੱਕ ਦੌੜਣ ਦੀ ਆਦਤ ਨੇ ਉਸ ਨੂੰ ਇਕ ਚੰਗਾ ਦੌੜਾਕ ਬਣਾ ਦਿੱਤਾ ।)
प्रतिदिन दौड़ कर स्कूल जाने की उनकी आदत ने उन्हें एक अच्छा धावक बनाया।

Question 3.
When did he join army ? How many attempts did he make for it ?
(ਉਹ ਕਦੋਂ ਚੁਣਿਆ ਗਿਆ ?)
वह सेना में कब भर्ती हुए ? इसके लिए उन्होंने कितने प्रयास किए ?
Answer:
He joined army in 1951. He made four attempts for it.
(ਉਹ 1951 ਵਿਚ ਆਪਣੀ ਚੌਥੀ ਕੋਸ਼ਿਸ਼ ਵਿੱਚ ਚੁਣਿਆ ਗਿਆ ।)
वह 1951 में सेना में भर्ती हुए। इसके लिए उन्होंने चार प्रयास किए।

(B) Write True / False for the following sentences:

(a) Milkha’s school was near his home.
(b) After partition, Milkha came to India.
(c) He tried to join the police.
Or
Give the opposites of the following words:
(a) day …………
(b) join ………….
(c) elder ………… .
Answer:
(a) False
(b) True
(c) False
Or
(a) night
(b) leave
(c) younger.

(10) Milkha Singh also won gold medal in Asian Games held in Tokyo, beating the Pakistani runner Abdul Khaliq. He was invited by the President of Pakistan to take part in a race to be held in Pakistan, but he did not want to go because of the bitter memories of the partition. At last, Pandit Jawahar Lal Nehru convinced him to go. Milkha Sing once again beat Abdul Khaliq in the race. The President of Pakistan was very much impressed. He remarked, “Milkha, you did not run, you actually flew.” He gave the title of ‘Flying Sikh’ to him.

(A) Question 1.
Whom did Milkha Singh beat in Asian Games at Tokyo ?
(ਟੋਕੀਓ ਵਿਚ ਹੋਣ ਵਾਲੀਆਂ ਏਸ਼ਿਆਈ ਖੇਡਾਂ ਵਿਚ ਮਿਲਖਾ ਸਿੰਘ ਨੇ ਕਿਸਨੂੰ ਹਰਾਇਆ. ?)
टोक्यो में होने वाली एशियाई खेलों में मिल्खा सिंह ने किसे पराजित किया ?
Answer:
Milkha Singh beat Pakistani runner Abdul Khaliq in Asian Games at Tokyo.
(ਟੋਕੀਓ ਵਿਚ ਹੋਣ ਵਾਲੀਆਂ ਏਸ਼ਿਆਈ ਖੇਡਾਂ ਵਿਚ ਮਿਲਖਾ ਸਿੰਘ ਨੇ ਪਾਕਿਸਤਾਨੀ ਦੌੜਾਕ ਅਬਦੁਲ ਖਾਲਿੰਕ ਨੂੰ ਹਰਾਇਆ ਸੀ ।
टोक्यो में होने वाली एशियाई खेलों में मिल्खा सिंह ने पाकिस्तानी धावक अब्दुल खालिक को पराजित किया।

Question 2.
Why did Milkha Singh not want to go to Pakistan ?
(ਮਿਲਖਾ ਸਿੰਘ ਪਾਕਿਸਤਾਨ ਕਿਉਂ ਨਹੀਂ ਜਾਣਾ ਚਾਹੁੰਦੇ ਸਨ ?)
मिल्खा सिंह पाकिस्तान क्यों नहीं जाना चाहता था ?
Answer:
He did not want to go to Pakistan because of the bitter memories of the partition.
((ਉਹ ਵੰਡ ਦੀਆਂ ਕੁੜੀਆਂ ਯਾਦਾਂ ਦੇ ਕਾਰਨ ਪਾਕਿਸਤਾਨ ਨਹੀਂ ਜਾਣਾ ਚਾਹੁੰਦੇ ਸਨ ।)
वह विभाजन की कटु यादों के कारण पाकिस्तान नहीं जाना चाहता था।

Question 3.
Who gave him the title of ‘Flying Sikh’ ?
(ਉਨ੍ਹਾਂ ਨੂੰ ‘Flying Sikh’ ਦਾ ਖਿਤਾਬ ਕਿਸਨੇ ਦਿੱਤਾ?)
उन्हें ‘Flying Sikh’ की उपाधि किसने दी ?
Answer:
The President of Pakistan gave him the title of ‘Flying Sikh’.
((ਉਨ੍ਹਾਂ ਨੂੰ ‘Flying Sikh’ ਦਾ ਖਿਤਾਬ ਪਾਕਿਸਤਾਨ ਦੇ ਰਾਸ਼ਟਰਪਤੀ ਨੇ ਦਿੱਤਾ)
उन्हें ‘Flying Sikh’ की उपाधि किसने ‘The President of Pakistan’.

(B) Write True / False for the following sentences:

(a) Abdul Khaliq was a Pakistani runner.
(b) Milkha Singh beat Abdul Khaliq.
(c) The President of India gave the title of ‘Flying Sikh to Milkha’.
(d) In Pakistan Abdul Khaliq beat Milkha.
Or
Give the opposites of the following words:
(a) won …………. .
(b) bitter ………… .
(c) gave ………. .
Or
Complete the following sentences:
(a) ………. convinced Milkha to go to Pakistan.
(b) He was invited to Pakistan to take part ……….
Answer:
(a) True
(b) True
(c) False
(d) False.
Or
(a) lost
(b) sweet
(c) took.
Or
(a) Pandit Jawahar Lal Nehru convinced Milkha to go to Pakistan.
(b) He was invited to Pakistan to take part in a race to be held there.

(11) Mahesh Das was born in 1528. Later, he became an advisor in the court of the Mughal emperor, Akbar. The Emperor gave him the name-Raja Birbal. He was one of the nine jewels of Akbar. He was known for his wit.

(A) Question 1.
When was Mahesh Das born ?
(ਮਹੇਸ਼ ਦਾਸ ਦਾ ਜਨਮ ਕਦੋਂ ਹੋਇਆ ਸੀ?)
महेश दास का जन्म कब हुआ?
Answer:
Mahesh Das was born in 1528.
((ਮਹੇਸ਼ ਦਾਸ ਦਾ ਜਨਮ 1528 ਵਿਚ ਹੋਇਆ |)
महेश दास का जन्म 1528 में हुआ

Question 2.
What did he become later ?
(ਬਾਅਦ ਵਿਚ ਉਹ ਕੀ ਬਣਿਆ ?)
बाद में वह क्या बना ?
Answer:
Later, he became an advisor in the court of the Mughal emperor Akbar.
((ਬਾਅਦ ਵਿੱਚ ਉਹ ਮੁਗ਼ਲ ਸਮਰਾਟ ਅਕਬਰ ਦਾ ਸਲਾਹਕਾਰ ਬਣਿਆ ॥)
बाद में वह मुग़ल सम्राट अकबर के दरबार में सलाहकार बना।

Question 3.
Who was known for his wit ?
(ਆਪਣੀ ਬੁੱਧੀਮਤਾ ਦੇ ਲਈ ਕੌਣ ਪ੍ਰਸਿੱਧ ਸੀ ?)
अपनी बुद्धिमत्ता के लिए कौन प्रसिद्ध था ?
Answer:
Raja Birbal was known for his wit.
(ਰਾਜਾ ਬੀਰਬਲ ਆਪਣੀ ਬੁੱਧੀਮਤਾ ਦੇ ਲਈ ਪ੍ਰਸਿੱਧ ਸੀ ।)
राजा बीरबल अपनी बुद्धिमत्ता के लिए प्रसिद्ध था।

(B) Write True / False for the following sentences:

(a) Akbar was born in 1528.
(b) Akbar was the Mughal Emperor.
(c) Mahesh Das was known for his bravery.
(d) Akbar had nine jewels in his court.
Or
Give the opposites of the following words:
(a) born …………. .
(b) known ………. .
(c) wit ……….
Or
Complete the following sentences:
(a) Akbar gave Mahesh Das the name
(b) Raja Birbal was one of the
Answer:
(a) False
(b) True
(c) False
(d) True.
Or
(a) died
(b) unknown
(c) foolishness / dullness.
Or
(a) Akbar gave Mahesh Das the name of Raja Birbal.
(b) Raja Birbal was one of the nine jewels of Akbar.

(12) Then, Akbar came down from his throne. He drew a dark, thick line with a piece of charcoal on the marble floor. Akbar stepped back and asked the courtiers to look at the dark line. Everyone looked at the line carefully. The Emperor said, “You have to make this line shorter but you should not touch it. Do not add to it or rub out any part of it.”

(A) Question 1.
What did Akbar draw on the marble floor ?
(ਸੰਗਮਰਮਰ ਦੇ ਫ਼ਰਸ਼ ‘ਤੇ ਅਕਬਰ ਨੇ ਕੀ ਖਿੱਚਿਆ ?)
संगमरमर के फर्श पर अकबर ने क्या खींचा ?
Answer:
Akbar drew a dark, thick line on the marble floor.
(ਅਕਬਰ ਨੇ ਸੰਗਮਰਮਰ ਦੇ ਫਰਸ਼ ‘ਤੇ ਇਕ ਮੋਟੀ ਕਾਲੀ ਲਾਈਨ ਖਿੱਚੀ ॥)
अकबर ने संगमरमर के फ़र्श पर एक मोटी काली लाइन खींची।

Question 2.
What were the courtiers asked to do ?
(ਦਰਬਾਰੀਆਂ ਨੂੰ ਕੀ ਕਰਨ ਦੇ ਲਈ ਕਿਹਾ ਗਿਆ ?)
दरबारियों से क्या करने के लिए कहा गया ?
Answer:
The courtiers were asked to make the line shorter.
(ਦਰਬਾਰੀਆਂ ਨੂੰ ਉਸ ਲਾਈਨ ਨੂੰ ਛੋਟਾ ਕਰਨ ਦੇ ਲਈ ਕਿਹਾ ਗਿਆ ।)
दरबारियों से उस लाईन को छोटा करने के लिए कहा गया।

Question 3.
What were the two conditions ?
(ਇਹ ਸ਼ਰਤਾਂ ਕਿਹੜੀਆਂ ਸਨ ?)
दो शर्ते कौन-सी थीं ?
Answer:
The two conditions were:
(i) They should not touch the line.
(ii) They should not add to it or rub out any part of it.

(i) ਉਹਨਾਂ ਨੂੰ ਲਾਈਨ ਨੂੰ ਛੂਹਣਾ ਨਹੀਂ ਚਾਹੀਦਾ.
(ii) ਉਨ੍ਹਾਂ ਨੂੰ ਇਸ ਵਿੱਚ ਸ਼ਾਮਲ ਨਹੀਂ ਕਰਨਾ ਚਾਹੀਦਾ ਅਤੇ ਨਾ ਹੀ ਇਸ ਦੇ ਕਿਸੇ ਹਿੱਸੇ ਨੂੰ ਰਗੜਨਾ ਚਾਹੀਦਾ ਹੈ।

(B) Write True / False for the following sentences:

(a) Akbar sat down on his throne.
(b) Everyone looked at the line carefully.
(c) The courtiers were allowed to touch the line.
(d) The line was drawn with a marble.
Or
Give the opposites of the following words:
(a) down ………….
(6) back ………….
(c) add ………. .
Or
Complete the following sentences:
(a) Everyone looked at the line ………….
(b) Akbar came down from his ………….
Answer:
(a) False
(b) True
(c) False
(d) False.
Or
(a) up
(b) forward
(c) subract.
Or
(a) Everyone looked at the line carefully.
(b) Akbar came down from his throne.

(13) Father : Every bank has computers which keep all the records. Whenever we deposit or withdraw money, the employees of the bank maintain a record of all the withdrawals and deposits in the computer.
Aman : How can we withdraw money ?
Father : The bank issues us a cheque book. We can withdraw money
using a cheque leaf, withdrawal slip or ATM card.

(A) Question 1.
What record do the bank employees maintain in the computers ?
(ਬੈਂਕ ਕਰਮਚਾਰੀ ਕੰਪਿਊਟਰ ਵਿਚ ਕਿਹੜੀ ਗੱਲ ਦਾ ਰਿਕਾਰਡ ਰੱਖਦੇ ਹਨ ?)
बैंक कर्मचारी कंप्यूटर में किस बात का रिकॉर्ड रखते हैं ?
Answer:
The bank employees maintain a record of all the withdrawals and deposits in the computer.
(ਬੈਂਕ ਕਰਮਚਾਰੀ ਕੰਪਿਊਟਰ ਵਿਚ ਧਨ ਕਢਵਾਉਣ ਅਤੇ ਜਮਾਂ ਕਰਨ ਦਾ ਰਿਕਾਰਡ ਰੱਖਦੇ ਹਨ । )
बैंक कर्मचारी कंप्यूटर में धन-निकासी तथा जमा धन का रिकॉर्ड रखते हैं।

Question 2.
How can we withdraw money from the bank ?
(ਅਸੀਂ ਬੈਂਕ ਵਿਚੋਂ ਪੈਸੇ ਕਿਸ ਤਰ੍ਹਾਂ ਕੱਢ ਸਕਦੇ ਹਾਂ ?)
हम बैंक से पैसा कैसे निकाल सकते हैं ?
Answer:
We can withdraw money using a cheque leaf, a withdrawal slip or ATM Card.
(ਅਸੀਂ ਪੈਸੇ ਚੈਕ, ਪੈਸੇ ਕਢਾਉਣ ਵਾਲੀ ਪਰਚੀ ਜਾਂ ਏ.ਟੀ.ਐਮ. ਕਾਰਡ ਰਾਹੀਂ ਕਢਾ ਸਕਦੇ ਹਾਂ)
हम चैक आहरण पत्ती अथवा ए०टी०एम० कार्ड द्वारा पैसे निकाल सकते हैं।

Question 3.
Does every bank have computers ?
(ਕੀ ਹਰ ਬੈਂਕ ਦੇ ਕੋਲ ਕੰਪਿਊਟਰ ਹੁੰਦੇ ਹਨ ?)
क्या हर बैंक के पास कम्प्यूटर होते हैं ?
Answer:
Yes, every bank has computers.
(ਹਾਂ, ਹਰ ਬੈਂਕ ਵਿਚ ਕੰਪਿਊਟਰ ਹਨ ।)
हां सभी बैंक के पास कम्प्यूटर हैं।

(B) Write True / False for the following sentences:

(a) Computers keep all the records in banks.
(b) The bank issues us a cheque book.
(c) We can use ony A.T.M card to withdraw money from the bank.
(d) ATM card is useless without withdrawl slip.
Or
Give the opposites of the following words:
(a) all …………..
(b) withdraw ………… .
(c) keep ………. .
Or
Complete the following sentences:
(a) ……….. issues us a cheque book.
(b) Every bank has computers which ……………
Answer:
(a) True
(b) True
(c) False.
Or
(a) some
(b) deposit
(c) destroy.
Or
(a) The bank issues us a cheque book.
(b) Every bank has computers which keep all the records.

(14) Father : ATM card is used to withdraw money at any time even when the bank is not working.
The bank also issues a credit card with which we can transfer money to make payments as I did in the morning. The bank provides us a locker also to keep our valuables.
Aman : How can I open an account in the bank ?
Father : You can open an account by providing an identity proof like the
Aadhar card and your latest photograph to the bank.

(A) Question 1.
What is the use of an ATM card ?
(ਏ.ਟੀ.ਐੱਮ. ਕਾਰਡ ਦੀ ਵਰਤੋਂ ਕੀ ਹੈ?)
ए०टी०एम० कार्ड का क्या उपयोग है ?
Answer:
ATM card is used to withdraw money from the bank at any time.
(ਏ.ਟੀ.ਐੱਮ. ਕਾਰਡ ਦਾ ਪ੍ਰਯੋਗ ਬੈਂਕ ਵਿਚ ਕਿਸੇ ਵੀ ਸਮੇਂ ਧਨ ਕਢਵਾਉਣ ਦੇ ਲਈ ਕੀਤਾ ਜਾਂਦਾ ਹੈ।)
ए०टी०एम० कार्ड का प्रयोग बैंक से किसी भी समय धन-निकासी के लिए किया जाता है।

Question 2.
How can we open an avejuit:ith the bank?
(ਅਸੀਂ ਬੈਂਕ ਵਿਚ ਆਪਣਾ ਖਾਤਾ ਕਿਵੇਂ ਖੋਲ੍ਹ ਸਕਦੇ ਹਾਂ ?) )
हम बैंक में अपना खाता कैसे खोल सकते हैं ?
Answer:
We can open an account with the bank by giving an identity proof like the Aadhar Card and our latest photograph to the bank.
(ਅਸੀਂ ਬੈਂਕ ਵਿਚ ਆਪਣੀ ਪਹਿਚਾਣ ਦਾ ਪ੍ਰਮਾਣ, ਜਿਵੇਂ ਕਿ ਅਧਾਰ ਕਾਰਡ ਅਤੇ ਆਪਣੀ ਨਵੀਂ ਫੋਟੋ ਦੇ ਕੇ ਖਾਤਾ ਖੋਲ੍ਹ ਸਕਦੇ ਹਾਂ )
हम बैंक में अपनी पहचान का प्रमाण जैसे कि आधार कार्ड तथा अपना नवीनतम फोटो देकर खाता खोल सकते हैं।

Question 3.
Where in a bank can we keep our valuables ?
(ਬੈਂਕ ਵਿਚ ਅਸੀਂ ਆਪਣੀਆਂ ਕੀਮਤੀ ਵਸਤੂਆਂ ਕਿੱਥੇ ਰੱਖ ਸਕਦੇ ਹਾਂ ?).
बैंक में हम अपनी कीमती वस्तुएँ कहां रख सकते हैं?
Answer:
We can keep our valuables in a locker provided by the bank.
(ਅਸੀਂ ਆਪਣੀਆਂ ਕੀਮਤੀ ਵਸਤੂਆਂ ਬੈਂਕ ਦੁਆਰਾ ਦਿੱਤੇ ਗਏ ਕਰ ਵਿਚ ਰੱਖ ਸਕਦੇ ਹਾਂ ।)
हम अपनी कीमती वस्तुएं बैंक द्वारा दिए गए लॉकर में रख सकते हैं।

(B) Write True / False for the following sentences:

(a) We can use A.T.M. card only when the bank is working.
(b) We can keep our valuables in a locker.
(c) We don’t need any identity proof to open a bank account.
(d) The bank provides us a computer.
Or
Give the opposites of the following words:
(a) credit ……………
(b) open …………..
(c) morning ………. .
Or
Complete the following sentences:
(a) With the help of a ………. we can …….. money to make payments.
(b) In the morning Father made payment using his ………….
Answer:
(a) False
(b) True
(c) False
(d) False
Or
(a) debit
(b) close
(c) evening.
Or
(a) With the help of a credit card we can transfer money to make payments.
(b) In the morning Father made payment using his credit card.

(15) Cutting of trees is giving us a warning,
That there is an increase in global warming.
It’s increasing day by day, we all are aware,
To control it, we really don’t care.

(A) Question 1.
What is it that is giving us a warning ?
(ਸਾਨੂੰ ਕਿਹੜੀ ਗੱਲ ਚੇਤਾਵਨੀ ਦੇ ਰਹੀ ਹੈ ?)
हमें कौन-सी बात चेतावनी दे रही है ?
Answer:
Cutting of trees is giving us a warning.
(ਦਰੱਖ਼ਤਾਂ ਦੀ, ਕਟਾਈ ਸਾਨੂੰ ਚੇਤਾਵਨੀ ਦੇ ਰਹੀ ਹੈ ।)
वृक्षों की कटाई हमें चेतावनी दे रही है।

Question 2.
What is the warning about ?
(ਇਹ ਚੇਤਾਵਨੀ ਕਿਸ ਦੇ ਬਾਰੇ ਵਿੱਚ ਹੈ ?)
यह चेतावनी किस बारे में है ?
Answer:
The warning is about increasing global temperature.
(ਇਹ ਚੇਤਾਵਨੀ ਵੱਧਦੇ ਹੋਏ ਵਿਸ਼ਵ ਤਾਪਮਾਨ ਦੇ ਬਾਰੇ ਵਿਚ ਹੈ ।)
यह चेतावनी बढ़ते हुए वैश्विक तापमान के बारे में है।

Question 3.
Are we aware of this warning ?
(ਕੀ ਸਾਨੂੰ ਇਸ ਚੇਤਾਵਨੀ ਦੀ ਜਾਣਕਾਰੀ ਹੈ ?)
क्या हमें इस चेतावनी की जानकारी है ?
Answer:
Yes, we all are aware about this warning.
(ਹਾਂ, ਸਾਨੂੰ ਸਾਰਿਆਂ ਨੂੰ ਇਸ ਚੇਤਾਵਨੀ ਦੀ ਜਾਣਕਾਰੀ ਹੈ ।)
हाँ, हम सबको इस चेतावनी की जानकारी है।

(B) Complete the following sentences:

(a) Global temperature is increasing ……………
(b) To control it, we really …………..
Answer:
(a) Global temperature is increasing day by day.
(b) To control it we really don’t care.

(16) High use of fertilizers has raised Earth’s temperature.
Which is, indeed, a threat to Mother Nature.
Our life will soon become a dreadful hell,
And on this planet, it’ll be difficult to dwell.
Now we should find solution for global warming,
To make earth worth living, for generations coming.

(A) Question 1.
What has raised Earth’s temperature ?
(ਧਰਤੀ ਦਾ ਤਾਪਮਾਨ ਕਿਹੜੀ ਚੀਜ਼ ਨੇ ਵਧਾਇਆ ਹੈ ?)
धरती का तापमान किस चीज़ ने बढाया है ?
Answer:
High use of fertilizers has raised Earth’s temperature.
(ਰਸਾਇਣਿਕ ਖਾਦਾਂ ਦੇ ਜ਼ਿਆਦਾ ਪ੍ਰਯੋਗ ਨੇ ਧਰਤੀ ਦੇ ਤਾਪਮਾਨ ਨੂੰ ਵਧਾ ਦਿੱਤਾ ਹੈ ।)
रासायनिक खादों के बहुत अधिक प्रयोग ने धरती के तापमान को बढ़ा दिया है।

Question 2.
What will be its result ?
(ਇਸ ਦਾ ਕੀ ਪਰਿਣਾਮ ਹੋਵੇਗਾ ?)
इसका क्या परिणाम होगा ?
Answer:
It will make our life a dreadful hell. It will become difficult for us to live on Earth.
(ਇਹ ਸਾਡੇ ਜੀਵਨ ਨੂੰ ਇਕ ਭਿਆਨਕ ਨਰਕ ਬਣਾ ਦੇਵੇਗਾ । ਸਾਡੇ ਲਈ ਧਰਤੀ ‘ਤੇ ਰਹਿਣਾ ਸੁਸਕਿਲ ਹੇ ਜਾਵੇਗਾ ।)
यह हमारे जीवन को एक भयानक नरक बना देगा। हमारे लिए धरती पर रह पाना मुश्किल हो जायेगा।

Question 3.
Why is it essential to find solution for global warming?
(ਵਿਸ਼ਵ ਤਾਪਮਾਨ ਦਾ ਹੱਲ ਲੱਭਣਾ ਕਿਉਂ ਜ਼ਰੂਰੀ ਹੈ ?)
वैश्विक तापमान का समाधान ढूंढना क्यों अनिवार्य है?
Answer:
It is essential to make earth worth living for coming generations.
(ਇਹ ਧਰਤੀ ਨੂੰ ਆਉਣ ਵਾਲੀਆਂ ਸੰਤਾਨਾਂ ਦੇ ਰਹਿਣ ਯੋਗ ਬਣਾਉਣ ਦੇ ਲਈ ਜ਼ਰੂਰੀ ਹੈ ॥)
यह धरती को आने वाली संतानों के रहने योग्य बनाने के लिए अनिवार्य है।

(B) Complete the following sentences:

(a) Our planet is …………………..
(b) Global warming is a threat to ………..
Answer:
(a) Our planet is Earth.
(b) Global warming is a threat to Mother Nature.

(17) This sect later developed into the great historic ‘Kuka Movement of the Namdhari Sikhs. In 1872, the Kuka Sikhs attacked Malerkotla where one Mr. Cowen was the British officer in charge of the town. After the attack on Malerkotla, sixty-six Kuka Sikhs surrendered themselves. Cowen was a very cruel and heartles officer.

(A) Question 1.
Whose movement was the Kuka Movement ?
(ਕੂਕਾ ਅੰਦੋਲਨ ਕਿਸ ਦਾ ਅੰਦੋਲਨ ਸੀ ?)
कूका आंदोलन किसका आंदोलन था ?
Answer:
The ‘Kuka Movement was the movement of the Namdhari Sikhs.
(‘ਕੂਕਾ ਅੰਦੋਲਨ’ ਨਾਮਧਾਰੀ ਸਿੱਖਾਂ ਦਾ ਅੰਦੋਲਨ ਸੀ ॥)
‘कूका आंदोलन’ नामधारी सिक्खों का आंदोलन था।

Question 2.
Who was Mr. Cowen ?
(ਮਿਸਟਰ ਕੇਵਰ ਭੇਂਟ ਮੀ ?)
मि० कोविन कौन था ?
Answer:
Mr. Cowen was the British officer in charge of Malerkotla.
(ਮਿ. ਕੋਵਨ ਇਕ ਅੰਗਰੇਜ਼ ਅਧਿਕਾਰੀ ਸਨ ਜਿਹੜੇ ਮਲੇਰਕੋਟਲਾ ਦੇ ਇੰਚਾਰਜ ਸਨ)
मि० कोवन एक अंग्रेज अधिकारी था जो मलेरकोटला का इंचार्ज था।

Question 3.
Complete the sentence:
………… Kuka Sikhs surrendered themselves.
Answer:
Sixty-six
((ਛਿਆਠ ॥)
छियासठ।

(B) Write True / False for the following sentences:

(a) The Kuka Sikhs attack Malerkotla in 1782.
(b) Mr. Cowen was a British Officer.
(c) Sixty six Kuka Sikhs surrundered themselves.
(d) Kuka Sikhs were Namdhari.
Or
Give the opposites of the following words:
(a) great …………. .
(b) town ………….
(c) cruel ………….
Answer:
(a) False
(b) True
(c) True
(d) True
Or
(a) small
(b) village
(c) kind.

(18) The year 1857 was not an ordinary year. The people of India everywhere were angry with the British Rule. They were burning with a desire to throw the British rulers out of India and attain independence. The British government was very powerful. But this did not discourage the fighters for freedom. They were out on the path of revolt, struggle and war.

(A) Question 1.
Who did the people want to throw out of India ?
(ਲੋਕ ਭਾਰਤ ਤੋਂ ਬਾਹਰ ਕਿਸਨੂੰ ਖਦੇੜਣਾ ਚਾਹੁੰਦੇ ਸਨ ?)
लोग भारत से बाहर किन को खदेड़ना चाहते थे?
Answer:
The people wanted to throw the British rulers out of India.
(ਲੋਕ ਅੰਗਰੇਜ਼ ਸ਼ਾਸਕਾਂ ਨੂੰ ਭਾਰਤ ਤੋਂ ਬਾਹਰ ਖਦੇੜਨਾ ਚਾਹੁੰਦੇ ਸਨ ॥)
लोग अंग्रेज शासकों को भारत से बाहर खदेड़ना चाहते थे ।

Question 2.
What did the Indians want to attain?
(ਭਾਰਤੀ ਕੀ ਪ੍ਰਾਪਤ ਕਰਨਾ ਚਾਹੁੰਦੇ ਸਨ ?) 
भारतीय क्या प्राप्त करना चाहते थे ?
Answer:
The Indians wanted to attain independence.
(ਭਾਰਤੀ ਸੁਤੰਤਰਤਾ ਪ੍ਰਾਪਤ ਕਰਨਾ ਚਾਹੁੰਦੇ ਸਨ ।)
भारतीय स्वतंत्रता प्राप्त करना चाहते थे।

Question 3.
Who were out on the path of revolt ?
(ਵਿਦਰੋਹ ਕਰਨ ਤੇ ਕੌਣ ਉਤਾਰੂ ਸਨ ?)
विद्रोह के पथ पर कौन अग्रसर थे ?
Answer:
The fighters for freedom were out on the path of revolt.
(ਸੁਤੰਤਰਤਾ ਸੈਨਾਨੀ ਵਿਦਰੋਹ ‘ਤੇ ਉਤਾਰੂ ਸਨ ॥)
स्वतंत्रता सेनानी विद्रोह के पथ पर अग्रसर थे।

(B) Write True / False for the following sentences:

(a) The British government was very weak.
(b) The people of India were angry with the British rule.
(c) Fighters for freedom were out on the path of revolt.
(d) The year 1875 was not an ordinary one.
Or
Give the opposites of the following words:
(a) ordinary ………….
(b) out …………
(c) war …………. .
Or
Complete the following sentences:
(a) The year 1857 was not an …………
(b) The British government was …………
Answer:
(a) False
(b) True
(c) True
(d) False
Or
(a) extra-ordinary
(b) in
(c) peace.
Or
(a) The year 1857 was not an ordinary year.
(b) The British government was very powerful.

(19) One Namdhari Sikh, named Waryam Singh, was too short in stature to reach the level of the cannon’s mouth. He was so brave that he collected some pebbles and stood upon them and then challenged the British officer to fire. Another boys Bishan Singh, was twelve years old. He was cut to pieces because he caught hold of the beard of the British officer, Mr. Cowen. Such was the great spirit of these fighters for freedom.

(A) Question 1.
Who was too short in stature to reach the level of the cannon’s mouth ?
(ਕੌਣ ਕੱਦ ਵਿਚ ਐਨਾ ਛੋਟਾ ਸੀ ਕਿ ਉਹ ਤੋਪ ਦੇ ਮੂੰਹ ਤਕ ਨਹੀਂ ਪਹੁੰਚ ਸਕਦਾ ਸੀ ?)
किसका कद इतना छोटा था कि वह तोप के मुंह तक नहीं पहुंच सकता था ?
Answer:
His name was Waryam Singh.
ਉਸਦਾ ਨਾਮ ਵਰਿਆਮ ਸਿੰਘ ਸੀ।
उसका नाम वशि्याम सिंह था

Question 2.
What did Waryam Singh do to reach the cannon’s mouth ?
(ਤੋਪ ਦੇ ਮੁੰਹ ਤੱਕ ਪਹੁੰਚਣ ਲਈ ਵਰਿਆਮ ਸਿੰਘ ਨੇ ਕੀ ਕੀਤਾ ?)
तोप के मुँह तक पहुंचने के लिए वरियाम सिंह ने क्या किया ?
Answer:
Waryam Singh collected some pebbles and stood upon them.
(ਵਰਿਆਮ ਸਿੰਘ ਨੇ ਕੁੱਝ ਪੱਥਰ ਇਕੱਠੇ ਕੀਤੇ ਅਤੇ ਉਨ੍ਹਾਂ ‘ਤੇ ਖੜ੍ਹਾ ਹੋ ਗਿਆ ।)
वरियाम सिंह ने कुछ पत्थर इकट्ठे किए और उन पर खड़ा हो गया।

Question 3.
How old was Bishan Singh ?
(ਬਿਸ਼ਨ ਸਿੰਘ ਦੀ ਉਮਰ ਕਿੰਨੀ ਸੀ ?)
वरियाम सिंह की आयू कितनी थी ?
Answer:
Bishan Singh was twelve years old.
(ਬਿਸ਼ਨ ਸਿੰਘ ਬਾਰਾਂ ਸਾਲਾਂ ਦਾ ਸੀ।)
वरियाम सिंह की आयू 12 वर्ष थी

(B) Write True / False for the following sentences:

(a) Waryam Singh was a Namdhari Sikh.
(6) Bishan Singh was twenty years old.
(c) Bishan Singh caught the British Officer by the car.
(d) Waryam Singh was a Namdhari Sikh.
Or
Give the opposites of the following words:
(a) short …………
(b) brave ……….
(c) old ………..
Answer:
(a) True
(b) False
(c) False
(d) True
Or
(a) tall
(b) coward
(c) new.

(20) One day, a bear felt hungry. So, he come out of his den to look for food. The bear went to the river to catch some fish. He stood by river to and saw enough fish to eat. He cought a small fish, but then he thought “This is too small fish to fill my stomach. I must catch bigger, fish. So, he let off the small fish and waited for some time.

(A) Question 1.
Why did bear come out of this den?
(ਭਾਲੂ ਆਪਣੀ ਗੁਫ਼ਾ (ਮਾਦ) ਵਿੱਚੋਂ ਬਾਹਰ ਕਿਉਂ ਆਇਆਂ ?)
भालू अपनी माद से बाहर क्यों आया ?
Answer:
The bear come out of his den to look for food.
(ਭਾਲੁ ਭੋਜਨ ਦੀ ਤਲਾਸ਼ ਵਿੱਚ ਬਾਹਰ ਆਇਆ )
भालू भोजन की तलाश में बाहर आया।’

Question 2.
What did bear see in the river ?
(ਭਾਲੂ ਨੇ ਨਦੀ ਵਿੱਚ ਕੀ ਦੇਖਿਆ ?)
भालू ने नदी में क्या देखा?
Answer:
He saw enough fish to eat.
(ਉਸ ਨੇ ਖਾਣ ਲਈ ਕਾਫ਼ੀ ਮੱਛੀ ਦੇਖੀ।)
उसने खाने के लिए बहुत सी मछलियां देखी।

Question 3.
What did the bear catch ?
(ਭਾਲੂ ਨੇ ਕੀ ਚੀਜ਼ ਫੜੀ ?)
भालू ने क्या चीज पकड़ी?
Answer:
He caught a small fish.
(ਉਸਨੇ ਇੱਕ ਛੋਟੀ ਮੱਛੀ ਫੜੀ।)
उसने एक छोदो मछली पकड़ी?

(B) Write the True/False for the following sentences.

(a) The bear came out of his den to look for food.
(b) The bear went to his den.
(c) He stood by the river and saw no fish to eat.
(d) One day a bear felt thirsty.
Or
Give the opposites of the following words
(a) catch ……………
(b) some ………..
(c) come ……..
Answer:
(a) True
(b) False
(c) False
(d) False
Or
(a) free
(b) many
(c) go.

(21) Then again a small fish came and he let it go thinking that the small fish would not fill his belly. This way he caught many small fish, but let all of them go off. By sunset, the bear had not caught any big fish. He slowly began to feel tired. At the end he remained empty stomach and thought “All those small fish, together would have filled up my belly, but now it was too late” He remained hungry that day.

(A) Question 1.
How many fish did he catch ?
(ਉਸਨੇ ਕਿੰਨੀਆਂ ਮੱਛੀਆਂ ਫੜੀਆਂ ?)
उसने कितनी मछलियां पकड़ी?
Answer:
He caught many fish.
(ਉਸਨੇ ਬਹੁਤ ਮੱਛੀਆਂ ਫੜੀਆਂ )
उसने बहुत मछलियां पकड़ी?

Question 2.
What did he do with them ?
(ਉਸਨੇ ਉਨ੍ਹਾਂ ਦਾ ਕੀ ਕੀਤਾ ?)
उसने उनका क्या किया?
Answer:
He let them go.
(ਉਸਨੇ ਉਨ੍ਹਾਂ ਨੂੰ ਛੱਡ ਦਿੱਤਾ ॥)
उसने उन्हें छोड़ दिया।

Question 3.
Why did he remain hungry that day ?
(ਉਸ ਦਿਨ ਉਹ ਭੁੱਖਾ ਕਿਉਂ ਰਹਿ ਗਿਆ ?)
उस दिन वह भूखा कये रह गाया ?
Answer:
He remained hungry because he let all the small fish go off.
ਉਸਨੂੰ ਇਸ ਲਈ ਭੁੱਖਾ ਰਹਿਣਾ ਪਿਆ ਕਿਉਂਕਿ ਉਸਨੇ ਸਾਰਿਆਂ ਛੋਟੀਆਂ ਮੱਛੀਆਂ ਨੂੰ ਛੱਡ ਦਿੱਤਾ ।)
उसे इसलिए भूखा रहना पड़ा क्योंकि उसने सभी छोटी मछलियों को छोड़ दिया।

(B) Write True / False for the following sentences:

(a) He let that small fish to go.
(b) Slowly he began to feel refreshed.
(c) He caught only big fish.
(d) He remained thirsty that day.
Or
Give the opposites of the following words:
(a) small …………..
(b) sunset …………………. .
(c) empty ………………… .
Answer:
(a) True
(b) False
(c) False
(d) False
Or
(a) big
(b) sunrise
(c) filled.

(22) A great batsman, Sachin Tendulkar was born on 24 April 1973 in Maharashtra. He liked cricket since his childhood. He was given the title ‘Master Blaster’ for his wonderful performance in the field of cricket. He holds several batting records. He has perfect cricket skills. He was conferred the Arjuna Award in 1994, the Rajiv Gandhi Khel Ratna Award in 1997-98 and the Bharat Ratna in 2013.

(A) Question 1.
When was Sachin born ?
(ਸਚਿਨ ਦਾ ਜਨਮ ਕਦੋਂ ਹੋਇਆ ਸੀ?)
सचिन का जन्म कब हुआ ?
Answer:
Sachin was born on 24 April 1973.
(ਸਚਿਨ ਦਾ ਜਨਮ 24 ਅਪ੍ਰੈਲ, 1973 ਨੂੰ ਹੋਇਆ ।)
सचिन का जन्म 24 अप्रैल 1973 को हुआ

Question 2.
What title was he given for his performance in cricket ?
(ਕ੍ਰਿਕੇਟ ਵਿੱਚ ਪ੍ਰਦਰਸ਼ਨ ਲਈ ਉਸ ਨੂੰ ਕਿਹੜੀ ਉਪਾਧੀ ਮਿਲੀ ?)
किक्रेट में प्रदर्शन के लिए उसे कौन-सी उपाधि मिली ?
Answer:
He was given the title ‘Master Blaster’.
(ਉਸਨੂੰ “Master Blaster’ ਦੀ ਉਪਾਧੀ ਦਿੱਤੀ ਗਈ )
उसे ‘Master Blaster’ की उपाधि दी गई

Question 3.
Name the Award title/ he won.
(ਉਸਨੂੰ ਮਿਲਣ ਵਾਲੇ ਪੁਰਸਕਾਰਾਂ/ਉਪਾਧੀਆਂ ਦੇ ਨਾਮ ਦੱਸੋ )
उस मिलने वाले पुरस्कारों के नाम बताओ।
Answer:
(i) The Arjuna Award in 1994.
(ਅਰਜੁਨ ਅਵਾਰਡ 1994 ਵਿਚ, )
(ii) The Rajeev Gandhi Khel Ratna Award in 1997-98.
(ਰਾਜੀਵ ਗਾਂਧੀ ਖੇਲ ਰਤਨ ਅਵਾਰਡ 1997-98 ਵਿਚ ॥)
(iii) The Bharat Ratna 2013.
(ਭਾਰਤ ਰਤਨ 2013)

(B) Write the True / False for the following sentences:

(a) Sachin holds no batting records.
(b) Sachin was born in Maharashtra.
(c) Sachin has perfect cricket skills.
(d) Sachin Tendulkar was an ordinary batsman.
Or
Give the opposites of the following words:
(a) childhood
(b) liked
(c) perfect.
Answer:
(a) False
(b) True
(c) True
(d) False.
Or
(a) youth
(b) disliked
(c) imperfect.

(23) Sonia was a little girl. She was six years old. She was playing in the backyard of her house She was looking at the different kinds of flowers. There came a beautiful butterfly. She tried to catch it but could not. So she was disappointed.

(A) Question 1.
What was the age of Sonia ?
(ਸੋਨੀਆ ਦੀ ਉਮਰ ਕਿੰਨੀ ਸੀ?)
सोनिया की उम्र क्या थी ?
Answer:
Sonia was six years old.
(ਸੋਨੀਆ ਦੀ ਉਮਰ ਛੇ ਸਾਲ ਸੀ)
सोनिया की उम्र छः साल थी

Question 2.
Why was Sonia disappointed ?
(ਸੋਨੀਆ ਨਿਰਾਸ਼ ਕਿਉਂ ਹੋਈ?)
सोनिया निराश क्यों थी ?
Answer:
Because she failed to catch the butterfly.
(ਕਿਉਂਕਿ ਉਹ ਤਿਤਲੀ ਨੂੰ ਫੜਨ ਵਿਚ ਅਸਫਲ ਰਹੀ ।
क्योंकि वह तितली को पकड़ने में असफल रही।

Question 3.
Where was Sonia ?
(ਸੋਨੀਆ ਕਿੱਥੇ ਸੀ?)
सोनिया कहाँ थी ?
Answer:
She was in the backyard of her house.
(ਉਹ ਆਪਣੇ ਘਰ ਦੇ ਪਿਛਲੇ ਵਿਹੜੇ ਵਿਚ ਸੀ ।)
वह अपने घर के पिछवाड़े में थी।

(B) Write whether the following statements are true or false:

(a) Sonia was nine years old.
(6) There were many kinds of flowers.
(c) Two beautiful butterflies came there.
(d) She could not catch the butterfly.
Answer:
(a) False
(b) True
(c) False
(d) True.

General Vocabulary

1. Class-room Objects

Bench
Bag
Chair
Map
Pen
Register
Table
Desk
Fan
Blackboard
Paper
Book
Pencil
Chalk
Sharpener
Duster

2. Dress

Pants
Cap
Shoes
Clothes
Skirt
Belt
Coat
Frock
Shirt
Socks

3. Counting

One
Two
Three
Four
Five
Six
Seven
Eight
Nine
Ten
Eleven
Twelve
Thirteen
Fourteen
Fifteen
Sixteen
Seventeen
Eighteen
Nineteen
Twenty
Thirty
Forty
Fifty
Sixty
Seventy
Eighty
Ninety
Hundred

4. Relations

Mother
Brother
Son
Father
Wife
Aunt
Daughter
Husband
Sister
Uncle

5. Colours

Orange
Blue
Red
Grey
Yellow
Maroon
Pink
Black
Brown
Green
Violet
White

6. Parts of the Body

Wrist
Hair
Finger
Mouth
Nail
Eyes
Chest
Neck
Back
Lips
Knee
Tongue
Ear
Shoulder
Head
Forehead
Cheek
Eyebrows
Nose
Tooth
Jaw
Chin
Hand
Thumb
Palm
Waist
Thigh
Arm
Leg
Тое
Foot
Heel

7. Days of the Week

Sunday
Monday
Tuesday
Wednesday
Thursday
Friday
Saturday

8. Months of the Year

January
February
March
April
May
June
July
August
September
October
November
December

9. Animals – Domestic (D) and Wild (W)

Ass (D)
Cow (D)
Bitch (D)
Deer (W)
Camel (D)
Dog (D)
Cat (D)
Elephant (W)
Goat (D)
Hare (D)
Horse (D)
Sheep (W)
Lion (W)
Snake (W)
Mare (D)
Wolf (W)
Monkey (W)
Zebra (W)

10. Birds

Cock
Hen
Crow
Parrot
Duck
Peacock

11. (a) Fruits and (b) Vegetables

(a) Apple
Mango
Date
Pear
Guava
Papaya
Banana
Melon
Grapes
Plum / Berry

(b) Cabbage
Potato
Peas
Tomato
Carrot
Radish
Lady’s Finger
Brinjal

12. Parts of a Computer

Monitor (Screen)
Printer
Processor
Keyboard
Mouse
Modem
Memory (RAM)
Floppy Disks
Hard Disks

Punjab State Board PSEB 5th Class English Book Solutions English Grammar Conversation Exercise Questions and Answers, Notes.

PSEB 5th Class English Grammar Conversation

WHAT

1. What is your name ? – My name is Raj.
2. What is your father ? – My father is a teacher.
3. What is the name of your father ? – The name of my father is Shri
4. What are you ? – I am a student.
5. What is she to you ? – She is my sister.
6. What colour is the sky ? – It is blue.
7. What do you see in the sky ? – I see birds in the sky.
8. What colour is your shirt ? – It is yellow.
9. What do you want ? – I want a pen.
10. What are you doing ? – I am writing a letter.

WHO

1. Who is she ? – She is Simran.
2. Who are you ? – I am Tejpal.
3. Who teaches you English ? – Shri B.S. Virk teaches us English.
4. Who is the Headmaster of your school ? – Mr. Singh is the Headmaster of our school.
5. Who is your mother ? – Mrs ………… is my mother.

WHOSE

1. Whose book is this ? – This is my book.
2. Whose house is that ? – That is Mohan’s house.
3. Whose toys are those ? – Those are Sheela’s toys.
4. Whose daughter is she ? – She is Mr. Sharma’s daughter.

WHICH

1. Which is your cap ? – The red one is my cap.
2. Which pen do you like ? – I like the red pen.
3. Which hand do you write with ? – I write with my right hand.
4. Which game do you play ? – I play cricket.
5. In which class do you read ? – I read irt the 5th class.
6. In which school do you read ? – I read in A.B. School.

WHERE

1. Where is your book ? – It is on the table.
2. Where do you live ? – I live at Rampur.
3. Where is your turban ? – It is on my head.
4. Where is Sri Harimandar Sahib ? – Sri Harimandar Sahib is in Amritsar.
5. Where is the Taj ? – The Taj is in Agra.
6. Where is the Red Fort ? – The Red Fort is in Delhi.

WHEN

1. When do you get up in the morning ? – I get up at 6 a.m.
2. When do you go to school ? – I go to school at 9 a.m.
3. When does the sun rise ? – The sun rises at 6 a.m.
4. When does your school open in summer ? – Our school opens at 7 a. m. in summer.
5. When does your school closein winter ? – Our school closes at 3 p.m. in winter
6. When does the sun set ? – The sun sets at 6 p.m.

WHY

1. Why are you late today ? – I missed the first bus.
2. Why do you go to school ? – We go to school to get education.
3. Why are you sad ? – I have lost my pen.

HOW

1. How do you do ? – I am quite well, thank you.
2. How are you ? – I am all right.
3. How old are you ? – I am ten years old.
4. How do you go to school ? – I go to school on foot.

HOW MANY

1. How many brothers are you ? – We are three brothers.
2. How many brothers have you ? – I have two brothers.
3. How many days has a week ? – A week has seven days.
4. How many months are there in a year ? – There are twelve months in a year.
5. How many students are there in your class ? – There are thirty students in our class.
6. How many teachers are there in your school ? – There are thirty teachers in our school.

Punjab State Board PSEB 5th Class English Book Solutions English Grammar Guided Composition Exercise Questions and Answers, Notes.

PSEB 5th Class English Grammar Guided Composition

1.
(ਕਿਸੇ ਵਿਅਕਤੀ ਵਸਤ ਜਾਂ ਜੀਵ ਦਾ ਵਰਣਨ ਕਰਦੇ ਸਮੇਂ ਮੁੱਖ ਤੌਰ ‘ਤੇ is/tv ਅਤੇ ਉਸ ਵਿਸ਼ੇਸ਼ਤਾ ਦੀ ਵਰਤੋਂ ਕੀਤੀ ਜਾਂਦੀ ਹੈ, ਜਿਵੇਂ-)किसी व्यक्ति, वस्तु या जीव का वर्णन करते समय मुख्य रूप से is/are तथा उसकी विशेषता का प्रयोग किया जाता है, जैसे-
(a) It is a small book.
(b) My school is very big.
(c) Ravi/My father is ………… years old.

2.
(ਵਸਤੂਆਂ ਅਤੇ ਜੀਵਾਂ ਦੇ ਆਕਾਰ, ਰੰਗ-ਰੂਪ ਦਾ ਵਰਣਨ ਕਰਦੇ ਸਮੇਂ ਮੁੱਖ ਤੌਰ ਤੇ It has ਦੀ ਵਰਤੋਂ ਕੀਤੀ ਜਾਂਦੀ ਹੈ, ਜਿਵੇਂ-)
वस्तुओं तथा जीवों के आकार, रंग-रूप का वर्णन करते समय मुख्य रूप से It has का प्रयोग किया जाता है, जैसे-
(a) It has 100 pages. (Book)
(b) It has four legs. (Cow,Table)

3.
(ਕਿਸੇ ਸਥਾਨ (Place) ਦਾ ਵਰਣਨ ਕਰਦੇ ਸਮੇਂ ਆਮ ਤੌਰ ‘ਤੇ It has……… There is ……….., There are ………….. ਦੀ ਵਰਤੋਂ ਹੁੰਦੀ ਹੈ, ਜਿਵੇਂ-)
किसी स्थान (Place) का वर्णन करते समय प्रायः It has…..; There is …………. There are ……………. का प्रयोग होता है, जैसे-
(a) It has a big playground. (School)
Or
There is a big playground in it.
(b) There are many flower plants in the garden.

4.
(Picture Composition ਵਿੱਚ ਦਿਖਾਈਆਂ ਗਈਆਂ ਗਤੀਵਿਧੀਆਂ ਲਈ Present Continuous Tense (is/am/are ਆਡੇ Verb et First Form + ing) ਦੀ ਵਰਤੋਂ ਕਰਨੀ ਚਾਹੀਦੀ ਹੈ, ਜਿਵੇਂ-)
Picture Composition में दिखाई गई गतिविधियों के लिए Present Continuous Tense (is/am/are तथा Verb की First Form + ing) का प्रयोग करना चाहिए; जैसे-
(a) Two girls are swinging.
(b) A man is walking on the grass.
(c) The sun is shining.
(d) Birds are flying.

5.
(ਹੋਰ ਗੱਲਾਂ ਦੀ ਵਿਆਖਿਆ ਲਈ ਦਿਖਾਈ ਗਈ ਸਥਿਤੀ ਨੂੰ ਧਿਆਨ ਵਿੱਚ ਰੱਖਦੇ ਹੋਏ ਸਹੀ Tense ਅਤੇ ਕਿਸੇ ਉਚਿੱਤ ਵਾਕ-ਰਚਨਾ ਦੀ ਵਰਤੋਂ ਕਰਨੀ ਚਾਹੀਦੀ ਹੈ, ਜਿਵੇਂ-)
अन्य बातों की व्याख्या के लिए दिखाई गई स्थिति को ध्यान में रखते हुए सही Tense तथा किसी उचित वाक्य रचना का प्रयोग करना चाहिए; जैसे-
(a) She/He gets up early in the morning. (Person)
(b) It gives us milk. (Cow)
(c) They give us fruit and wood. (Trees)

ਵਿਸ਼ੇਸ਼ ਨੋਟ-Textbook ਦੀ Exercises ਵਿੱਚ ਦਿੱਤੇ ਗਏ Composition Writing ਦੇ Topics ਦਾ ਅਧਿਐਨ ਜ਼ਰੂਰ ਕਰੋ । ਪ੍ਰੀਖਿਆ ਦੇ ਨਜ਼ਰੀਏ ਤੋਂ ਉਹ ਸਭ ਤੋਂ Important ਹਨ ।

विशेष नोट-Textbook की Exercises में दिए गए Composition Writing के Topics का अध्ययन अवश्य करें। परीक्षा की दृष्टि से ये सबसे Important हैं।

A.1. Picture (Visual) Composition

Write four/five sentences on each of the given pictures.
(1)

1. The sun is shining.
2. A bird is flying.
3. An elephant is standing near a big tree.
4. Some flowers are blooming.
5. Five stars are shining around the moon.

(2)

1. It is a rainy day.
2. It is raining heavily.
3. Two boys and two girls are having fun.
4. Three children are standing under umbrellas.
5. They are feeling the rain drops.
6. A girl is splashing water with her feet.
7. All look happy.

(3)

1. A man is asking about the clothes.
2. The woman is selling clothes for Christmas.
3. The dresses are very colourful.
4. The cap is for Santa Claus.

(4)

1. It is a hilly area.
2. There are small huts on the hills.
3. A tree stands near them.
4. Besides the hills, there is a big tree.
5. A river/canal is flowing near it.
6. There is a bridge to cross it.
7. The scene is very beautiful.

(5)

1. It is a morning scene.
2. The sun is up.
3. There is a big hut.
4. It is in front of a tall tree.
5. There is a flower bed near the tree.
6. A boy and his sister are watering the plants.

(6)

1. It is a snowy weather.
2. Some children are playing on the snow.
3. They are wearing warm clothes.
4. Their caps are very beautiful.
5. A girl is putting on a cap on the funny statue.
6. A boy is lying on the snow.
7. A puppy is running towards him.
8. A leafy plant is the beauty of the scene.

(7)

1. It is a dirty place on the road side.
2. Heaps of garbage are lying here and there.
3. It is giving a foul smell.
4. It may spread diseases.
5. A team of local workers is removing it.
6. They are collecting it into bags.
7. They may also fall ill.
8. They have masks on their face for safety.

(8)

1. It is a big library.
2. Books are lying on shelves.
3. Some books are big; some are small.
4. Two boys are reading books.
5. A girl is taking out a book to read.
6. There is clock on the wall.

A. 2. Composition-Completion of Sentences

Write five sentences on each of the given topics.

1. My Grandmother (very caring, love, sixty-five, stories)
(a) My …………..
(6) She ……………
(c) She tells ………….
(d) She ……………
(e) I ………….
Answer:
(a) My grandmother is sixty-five years old.
(b) She is very caring.
(c) She tells us interesting stories.
(d) She loves us very much.
(e) I enjoy her company very much.

ਨੋਟ-ਇਸੇ ਆਧਾਰ ‘ਤੇ ਤੁਸੀਂ ਆਪਣੀ Mother, Father ਅਤੇ Grandfather ਦੇ ਬਾਰੇ ਵਿੱਚ ਵੀ ਲਿਖ ਸਕਦੇ ਹੋ ।

नोट-इसी आधार पर आप अपनी Mother, Father तथा Grandfather के बारे में भी लिख।

2. My Younger Brother/Sister
(cute, naughty, tears, loves to play, two years old)

(a)- My younger brother/sister is two years old.
(b) He/She is very cute.
(c) He/She loves to play with moving toys and speaking dolls.
(d) He/She is very naughty.
(e) He/She often tears my books.
(f) love him/her very much.

3. My Best Friend
(obeys, old, like, class-fellow, good student)

(a) Ravi/Raveena is my best friend.
(b) He/She is nine years old.
(c) He/She is my class-fellow.
(d) He/She is a good student.
(e) He/She obeys his/her teachers.
(f) All like him/her very much.

4. My Teacher
(age, teaches, like, helpful)

(a) The name of my teacher is Mr. Anmol Sharma.
(b) He is 30 years old.
(c) He teaches us English.
(d) All of us like him very much.
(e) He helps the poor students.

5. My School
(rooms, teachers, large, loving and kind, read, well-qualified)

(a) I read in S.S. Secondary School.
(b) It is a big school.
(PB. 2020) (c) It has 30 rooms.
(d) It has a large playground.
(e) There are 25 teachers in our school.
(f) They are well-qualified.
(g) They are loving and kind also.

6. Trees
(useful, furniture, purify, things, fruits, shade, shelter).

(a) Trees are very useful.
(b) They give us many things.
(c) They give us fruits to eat.
(d) They give us cool shade in summer.
(e) They give shelter to many birds
(f) They give us wood to make furniture.
(g) They purify air for us.

7. My Pet Dog
(barks, plays, pet, little, fast, black, bread and meat, watches)

(a) Tomy is my pet dog.
(b) It is a little black dog.
(c) It eats bread and meat.
(d) It plays with the ball.
(e) It runs very fast.
(f) It watches our house at night.
(g) It barks at strangers.
(h) I love playing with Tomy.

8. A Computer
(T.V. set, useful, operate, keyboard, printer, mouse, screen, monitor)

(a) A computer is very useful.
(b) It looks like a T.V. set.
(c) It has a camera in it.
(d) Its screen is called monitor.
(e) Its brain is called C.P.U.
(f) It has a printer also.
(g) We operate it with the help of a keyboard and a mouse.

9. Seasons
(winter, summer, autumn, rains, hot, cold season)

(a) There are four seasons in a year.
(b) Their names are Summer, Winter, Rainy and Autumn Season.
(c) Summer is a hot season.
(d) The sun is very bright in Summer.
(e) Winter is a cold season.
(f) It rains in the rainy season.
(g) In Autumn trees fall off their leaves.

10. Corona (Golden Safety Rules)
(Do’s and Don’ts)

(wash regularly, home, safe, sanitiser, distance)
(crowded place, touch, spit, mouth and nose covered)

Do’s
(a) Stay home, stay safe.
(b) Always cover your face with a clean mask or a handkerchief.
(c) Keep distance of atleast 1 meter from others.
(d) Wash your hands with soap regularly.
(e) Clear your hands with a sanitiser.

Don’ts
(a) Don’t go out of your home.
(b) If necessary, don’t go out without your mouth and nose covered.
(c) Do not spit here and there.
(d) Don’t touch your face.
(e) Don’t go near a crowded place.

A.3. PARAGRAPHS

(Based on Outlines)

1. Develop a paragraph on ‘My Book’ from the hints given below:
Hints : I have English Reader a small book eighty pages …………. from the market ………….. many pictures ………… ten stories ………… fifteen rupees ………….. like ………. read daily.

1. MY BOOK

I have a book. It is an English Reader. It is a small book. It has eighty pages. It has many pictures. It has ten stories. It has two poems. I bought it from the market. Its price is fifteen rupees. It is a good book. I like it very much. I read it daily.

2. Develop a paragraph on ‘My Chair’ from the hints given below:
Hints : A chair …………. wood and cane …………. carpenter …………. two arms …………. four legs …………. good seat …………. a fine back …………. from the market …………. like very much.

2. MY CHAIR

I have a chair. It is made of wood and cane (). A carpenter made it. It is painted brown. It has two arms. It has four legs. It has a good seat. It has a fine back. It is to sit in. I bought it from the market. Its price is two hundred rupees. I like it very much.

3. Develop a paragraph on ‘My Cow’ from the hints given below:
Hints : Love pets …………. my pet …………. white cow …………. big body …………. four legs …………. two horns …………. long tail …………. four teats …………. gives us milk …………. useful …………. like very much.

3. MY COW
Or
MY PET

I love pets. My pet is a cow. It is a white cow. It has a big body. It has four legs. It has two horns. It has a long tail. It has four teats. It eats grass. It gives us milk. Its milk is sweet and useful. My cow is very gentle. I like it very much.

4. Develop a paragraph on ‘My Horse’ from the hints given below:
Hints: Horse …………. pet animal …………. runs fast …………. pulls tongas …………. in army …………. my horse …………. brown …………. big body …………. bushy tail …………. grass …………. rides …………. very faithful …………. like …………. .

4. THE/MY HORSE

The horse is a pet animal. It is very useful. It runs very fast. It pulls tongas, It is used in the army. I, too, have a horse. It is a brown horse. It has a big body. It has four legs. It has a bushy tail. It eats grain and grass. It gives us rides. My horse is very faithful. I like it very much.

5. Develop a paragraph on ‘Our School from the hints given below:
Hints : S.D. Secondary School …………. outside the city …………. a big school …………. thirty rooms …………. a playground …………. beautiful garden …………. the best school …………. proud of my school.

5. OUR SCHOOL

I read in the S.D. Secondary School. It is outside the city. It is a big school. It has thirty rooms. It has a big hall. It has a playground. It has a beautiful garden. Mr. S.P. Singh/Ms. Karamjeet Kaur is our Principal. Our school is the best school in the city. I am proud of my school.

6. Develop a paragraph on ‘My Best Friend’ from the hints given below:
Hints : Ravi …………. my class-fellow …………. years old …………. a doctor …………. teacher gets up …………. a walk …………. good student …………. obeys his teachers …………. like very much.

6. MY BEST FRIEND

Ravi is my best friend. He is my class-fellow. He is eleven years old. He is very handsome. His father is a doctor. His mother is a teacher. He gets up early. He goes out for a walk. He is a good student. He is a good player. He is a fine singer. He is very hard-working. He obeys his teachers. All the teachers like him. I also like him very much.

7. Develop a paragraph on ‘My Bicycle’ from the hints given below:
Hints : I have …………. Hero bicycle …………. fancy basket …………. a musical bell …………. brakes powerful …………. best companion …………. shopping on it …………. a high speed …………. proud of my bicycle.

7. MY BICYCLE

I have a red bicycle. Its make is Hero. There is a fancy basket on its handle. It has a mirror and a musical bell. Its brakes are very powerful. The bicycle at once stops when I apply them. My bicycle is my best companion. I go to school on it. I go for shopping on it. I always keep it neat and clean. It runs at a high speed. I feel like flying on slopes (CMF). I have won many cycle races with it. I am proud of my bicycle.

8. Develop a paragraph on ‘A Clock’ from the hints given below:
Hints : …………. a useful device …………. the time …………. on the wall …………. very costly …………. digits golden Roman …………. shine …………. help to me — active day and night …………. like it.

8. A CLOCK

A clock is a useful device. It tells us the time. It is hung on the wall. I have a big clock. Its make is H.M.T. It is very costly. It has a silver dial. Its digits are golden Roman. Its hands too have golden lining in them. They shine in the dark. My clock is a great help to me. It keeps me regular and punctual. I am never late for school. My clock is active day and night. I wish to be active like it.

B. APPLICATIONS

1. Application For Sick Leave
Write an application to the Headmaster of your school for sick leave.

The Headmaster
D.A.V. High School
Gurdaspur
Sir

I beg to say that I am ill. I cannot come to school. Kindly grant me leave for two days.
Thanking you

Yours obediently
Ram Singh
VA
March 2, 20…….

2. Application For Leave For An Urgent Piece of Work
Write an application to the Principal of your school for leave for an urgent piece of work.

The Principal
Govt. Sr. Sec. School
Kartarpur
Sir

I have an urgent piece of work (feat chall) at home. So I cannot come to school. Kindly grant me leave for one day.
Thanking you

Yours obediently
Mohan Singh
VD
March 2, 20…….

3. Application For Marriage Leave
Write an application to the Principal of your school for marriage leave.

The Principal
Govt. Senior Secondary School
Jalandhar City
Madam

I beg to say that the marriage of my elder brother takes place next week. So I cannot come to school. Kindly grant me leave for four days. Thanking you

Yours obediently
Geeta
VD
March 4, 20…….

4. Application For Full Fee Concession
Write an application to the Headmaster of your school for full fee concession.

The Headmaster
Government High School
Talwara
Sir

I beg to say that I am a student of V A. My father is a poor man. He cannot pay my school fee. I am a good student. I always get good marks. Kindly grant me full fee concession.
Thanking you

Yours obediently
Manoj
VA
March 8, 20…..

C. NOTICES

ਨੋਟਿਸ (Notice) ਕਿਸੇ ਘਟਣ ਵਾਲੀ ਜਾਂ ਘੱਟ ਚੁੱਕੀ ਘਟਣਾ ਦੇ ਬਾਰੇ ਵਿੱਚ ਸੂਚਨਾ ਹੈ । ਇਸ ਦੁਆਰਾ ਕਿਸੇ ਆਦੇਸ਼, ਪ੍ਰਾਰਥਨਾ ਜਾਂ ਚੇਤਾਵਨੀ ‘ਤੇ ਅਮਲ ਕਰਨ ਦੀ ਸੂਚਨਾ ਦਿੱਤੀ ਜਾਂਦੀ ਹੈ । ਯਾਦ ਰੱਖਣ ਯੋਗ ਗੱਲਾਂ

1. ਨੋਟਿਸ ਦੀ ਭਾਸ਼ਾ ਉਦੇਸ਼ਪੁਰਵਕ ਹੋਣੀ ਚਾਹੀਦੀ ਹੈ । ਇਸ ਵਿਚ ਨਿੱਜੀਪਨ ਨਹੀਂ ਹੋਣਾ ਚਾਹੀਦਾ ।
2. ਦਿੱਤੀ ਜਾਣ ਵਾਲੀ ਸੂਚਨਾ ਪੁਰੀ ਹੋਣੀ ਚਾਹੀਦੀ ਹੈ ।
3. ਇਸ ਦਾ ਪ੍ਰਸਤੁਤੀਕਰਨ ਸੰਖੇਪ ਅਤੇ ਸਪਸ਼ਟ ਹੋਣਾ ਚਾਹੀਦਾ ਹੈ । ਨੋਟਿਸ ਦਾ ਉਦੇਸ਼ ਸਪਸ਼ਟ ਹੋਵੇ ।
4. ਜਿੱਥੇ ਤਕ ਹੋ ਸਕੇ ਨੋਟਿਸ ਵਿਚ ‘T’ ਅਤੇ ‘You’ ਦੇ ਪ੍ਰਯੋਗ ਤੋਂ ਬਚੋ ।
5. ਨੋਟਿਸ ਲਿਖੇ ਜਾਣ ਦੀ ਤਾਰੀਖ ਦਾ ਉਲੇਖ ਜ਼ਰੂਰ ਕਰੋ ।
6. ਵਿਸ਼ਾ-ਵਸਤੂ ਨਾਲ ਸੰਬੰਧਿਤ ਸਥਾਨ, ਸਮਾਂ ਅਤੇ ਕਾਰਜਕ੍ਰਮ ਦੀ ਸਪਸ਼ਟ ਜਾਣਕਾਰੀ ਦਿਓ । . 
7. ਨੋਟਿਸ ਜਾਰੀ ਕਰਨ ਵਾਲੇ ਵਿਅਕਤੀ ਦੇ ਅਹੁਦੇ ਦੇ ਨਾਂ ਅਤੇ ਉਸ ਦੇ ਹਸਤਾਖ਼ਰ ਨੋਟਿਸ ਵਿੱਚ ਹੋਣੇ ਚਾਹੀਦੇ ਹਨ ।
8. ਨੋਟਿਸ ਦਿੱਤੀ ਗਈ ਸ਼ਬਦ-ਸੀਮਾ ਵਿਚ ਹੀ ਲਿਖਣਾ ਚਾਹੀਦਾ ਹੈ ।

नोटिस (Notice) किसी घटित होने वाली या घटित हो चुकी घटना के बारे में सूचना होती है। इसके द्वारा किसी आदेश, प्रार्थना अथवा चेतावनी पर अमल करने की सूचना दी जाती है।

याद रखने योग्य बातें-
1. नोटिस की भाषा उद्देश्यपूरक होनी चाहिए। इसमें निजीपन नहीं होना चाहिए।
2. दी जाने वाली सूचना पूर्ण होनी चाहिए।
3. इसका प्रस्तुतीकरण संक्षिप्त और स्पष्ट होना चाहिए। नोटिस का उद्देश्य भी स्पष्ट हो।
4. जहां तक हो सके नोटिस में ‘I’ तथा ‘You’ के प्रयोग से बचें। 5. नोटिस लिखे जाने की तिथि का उल्लेख अवश्य करें।
6. विषय-वस्तु से सम्बन्धित स्थान, समय तथा कार्यक्रम आदि की स्पष्ट जानकारी दें।
7. नोटिस जारी करने वाले व्यक्ति के पद का नाम तथा उसके हस्ताक्षर भी नोटिस में होने चाहिएं।
8. नोटिस दी गई शब्द-सीमा में ही लिखना चाहिए।

Format of a Notice

NOTICE

HEADING / TITLE

DATE

CONTENT

SIGNATORY

Important Notices

1. Notice About Something Found
You have found a purse lying in one of the lawns of your school. Write a notice asking the owner of the purse to contact you.

NOTICE
March 7, 20…..
FOUND ! FOUND ! FOUND !

This is to inform all students that a purse has been found lying in! one of the school lawns. It is a black leather purse containing some money. The owner should contact the undersigned.

Gulshan Rai
Roll No. 10,
VA

2. Notice for a Lost Wrist Watch

You have lost your wrist watch in your school. Write a notice about the loss giving the particulars of the watch. Also announce a reward for the finder.

NOTICE
LOST ! LOST ! LOST !
April 10, 20….

This is to inform all students that I lost my wrist watch yesterday in 1 the school during the recess period. It is an H.M.T. watch with a golden case and a golden chain. He/She who happens to find it should contact the undersigned immediately. He (She) will be suitably i rewarded.

Aastha Jindal
Roll No. 2,
VD

3. Notice about a Lost Pen
You have lost your pen somewhere in your school. Write a notice about it.

NOTICE
LOST ! LOST ! LOST !
12 March, 20…..

A new gel pen has been lost somewhere in the school ground. The pen is of Reynold make with blue colour. The finder is requested || to return it to the undersigned or deposit it with the school office.

I Kulbir Singh
Roll No. 2
VB

4. Books for Sale
Ashok Mathur of Class V A has just passed his annual examination. Two of his books are in fairly good condition and he wants to sell them at reduced prices. He puts up a notice on the school notice-board giving all the necessary details. Write this notice in the space below, using not more than 50 words.

NOTICE
BOOKS FOR SALE
March 22, 20…..

Two books for class IV fairly in good condition are for sale. The books and their reduced prices are given below:
1. Social Science Rs 30/- only
2. English Reader Rs 20/- only
Those who are interested should contact the undersigned.

Ashok Mathur
Roll No. 3.
VA

Punjab State Board PSEB 5th Class English Book Solutions English Grammar General Topics Exercise Questions and Answers, Notes.

PSEB 5th Class English Grammar General Topics

1. Change Of Number

2. Change Of Gender

3. Degrees of Adjectives

4. The Articles

A, An तथा The को Articles कहा जाता है। A का प्रयोग व्यंजन (consonant) की आवाज़ से शुरू होने वाले एकवचन noun से पहले तथा An का प्रयोग स्वर (vowel) की आवाज़ से शुरू होने वाले एकवचन noun से पहले किया जाता है। The का प्रयोग विशेष वस्तुओं के नामों से पहले होता है।

The को निश्चित उपपदं (definite article) कहा जाता है। A, an को अनिश्चित उपपद (indefinite articles) कहा जाता है।

Filling the Blanks with Articles:

1. He has …………… apple. (an)
2. Mr. Ram Lai is …………… doctor. (a)
3. I am …………… best player of football. (the)
4. Always speak …………… truth. (the)
5. He gave me …………… onion. (an)
6. …………… moon is bright. (The)
7. He rode …………… elephant. (an)
8. Balbir is …………… honest man. (an)
9. I want …………… inkpot. (an)
10. Sita is …………… most beautiful girl of our class. (the)
11. June is …………… hottest month. (the)
12. …………… Sutlej is the main river of the Punjab. (The)
13. I was there for …………… hour. (an)
14. I live in …………… village. (a)
15. The boys are making …………… noise. (a)
16. …………… owl cannot see during daytime. (An)
17. …………… sun shines brightly. (The)
18. …………… earth moves round the sun. (The)
19. …………… sky is blue. (The)
20. I have …………… new pen. (a)
21. She has …………… old pen. (an)
22. …………… Ganga is a holy river. (The)

5. Three Forms of the Verb

Present – Past – Past Participle
Add – added – added
Ask – asked – asked
Be (is, am, are) – was (were) – been
Become – became – become
Begin – began – begun
Break – broke – broken
Bring – brought – brought
Build – built – built
Buy – bought – bought
Catch – caught – caught
Call – called – called
Come – came – come
Cut – cut – cut
Drink – drank – drunk
Do – did – done
Eat – ate – eaten
Fly – flew – flown
Fall – fell – fallen
Find – found – found
Go – went – gone
Get – got – got
Give – gave – given
Help – helped – helped
Jump – jumped – jumped
Keep – kept – kept
Laugh – laughed – laughed
Look – looked – looked

Like – liked – liked
Make – made – made
Obey – obeyed – obeyed
Open – opened – opened
Play – played – played
Reach – reached – reached
Ring – rang – rung
Run- ran – run
See – saw – seen
Sell – sold – sold
Send – sent – sent
Stop – stopped – stopped
Sleep – slept – slept
Sit – sat – sat
Speak – spoke – spoken
Sing – sang – sung
Stand – stood – stood
Think – thought – thought
Take – took – taken
Tear – tore – torn
Tell – told – told
Teach – taught – taught
Wash – washed – washed
Work – worked – worked

Punjab State Board PSEB 5th Class English Book Solutions English Grammar Important Questions Exercise Questions and Answers, Notes.

PSEB 5th Class English Grammar Important Questions

Examination Style Questions (Based on Sample Paper)

Read the following questions and choose correct option for your answer.

I. Question 1.
Tick the meaning of the word ‘popular’.
(a) great
(b) famous
(c) rich
(d) good
Answer:
(b) famous.

Question 2.
Tick the meaning of the word ‘ordinary’.
(a) orderly
(b) normal
(c) bravely
(d) wonderful
Answer:
(b) normal.

Question 3.
Tick the meaning of word ‘Wonderful’.
(a) ordinary
(b) complex
(c) fearful
(d) splendid.
Answer:
(d) splendid.

Question 4.
Tick the meaning of the word ‘Odour’
(a) order
(b) smell
(c) a door
(d) ordinary.
Answer:
(b) smell.

Question 5.
Tick the meaning of the word ‘Swift’.
(a) fast
(b) drift
(c) rift
(d) slow.
Answer:
(a) fast.

Question 6.
Tick the meaning of the word ‘Curious’.
(a) careless
(b) eager
(c) careful
(d) serious.
Answer:
(b) eager.

II. Question 1.
Pick the word with correct spellings.
(a) problum
(b) prblam
(c) problem
(d) probleme.
Answer:
(c) problem.

Question 2.
Pick the word with correct spellings.
(a) posibal
(b) possibel
(c) possible
(d) posible.
Answer:
(c) possible.

Question 3.
Pick the word with correct spellings.
(a) nation
(b) nassion
(c) nashion
(d) nateion.
Answer:
(a) nation.

Question 4.
Pick the word with correct spellings.
(a) tortiose
(b) tortoise
(c) tortoise
(d) tortoies.
Answer:
(b) tortoise.

Question 5.
Pick the word with correct spellings.
(a) receive
(b) recieve
(c) receev
(d) riceive.
Answer:
(b) recieve.

Question 6.
Pick the word with correct spellings.
(a) gloori
(b) glory
(c) glowry
(d) galory.
Answer:
(b) glory.

III. Question 1.
Read carefully and fill in the blank with the correct option.

Mother is …………..
(a) afraid
(b) angry
(c) happy
(d) Worried
Answer:
(c) happy.

Question 2.
Read carefully and fill in the blank with the correct option.

The dog is …………..
(a) helpful
(b) sad
(c) angry
(d) playful.
Answer:
(d) playful.

Question 3.
Read carefully and fill in the blank with the correct option.

Mother is ……….
(a) fearful
(b) careful.
(c) harmful
(b) Beautiful
Answer:
(b) careful.

Question 4.
Read carefully and fill in the blank with the correct option.

Father is …………..
(a) selfish
(b) careful
(c) careless
(d) caring.
Answer:
(d) caring.

Question 5.
Read carefully and fill in the blank with the correct option.

The boy is ………….
(a) sad
(b) worried
(c) angry
(d) cheerful.
Answer:
(a) sad.

Question 6.
Read carefully and fill in the blank with the correct option.

Mother is …………
(a) delighted
(b) worried
(c) angry
(d) all these.
Answer:
(b) worried.

IV. Question 1.
Choose the correct option for the given riddle.
I’m just like you. Wherever you go, I go with you.
(a) mother
(b) air
(c) shadow
(d) sunlight.
Answer:
(c) shadow.

Question 2.
Choose the correct option for the given riddle.
You see me with a bowl at the temple door. I ask for money as i am poor.
(a) priest
(b) beggar
(c) policeman
(d) devotee.
Answer:
(b) beggar

Question 3.
Choose the correct option for the given riddle.
I fly with land and passengers very very high in the sky.
(a) bird
(b) kite
(c) aeroplane
(d) balloon.
Answer:
(c) aeroplane.

Question 4.
Choose the correct option for the given riddle.
I have a face and two hands but no arms or legs.
(a) owl
(b) clock
(c) zebra
(d) map.
Answer:
(b) clock.

Question 5.
Choose the correct option for the given riddle.
I have a neck but no head ?
(a) glass
(b) umbrella
(c) mirror
(d) bottle.
Answer:
(d) bottle.

Question 6.
Choose the correct option for the given riddle.
Something that belongs to you but is used more by others.
(а) your books
(b) your name
(c) your pen
(d) your parents.
Answer:
(b) your name

Question 7.
Choose the correct option for the given riddle.
You will buy me to eat but never eat me. What am I ?
(a) plate
(b) egg
(c) toffee
(d) chocolate.
Answer:
(a) plate.

V. Question 1.
Encircle the name of the dress hidden in the grid.

(a) shirt
(b) socks
(c) coat
(d) vest.
Answer:
(c) coat.

Question 2.
En cirlce the name of the wild animal hidden in the grid.

(a) bear
(b) lion
(c) tiger
(d) zebra.
Answer:
(b) lion.

Question 3.
Encircle the name of the pet animal hidden in the grid.

(a) camel
(b) sheep
(c) cow
(d) goat.
Answer:
(c) cow.

Question 4.
Encircle the name of the bird hidden in the grid.

(a) crow
(b) pigeon
(c) sparrow
(d) parrot.
Answer:
(a) crow.

Question 5.
Encircle the name of the fruit hidden in the grid.

(a) banana
(b) orange
(c) mango
(d) apple.
Answer:
(c) mango.

Question 6.
Encircle the name of the sport hidden in grid.

(a) cricket
(b) hockey
(c) badminton
(d) football.
Answer:
(b) hockey.

VI. Question 1.
Read the advertisement and answer the question.
Book Fair
From 24 May to 26 May
Friday to Sunday
Ticket – Adult Rs 50
Children Rs 20

On which day will the book fair end ?
(a) Friday
(b) thursday
(c) Saturday
(d) Sunday.
Answer:
(d) Sunday.

Question 2.
Read the advertisment and answer the question.

On which day will the trade fair start ?
(a) Monday
(b) Wednesday
(c) Tuesday
(d) Sunday.
Answer:
(a) Monday.

Question 3.
Read the advertisment and answer the question.

On which day will the trade fair end ?
(a) Sunday
(b) Monday
(c) Friday
(d) Wednesday.
Answer:
(d) Wednesday.

Question 4.
Read the advertisment and answer the question.

How much does a ticket for an adult cost ?
(a) Rs. 50
(b) Rs. 60
(c) Rs. 100
(d) Rs. 40
Answer:
(c) Rs. 100.

Question 5.
Read the advertisment and answer the question.

On which day will the book fair start ?
(a) Friday
(b) Tuesday
(c) Saturday
(d) Sunday.
Answer:
(b) Tuesday.

Question 6.

What is the duration of the fair.
(a) 15-18 April
(b) 16-18 April
(c) 18-15 April
(d) 8-10 April
Answer:
(a) 15-18 April.

VII. Question 1.
Which of the following is a Proper Noun ?
(a) animal
(b) flower
(c) India
(d) colour.
Answer:
(c) India

Question 2.
Which of the following is a Proper Noun ?
(a) district
(b) Amritsar
(c) city
(d) village
Answer:
(b) Amritsar.

Question 3.
Which of the following is a Common Noun ?
(a) clock
(b) Monday
(c) Delhi
(d) Jaspreet
Answer:
(a) clock.

Question 4.
Which of the following not is not a common noun ?
(a) train
(b) room
(c) teacher
(d) Delhi.
Answer:
(d) Delhi.

Question 5.
Which of the following is a Proper Noun ?
(a) peacock
(b) Nepal
(c) country
(d) uncle.
Answer:
(b) Nepal.

Question 6.
Which of the following is not a Proper Noun ?
(a) rose
(b) flower
(c) marigold
(d) Lily.
Answer:
(b) flower.

VIII. Question 1.
Pala said to Beero, “Dear ! Look at the buildings. How tall they are !” For whom is the underlined word used ?
(а) Pala
(b) buildings
(c) Beero
(d) both Pala and Beero
Answer:
(b) Buildings.

Question 2.
In the evening he found a wonderful pair of shoes on his table.
For whom is the underlined word used for ?
(a) evening
(b) table
(c) pair of shoes
(d) he
Answer:
(c) pair of shoes.

Question 3.
The women said, “Those little men have made us rich.”
For whom is the underlined word used for ?
(a) women
(b) men
(c) rich people
(d) both men and women.
Answer:
(b) men.

Question 4.
Beero said to Pala, “You need to look around you. People have beautiful homes but they are not using them properly. For whom is the underlined word used for ?
(a) Beero and Pala
(b) people
(c) homes
(d) both homes and people.
Answer:
(c) homes.

Question 5.
Beero responded, “They are not using their toilets. They defecate in the open, near my house.” Which is the proper place for underlined.
(a) open
(b) toilets
(c) Beero’s house
(d) all the above.
Answer:
(b) toilets.

Question 6.
The Emperor said, “You have to make this line shorter but you should not touch it.”
For Whom is the underlined word used for ?
(a) courtiers
(b) emperor
(c) floor
(d) lines
Answer:
(d) line.

IX. Question 1.
Whichzof the following can be described, using the word ‘tall’ ?
(a) mountain
(b) rope
(c) man
(d) pencil.
Answer:
(c) man.

Question 2.
Which of the following can be described using the word ‘short’ ?
(a) mountain
(b) house
(c) school
(d) man.
Answer:
(d) man.

Question 3.
Which of the following can be described using the word long ?
(a) horse
(b) river
(c) building
(d) none of these.
Answer:
(b) nvers.

Question 4.
Which of the following can’t be described using the word ugly ?
(a) man
(b) girl
(c) thing
(d) bread
Answer:
(d) bread

Question 5.
Which of the following can be described using the word wise ?
(a) boy
(b) minister
(c) sport
(d) lady.
Answer:
(c) sport.

Question 6.
Which of the following can be described using the word ‘great’ ?
(a) Zoo
(b) Circle
(c) Elephant
(d) Personality.
Answer:
(d) Personality.

X. Question 1.
Fill in the blank, using the correct option.
He …………. here daily.
(a) come
(b) coming
(c) comes
(d) coming.
Answer:
(c) comes.

Question 2.
Fill in the blank using the correct option.
He always …………… up before sunrise.
(a) get
(b) gets
(c) gotten
(d) getting.
Answer:
(b) gets.

Question 3.
Fill in the blank using the correct option.
He ……………. a fine speech yesterday.
(a) makes
(b) make
(c) made
(d) maked.
Answer:
(c) made.

Question 4.
Fill in the blank using the correct option.
Sita …………… a flower and went home.
(a) pluck
(b) plucked
(c) plucking
(d) will pluck.
Answer:
(b) plucked.

Question 5.
Fill in the blank using the correct option.
We shall ……………. school tomorrow.
(a) go
(b) gone
(c) went
(d) going.
Answer:
(a) go.

Question 6.
Fill in the blank using the correct option.
Birds ………….. in the sky.
(a) fly
(b) flyes
(c) flies
(d) flying.
Answer:
(a) fly

XI. Question 1.
Choose the correct statement.
(a) boys is making a noise.
(b) boy were making a noise.
(c) boys was making a noise.
(d) boys were making a noise.
Answer:
(d) boys were making a noise.

Question 2.
Choose the correct statement.
(a) the girls is making a doll.
(b) the girls are making a doll.
(c) the girls was making a doll.
(d) the girl were making a doll.
Answer:
(b) the girls are making a doll.

Question 3.
Choose the correct statement.
(a) we are playing a match.
(b) we is playing a match.
(c) we was playing a match.
(d) we am playing a match.
Answer:
(a) we are playing a match.

Question 4.
Choose the correct statement.
(a) the students is running a race.
(b) the student are running a race.
(c) the students was running a race.
(d) the students were running a race.
Answer:
(d) the students were running a race.

Question 5.
Choose the correct statement.
(a) my mother is always active.
(b) my mother are always active.
(c) my mother were always active.
(d) none of these.
Answer:
(a) my mother is always active.

Question 6.
Choose the correct statement.
(a) Sonu is a new bike.
(b) Sonu have a new bike.
(c) Sonu has a new bike.
(d) Sonu was a new bike.
Answer:
(c) Sonu has a new bike.

XII. Question 1.
Choose the word having silent letter.
(a) read
(b) dance
(c) talk
(d) run.
Answer:
(c) talk.

Question 2.
Choose the word having silent letter.
(а) take
(b) walk
(c) work
(d) dance.
Answer:
(b) walk.

Question 3.
Choose the word having silent letter.
(a) knife
(b) life
(c) dance
(d) blade.
Answer:
(a) knife.

Question 4.
Choose the word having silent letter.
(а) post
(b) knock
(c) road
(d) mango.
Answer:
(b) knock.

Question 5.
Choose the the word having silent letter.
(a) exxpert
(b) Teacher
(c) student
(d) witch.
Answer:
(d) witch.

Question 6.
Choose the word having silent letter.
(а) speak
(b) listen
(c) read
(d) work
Answer:
(b) listen.

XIII. Question 1.
Choose the odd one out.
(a) cow
(b) crow
(c) fox
(d) wolf
Answer:
(b) crow.

Question 2.
Choose the odd one out.
(a) wolf
(b) parrot
(c) crow
(d) pigeon
Answer:
(a) wolf.

Question 3.
Choose the odd one out.
(a) holi
(b) republic day.
(c) diwali
(d) eid
Answer:
(b) republic day.

Question 4.
Choose the odd one out.
(a) goat
(b) owl.
(c) sheep
(d) cow.
Answer:
(b) owl.

Question 5.
Choose the odd one out.
(a) fruit
(b) leaves
(c) branch
(d) bank
Answer:
(d) bank.

Question 6.
Choose the odd one out.
(a) mouse
(b) monitor
(c) locker
(d) keyboard
Answer:
(c) locker.

XIV. Question 1.
Fill in the blank, using the correct option.
She will distribute sweets ………….her classmates.
(a) with
(b) among
(c) between
(d) in.
Answer:
(b) among.

Question 2.
Fill in the blank using correct option.
My uncle knocked …………. the door.
(a) out
(b) from
(c) at
(d) on.
Answer:
(c) at

Question 3.
Fill in the blank using correct option.
I do not agree ………… you.
(a) to
(b) by
(c) from
(d) with.
Answer:
(d) with.

Question 4.
Fill in the blank using correct option.
We will see you …………. Monday.
(a) on
(b) at.
(c) for
(d) with.
Ans.
Answer:
(a) on

Question 5.
Fill in the blank using correct option.
He was absent ……….. its school.
(a) out
(b) from
(c) at
(d) in.
Answer:
(b) from.

Question 6.
Fill in the blank using correct option.
Jaspreet is taller ……….. Rajan.
(a) from
(b) by
(c) than
(d) then
Answer:
(c) than

XV. Question 1.
Choose the verb.
(a) wash
(b) afraid
(c) fine
(d) dirty.
Answer:
(a) wash.

Question 2.
Choose the verb.
(a) young
(b) good
(c) buy
(d) tall.
Answer:
(c) buy.

Question 3.
Choose the verb.
(a) now
(b) already
(c) long
(d) talk.
Answer:
(d) talk.

Question 4.
Choose the verb.
(a) gift
(b) give
(c) before
(d) present.
Answer:
(b) give.

Question 5.
Choose the verb.
(a) happy
(b) sorry
(c) Pretty
(d) sing.
Answer:
(d) sing

Question 6.
Chooe the verb.
(a) catch
(b) red
(c) beg
(d) weak.
Answer:
(a) catch

XVI. Question 1.
Tick the correct statement.
(a) They going now home are.
(b) They are now home going.
(c) They are going home now.
(d) They are going now home.
Answer:
(c) They are going home now.

Question 2.
Tick the correct statement.
(a) Brightly lamp is burning.
(b) lamp brightly is burning.
(c) lamp is burning bright.
(d) lamp is burning brightly.
Answer:
(d) Lamp is burning brightly.

Question 3.
Tick the correct statement.
(a) He has finished already his work.
(b) He already his work has finished.
(c) He has already finished his work.
(d) Already has he finished his work
Answer:
(c) He has already finished his work.

Question 4.
Tick the correct statement.
(a) Meenu is my best friend.
(b) Meenu is my better friend.
(c) Meenu is good my friend.
(d) Meen is best my friend.
Answer:
(a) Meenu is my best friend.

Question 5.
Tick the correct statement.
(a) I then reading a newspaper.
(b) I was then a newspaper reading.
(c) I was newspaper reading then.
(d) I was reading a newspaper then.
Answer:
(d) I was reading a newspaper then

Question 6.
Tick the correct statement.
(a) They very poor become.
(b) They had become very poor.
(c) They very poor had become.
(d) They had became very poor.
Answer:
(b) They had become very poor.

Punjab State Board PSEB 11th Class English Book Solutions English Grammar Transformation of Sentences & Removal and Use of ‘Too’ Exercise Questions and Answers, Notes.

PSEB 11th Class English Grammar Transformation of Sentences & Removal and Use of ‘Too’

A. Transformation of Sentences

1. Assertive sentences : ये वाक्य किसी बात, तथ्य अथवा घटना का वर्णन करते हैं। ये वाक्य तीन प्रकार के हो सकते हैं-
(i) Affirmative

• Mohan wrote a letter.
• I helped my friends.
• She hopes to pass this year.
• Mohan has two pens.

(ii) Negative

• Mohan did not write a letter.
• I did not help my friends.
• She does not hope to pass this year.
• Mohan has no pen.

(iii) Emphatic

• Mohan did write a letter.
• I did help my friends.
• She does hope to pass this year.
• You do tell lies.

2. Interrogative sentences : इस प्रकार के वाक्यों में कोई प्रश्न पूछा जाता है। इन के अन्त में प्रश्न-वाचक चिन्ह (?) – Question Mark – लगाया जाता है।

• What is your father ?
• Why are the boys making a noise ?
• Are you not happy here?
• Did the teacher punish him ?

3. Imperative sentences : इस प्रकार के वाक्यों में किसी आदेश, आज्ञा, उपदेश, प्रार्थना, आदि को व्यक्त किया जाता है। इन वाक्यों में कर्ता शब्द (You) का प्रयोग नहीं किया जाता है।

• Bring a glass of water, please.
• Tell him to leave the room at once.
• Switch on the lights.
• March forward.

4. Exclamatory sentences : इस प्रकार के वाक्यों में किसी अकस्मात शोक, हर्ष, हैरानी, घृणा, आदि के भाव को व्यक्त किया जाता है। इनके अन्त में (अथवा विस्मय-बोधक शब्द के अन्त में) विस्मय-वाचक चिन्ह (!) – Mark of Exclamation – लगाया जाता है।

• Hurrah ! we have won.
• Alas ! he is ruined.
• What a lovely flower (it is) !
• How foolish he is !

5. Optative sentences : इस प्रकार के वाक्य किसी इच्छा अथवा प्रार्थना को प्रकट करते हैं। इनके अन्त में विस्मय-वाचक चिन्ह (!) – Mark of Exclamation – लगाया जाता है।

• May you succeed in life !
• O that she were alive!
• Would that I were young again !
• If only I could win the first prize !

नोट : परीक्षा-पत्र में प्राय: Assertive वाक्यों को ही Negative या Interrogative रूप में बदलने के लिए कहा जाता है। इस सम्बन्ध में निम्नलिखित नियम याद रखिए।

1. V₁ वाले वाक्यों को Negative या Interrogative में बदलने के लिए helping verb के रूप में do का प्रयोग किया जाता है।

2. V₁ + s / es वाले‌ ‌वाक्यों‌ ‌को‌ ‌Negative‌ ‌या‌ ‌Interrogative‌ ‌बनाने‌ ‌के‌ ‌लिए‌ ‌helping‌ ‌ verb‌ ‌के‌ ‌रूप‌ ‌में‌ ‌does‌ ‌का‌ ‌प्रयोग‌ ‌किया‌ ‌जाता‌ ‌है।‌ ‌यह‌ ‌बात‌ ‌ध्यान‌ ‌रखने‌ ‌योग्य‌ ‌है‌ ‌कि‌ ‌does‌ ‌लगाने‌ ‌के‌ ‌बाद‌ ‌V₁‌ ‌ के‌ ‌साथ‌ ‌लगा‌ ‌हुआ‌ ‌s‌ ‌/‌ ‌es‌ ‌हटा‌ ‌दिया‌ ‌जाता‌ ‌है।‌

3. V₂ वाले वाक्यों को Negative या Interrogative बनाने के लिए helping verb के रूप में did का प्रयोग किया जाता है। यह बात ध्यान रखने योग्य है कि did लगाने के बाद V₂ के स्थान पर V₁ का प्रयोग किया जाता है।

4. Interrogative वाक्यों में helping verb का प्रयोग वाक्य के आरम्भ में किया जाता है।

Transformation of Sentences

Affirmative to Negative

Affirmative – Negative
1. He was the most loved boy. – He was not the most loved boy.
2. The teacher was present. – The teacher was not present.
3. He killed the snake. – He did not kill the snake.
4. He does his duty. – He does not do his duty.
5. I am coming to your house. – I am not coming to your house.
6. The sun sets at 6 p.m. – The sun does not set at 6 p.m.
7. He is writing a letter. – He is not writing a letter.
8. I can solve this sum. – I cannot solve this sum.
9. The moon rose at 10 o’clock. – The moon did not rise at 10 o’clock.
10. It may rain today. – It may not rain today.
11. He is my friend. – He is not my friend.
12. You are a lucky man. – You are not a lucky man.

II. Assertive to Interrogative

Assertive – Interrogative
1. They laughed at us. – Did they laugh at us?
2. She is beautiful. – Is she beautiful ?
3. He does his duty. – Does he do his duty ?
4. He can help you. – Can he help you ?
5. We should respect our parents. – Should we respect our parents ?
6. We built a house there. – Did we build a house there?
7. It may rain today. – May it rain today?
8. We must go there. – Must we go there?
9. You are late. – Are you late ?
10. He was happy. – Was he happy ?
11. We shall come soon. – Shall we come soon?

III. Interrogative to Assertive

Interrogative – Assertive
1. Who can tell the future ? – Nobody can tell the future.
2. When can their glory fade ? – Their glory can never fade.
3. Who wants to die? – Nobody wants to die.
4. Who can challenge me? – Nobody can challenge me.
5. Shall I ever forget those happy days. – I shall never forget those happy days?
6. Who does not love his country? – Everybody loves his country.

IV. Exclamatory to Assertive

Exclamatory – Assertive
1. How interesting the story is ! – The story is very interesting.
2. How foolish I am! – I am very foolish.
3. What a lovely rose ! – The rose is very lovely.
4. What a nice book it is ! – It is a very nice book.
5. O that I were young again ! – I wish I were young again.
6. Alas ! I am undone. – It is sad that I am undone.

Change of Tenses in Sentences

अब नीचे दिए गए उदाहरणों का अध्ययन ध्यानपूर्वक कीजिए

Changing into Present Indefinite Tense

1. He spoke the truth. – He speaks the truth.
2. He was reading the newspaper. – He reads the newspaper.
3. The moon rose at 7 o’clock. – The moon rises at 7 o’clock.
4. I did not write a letter. – I do not write a letter.

Changing into Past Indefinite Tense

1. Mohan buys a beautiful pen. – Mohan bought a beautiful pen.
2. I go to see the fair. – I went to see the fair.
3. My brother is preparing tea. – My brother prepared tea.
4. She does her duty well. – She did her duty well.

Changing into Future Indefinite Tense

1. I gave him ten rupees. – I shall give him ten rupees.
2. I am doing it honestly. – I shall do it honestly.
3. I went to see the fair. – I shall go to see the fair.
4. He has been deceiving you. – He will deceive you.

Changing into Present Continuous Tense

1. Was he speaking the truth? – Is he speaking the truth?
2. I have taken my breakfast. – I am taking my breakfast.
3. The old man had died. – The old man is dying.
4. He will go to Delhi. – He is going to Delhi.

Changing into Past Continuous Tense

1. You will not trust him. – You were not trusting him.
2. The teacher called the roll. – The teacher was calling the roll.
3. He speaks the truth. – He was speaking the truth.
4. I took the rope for a snake. – I was taking the rope for a snake.

Changing into Future Continuous Tense

1. The sun rises at six in the the morning. – The sun will be rising at six in morning.
2. I have done the sums. – I shall be doing the sums.
3. You committed the mistake. – You will be committing the mistake.
4. Are you drinking water ? – Will you be drinking water ?

Changing into Perfect Tenses

Present Perfect Tense – Past Perfect Tense – Future Perfect Tense
1. I have done. my work. – I had done my work. – I shall have done my work.
2. He has visited Delhi. – He had visited Delhi. – He will have visited Delhi.

Changing into Perfect Continuous Tenses

Present Perfect Continuous Tense – Past Perfect Continuous Tense – Future Perfect Continuous Tense
1. She has been weeping since morning. – She had been weeping since morning – She will have been weeping since morning.
2. It has been raining for the last 4 days. – It had been raining for the last 4 days. – It will have been raining for the last 4 days.

Exercise

Change the following sentences as directed.
1. He does not run very fast. (Positive)
2. Sohan plays hockey. (Future Perfect Tense)
3. Once a thief always a thief. (Negative Interrogative)
4. Who kills the mad dogs ? (Past Perfect)
5. He gets up early in the morning. (Negative)
6. She prefers tea to milk. (Interrogative)
7. His dog wanders all night. (Negative)
8. Ram is taking his meals. (Future Indefinite Tense)
9. Kapil is the best player of cricket. (Interrogative)
10. Where does your brother live ? (Past Continuous)
11. She wrote a beautiful poem. (Negative)
12. I knew him. (Future Indefinite Tense)
13. What a lovely child ! (Assertive)
14. She wrote a letter. (Negative)
15. You told a lie. (Present Continuous Tense)
16. The old man had died. (Present Continuous Tense)
17. He spoke the truth. (Present Indefinite)
18. Who does not love his country? (Assertive)
19. Gandhiji was the greatest leader of India. (Negative)
20. He is my friend. (Negative)
21. I did not write a letter. (Present Indefinite Tense)
22. Who wants to be a slave ? (Assertive)
23. My brother is singing a song. (Negative)
24. He went to Delhi. (Negative)
25. What a beautiful flower ! (Assertive)
26. She sings a beautiful song. (Future Continuous)
27. This river freezes in winter. (Past Indefinite)
28. What a nice book it is ! (Assertive)
29. Did they kill the mad dog? (Negative)
30. He finished his work. (Interrogative)
Answer:
1. He runs very fast.
2. Sohan will have played hockey.
3. Is once a thief not always a thief?
4. Who had killed the mad dogs ?
5. He does not get up early in the morning.
6. Does she prefer tea to milk ?
7. His dog does not wander all night.
8. Ram will take his meals.
9. Is Kapil the best player of cricket?
10. Where was your brother living?
11. She did not write a beautiful poem.
12. I will know him.
13. The child is very lovely.
14. She did not write a letter.
15. You are telling a lie.
16. The old man is dying.
17. He speaks the truth.
18. Everybody loves his country.
19. No other leader of India was so great as Gandhiji.
20. He is not my friend.
21. I do not write a letter.
22. Nobody wants to be a slave.
23. My brother is not singing a song.
24. He did not go to Delhi.
25. The flower is very beautiful.
26. She will be singing abeautiful song.
27. This river froze in winter.
28. It is a very nice book.
29. Did they not kill the mad dog?
30. Did he finish his work ?

B. Interchange of Degrees of Comparison

Interchange of as adjective opt degree of comparison बदलना संभव होता है।
1. जब किसी वस्तु अथवा व्यक्ति की तुलना उसी प्रकार की किसी अन्य वस्तु अथवा व्यक्ति से की जाए तो हम Positive अथवा Comparative Degree का प्रयोग कर सकते हैं।

निम्नलिखित उदाहरणों का अध्ययन करें:

1. He is as dull as an ass. (Positive)
An ass is not duller than he is. (Comparative)
2. She is not so wise as her sister. (Positive)
Her sister is wiser than she is. (Comparative)

(Comparative) उपयुक्त उदाहरणों से यह स्पष्ट है कि Positive Degree का कोई affirmative वाक्य Comparative Degree, में negative बन जाता है तथा इसका विपरीत भी सत्य है।
Affirmative वाक्य में Positive Degree के साथ as …….. as का प्रयोग किया जाता है।
He is as dull as an ass.

Negative वाक्य में Positive Degree के साथ so …….. as का प्रयोग किया जाता है।.
She is not so wise as her sister.

2. जब एक वस्तु अथवा व्यक्ति की तुलना उसी प्रकार की अन्य कई वस्तुओं अथवा व्यक्तियों से करनी हो, तो हम किसी भी degree का प्रयोग कर सकते हैं – Positive, Comparative अथवा Superlative.

Adjective Degree:

Superlative – Comparative – Positive
the best – better than any other – no other …………… So good as
not the best – not better than some others – some ………. at least as good as
one of the best (of) – better than most others – very few …………… so good as
not one of the best – some others better than – not so good as some others.

Exercise

Change the Degree of Comparison without changing the meaning.

I. 1. She is as cunning as a fox.
2. He is as brave as a lion.
3. His skin is as black as coal.
4. Liza is as beautiful as the moon.
5. He runs as fast as a deer.
6. She is not so beautiful as her sister.
7. A bird can’t fly so fast as an aeroplane.
8. Iron is not so heavy as gold.
Answer:
1. 1. A fox is not cleverer than she (is).
2. A lion is not braver than he (is).
3. Coal is not blacker than his skin.
4. The moon is not more beautiful than Liza.
5. A dear does not – run faster than he.
6. Her sister is more beautiful than she (is).
7. An aeroplane can fly faster than a bird.
8. Gold is heavier than iron.

II. 1. Abdul is taller than Hamid.
2. A wise enemy is better than a foolish friend.
3. The younger sister is cleverer than the elder.
4. A live ass is stronger than a dead lion.
5. I work harder than you do.
6. Sham is not more hard-working than Prem.
7. A rose is not fairer than she is.
8. This room is not larger than that one.
Answer:
1. Hamid is not so tall as Abdul.
2. A foolish friend is not so good as a wise enemy.
3. The elder sister is not so clever as the younger.
4. A dead lion is not so strong as a live ass.
5. You do not work so hard as I do.
6. Prem is as hard-working as Sham.
7. She is as fair as a rose.
8. That room is as large as this one.

III. 1. Mumbai is the best seaport in India.
2. Riding is the best kind of exercise.
3. She is the most beautiful girl in our class.
4. Shakespeare was the greatest dramatist of England.
5. Forgiveness is the noblest form of revenge.
6. Ashok is not the best player of our team.
7. Quinine is not the bitterest of all medicines.
8. She is not the wisest of all the girls in our class.
Answer:
1. Mumbai is better than any other seaport in India.
(or)
No other seaport in India is so good as Mumbai.
2. Riding is better than any other exercise.
(or)
No other exercise is so good as riding.
3. She is more beautiful than any other girl in our class.
(or)
No other girl in our class is so beautiful as she.
4. Shakespeare was greater than any other dramatist of England.
(or)
No other dramatist of England was so great as Shakespeare.
5. Forgiveness is nobler than any other form of revenge.
(or)
No other form of revenge is so noble as forgiveness.
6. Ashok is not better than some other players of our team.
(or)
Some other players are at least as good as Ashok.
7. Quinine is not more bitter than some other medicines.
(or)
Some other medicines are at least as bitter as quinine.
8. She is not wiser than some other girls in our class.
(or)
Some other girls in our class are at least as wise as she.

C. Transformation of Simple, Compound & Complex Sentences

1. Converting Simple Sentences into Compound Sentences

Simple sentence को Compound sentence में परिवर्तित करने के लिए वाक्य के किसी शब्द या (Co-ordinate clause) निम्नलिखित उदाहरणों का अध्ययन ध्यानपूर्वक करें:

Simple – Compound

1. He must work very hard to make up for the lost time. – He must work very hard and make up for the lost time.
2. To our great surprise, he betrayed and his own friends. – He betrayed his own friends, this surprised us greatly.
3. Besides robbing the poor man, he also murdered him. – He not only robbed the poor man but also murdered him.
4. He must work very hard to win the first prize. – He must work very hard, or he will not win the first prize.
5. He must not attempt to cheat on pain of punishment. – He must not attempt to cheat, or he will be punished.
6. Owing to ill-luck, he met with a bad accident. – He was unlucky, and met with a bad accident.
7. The teacher punished the boy for disobedience. – The boy was disobedient, and the teacher punished him.

2. Converting Compound Sentences into Simple Sentences

Compound sentence को Simple sentence में परिवर्तित करने के लिए वाक्य के किसी एक Co-ordinate clauses को बदल कर वाक्यांश बना दें।
निम्नलिखित उदाहरणों का अध्ययन ध्यानपूर्वक करें:

Compound – Simple

1. We must eat, or we cannot live. – We must eat to live.
2. He must not be late, or he will be punished. – In the event of his being late, he will be punished.
3. He finished his homework and put away his books. – Having finished his homework, he put away his books.
4. You must either pay the bill at once or return the goods. – Failing immediate payment of the bill, the goods must be returned.
5. He was a mere boy, yet he offered to fight the giant. – In spite of his being a mere boy, he offered to fight the giant.
6. Not only did his father but his mother also give him money.
Besides his father, his mother also gave him money.
7. The men endured all the horrors of the campaign and not one of them complained at all.
The men endured all the horrors of the campaign without any of them making a complaint.

3. Converting Simple Sentences into Complex Sentences

Simple sentence को Complex sentence में परिवर्तित करने के लिए वाक्यांश को विस्तृत करके Subordinate clause ( जोकि कोई Noun, Adjective, या Adverb clause हो सकती है) बना

1. Listen to what the teacher says.
2. The fact is that he knows nothing.
3. He was told that he must not be late again.
4. That John was a thief was not known to me.
5. I was shocked to hear that his only son had died.
6. It is true that he risked his own life to save the child.
7. Learning that my brother had received serious injuries, I left for Shimla.

Adjective Clause वह उपवाक्य हातो है जोकि, किसी अन्य उपवाक्य के किसी शब्द के संबंध में एक विशेषण का कार्य कर रहा होता है। निम्नलिखित Complex Sentences में प्रत्येक वाक्य में रेखांकित शब्द Adjective Clause बनाते हैं।

1. There was none but wept.
2. He is not such a man as can be trusted.
3. The company that supplied goods has failed.
4. The house where my brother lives has been sold.
5. The complaint which he made against me is false.

Adverb Clause वह उपवाक्य हातो है जोकि, किसी अन्य उपवाक्य के किसी शब्द के संबंध में एक क्रिया विशेषण का कार्य कर रहा होता है।
प्रत्येक निम्नलिखित Complex Sentences में रेखांकित शब्द Adverb Clause बनाते हैं। .
1. He talks as if he were mad.
2. I work hard so that I may pass.
3. Where there is a will, there is a way.
4. You should act as the doctor advises you.
5. When the cat is away the mice will play.
6. As far as I know, Ram Lal is not to blame.
7. As you are over twelve, you will have to pay full fare.

निम्नलिखित उदाहरणों का अध्ययन ध्यानपूर्वक करें:

Simple – Complex
1. He confessed his crime. – He confessed that he was guilty.
2. His silence proves his guilt. – The fact that he is silent proves his guilt,
3. He bought his uncle’s house. – He bought the house which belonged to his uncle.
4. On the arrival of the police, the crowd dispersed. – The crowd dispersed as soon as the police arrived.
5. He owed his success to his father. – It was owing to his father that he suceeded.
6. He worked hard to pass the examination. – He worked hard that he might pass the examination.
7. Rahul, being jealous of Amit, struck him. – Rahul struck Amit because he was jealous of him.
8. Only students are admitted. – If you are not a student, you won’t be admitted.
9. The manangement is thoroughly it could be. – The management is as corrupt as corrupt.
10. A man’s modesty is in inverse proportion to his ignorance. – The more ignorant a man is, the less modest he is.

4. Converting Complex Sentences into Simple-Sentences

Complex sentence is Simple sentence किसी वाक्यांश में परिवर्तित कर देते हैं।
निम्नलिखित उदाहरणों का अध्ययन ध्यानपूर्वक करें :

Complex – Simple
1. Tell me where you live. – Tell me your address.
2. How long I shall stay is doubtful. – The duration of my stay is doubtful.
3. He died in the village where he was born. – He died in his native village.
4. The moment which is lost is lost for ever. – A lost moment is lost for ever.
5. Those that are healthy have no need of the physician. – Healthy persons have no need of the physician.
6. We came upon a hut where a peasant lived. – We came upon a peasant’s hut.
7. The exact time when this happened is not certain. – The exact time of its happening is not certain.
8. The place where the Buddha was cremated has been discovered. – The place of the Buddha’s cremation has been discovered.
9. I have no advice that I can offer you. – I have not advice to offer you.
10. You can eat as much as you like. – You can eat to your heart’s content.

5. Converting Compound Sentences into Complex Sentences

निम्नलिखित उदाहरणों का अध्ययन ध्यानपूर्वक करें:

Compound – Complex
1. Search his pockets, and you will find the watch. – If you search his pockets, you will find the watch.
2. Do as I tell you, or you will you have to suffer. – Unless you do as I tell you, will have to suffer.
3. The lion was wounded, but not killed. – The lion was not killed although he was wounded.
4. Waste not, want not. – If you do not waste a thing, you will never feel its want.
5. He saw the danger, but kept on advancing. – Although he saw the danger, he kept on advancing.
6. He saw the danger and paused. – When he saw the danger, he paused.
7. He aimed at winning the prize, and worked hard. – He worked hard so that he might win the prize.
8. He was late, and missed the train. – As he was late, he missed the train.
9. He lives in Cherrapoonji and it rains there almost daily. – He lives in Cherrapoonji where it rains almost daily.
10. He wishes to become learned therefore, he is studying hard. – He is studying hard because he wishes to become learned.

6. Converting Complex Sentences into Compound Sentences

निम्नलिखित उदाहरणों का अध्ययन ध्यानपूर्वक करें:

Complex – Compound
1. I am certain you have made a mistake. – You have made a mistake, and I am certain of this.
2. I am glad that he has recovered from illness. – He has recovered from illness, and this gladdens me.
3. We can prove that the earth is round. – The earth is round, and we can prove this.
4. I have found the book that I had lost. – I had lost a book, but I have found it.
5. As soon as he got the message, he left in a taxi. – He got the message, and immediately he left in a taxi.
6. He worked hard so that he might win the prize. – He aimed at winning the prize and worked hard.
7. If he is at home, I shall see him. – He may be at home, and in that case I shall see him.
8. He lost more than he could afford. – He could not afford to lose so much as he did.
9. He is more a philosopher than a poet. – He is something of a poet, but more than that he is a philosopher.
10. If you do not hurry, you will miss the train. – You must hurry, or you will miss the train.

Exercise

1. Rewrite the following Simple sentences as Compound ones.

1. To avoid punishment, he ran away.
2. He was rejected owing to ill health.
3. Owing to drought, the crop is short.
4. With a great effort, he lifted the box.
5. Notwithstanding several efforts, he failed.
6. Being a cripple, he cannot ride a horse.
7. He must resign on pain of public protest.
8. Being dissatisfied, he resigned his position.
Answer:
1. He wanted to avoid punishment; therefore, he ran away.
2. He was not in good health; therefore, he was rejected.
3. There was drought; therefore, the crop is short.
4. He applied a great effort and lifted the box.
5. He made several efforts, yet he failed.
6. He is a cripple, and therefore, he cannot ride a horse.
7. He must resign, or the public will protest against him.
8. He was dissatisfied, and resigned his position.

2. Rewrite the following Compound sentences as Simple ones.

1. Be good and you will be happy.
2. He is rich, yet he is not contented.
3. Make haste, or else you will be late.
4. He tried hard, but he did not succeed.
5. He did this and so offended his master.
6. I called him, but he gave me no answer.
7. The girls not only sang, but danced also.
8. I have a lot of work and must do it now.
Answer:
1. Being good, you will be happy.
2. In spite of being rich, he is not contented.
3. Without making haste, you will be late.
4. In spite of trying hard, he did not succeed.
5. By doing this, he offended his master.
6. In spite of my calling him, he gave no answer.
7. Besides singing, the girls danced also.
8. Having a lot of work, I must do it now.

3. Convert the following Simple sentences into Complex sentences.

1. Tell the truth.
2. I request your help.
3. He confessed his fault.
4. I wish you to be quiet.
5. I overheard all his remarks.
6. He hoped to win the prize.
7. I expect to meet Rama tonight.
8. He is said to be a millionaire.
Answer:
1. Tell me what the truth is.
2. It is my request that you should help me.
3. He confessed that it was his fault.
4. I wish that you should be quiet.
5. I overheard all that he remarked.
6. He hoped that he would win the prize.
7. I expect that I will (shall / would) meet Rama tonight.
8. It is said that he is a millionaire.

4. Turn each of the following Complex sentences into a Simple sentence.

1. It is time you went.
2. Grant me what I ask.
3. Come when you can.
4. Tell me where you live.
5. Tell me how old you are.
6. Work as hard as you can.
7. I shall remain where I am.
8. He ran as fast as he could.
Answer:
1. It is time for you to go.
2. Grant me my request.
3. Come when possible.
4. Tell me the
place of your residence.
5. Tell me your age.
6. Work hard to the best of your ability.
7. I shall remain in my place.
8. He ran at the highest possible speed.

5. Convert the following Compound sentences into Complex sentences

1. We will win or die.
2. Be just, and fear not.
3. You or I must go away.
4. He is poor, but contented.
5. Listen, and I will tell you all.
6. You called me, and here I am.
7. Be quiet, or I shall punish you.
8. She must weep, or she will die.
Answer:
1. If we don’t win, we will die.
2. If you are just, you will have no fear.
3. If you do not
go away, I must.
4. Though he is poor, he is contented.
5. If you listen, I will tell you all.
6. As you called me, I am here.
7. If you are not quiet, I shall punish you.
8. If she does not weep, she will die.

6. Convert the following Complex sentences into Compound sentences.

1. He fell as I fired.
2. Come when you like.
3. We eat that we may live.
4. I could answer if I chose.
5. I know what you told him.
6. I do not think he will come.
7. If you run, you will be in time.
8. His bark is worse than his bite.
Answer:
1. I fired and he fell.
2. Come at any time, and you have the option in the matter.
3. We wish to live; and therefore, we eat.
4. I can answer, but I don’t choose to.
5. You told him something and I know it.
6. He will not come and I think so.
7. Run and you will be in time.
8. His bite is bad, but his bark is worse.

D. Removal And Use of ‘Too’

1. The news is too good to be true. = The news is so good that it cannot be true
2. She is too clever not to see through your tricks. = She is so clever that she will see through your tricks.
3. These mangoes are so cheap that they cannot be good. = These mangoes are too cheap to be good.

Exercise

I. Rewrite the following sentences removing the Adverb ‘too’.

1. This tree is too high for me to climb.
2. He speaks too fast to be understood.
3. He is far too stupid for such a difficult job.
4. She is sobbing too deeply to answer.
5. This fact is too evident to require proof.
6. Her dress is too striking not to attract attention.
7. He is too young to go on his own.
8. It is too late to do anything about it now.
Answer:
1. 1. This tree is so high that I can’t climb it.
2. He speaks so fast that he can’t be understood.
3. He is so stupid that he can’t do such a difficult job.
4. She is sobbing so deeply that she can’t answer.
5. This fact is so evident that it does not require any proof.
6. Her dress is so striking that it can’t fail to attract attention.
7. He is so young that he can’t go on his own.
8. It is so late that nothing can be done about it now.

II. Rewrite the following sentences using the Adverb ‘too’.

1. It is never so late that you can’t mend.
2. He is so proud that he won’t beg.
3. My heart is so full that I can’t find words to express myself.
4. He was so late that he couldn’t hear the first speech.
5. He is so ignorant that he can’t work as a postman.
6. The man was so old that it was not proper to rebuke him.
7. He is so weak that he cannot play.
8. Harish was so intelligent that he could understand this point.
Answer:
2. 1. It is never too late to mend.
2. He is too proud to beg.
3. My heart is too full for words.
4. He was too late to hear the first speech.
5. He is too ignorant to work as a postman.
6. The man was too old for a rebuke.
7. He is too weak to play.
8. Harish was too intelligent not to understand this point.

Miscellaneous Exercise (Based on Textual Sentences)

I. Rewrite the following sentences after removing ‘too’.

1. We must set things right before it is too late.
2. Water is too important a resource to be wasted.
3. Barb was too afraid to make a reply.
4. Sudha was too scared to go to meet Mr. JRD.
5. She was too old to have grown older.
6. Major Som Nath was too brave to quit even in the face of heavy firing.
7. I’m not too sure about it.
8. To spend too much time in studies is sloth.
9. We must hear the warning bells before it is too late.
Answer:
1. We must set things right before it is so late that we cannot mend them.
2. Water is so important a resource that it should not be wasted.
3. Barb was so afraid that she could not make a reply.
4. Sudha was so scared that she could not go to meet Mr. JRD.
5. She was so old that she could not have grown older.
6. Major Som Nath was so brave that he could not quit even in the face of heavy firing.
7. I’m not very sure about it.
8. It is sloth to spend so much time in studies.
9. We must hear the warning bells before it is so late that we can do nothing

II. Rewrite the following sentences using ‘too’.

1. We are so lazy that we do not care to lift the garbage lying around us.
2. The atombombs dropped on Hiroshima and Nagasaki were really very destructive.
3. After his encounter with the grizzly, Malcolm had become so ugly that nobody could love him.
4. Scars are not so powerful that they can change the person.
5. The need of water conservation is so grave that it cannot be ignored.
Answer:
1. We are too lazy to care to lift the garbage lying around us.
2. The atom bombs dropped on Hiroshima and Nagasaki were too destructive.
3. After his encounter with the grizzly, Malcolm had become too ugly to be loved by anyone.
4. Scars are not too powerful to change the person.
5. The need of water conservation is too grave to be ignored.

Exercises From Grammar Book (Fully Solved)

Exercise 1
Look at the following sentences and identify them as simple, compound or complex sentences.

1. This is the town where he was born.
2. Be kind and help the poor.
3. He is a good player.
4. He saw a man who was limping.
5. Stay healthy and cheerful.
6. He knew what he wanted from them.
7. I shall speak to you when I reach home.
8. In spite of hard work, he could not win.
9. He heard what I said.
10. They shall come home now.
Answer:
1. Complex sentence
2. Compound sentence
3. Simple sentence
4. Complex sentence
5. Simple sentence
6. Complex sentence
7. Complex sentence
8. Simple sentence
9. Complex sentence
10. Simple sentence.

Exercise 2
Identify the following sentences as simple, compound or complex sentences.

1. He must weep or he will die.
2. I know Rajesh is a clever person.
3. The village in which he lives is every small.
4. The train leaves at 5 p.m.
5. There is no student but loves good teachers.
6. Whoever is learned is respected.
7. What you say is quite correct.
8. He is too fat to run.
9. He is so fast that you cannot catch him.
10. He is very rich yet very humble.
Answer:
1. Compound sentence
2. Complex sentence
3. Complex sentence
4. Simple sentence
5. Complex sentence
6. Simple sentence
7. Complex sentence
8. Simple sentence
9. Complex sentence
10. Complex sentence.

Exercise 3
Identify the following sentences as simple, compound or complex sentences.

1. I can prove that he is a liar.
2. God made man and man made a machine.
3. He is more a fool than a cheat.
4. He was tall and handsome.
5. He says that he will win.
6. This is the business in which huge profits can be earned.
7. Moga is the place of my birth.
8. As you sow so shall you reap.
9. We must eat to live.
10. I hoped that it was true.
Answer:
1. Complex sentence
2. Compound sentence
3. Simple sentence
4. Simple sentence
5. Complex sentence
6. Complex sentence
7. Simple sentence
8. Complex sentence
9. Simple sentence
10. Complex sentence.

Exercise 4
Transform the following simple sentences into compound sentences.

1. He shall succeed with your assistance.
2. Climbing up the table, he cleaned the ceiling fan.
3. You must rest to avoid losing your health.
4. Work hard to pass the examination.
5. Through his sincere efforts, he won the first position.
6. Besides being hard-working, he is intelligent.
7. Coming here, he took away my books.
8. Owing to his indiscipline, he was reprimanded.
9. Seeing a tiger coming, he fled.
10. Going to New York, Manav met his friend.
Answer:
1. Assist him, and he shall succeed.
2. He climbed up the table and cleaned the ceiling fan.
3. You must rest or you will lose your health.
4. Work hard and you will pass the examination.
5. He made sincere efforts and won the first position.
6. He is not only hard-working, but also intelligent.
7. He came here and (he) took away my books.
8. He showed indiscipline, therefore he was reprimanded.
9. He saw a tiger coming and he fled.
10. Manav went to New York and met his friend.

Exercise 5
Transform the following simple sentences into compound sentences.

1. Getting out of the car, Mr. Rattan Tata walked away.
2. Being hungry, he needed food.
3. We were surprised to see him at our place.
4. He will compel you to leave the room.
5. Being lazy, he failed.
6. He fell asleep on account of being tired.
7. In spite of being poor he is very honest.
8. Notwithstanding his failure, he is still hopeful.
9. She was credited for her hard work.
10. Taking a stone, the threw at the dog.
Answer:
1. Mr. Rattan Tata got out of the car and walked away.
2. He was hungry, therefore he needed food.
3. We saw him at our place and (we) were surprised.
4. He will compel you and you will leave the room.
5. He was lazy, therefore he failed.
6. He was tired and fell asleep.
7. He is poor but he is honest.
8. He failed but he is still hopeful.
9. She worked hard, therefore she was credited.
10. He took a stone and threw it at the dog.

Exercise 6
Transform the following simple sentences into complex sentences.

1. He is sure of his success.
2. She doesn’t remember the exact date of her birth.
3. He knows the name of the best player in this team.
4. She promised to help me.
5. I owe my success to his guidance.
6. India is my land of birth.
7. His victory is certain.
8. His remarks pleased all.
9. He died in his youth.
10. He is too fat to run.
Answer:
1. He is sure that he will succeed.
2. She doesn’t remember the exact date when she was born.
3. He knows what the name of the best player in this team is.
4. She promised that she would help me.
5. I owe my success to him because he guided me.
6. India is the land where I was born.
7. It is certain that victory will be his.
8. It was his remarks that pleased all.
9. When he died, he was very young.
10. He is so fat that he cannot run.

Exercise 7
Transform the following simple sentences into complex sentences.

1. I wish him success.
2. India’s victory is certain.
3. It is the way to learn new ideas.
4. We must help him, a poor man.
5. He is too weak to walk.
6. This book is too good to lose.
7. It is his duty to safeguard his children.
8. Father desired him to succeed.
9. Work hard for success.
10. He acts like a joker.
Answer:
1. It is my wish that he may succeed.
2. It is certain that victory will be India’s.
3. It is the way through which one can learn new ideas.
4. We must help him because he is a poor man.
5. He is so weak that he cannot walk.
6. This book is so good that I cannot afford to lose it.
7. It is his duty that he should safeguard his children.
8. Father desired that he (his son) should succeed.
9. Work hard so that you may succeed.
10. He acts as if he were a joker.

Exercise 8
Transform the following simple sentences into complex sentences.

1. Owing to repeated failures, she made no effort.
2. He is too weak to stand.
3. They went to Chandigarh to buy a car.
4. He would be very pleased to help us in any way.
5. Of all the players, Virat is the best batsman.
6. On reaching the city, she went straight to her college.
7. Being lost in thoughts, I could not see him.
8. He got plucked in the tests because of his careless attitude.
9. On being caught red-handed, he had to admit his mistake.
10. In spite of all his riches, he had no peace of mind.
Answer:
1. She made no effort because she had failed repeatedly.
2. He is so weak that he cannot stand.
3. They went to Chandigarh so that they could buy a car there.
4. He would be very pleased if he could help us in any way.
5. As a batsman, Virat is so good that he cannot be compared with any other player.
6. When she reached the city, she went straight to her college.
7. I could not see him because I was lost in my thoughts.
8. He got plucked in the tests because he had a careless attitude.
9. He had to admit his mistake when he was caught red-handed.
10. He had all his riches, yet he had no peace of mind.

Exercise 9
Transform the following simple sentences into complex sentences.

1. He felt utterly helpless.
2. Sheena admitted her guilt.
3. The boss is likely to punish her.
4. He has informed her of her result.
5. That is the way to do it.
6. Having renounced the world, these monks live on charity.
7. He comes of a rich and noble family.
8. There I saw many beautiful palaces.
9. She likes my style.
10. He seems to be a fool.
Answer:
1. He felt that he was utterly helpless.
2. Sheena admitted that she was guilty.
3. It is likely that the boss will punish her.
4. He has informed her about what her result is.
5. That is the way how it should be done.
6. These monks live on charity because they have renounced the world.
7. He comes of a family which is rich and noble.
8. I saw that there were many beautiful palaces there.
9. She says admiringly that my style is very good.
10. It seems that he is a fool.

Exercise 10
Transform the following simple sentences into complex sentences.

1. Ms Sushma is said to be a good doctor.
2. The east wind cut like a knife.
3. Don’t leave the station without permission.
4. She speaks very fast for me to understand.
5. He is a lazy lad.
6. I like solving such problems.
7. His face expressions tell us a different story.
8. Today is the last day of this test match.
9. On being questioned, he confessed his fault.
10. Tell us the facts,
Answer:
1. It is said that Ms Sushma is a good doctor.
2. It seemed as if the east wind was cutting like a knife.
3. Don’t leave the station unless you are permitted.
4. She speaks so fast that I cannot understand her.
5. He is a lad who is lazy.
6. I am happy when I have to solve such problems.
7. His face expressions tell us that the actual story is different.
8. Today is the day which is the last day of this test match.
9. He confessed his fault when he was questioned.
10. Tell us what the facts are.

Exercise 11
Transform the following compound sentences into complex sentences.

1. He saw the lion and fled.
2. He got the message and replied at once.
3. I saw the thief and caught him.
4. You have to write it or you will forget it.
5. The teacher explained the sum and students noted it.
6. He finished his breakfast and left the table.
7. He was upset, but did not lose heart.
8. Be simple and you will win hearts.
9. She tried her best, but could not catch the thief.
10. The principal addressed the students and gave his message.
Answer:
1. He fled when he saw the lion.
2. He replied at once when he got the message.
3. As soon as I saw the thief, I caught him.
4. If you do not write it, you will forget it.
5. After the teacher had explained the sum, the students noted it.
6. He left the table after he had finished his breakfast.
7. Though he was upset, yet he did not lose heart.
8. You will win hearts if you are simple.
9. She could not catch the thief though she tried her best.
10. The principal gave his message to the students when he addressed them.

Exercise 12
Transform the following compound sentences into complex sentences.

1. He had lost the book and he has found it.
2. She read the letter and took off.
3. You must run or you will miss the meeting.
4. The nation calls us and we must respond.
5. We must eat or we can’t live.
6. He is unwell, but he doesn’t rest.
7. He must admit his fault or he will be thrown away.
8. Spare the rod and spoil the child.
9. Take care of your health and you will live longer.
10. Show me his picture, I will find him.
Answer:
1. He has found the book which he had lost.
2. As soon as she read the letter, she took off.
3. You will miss the meeting if you do not run.
4. We must respond when the nation calls us.
5. We can’t live if we don’t eat.
6. He doesn’t rest though he is unwell.
7. He will be thrown away if he does not admit his fault.
8. The child will be spoiled if you spare the rod.
9. If you take care of your health, you will live longer.
10. If you show me his picture, I will find him.

Exercise 13.
Transform the following compound sentences into complex sentences.

1. He must be advised or he will falter.
2. I must be informed about your loss or I shall not bother.
3. He cares for me so I respect him.
4. Write as I say or I will not help you.
5. She wrote to me and I responded immediately.
6. Be truthful and fear not.
7. Keep quiet or you will be punished.
8. We called the peon, but she did not appear.
9. She worked very hard, yet she did not top her class.
10. He was unhappy with his boss, so he left the job.
Answer:
1. He will falter if he is not advised.
2. If I am not informed about your loss, I shall not bother.
3. I respect him because he cares for me.
4. I will not help you if you don’t write as I say.
5. I responded immediately when she wrote to me.
6. You need not fear if you are truthful.
7. You will be punished if you don’t keep quiet.
8. The peon did not appear when we called her.
9. She did not top her class though she had worked very hard.
10. He left the job because he was unhappy with his boss.

Exercise 14
Transform the following compound sentences into complex sentences.

1. She asked me and I gave her all the details of the incident.
2. Either you tell us the truth or you will be sacked.
3. Finish this work in time, or I will not give any more orders.
4. He threw off his shirt and jumped into water to pull out the drowning child.
5. He has a charming smile, therefore, he has made many fans.
6. I found his mobile and he was very thankful to me.
7. He is an old friend, therefore, I respect him.
8. She must weep, or she will fall sick.
9. Milkha Singh was hard-working and he won many races.
10. They were afraid and they ran away.
Answer:
1. I gave her all the details of the incident when she asked me.
2. You will be sacked if you don’t tell us the truth.
3. I will not give any more orders if you don’t finish this work in time.
4. He threw off his shirt and jumped into water because he wanted to pull out the drowning child.
5. He has made many fans because he has a charming smile.
6. He was very thankful to me because I had found his mobile.
7. I respect him because he is an old friend.
8. She will fall sick if she doesn’t weep.
9. Milkha Singh won many races because he was hard working.
10. They ran away because they were afraid.

Exercise 15
Transform the following compound sentences into complex sentences.

1. He must resign, or he will be thrown out unceremoniously.
2. I rang up at your number, but you never responded.
3. Be diligent and you will succeed.
4. Be nice to others and you will be happy.
5. I not only make promises, but also fulfil them.
6. He took a break and started working again.
7. He should not be late or he may be fined.
8. Listen and she will explain.
9. Waste not, want not.
10. Give the papers to my secretary and I will sign them.
Answer:
1. He will be thrown out unceremoniously if he does not resign.
2. You never responded whenever I rang up at your number.
3. You will succeed if you are diligent.
4. You will be happy if you are nice to others.
5. I fulfil promises whenever I make them.
6. He started working again after he had taken a break.
7. He may be fined if he is late.
8. She will explain if you listen.
9. You will not want if you don’t waste.
10. I will sign the papers if you give them to my secretary.

Exercise 16
State which of the following sentences are ‘Compound and which are ‘Complex’.

1. He must cry or he will die.
2. He knows Reena who is a clever girl.
3. The village in which I was born is very small.
4. I returned home because I was tired.
5. He will treat them, as they treated him.
6. God made rivers and man made dams.
7. A guest is unwelcome when he stays too long.
8. He is not only handsome, but also clever.
9. I liked what he suggested.
10. She was sick, but still she attended school.
Answer:
1. Compound sentence
2. Complex sentence
3. Complex sentence
4. Complex sentence
5. Complex sentence
6. Compound sentence
7. Complex sentence
8. Compound sentence
9. Complex sentence
10. Compound sentence.

Exercise 17
State which of the following sentences are ‘Compound and which are ‘Complex’.

1. As I was sick, I could not go out.
2. The higher we go, the cooler it is.
3. We wish he should win.
4. She was ill, therefore, she could not come to school.
5. I was tired and fell asleep.
6. He had no advice that he could offer.
7. She is rich but she is miserly.
8. I will help him with the resources that he needs.
9. When he was questioned, he faltered.
10. She is very intelligent yet simple.
Answer:
1. Complex sentence
2. Complex sentence
3. Complex sentence
4. Compound sentence
5. Compound sentence
6. Complex sentence
7. Compound sentence
8. Complex sentence
9. Complex sentence
10. Compound sentence.

Exercise 18 (a)
State which of the following sentences are Compound and which are ‘Complex’.

1. This is the place where Gandhiji stayed.
2. I know what is in this packet.
3. I picked up the chalk and wrote on the board.
4. He can go wherever he likes.
5. Neither you nor he is wrong.
6. Don’t talk while I am speaking.
7. It was not so big as I thought.
8. It seems as if it might rain.
9. I will help her when she needs any help.
10. I got up and walked out of the meeting.
Answer:
1. Complex sentence
2. Complex sentence
3. Compound sentence
4. Complex sentence
5. Compound sentence
6. Complex sentence
7. Complex sentence
8. Complex sentence
9. Complex sentence
10. Compound sentence.

Exercise 18 (b)
Transform the following sentences into interrogative.

1. It is useless to blame her.
2. Everyone knows him.
3. Our soldiers are exceptionally brave.
4. It is not a foolish idea.
5. The deaf cannot hear.
6. Everyone loves his parents.
7. His arguments are baseless.
8. Cowards die many times before their death.
9. A rolling stone gathers no moss.
10. This is not the way a gentleman should behave.
Answer:
1. Isn’t it useless to blame her ?
2. Who doesn’t know him ?
3. Aren’t our soldiers exceptionally brave ?
4. Is it a foolish idea ?
5. Can the deaf hear ?
6. Who does not love his parents?
7. Aren’t his arguments baseless ?
8. Don’t cowards die many times before their death ?
9. Can a rolling stone gather moss ?
10. Is it the way a gentleman should behave ?

Exercise 19
Transform the following sentences into assertive.

1. Why cry over spilt milk ?
2. Why be dishonest ?
3. Who is free from guilt ?
4. Who can serve two masters ?
5. Is honesty not the best policy?
6. Why waste time in useless arguments ?
7. Why go to him ?
8. Who would not like to be a millionaire ?
9. Who likes to be the last ?
10. Can anybody say that he has never told a lie ?
Answer:
1. It is no use crying over spilt milk.
2. You should not be dishonest.
3. Nobody is free from guilt.
4. Nobody can serve two masters.
5. Honesty is the best policy.
6. It is no use wasting time in useless arguments.
7. It is no use going to him.
8. Everybody would like to be a millionaire.
9. Nobody likes to be the last.
10. Nobody can say that he has never told a lie.

Exercise 20
Transform the following exclamatory sentences into assertive.

1. O for a cup of coffee !
2. Would that I were an engineer !
3. How stupid of him to say that.
4. Alas ! He has lost his purse.
5. Hurrah ! We have won.
6. Oh ! you are going.
7. If I were the Prime Minister of India !
8. What a great start of the day !
9. What a lovely face !
10. How sweet of you!
Answer:
1. I long for a cup of coffee.
2. My greatest wish is to become an engineer.
3. It was very stupid of him to say that.
4. It is very sad that he has lost his purse.
5. It is a matter of great joy that we have won.
6. It is sad that you are going.
7. My greatest ambition is to become the Prime Minister of India.
8. It is a very great start of the day.
9. It is a very lovely face.
10. It is very sweet of you.

Exercise 21
Transform the following sentences into exclamatory.

1. I wish I had a cup of tea.
2. It was a very nice day.
3. It is very sad that she is undone.
4. It is very odd.
5. He narrates the story very cleverly.
6. Swami Vivekananda was a great speaker.
7. Kishore was, indeed, a great singer.
8. It is very stupid of him to critize others.
9. Man is a strange piece of work.
10. Death is very dreadful.
Answer:
1. O for a cup of tea !
2. What a nice day it was !
3. Alas ! She is undone.
4. How odd it is !
5. How cleverly he narrates the story !
6. What a great speaker Swami Vivekananda was !
7. What a great singer Kishore was !
8. How stupid of him to criticize others !
9. What a strange piece of work man is !
10. How dreadful death is !

Exercise 22
Transform the following sentences, using comparative degree of comparison.

1. He is not so tall as his brother.
2. He is the most honest employee.
3. No other boy is as good as Parth.
4. Jill is not as beautiful as Maggie.
5. I have never seen such a beautiful girl as Rekha.
6. No other metal is as useful as iron.
7. This book is not so good as that.
8. Himalaya runs as fast as a horse.
9. Mandeep is as strong as Sandeep.
10. Alisha does not sing so well as Lata.
Answer:
1. His brother is taller than him.
2. He is more honest than any other employee.
3. Parth is better than any other boy.
4. Maggie is more beautiful than Jill.
5. Rekha is more beautiful than any other girl I have ever seen.
6. Iron is more useful than any other metal.
7. That book is better than this one.
8. A horse doesn’t run faster than Himalaya.
9. Sandeep is not stronger than Mandeep.
10. Lata sings better than Alisha.

Exercise 23
Transform the following sentences, using positive degree of comparison.

1. She is not more intelligent than I.
2. Ravi is smarter than Kishan.
3. This poem is better than that.
4. Mercedes car runs faster than Honda bikes.
5. My brother is richer than me.
6. Mussoorie is cooler than Dehradun.
7. Water is the best drink.
8. Mother India is one of the best Indian movies.
9. Ashoka the Great was one of the best kings of all times.
10. Lead is the heaviest metal.
Answer:
1. I am as intelligent as she (is).
2. Kishan is not so smart as Ravi.
3. That poem is not so good as this one.
4. Honda bikes don’t run so fast as a Mercedes car.
5. I am not as rich as my brother.
6. Dehradun is not so cool as Mussoorie.
7. No other drink is so good as water.
8. Very few Indian movies are so good as Mother India.
9. Very few kings were so good as Ashoka the great in all times.
10. No other metal is so heavy as lead.

Exercise 24
Transform the following sentences, using superlative degree of comparison.

1. Kalidas is greater than any other poet in India.
2. He is more liberal than many people.
3. My city is cleaner than many other cities of Punjab.
4. Birds do not fly as fast as airplanes.
5. Simran is more intelligent than her friends.
6. No other hill station in India is so busy as Mussoorie in India.
7. No other flower is so good as rose.
8. No other season is so pleasant as spring.
9. Very few film-makers were as good as Raj Kapoor.
10. He is smarter than any other boy in our neighbourhood.
Answer:
1. Kalidas is the greatest poet in India.
2. He is one of the most liberal people.
3. My city is one of the cleanest cities of Punjab.
4. Airplanes fly the fastest.
5. Simran is the most intelligent girl among her friends.
6. Mussorie’ is the busiest hill station in India.
7. Rose is the best flower.
8. Spring is the most pleasant season.
9. Raj Kapoor was one of the best film-makers.
10. He is the smartest boy in our neighbourhood.

‘Do As Directed’ Type Exercises From Grammar Book (Fully Solved)

Exercise 1
Do as directed

1. The teacher is too weak to control the class. (remove ftoo)
2. Bullet trains run faster than mail trains. (Change the degree of comparison)
3. He hoped to pass the test. (Change into a complex sentence)
4. He was honoured for his honesty. (Change into a compound sentence)
5. He must run fast or he will not catch the train. (Change into a simple sentence)
6. Mumbai is the largest metro city of India. (Use positive degree of comparison)
7. Who doesn’t make mistakes ? (Change into an assertive sentence)
8. The case is too urgent to be postponed. (Removee too)
9. One more mistake and he will be fired. (Use If)
10. As soon as he lay on bed, the postman rang the doorbell. (Change into a negative sentence)
Answer:
1. The teacher is so weak that she cannot control the class.
2. Mail trains do not run so fast as the bullet trains.
3. He hoped that he would pass the test.
4. He was honest and so he was honoured.
5. He must run fast to catch the train.
6. No other metro city is so large in India as Mumbai.
7. Everyone makes mistakes.
8. The case is so urgent that it cannot be postponed.
9. If he makes one more mistake, he will be fired.
10. No sooner did he lie on bed than the postman rang the doorbell.

Exercise 2
Do as directed.

1. He is too composed to lose temper. (remove too)
2. She is not so intelligent her as sister is. (Change the degree of comparison)
3. He sold his lame horse.(Change into a complex sentence)
4. He asked for too much money as he was greedy. (Change into a compound sentence)
5. It was a possibility that was vague. (Change into a simple sentence)
6. Ram is the tallest boy. (Use positive degree of comparison)
7. A sailor and afraid of storm! (Change into an assertive sentence)
8. She is so old that she cannot walk. (Use ‘too)
9. You cannot win competition unless you work hard. (Use If)
10. Everyone will believe her word. (Change into a negative sentence)
Answer:
1. He is so composed that he cannot lose temper.
2. Her sister is more intelligent than her.
3. He sold his horse that was lame.
4. He was greedy, so he asked for too much money.
5. It was a vague possibility.
6. No other boy is as tall as Ram.
7. A sailor is so brave that he cannot be afraid of storm.
(or)
A sailor cannnot be afraid of a storm.
8. She is too old to walk.
9. If you don’t work hard, you can’t win the competition.
10. Nobody will disbelieve (doubt) her word.

Exercise 3
Do as directed.

1. She is too practical to understand real love. (remove ‘too)
2. As soon as he saw a lion, he began shuddering with fear. (Rewrite using ‘No sooner….than)
3. The child is playing with a broken doll. (Change into a complex sentence)
4. The sun having set, we returned home. (Change into a compound sentence)
5. He admitted that he was at fault. (Change into a simple sentence)
6. Very few kings were as great as Ashoka. (Change degree of comparison)
7. How fast Milkha ran! (Change into an assertive sentence)
8. She is so weak that she can’t even sit. (Use ‘too)
9. You will feel tired if you don’t take proper rest. (Use ‘unless)
10. She is a great artist. (Change into an exclamatory sentence)
Answer:
1. She is so practical that she cannot understand real love.
2. No sooner did he see a lion than he began shuddering with fear.
3. The child is playing with a doll that is broken.
4. The sun had set and we returned home.
5. He admitted his fault.
6. Ashoka was greater than many other kings.
7. Milkha ran very fast.
8. She is too weak even to sit.
9. Unless you take proper rest, you will feel tired.
10. What a great artist she is !

Exercise 4
Do as directed.

1. These fruits are too cheap to be good. (Remove ‘too)
2. As soon as I stepped out of my office, it started raining heavily. (Rewrite using “No sooner….than)
3. Everyone believes in my sincerity. (Change into a complex sentence)
4. Owing to his sickness, he could not play the match. (Change into a compound sentence)
5. She told me where she lived. (Change into a simple sentence)
6. The peacock is the most beautiful bird. (Change degree of comparison)
7. What a great shot ! (Change into an assertive sentence)
8. That man is too arrogant to be pleased. (Use ‘too)
9. You will fall sick if you don’t stop eating. (Use ‘unless)
10. This painting is a great piece of art. (Change into an exclamatory sentence)
Answer:
1. These fruits are so cheap that they cannot be good.
2. No sooner did I step out of my office than it started raining heavily.
3. Everyone believes that I am sincere.
4. He was sick and so he could not play the match.
5. She told me the place of her residence.
6. No other bird is so beautiful as the peacock.
7. It was a very good shot.
8. That man is so arrogant that he cannot be pleased.
9. Unless you stop eating, you will fall sick.
10. What a great piece of art this painting is !

Exercise 5
Do as directed.

1. This news is too good to be true. (Remove ‘too)
2. Hardly had he finished his work when his boss gave him another task. (Rewrite using ‘No sooner….than)
3. He is too proud to appreciate anyone else. (Change into a complex sentence)
4. Being lazy, he failed. (Change into a compound sentence)
5. The iron rod was so hot that he could not touch it.(Change into a simple sentence)
6. He is smarter than any other employee in his office. (Change degree of comparison)
7. Money cannot buy happiness. (Change into an interrogative sentence)
8. The water in the pool is so cold that one cannot swim in it. (Use ‘too)
9. He is poor. He is honest. (Use though)
10. Is there anything greater than the love for your motherland ? (Change into a negative sentence)
Answer:
1. This news is so good that it cannot be true.
2. No sooner did he finish his work than his boss gave him another task.
3. He is so proud that he cannot appreciate anyone else.
4. He was lazy, so he failed.
5. The iron rod was too hot for him to touch it.
6. He is the smartest employee in his office.
(or)
No other employee in his office is so smart as he is.
7. Can money buy happiness ?
8. The water in the pool is too cold for anyone to swim in it.
9. He is honest though he is poor.
10. Nothing is greater than the love for your motherland.

Exercise 6
Do as directed.

1. The tree was too tall for me to climb it. (Remove too)
2. As soon as the sun rose, the clouds disappeared. (Rewrite using ‘No sooner….than)
3. I saw the danger and moved on. (Change into a complex sentence)
4. With a great effort he passed the examination. (Change into a compound sentence)
5. If weather permits, they will play the match. (Change into a simple sentence)
6. Kapil was the best of all all-rounders in the world. (Change degree of comparison)
7. He never drinks milk. (Change into an interrogative sentence)
8. He is so slow that he cannot win the race. (Use too)
9. We cannot go for picnic unless it stops raining. (Rewrite using ‘if)
10. Ram was older than Sham. (Change into a negative sentence)
Answer:
1. The tree was so tall for me that I could not climb it.
2. No sooner did the sun rise than the clouds disappeared.
3. Even though I saw the danger, I moved on.
4. He put in a great effort and passed the examination.
5. Weather permitting, they will play the match.
6. No other all-rounder in the world was so good as Kapil.
(or)
Kapil was better than any other all-rounder in the world.
7. Does he ever drink milk ?
8. He is too slow to win the race.
9. If it does not stop raining, we cannot go for picnic.
10. Sham was not so old as Ram.

Exercise 7
Do as directed.

1. The iron is too hot for me to touch. (Remove ‘too)
2. As soon as the moon rose, the stars disappeared.(Rewrite using No sooner….than)
3. He tried hard but he failed. (Change into a complex sentence)
4. He is learned but he is not courteous. (Change into a complex sentence)
5. He failed though he worked hard. (Change into a simple sentence)
6. Hari is as tall as Shyam. (Change degree of comparison)
7. The police will take steps to control the situation. (Change into interrogative sentence)
8. He is so stupid that he cannot handle such a situation. (Use ‘too)
9. Can money buy health ? (Change into an assertive sentence)
10. He played a great shot. (Change into an exclamatory sentence)
Answer:
1. The iron is so hot for me that I cannot touch it.
2. No sooner did the moon rise than the
stars disappeared. 3. Though he tried hard, he failed.
4. Though he is learned, he is not courteous.
5. He failed in spite of working hard.
6. Shyam is not taller than Hari.
7. Won’t the police take steps to control the situation ?
8. He is too stupid to handle such a situation.
9. Money cannot buy health.
10. What a great shot he played !

Exercise 8
Do as directed.

1. He is too slow to learn. (Remove too)
2. As soon as he saw the tiger, he took to heels. (Rewrite using No sooner….than)
3. This is Gandhiji’s birthplace. (Change into a complex sentence)
4. When the day dawned, we got up. . (Change into a compound sentence)
5. The boy was so weak that he could not stand properly. (Change into a simple sentence)
6. Akshay is the best comedy actor. (Change degree of comparison)
7. Walk fast. You may miss the train. (Combine these sentences)
8. This news is so good that it cannot be true. (Use ‘too)
9. She confessed her fault. (Change into a complex sentence)
10. There is a doctor in the stadium. (Change into an interrogative sentence)
Answer:
1. He is so slow that he cannot learn.
2. No sooner did he see the tiger than he took to heels.
3. This is the place where Gandhiji was born.
4. The day dawned and we got up.
5. The boy was too weak to stand properly.
6. No other comedy actor is so good as Akshay.
(or)
Akshay is better than any other comedy actor.
7. Walk fast or you may miss the train.
(or)
If you don’t walk fast, you may miss the train.
8. This news is too good to be true.
9. She confessed that she was at fault.
10. Isn’t there a doctor in the stadium ?

Exercise 9
Do as directed.

1. He was too late to attend the meeting. (Remove ‘too)
2. As soon as his examination started, he started writing the answers. (Rewrite using No sooner….than)
3. Obey your parents or you will come to grief. (Change into a complex sentence)
4. He punished his servant because he behaved rudely. (Change into a compound sentence)
5. This tree is so high that none can climb it. (Change into a simple sentence)
6. Love is greater than any other thing in the world. (Change degree of comparison into superlative degree)
7. If you read, you will learn. (Remove if)
8. He is so arrogant that no one can like him. (Use ‘too)
9. None but sincere people can achieve success. (Change into affirmative)
10. Only Rekha has done justice to her role. (Replace ‘Only’ by ‘none)
Answer:
1. He was so late that he could not attend the meeting.
2. No sooner did his examination start than he started writing the answers.
3. If you don’t obey your parents, you will come to grief.
4. His servant behaved rudely so he punished him.
5. This tree is too high for anyone to climb it.
6. Love is the greatest thing in the world.
7. You read and you will learn.
(or)
Unless you read, you will not learn.
8. He is too arrogant for anyone to like him.
9. Only sincere people can achieve success.
10. None but Rekha has done justice to her role.

Exercise 10
Do as directed.

1. She is too strong for her opponents. (Remove ‘too)
2. No sooner did I hear of his success than I congratulated him. (Remove No sooner….than)
3. I have no time to waste. (Change into a complex sentence)
4. The play being over, the audience left the hall. (Change into a compound sentence)
5. As soon as I arrived, I sent for her. (Change into a simple sentence)
6. This city has more parks than playgrounds. (Change degree of comparison)
7. Unless you write the correct answer, you will not get full marks.(Remove ‘Unless)
8. Tea is sweeter than it should be. (Use too)
9. He is insincere. (Change into negative)
10. Only the polar star remained in sight. (Replace ‘Only’ by ‘all)
Answer:
1. She is so strong that her opponents cannot compete with her.
2. As soon as I heard of his success, I congratulated him.
3. I have no time which I can waste.
4. The play was over and the audience left the hall.
5. Soon after my arrival, I sent for her.
6. This city does not have so many playgrounds as parks.
7. If you don’t write the correct answer, you will not get full marks.
8. Tea is too sweet for it to be good.
9. He is not sincere.
10. All the stars, except the polar star, were not in sight.

Exercise 11
Do as directed.

1. She is too proud to listen. (Remove ‘too)
2. No sooner did he start his bike than the petrol finished in the fuel tank. (Remove “No sooner….than)
3. A hard-working person succeeds in life. (Change into a complex sentence)
4. He fell asleep on account of being tired. (Change into a compound sentence)
5. He is sure that he will succeed. (Change into a simple sentence)
6. Our shopkeeper sells at a cheaper rate than any other shopkeeper in the area. (Change degree of comparison)
7. If you do not water the plants, they will not bloom. (Remove If )
8. It is never so late that one cannot tell the truth. (Use ‘too)
9. She is trying many dresses. (Change into negative)
10. She came here ten years ago. (Replace ‘ago’ by ‘since)
Answer:
1. She is so proud that she won’t listen.
2. As soon as he started his bike, the petrol finished in the fuel tank.
3. A person who is hard-working succeeds in life.
4. He was tired and so fell asleep.
5. He is sure of his success.
6. No other shopkeeper in the area sells at so cheap rate as our shopkeeper.
7. In the event of not being watered, the plants will not bloom.
(or)
Unless you water the plants, they will not bloom.
8. It is never too late for anyone to tell the truth.
9. She is not trying just a few dresses.
10. It is ten years since she came here.

Exercise 12
Do as directed.

1. The news is too good to be true. (Remove ‘too)
2. As soon as the referee blew the whistle, the athletes started running. (Use “No sooner….than)
3. The child was pleased at having the toy. (Change into a complex sentence)
4. Both the parties were happy with the solution that they arrived at. (Change into a compound sentence)
5. Men who work hard rise in life. (Change into a simple sentence)
6. He is not cleverer than his brother. (Change degree of comparison)
7. If you study, you will pass. (Remove ‘if)
8. Very few countries are as great as India. (Change into superlative degree of adjective)
9. Sometimes Kamal acts foolishly. (Change into negative)
10. Ten years have passed since we met. (Use ‘ago)
Answer:
1. This news is so good that it can’t be true.
2. No sooner did the referee blow the whistle than the athletes started running.
3. The child was pleased when it got the toy.
4. Both the parties arrived at a solution and they were happy about it.
5. Hard-working men rise in life.
6. His brother is as clever as he is.
7. Unless you study, you won’t pass.
8. India is one of the greatest countries.
9. Sometimes Kamal does not act wisely.
10. We met ten years ago.

Exercise 13
Do as directed.

1. She was too busy to go to cinema. (Remove ‘too)
2. No sooner did I learn of his illness than I took him to the doctor. (Rewrite using ‘as soon as)
3. He is poor but he is honest. (Change into a complex sentence)
4. He was not only punished, but also fined. (Change into a compound sentence)
5. It was a trick that was difficult to understand. (Change into a simple sentence)
6. Kolkata is the most populated city of India. (Change degree of comparison)
7. Speak the truth and you don’t have to be afraid. (Use if )
8. Who does not want peace ? (Change into assertive)
9. She did not forgive me. (Change into positive)
10. Everyone admires the brave. (Change into interrogative)
Answer:
1. She was so busy that she could not go to the cinema.
2. As soon as I learnt of his illness, I took him to the doctor.
3. He is honest although he is poor.
4. He was punished and also he was fined.
5. The trick was too difficult to understand.
6. No other city of India is so populated as Kolkata.
(or)
Kolkata is more populated than any other city of India.
7. If you speak the truth, you don’t have to be afraid.
8. Everybody wants peace.
9. She refused to forgive me.
10. Who does not admire the brave?

Exercise 14
Do as directed.

1. The soldiers are too disciplined to violate rules. (Remove ‘too)
2. If a woman is educated, her whole family is benefitted. (Rewrite using ‘Unless)
3. I love India, my motherland. (Change into a complex sentence)
4. He has all the virtues of a great man except straightforwardness. (Change into a compound sentence)
5. I was very tired after the day’s work and I sat down to take some rest. (Change into a simple sentence)
6. A wise foe is better than foolish friend. (Change degree of comparison)
7. We share our room and books. (Use not only…..but also)
8. Does the moon not revolve around the earth ? (Change into assertive)
9. You are not without wisdom. (Change into positive)
10. He drives very fast. (Change into interrogative)
Answer:
1. The soldiers are so disciplined that they don’t violate rules.
2. Unless a woman is educated, her whole family won’t be benefitted.
3. I love India which is my motherland.
4. He has all the virtues of a great man, but he is not a straightforward man.
5. Being very tired after the day’s work, I sat down to take some rest.
6. A foolish friend is not so good as a wise foe.
7. We share not only our room, but also our books.
8. The moon revolves around the earth.
9. You are wise.
(or)
You possess wisdom.
10. Doesn’t he drive very fast ?

Exercise 15
Do as directed.

1. It is too foggy outside to see anything. (Remove ‘too)
2. He left for USA two years ago. (Rewrite using Since)
3. Please write your address. (Change into a complex sentence)
4. She could not run fast as she was fat. (Change into a compound sentence)
5. Though he ran fast, he could not catch me. (Change into a simple sentence)
6. Swimming is the best exercise. (Change degree of comparison)
7. Seeta and Geeta are beautiful. (Use not only….. but also)
8. Does anyone like cheats ? (Change into assertive)
9. This sum is not difficult. (Change into positive)
10. That is not the way a lady should behave. (Change into interrogative)
Answer:
1. It is so foggy outside that it is not possible to see anything.
2. It is two years since he left for USA.
3. Please write where you live.
4. She was fat, so she could not run.
5. He could not catch me in spite of running fast.
6. No other exercise is so good as swimming.
(or)
Swimming is better than any other exercise.
7. Not only Seeta, but Geeta is also beautiful.
8. Nobody likes cheats.
9. This sum is easy.
10. Is this the way a lady should behave ?

Exercise 16
Do as directed.

1. He is too smart to lose his focus. (Remove ‘too)
2. Walk fast. You might miss the bus. (Rewrite using ‘lest….should)
3. He is a hard-working person. (Change into a complex sentence)
4. Hurry up or you will be late for school. (Change into a complex sentence)
5. Since India is my motherland, I love her. (Change into simple sentence)
6. He is as wise as his brother (Change degree of comparison)
7. He is a painter and a poet. (Use not only…..but also)
8. How shameful ! (Change into assertive)
9. He is very talented. (Use too)
10. You cannot please everyone. (Change into interrogative)
Answer:
1. He is so smart that he can’t lose his focus.
2. Walk fast lest you should miss the bus.
3. He is a person who works hard.
4. You will be late if you don’t hurry up.
5. India being my motherland, I love her.
6. His brother is not wiser than him.
7. He is not only a painter but also a poet.
8. It is very shameful of him.
9. He is too talented.
10. Can you please everyone ?

Punjab State Board PSEB 11th Class English Book Solutions English Reading Comprehension Unseen Passages Exercise Questions and Answers, Notes.

PSEB 11th Class English Reading Comprehension Unseen Passages

Examination-Style Fully Solved Passages

Passage 1

In many countries, only one language, the mother tongue, is enough to satisfy the need for expression of most of their inhabitants2. In India, however, the position is more complicated3. Here, an educated man is called upon to master more than one language. There is, first of all, the language that he learns on his mother’s lap, and through which he expresses his first needs and feelings. Naturally, it is in this that he gains the most proficiency4. But since India is a very large country, we really need an extra language – a lingua franca) — as a means of communication with states other than our own. But even this is not enough. An educated Indian also requires the mastery of an international language, one that is widely understood and is used in the dealings of one country with another. Ideally speaking then, an educated Indian should be able to read and write in three languages, and moreover, should be able to express himself in all three with ease and fluency’.

Question 1.
In many countries, only ………… is enough for people to express their ideas.
(a) mother tongue
(b) a foreign language
(c) local language
(d) none of the above.
Answer:
(a) mother tongue

Question 2.
In India, an educated man is expected to master …………….
(a) his mother tongue
(b) a foreign language
(c) more than one language
(d) the national language.
Answer:
(c) more than one language

Question 3.
What purpose does the language used by us serve ?
Answer:
It serves our need for expression and communication.

Question 4.
What is the position in India regarding the languages ?
Answer:
Here an educated person is expected to master three lauguages – the mother tongue, a lingua franca and a foreign language.

Question 5.
An educated Indian is supposed to be able to read and write in ……….. languages.
Answer:
three.

Question 6.
Match the words in column A with their meanings in column B.

A	B
big	most
sufficient	enough
	large

Answer:
big – large;
sufficient – enough.

Passage 2

Food can maintain? or save life. It can destroy life as well. Proper food serves the purpose of medicine. Improper food works as poison and causes diseases. We may take pride in calling ourselves civilized; but we flout? all the norms about the quality or quantity of food. We mostly eat processed foods. We have drifted away from mother nature. Thus the incidence of diabetes has increased very much. According to a survey, diabetes was rare in the natives of Canada a few years ago. With the advent of processed and junk foods, the incidence of diabetes has shot up within a very short time. By offering chocolates, cakes and ice creams too often to our children and by attending parties every other day, we in fact, invite obesity and diabetes.

Question 1.
Food can …………… our life.
(a) maintain
(b) save
(c) destroy
(d) all the above.
Answer:
(d) all the above.

Question 2.
The incidence of diabetes has …………… very much.
(a) decreased
(b) drifted
(c) increased
(d) rare.
Answer:
(c) increased

Question 3.
What is improper food ?
Answer:
Food that is harmful to health is improper food.

Question 4.
How have we changed our food habits ?
Answer:
We have started eating processed foods.

Question 5.
Diabates used to be ………… in the natives of Canada earlier.
Answer:
rare.

Question 6.
Match the words in column A with their opposites in column B.

A	B
destroy	advent
mostly	create
	rarely

Answer:
destory → create;
mostly → rarely.

Passage 3

Among the manifold’ misfortunes that may befall humanity, the loss of health is one of the severest?. All the joys, which life can give, can’t outweigh the suffering of the sick. Give the sick man everything and leave him with his suffering, he will feel half the world is lost to him. Lay him on a soft silken couch3, he will nevertheless4 groan sleepless under the presence of his suffering, while the miserable beggar, blessed with health, sleeps sweetly on the hard ground. Spread his table with dainty meals and choicest drinks and he will thrust back the hand that proffers them, and envy the poor man who thoroughly enjoys his dry crust. Surround him with the pomp of kings; let his chair be a throne and his crutch a world-swaying sceptre, he will look with contemptuous eyes on marble, on gold, on purple, and would deem himself happy, could he enjoy, even were it under a thatched roof, the health of the meanest of his servants.

Question 1.
Loss of ………….. is one of the severest misfortunes.
(a) joy
(b) health
(c) money
(d) humanity.
Answer:
(b) health

Question 2.
The man who is suffering will be happy to ……..
(a) get marble
(b) receive gold
(c) be blessed with purple
(d) get back good health.
Answer:
(d) get back good health.

Question 3.
Which misfortune is regarded as one of the severest ?
Answer:
Loss of health is regarded as one of the severest misfortunes.

Question 4.
What is the strongest wish of a sick man ?
Answer:
His strongest wish is to get rid of his suffering.

Question 5.
A sick man will hate-marble, gold or …………..
Answer:
purple.

Question 6.
Match the words in column A with their meanings in column B.

A	B
purpose	result
obesity	fatness
	aim

Answer:
purpose → aim;
abesity → fatness.

Passage 4

Good manners are not inherited?. And they don’t come naturally to intelligent people. They have to be learnt and practised. They are based upon the concept of consideration3 for others. They are easy to acquire4 and there is nothing more profitable. Good manners are a necessary complement in every walk of life, especially in business. Organisations with competent and well-mannered representatives enjoy a good reputation. The morale8, productivity and profits of such an organisation will be high. And this, in turn, will attract more business. There is no particular place or time when a person should show or begin his elementaryo courtesy. Courtesy, etiquette 10 and manners are tools that one should always carry with oneself, wherever one goes. The first step to success in life is treating others as courteously as we would wish others to treat us.

Question 1.
Good manners don’t come ………. to intelligent people.
(a) ever
(b) unnaturally
(c) easily
(d) naturally.
Answer:
(d) naturally.

Question 2.
Good manners are more ………….. than any other thing.
(a) inherited
(b) profitable
(c) difficult
(d) practised.
Answer:
(b) profitable

Question 3.
What are good manners ?
Answer:
Good manners mean a consideration for others.

Question 4.
How are good manners aequired ?
Answer:
Good manners have to be learnt and practised.

Question 5.
We must treat others as ………….. as we would wish them to treat us.
Answer:
courteously.

Question 6.
Match the words in column A with their opposites in column B.

A	B
naturally	nowhere
everywhere	artificially
	particularly

Answer:
naturally → artificially;
everywhere → nowhere.

Passage 5

For a student, walking is preferable to all other exercises. The advantage of this model of exercise is that it is simple. The apparatus2 is all at hand. You need not wait for the importationof machinery. It is in the open air that the lungs can at once receive the pure air of heaven and the eyes gaze upon hill and dale“, upon trees and flowers, upon the objects animate and inanimates. The very objects of sight and sound cheer the mind and raise the spirit. Another advantage of walking is that you can have a friend to walk with and unbend the mind by pleasant conversation. Once try the method of walking with a friend regularly for a few weeks and you will be surprised at the marvellouso results. On those afternoons when study is not required, be sure to take a long walk and lay up health for days to come.

Question 1.
The advantage of walking is that it is ………..
(a) complicated
(b) tough
(c) simple
(d) energy-giving.
Answer:
(c) simple

Question 2.
The objects of sight and sound ………….. the mind.
(a) lift
(b) increase
(c) sharpan
(d) cheer.
Answer:
(d) cheer.

Question 3.
Which exercise is preferable for a student ?
Answer:
For a student, walking is preferable to all other exercises.

Question 4.
How are the lungs of a walker benefited ?
Answer:
His lungs receive the pure air of heaven.

Question 5.
The advantage of walking is that it is ………..
Answer:
simple.

Question 6.
Match the words in column A with their meanings in column B.

A	B
advantage	benefit
heaven	export
	paradise

Answer:
advantage → benefit;
heaven → paradise.

Passage 6

If a man’s house is full of bottles of medicines, we think that the man is probably sick; but if his house is full of books, we concludea that he is intelligent. We consider the bottles of medicines as the sign of a sick body, but we do not consider the books as the sign of a sick mind. Surely that is not right. The wise men of the past took no pains to make life literate”; but they did much to make it meaningful. Books, after all, contain only letters. It is useless to hope to make life meaningful merelys by collecting books. Can we know swimming merely by reading a book on swimming ?

Real knowledge is to be found in life itself. Until we understand this, we shall never taste true knowledge.

Question 1.
There will be many bottles of medicines in a …………… man’s house.
(a) sick
(b) healthy
(c) plump
(d) weak.
Answer:
(a) sick

Question 2.
The wise men of the past did much to make life
(a) easy
(b) simple
(c) luxurious
(d) meaningful.
Answer:
(d) meaningful.

Question 3.
What will we think if we see bottles of medicines in somebody’s house ?
Answer:
We will think that the man is probably sick.

Question 4.
What did the wise men of the past want to do?
Answer:
They wanted to make the life meaningful.

Question 5.
Real knowledge is to be found in ………….. itself.
Answer:
life.

Question 6.
Match the words in column A with their opposites in column B.

Α	B
sick	wrong
Right	healthy
	Read

Answer:
sick → healthy;
right → wrong.

Passage 7

People fight to settle disputes. Fighting means killing and civilized’ people ought to be able to find some way of settling their disputes? other than by seeing which side can kill the greater number of the other side, and then saying that the side which has killed most has won; and not only has won, but because it has won has been in the right. For that is what going to war means; it means saying that might is right. That is what the history of mankind on the whole has been like. Even our own age has fought the two greatest wars in history, in which millions of people were killed and mutilated.

And today while it is true that people don’t fight and kill each other in the streets, this is to say, we have got to the stage of keeping the rules or behaving properly towards each other in daily life, nations and countries have not learnt to do this yet and still behave like savages.

Question 1.
Civilized people do not indulge in …………
(a) killings
(b) parties
(c) donations
(d) gambling.
Answer:
(a) killings

Question 2.
Our age has fought two ………….. wars in history.
(a) recorded
(b) greatest
(c) atomic
(d) important.
Answer:
(b) greatest

Question 3.
What should civilized people do ?
Answer:
They should find some peaceful way to settle their disputes.

Question 4.
What does ‘might is right’ mean?
Answer:
It means that the person who wins thinks himself to be right.

Question 5.
Now people don’t fight and kill each other in ……….
Answer:
streets.

Question 6.
Match the words in column A with their meanings in column B.

A	B
dispute	treatment
behaviour	disagreement
	action

Answer:
dispute → disagreement;
behaviour → treatment.

Passage 8

Cheating in examinations is a major defectof our educational system. Cheating has become so common that students consider it their birthright to use unfair means in the examination. The root cause of this evil lies in our schools. Short-cuts have replaced hard labour. Guides and notes are encouraged in place of textbooks. In all school examinations, except the Middle and the Matric examinations, teachers show leniency3 to the students and pass most of them. Sometimes, even the parents are at fault. They get their undeserving wards promoted“ to the higher class. The students don’t cultivate the habit of self-study and hard work. Then in the Board Examination, students resort to copying. The teachers on duty encourage the students to use unfair means to pass the examinations. The desire to show good results motivates them to adopt wrong methods.

Question
1. Students consider it their ………….. to cheat in examinations.
(a) rule
(b) duty
(c) responsibility
(d) birthright.
Answers:
(d) birthright.

Question 2.
The teachers on duty …………. students to use unfair means.
(a) deny
(b) refuse
(c) encourage
(d) discourage.
Answers:
(c) encourage

Question 3.
What does the given passage call as the major defect?
Answers:
It calls cheating in examinations as the major defect.

Question 4.
How are the parents responsible for the evil of cheating?
Answers:
They want their undeserving wards promoted to their higher class.

Question 5.
The students don’t cultivate the habit of ………….. and …………. work.
Answers:
self-study, hard.

Question 6.
Match the words in column A with their meanings in column B.

A	B
major	defect
fault	leniency
	biggest

Answers:
major → biggest;
fault → defect.

Passage 9

Living on the earth is rather like being at the bottom of a sea hundreds of miles deep. Without atmosphere, there would be no people or animals, birds or fishes, trees or plants. There would be no weather, winds or rain. And there would be no blue sky, no rosy sunsets or dawns. Fire would be impossible without air, for burning is the union of oxygen with whatever is burned. Nor would there be any noise, which is the vibration? of air waves against our eardrums.

By day, the atmosphere serves as a great sunshade. It protects3 the earth from the full force of the sun by absorbing most of its harmful radiation4. But for the atmosphere, the daytime temperature would rise to 230 degrees Fahrenheit, hotter than boiling water. By night, the air acts like a giant greenhouse. It imprisons the heat collected during the day and prevents it from spreading into space.

Question 1.
Without …………., there would be no life on the earth.
(a) atmosphere
(b) gases
(c) sea
(d) weather.
Answer:
(a) atmosphere

Question 2.
By day, the atmosphere serves as a great ………….. .
(a) ball
(b) star
(c) sunshade
(d) vibration.
Answer:
(c) sunshade

Question 3.
What is living on the earth like ?
Answer:
It is like being at the bottom of a deep sea.

Question 4.
What does the atmosphere serve as ?
Answer:
It serves as a great sunshade.

Question 5.
By night, the air acts like a giant ……………
Answer:
greenhouse.

Question 6.
Match the words in column A with their opposites in column B.

A	B
bottom	enjoy
protect	top
	kill

Answer:
bottom → top;
protect → kill.

Passage 10

Subhash Chandra Bose was a great leader of India. He was born on 23rd January 1896. People called him ‘Netaji’ because he led them to the right path. He went to jail many times. Soon he found out that more efforts had to be made to make India free. The British power was getting weakened in the second world war. He thought of striking it from all sides. One day, he escapedfrom Kolkata in the guise of a Pathan and went to Germany. From there, he went to Japan. He organised the Indian National Army that fought many battles against the British armies. He said to his countrymen, “Give me blood and I will give you freedom.” At this appeal, hundreds of Indians abroad4 gave their all for the good of India. It was a bad day for India when Netaji died in an air crash. We shall always remember him as the greatest fighter for the freedom of India.

Question 1.
People called Subhash Chandra Bose as ………….. .
(a) Netaji
(b) great leader
(c) a revolutionary
(d) great guide.
Answer:
(a) Netaji

Question 2.
His army fought many battles against the ………….. army.
(a) British
(b) German
(c) Japanese
(d) foreign.
Answer:
(a) British

Question 3.
Why did the people call Bose as ‘Netaji’?
Answer:
Because he led them to the right path.

Question 4.
What did he organize ? Why?
Answer:
He organised Indian National Army to fight British armies.

Question 5.
His slogan was ‘Give me …………. and I will give you freedom.
Answer:
blood.

Question 6.
Match the words in column A with their meanings in column B.

A	B
effort	correct
right	endeavour
	up

Answer:
effort → endeavour;
right → correct.

Passage 11

Deserts are wild waste stretches of land, usually covered with sand, where little can grow and few can live. There are deserts in Africa, Asia, Australia and America, but the greatest and the most famous is the Sahara covering about 35 lakh square miles of northern Africa. It extends from the Mediterranean southward to the Sudan, and from the Red Sea westward to the Atlantic Ocean.

Crossing a desert has always been a risky adventure. Temperatures are very high during the day and may fall below zero at night. Going from one oasis! to another may be a march of several days. The lack of water, the fierce? rays of the sun, sandstorms and other hardships have always made journeys across such deserts as the Sahara the worst kind of travel in the world. The old tracks across the sand are lined with the bones of men and beasts that have met their fate. Horses and oxen are not suited to these conditions and the only animal that can be used is the camel, which has a peculiar stomach in which water can be stored. Besides being able to go for three days without drinking, it can also live for a long time on small quantities of food. Since ancient times, the camel has been called the ship of the desert’.

Question 1.
Deserts are wild waste …………. of land covered with sand.
(a) stretches
(b) spread
(c) spaces
(d) places.
Answer:
(a) stretches

Question 2.
Crossing a desert has always been a ………….. adventure.
(a) huge
(b) giant
(c) risky
(d) preferable.
Answer:
(c) risky

Question 3.
What is a desert ?
Answer:
A desert is a wild waste stretch of land covered with sand.

Question 4.
What is the camel called since ancient times ?
Answer:
It has been called the ship of the desert.

Question 5.
………….. is a place in the desert where some plants grow.
Answer:
Oasis.

Question 6.
Match the words in column A with their meanings in column B.

A	B
lack	shortage
beasts	vegetation
	animals

Answer:
lack → shortage;
beasts → animals.

Passage 12

In the modern times, with the coming of the motor cars and the aeroplanes, the desert may be said to have been conquered as far as travel is concerned. There are still special dangers for the car, including breakdowns, but an aeroplane can fly over a desert as easily as anywhere else. However the journey is made, wireless messages can be sent out if help is needed.

Yet in other ways, the desert has not yielded. It is the enemy and never the friend of man. Just as the sea, year after year, gradually eats away the land, so the sand of the desert, blown by the winds, advances yard by yard over the cultivated? fields. The remains of the old towns and villages, half-buried in the sand show that men once lived and grew their crops where there is no life today. One of the problems of the present century is how to stop this continuous extension4 of sandy wastes and save food-producing districts from destruction.

Question 1.
What modes of transport have been mentioned in the passage ?
(a) Motor cars.
(b) Aeroplanes.
(c) Both (a) & (b).
(d) Camels.
Answer:
(c) Both (a) & (b).

Question 2.
If help is needed, …………. message can be sent.
(a) telegraphic
(b) telephone
(c) wireless
(d) satellite.
Answer:
(c) wireless

Question 3.
What does the author think about the desert ?
Answer:
He thinks that the desert is an eneny which can never be a friend of man.

Question 4.
What is the problem of the present century ?
Answer:
It is how to stop the continuous extension of sandy wastes.

Question 5.
The desert is …………… the friend of man.
Answer:
never.

Question 6.
Match the words in column A with their opposites in column B.

А	B
modern	friend
foe	ancient
	enemy

Answer:
modern → ancient;
foe → friend.

Passage 13

In India, every boy and every girl now reading in schools is the future citizen of the country. Although he or she is not immediately called upon to vote or to make laws, he or she will have to do this in the near future. As the citizen of tomorrow, every young man should, therefore, prepare himself for the great task. The young citizen’s first duty is to learn how not to hurt- others, to be careful not to play unfairly, in the playing field, and not to speak a word which may give pain to anyone, not to make fun of others, or say things behind one’s back. He should not waste or throw away a grain4 of food, considering that there are many poor people in India who do not have enough to eat. Lastly, he should get training in the use of his leisure”.

Question 1.
Every …………. reading in schools in the future citizen of the country.
(a) boy
(b) girl
(c) both (a) and (b)
(d) child.
Answer:
(c) both (a) and (b)

Question 2.
We should not waste or throw a …………… of food.
(a) piece
(b) fragment
(c) grain
(d) lot.
Answer:
(c) grain

Question 3.
What is the great task of a citizen ?
Answer:
It is to lead a disciplined life.

Question 4.
Who is the future citizen of the country ?
Answer:
Every school-going boy and girl.

Question 5.
We should not waste or …………… away even a grain of food.
Answer:
throw.

Question 6.
Match the words in column A with their meanings in column B.

A	B
hurt	sufficient
enough	injure
	lack

Answer:
hurt → injure;
enough → sufficient.

Passage 14

Gandhiji’s mother was a very sweet, kind and religious woman. She visited the temple daily, often taking her little son with her. She fasted frequently2, too. Once she made a vow to eat only one meal a day for four months, and not to take even that one meal unless she had first seen sunshine. As she had made this vow in the rainy season, it was often difficult to see sunshine at all. Her children, who could not bear4 to think of their dear mother going without food all the twenty-four hours, would stand staring up at the sky waiting to catch the first gleams of the sun.

As soon as a ray appeared, they would dash? into the house and call their mother to come and see for herself. By the time she came out, the sun had often gone behind the clouds again. “It does not matter,” she would say cheerfully. “God does not want me to eat today,” and back she would go to her household tasks. In this way, Gandhiji learnt from his good mother how to do penance cheerfully for love of God.

Question 1.
Gandhiji’s mother was a very …………… woman.
(a) sweet
(b) kind
(c) religious
(d) all of the above.
Answer:
(d) all of the above.

Question 2.
Gandhiji’s mother made the vow in the …………. season.
(a) summer
(b) rainy
(c) pleasant
(d) rough.
Answer:
(b) rainy

Question 3.
What could the children not bear?
Answer:
They could not bear their mother going without food all the 24 hours.

Question 4.
What did Gandhiji learn from his mother?
Answer:
He learnt from his mother how to do penance cheerfully.

Question 5.
In the rainy season, it was often difficult to see …………… at all.
Answer:
sunshine.

Question 6.
Match the words in column A with their opposites in column B.

Α	B
sweet	cruel.
kind	noble
	bitter

Answer:
sweet → bitter;
kind → cruel.

Passages From Grammar Book (Fully Solved)

Passage 1

There are in our country, as in other countries of the world, thousands of differently abled persons, such as those who are blind or deaf and dumb. In some cases, these persons may have been born blind or deaf while in others, they may have gone blind or deaf as a result of some illness or accident.

You sometimes hear people say of such handicapped persons : “It is the work of fate.” or “It is the will of God.” Some even say, “They suffer the fruits’ of their own actions in the past.” Even the parents of differently abled children often express such feelings and opinions, and they scarcely ever think of how they can help these unfortunate ones. This certainly is not the way to look at the problems of the differently abled.
Whatever may be the cause of their suffering, we have got to treat the differently abled with sympathy and understanding. In many instances, physically challenged children suffer neglect and are left to themselves in their homes.

This makes their life extremely sad and lonely3. Our first duty is to make these children happier and less lonely by engaging them in different activities suitable for them. Secondly, we have got to educate these children and help them to live meaningful lives. We should secure“ benefits of education for them in schools intended for them. We ought to make them self-reliant by creating suitable opportunities for them. They will then have a sense of achievement. We will also feel satisfied that we have done our duty towards them.

Question 1.
How should we treat the differently abled children ?
(a) Indifferently.
(b) With sympathy and understanding.
(c) By ignoring their loneliness.
(d) By neglecting them.
Answer:
(b) With sympathy and understanding.

Question 2.
According to the author, what is our first duty towards handicapped children ?
(a) To make them homeless.
(b) To make them healthy.
(c) To make them happier and less lonely.
(d) To make them run fast.
Answer:
(c) To make them happier and less lonely.

Question 3.
How do some children become differently abled ?
Answer:
Some of them may be born handicapped, e.g. deaf, dumb, etc. Others may become handicapped later due to some illness or accident.

Question 4.
What makes the life of handicapped children sad and lonely?
Answer:
The handicapped children start feeling sad and lonely when they have to suffer neglect and are left to their lot in their homes.

Question 5.
Fill in the blank with a suitable word from the passage.
We must ……… these children with love and sympathy.
Answer:
treat.

Question 6.
Match the words under column A with their meanings in column B:

A	B
sympathy	sad
unhappy	nearly
	feeling pity and tenderness

Answer:
sympathy → feeling pity and tenderness;
unhappy → sad.

Passage 2

In a reversal of the norm elsewhere, in India policy-makers and economists have become optimists’ while bosses do the worrying. The country’s Central Bank has predicted that India’s economy is likely to grow at a double-digit rate during the next 20-30 years. India has the capability with its vast labour and lauded? entrepreneurial spirit. But the private sector which is supposed to do the heavy lifting that turns India from the tenth largest economy to the third largest by 2030, has become fed up.

Business people often crib about India’s problems, but their irritation this time has a nervous edge. In the first quarter of 2011, GDP grew at an annual rate of 7.8 per cent; in 2015-17, it managed 6-7 per cent. The economy may be slowing naturally as the low interest rates and public spending that got India through the global crisis are belatedly withdrawn. At the same time, the surge in inflation caused by exorbitant food prices has spread more widely, casting doubt over whether India can grow at 8-10 per cent in the medium term without overheating.

Question 1.
What rate of growth does the Central Bank predict for the Indian economy for the next 20-30 years ?
(a) Eight per cent.
(b) Seven per cent.
(c) Double-digit per cent.
(d) Five per cent.
Answer:
(c) Double-digit per cent.

Question 2.
Who is supposed to do the heavy lifting to turn India into third largest economy ?
(a) Govt. agencies.
(b) Private sector.
(c) Public sector.
(d) Property owners.
Answer:
(b) Private sector.

Question 3.
What is India’s capability to grow based on ?
Answer:
It is based on our large labour force and the entrepreneurial spirit of our people.

Question 4.
What is casting doubts over India’s growth rate ?
Answer:
Two main reasons for the doubt are (i) withdrawal of low interest rates and public spending and (ii) a surge in inflation brought about by extremely high food prices.

Question 5.
Fill in the blank with a suitable word from the passage.
He suffered from a ……… breakdown.
Answer:
nervous.

Question 6.
Match the words under column A with their meanings in column B:

A	B
crisis	large
optimist	difficult phase
	one who looks forward to a positive outcome

Answer:
crisis → difficult phase;
optimist → one who looks forward to a positive outcome.

Passage 3

Brain drain, also referred to as human capital flight, is the action of having highly skilled and educated people leaving their country to work abroad. It has actually become one of the most serious concerns’ for the developing nations. While many people believe that immigration is a personal choice that must be understood and respected, others look at this phenomenon from a different perspective?. What makes those people leave their country, their own people, should be seriously considered and a distinction between pull and push factors must be made. The push factors include low wages and a lack of satisfactory working and living conditions.

Social unrest, political conflicts and wars may also be the determining causes. The pull factors, however, include intellectual freedom and substantial funds for research. Brain drain has a negative impact on the economic prospects and competitiveness of sender countries. It reduces the number of dynamic and creative people who can contribute to the development of their country. Likewise, with more entrepreneurs taking their investments abroad, developing countries are missing the opportunity of wealth creation.

Question 1.
The term “brain drain’ is also referred to as:
(a) Capital flight
(b)Human capital flight
(c) Pull factors
(d) Push factors.
Answer:
(b)Human capital flight

Question 2.
Brain drain has terrible consequences on the economic development of:
(a) Sending countries
(b) Receiving countries
(c) Developed countries
(d) Undeveloped countries.
Answer:
(a) Sending countries

Question 3.
What do you mean by the term ‘brain drain’ ?
Answer:
‘Brain drain’ is the name given to the activity of the skilled people of a country migrating to foreign countries for better prospects.

Question 4.
Enumerate the push factors that lead to brain drain.
Answer:
The push factors behind ‘brain drain’ are low wages and a lack of satisfactory working and living conditions in the sending countries.

Question 5.
Fill in the blank with a suitable word from the passage.
Every citizen must ……. to the development of the nation wholeheartedly.
Answer:
contribure.

Question 6.
Match the words under column A with their meanings in column B:

A	B
conflicts	decrease
reduce	disputes
	increase

Answer:
conflicts → disputes;
reduce → decrease.

Passage 4

One night a man came to our house and told me, “There is a family with eight children. They have not eaten for days.” I took some food and went out. When I finally came to the family, I saw the faces of those little children disfigured’ by hunger. There was no sorrow or sadness in their faces, just the deep pain of hunger. I gave the rice to the mother. She divided it into two and went out, carrying half the rice with her. When she came back, I: asked her, “Where did you go ?” She gave me this simple answer, “To my neighbour, they are also hungry.” I was not surprised because poor people are generous but I was surprised that she knew they were hungry.

As a rule, when we are suffering, we are so focused on ourselves, we have no time for others. We become selfish and self-centred. Having experienced the pangs“ of sufferings, we should rather extend a helping hand to the poor and the needy.

Question 1.
The faces of the children reflected:
(a) sorrow
(b) joy
(c) hunger
(d) greediness.
Answer:
(c) hunger

Question 2.
The action of the mother shows:
(a) selfishness
(b) hatred
(c) gratitude
(d) love.
Answer:
(d) love.

Question 3.
Where did the mother go after dividing the rice and why ?
Answer:
She went to her neighbour’s house. She went there to share rice with them. She knew they were hungry like her and also needed food like her.

Question 4.
Why was the gentleman surprised ?
Answer:
The man knew that the poor people are generous, but he was surprised to find that the woman knew for certain that her neighbour’s family was also hungry like her own family. Normally, the people remain selfcentred.

Question 5.
Fill in the blank with a suitable word from the passage.
The faces of the little children were ………
Answer:
disfigured.

Question 6.
Match the words under column A with their opposites under column B:

A	B
simple	complicated
generous	hatred
	selfish

Answer:
simple → complicated;
generous → selfish.

Passage 5

Health and hygiene go hand in hand. Health refers to a state of sound mind and physically fit body, free from any form of sickness, disorder or ailment’. Hygiene refers to the good practices that prevent disease and lead to good health through cleanliness, proper sewage? disposal, balanced and nutritious food, regular exercise, proper sleep, pure and fresh air and supply of safe drinking water. The proverb, ‘Health is Wealth’, is truly said of all things in the world. Health is the most valuable thing that one can possess.

Money is undoubtedly a prized’ possession, but can it provide pleasure to a ruined health? As body and mind are closely related, the mind can never be sound and cheerful without sound health. An unhealthy man may have intelligence, merit and wealth, but he cannot put them to use and reap their benefits. We must, therefore, adopt proper hygienic measures to preserve and maintain good health. Too much work or exercise, eating or drinking are injurious to health. A regulated life coupled with clear and pure mind makes life worth living.

Question 1.
Hygiene refers to practices that lead to good health through:
(a) balanced diet
(b) impure air
(c) abundant wealth
(d) regular play.
Answer:
(a) balanced diet

Question 2.
Life can become worth living through:
(a) gambling and drinking
(b) regulated life
(c) pure mind
(d) healthy body.
Answer:
(b) regulated life

Question 3.
What does the term ‘health refer to ?
Answer:
Health refers to a state of sound mind and a body that is physically fit and free from sickness, disorder or ailment.

Question 4.
Can a wealthy but unhealthy man enjoy life?
Answer:
No, an unhealthy man cannot enjoy life because nothing can provide pleasure to a man with ruined health. And without sound health, man cannot have a sound mind also.

Question 5.
Fill in the blank with suitable words from the passage.
The selection in Army service is made purely on ……… .
Answer:
the soundness of both body and mind.

Question 6.
Match the words under column A with their opposites under column B:

A	B
fresh	use
preserve	stale
	destroy

Answer:
fresh → stale;
preserve → destroy.

Passage 6

Named after former Prime Minister, Inder Kumar Gujral’s mother, Pushpa Gujral Science City located on Jalandhar-Kapurthala Road, just west of Jalandhar, is extremely thrilling. Almost every branch of science is exhibited, right from physical, applied, natural and social sciences to health sciences, human evolution and civilization, engineering, technology, agriculture, the environment, ecosystems and Jurassic Park. In the Dome Theatre, large-format films are projected on 23-metre tilted dome. Semi-circular giant dome screen produces huge images that soar and swoop above, beside and behind you, to give you a spectacular, immiscible? experience.

The Light-Speed 3-D Digital Theatre presents three-dimensional computer graphics, videos and the most advanced animation. Amazing Living Machine Gallery presents the intricate structures and functions of the human body using large human models. The Flight Simulator provides the visitors a sense of adventure. The Laser Theatre presents laser shows that carry the visitors to a wonderland to experience a mind-boggling mix of sound and laser beams. The Dinosaur Park displays the evolution of dinosaurs and the probable reasons for their extinction”. There is also a kids’ park containing tunnels, rides, bouncers, etc. and an artificial lake that allows the visitors to indulge in boating. With all the wonderful attractions, a visit to the Science City would be highly informative and enjoyable.

Question 1.
Which facility provides a sense of adventure ?
(a) Kids’ Park.
(b) Dome Theatre.
(c) Flight Simulator.
(d) Laser Theatre.
Answer:
(c) Flight Simulator.

Question 2.
Large human models are found in:
(a) Amazing Living Machine Gallery
(b) Dinosaur Park
(c) Digital Theatre
(d) Laser Theatre.
Answer:
(a) Amazing Living Machine Gallery

Question 3.
Where is the Pushpa Gujral Science City located ?
Answer:
It is located on Jalandhar-Kapurthala Road, just west of Jalandhar.

Question 4.
What is displayed in the Dinosaur Park ?
Answer:
The Dinosaur Park displays the evolution of dinosaurs and the likely reasons of their extinction.

Question 5.
Fill in the blank with a suitable word from the passage.
The works of famous painters are ……… in the Tagore Art Gallery.
Answer:
exhibited.

Question 6.
Match the words under column A with their meanings under column B:

A	B
spectacular	huge
giant	amazing

Answer:
spectacular – amazing;
giant – huge.

Passages For Practice

Passage 1

Democratic societies from the earliest times have expected their governments to protect the weak from the strong. No ‘era of good feeling’ can justify discharging the police force or giving up the idea of public control over concentrated private wealth. On the other hand, it is obvious that a spirit of self-deniall and moderation? on the part of those who hold economic power, will greatly soften the demand for absolute equality. Men are more interested in freedom and security than in an equal distribution of wealth. The extent to which the government must interfere with business, therefore, is not exactly measured by the extent to which economic power is concentrated into a few hands. The required degree of government interference depends mainly on whether economic powers are oppressively used and on the necessity of keeping economic factors in a tolerable state of balance.

The powers of government are unavoidably increased, whichever political party may be in office. The growth of government is a necessary result of the growth of technology and of the problems that go with the use of machines and science. Since the government, in our nation, must take on more powers to meet its problems, there is no way to preserveo freedom except by making democracy more powerful.

Question 1.
Democratic societies from the earliest times have expected their governments to ……… the weak from the strong.
(a) protect
(b) differentiate
(c) separate
(d) preserve.

Question 2.
Two things in which men are more interested than in equal distribution of wealth
are (a) freedom and power
(b) freedom and security
(c) power and security
(d) power and insecurity

Question 3.
What do you mean by the growth of government ?

Question 4.
How can we justify ‘era of good feeling’ ?

Question 5.
The growth of ………… is the necessary result of the growth of ……….. .

Question 6.
Match the words in column A with their meanings in column B.

Α	B
growth	bear
tolerate	decline
	development

Passage 2

In today’s world, everybody talks much about his rights. There is a great hue and cry if our rights are infringed?. But nobody seems to bother much about his duties. That is why there is a great unrest in our present-day life. Actually duties come first, and rights afterwards.

Many a time, one man’s right is another man’s duty and vice versa4. For example, every man has the right to have an undisturbed sleep. So it becomes the duty of his neighbour not to tune his radio at too high a pitch. If we want to enjoy our rights, we should act in such a way that the rights of others are not trespassed. It can happen only if we take due account of our duties also. In short, rights and duties are complementary things and not contradictory?

It is difficult to agree on which rights should be guaranteed to a citizen. For example, does a child have the right to be educated in his / her mother tongue ? Some enthusiasts may say ‘certainly’. But others may say that while everyone must have a right to education, the government should not be forced to spend money to employ special teachers for the language of every group and community. Take another example. Does every adult have a right to job? Some will say ‘yes’. Others will disagree and say that this is a privilege’, not a right. They say that in these days of population explosion, it is not possible for any government to ensure10 full employment.

Question
1. There is a great unrest in present-day life because ………….
(a) everybody wants to enjoy his rights
(b) nobody wants to bother about his duties
(c) both (a) and (b)
(d) neither (a) nor (b).

Question 2.
We should act in such a way that other’s ……… .
(a) rights are trespassed
(b) duties are trespassed
(c) rights are not trespassed
(d) duties are not trespassed.

Question 3.
What argument can be given against the right of every adult to have a job?

Question 4.
Give one example from the passage to show that one man’s right is another man’s duty.

Question 5.
It is not ………. for any government to ……… full employment.

Question 6.
Match the words in column A with their meanings in column B.

Α	B
encroached	infringed
violated	allowed
	trespassed

Passage 3

1. Summer vacation offers families dilemmas and opportunities. For too many kids, it becomes a period of intellectual passivity and stalled personal growth. For others and their parents – it’s a time of overload and frantic scheduling.

2. “Summer is a great time for parents to build relationship with their children,” says a renowned child psychologist. And it’s an opportunity both for the kids to learn and for the family to grow together. To make this a reality, educators and psychologists point to several simple strategies that parents can start planning before summer gets underway.

3. “Summer’s a perfect time for kids to test the skills they’ve learnt in a classroom and use them in new ways,” notes a well-known educator. Comparing prices in a grocery shop can sharpen children’s mental maths skills. Taking measurements to build a new tree house or design a simple plaything teaches geometry. Car trips provide opportunities to study maps and learn geography. Some libraries offer free summer reading programmes for children. “It’s the daily doses of stimulation? intellectual, creative, esteembuilding – that parents can give their children that have the greatest impact,” says an eminent researcher.

Question 1.
Parents can give their kids a special summer vacation by ……….
(a) building relationship with them
(b) providing them opportunities to use their skills
(c) giving them daily doses of stimulation
(d) all of the three.

Question 2.
………. is a great time for parents to build relationship with their children.
(a) Spring
(b) Summer
(c) Winter
(d) Autumn

Question 3.
Mention the three ways in which children can use the skills learnt in class.

Question 4.
What do car trips provide ?

Question 5.
To test the ……… children have learnt in their classroom ………. is a perfect time.

Question 6.
Match the words in column A with their meanings in column B.

Α	B
stimulation	talent
skill	passion
	encouragement

Passage 4

1. The Egyptian mummies have always remained a fascination for all. The method of embalming? or treating the dead body, that the ancient Egyptians used, is called mummification. Using special processes, they removed all moisture from the dead body, leaving only a dried form that would not easily decay. It was an important Egyptian religious belief to preserve the dead body in as lifelike a manner as possible.

2. The first step in the process was the removal of all internal parts that might decay rapidly. The brain was removed by carefully inserting special hooked instruments up through the nostrils. It was a delicate operation, one which could easily disfigure the face. The embalmers then removed the organs of the abdomen and chest through a cut usually made on the left side of the abdomen. They left only the heart in place, believing it to be the centre of a person’s being and intelligence. The other organs were preserved separately, with the stomach, liver, lungs, and intestines placed in special boxes or jars. Such jars are today called the canopic jars.

3. The embalmers next removed all moisture from the body. This they did by covering the body with natron, a type of salt which has great drying properties, and by placing additional natron packets inside the body.

Question 1.
Mummification is a process by which a dead body is ………..
(a) destroyed
(b) preserved
(c) mutilated
(d) cremated.

Question 2.
The process of mummification was followed by ancient ………….
(a) Egyptians
(b) Aryans
(c) Europeans
(d) Negroes.

Question 3.
What was done with the internal parts of the body and why ?

Question 4.
What was done with those organs that were removed from the dead body ?

Question 5.
The method of ………. or treating the dead body is called mummification.

Question 6.
Match the words in column A with their meanings in column B.

A	B
disfigure	dislocate
sunken	hollow
	mutilate

Passage 5

1. Trees are the most useful gift of nature to man. Without trees, it would be a very bleak? world. Without them, life would be impossible. Now let’s see what trees do for us.
2. Men in olden days used wood for making their homes, rafts?, canoes3 and weapons. They used it as fuel to cook and to keep themselves warm.

3. In addition to wood, man was dependent on trees for many other things also. He got from them fruits and nuts for his food. Leaves of the palm and other trees were used for thatching roofs. The bark and the leaves of trees were used for clothing. Utensils were made from calabashes, coconuts and the shells of other fruits. A number of medicines, dyes, tanning materials and species were obtained from trees.

4. In present times also, man is no less dependent on trees. He has, no doubt, invented many things that can take the place of wood. He has begun to use concrete, steel, glass and plastics in place of wood. But even then, the demand for wood has increased vastly. For example, we
need lots and lots of wood for making paper, cardboard and packing cases.

5. Trees are invaluable for other reason also. There is oxygen in the air, but it is being constantly used up and turned into carbon dioxide. When animals breathe and things burn, oxygen is consumed and carbon dioxide is produced. The green leaves of trees absorb this carbon dioxide. With the help of sunlight, they break it into carbon and oxygen. The carbon is used by green leaves to make starch. The oxygen is released back into the air.

Question 1.
If there were no trees, no ………. would be possible on the earth.
(a) life
(b) death
(c) nature
(d) calamity.

Question 2.
Utensils were made from ……….
(a) calabashes
(b) coconuts
(c) shells of other fruits
(d) all of these three.

Question 3.
How did men in olden days make use of trees ?

Question 4.
How do trees treat the carbon dioxide produced by us ?

Question 5.
For making paper, cardboard and packing cases, we need ………….

Question 6.
Match the words in column A with their meanings in column B.

A	B
invaluable	priceless
bleak	fragile
	miserable

Passage 6

1. Grammar can be a way of comparing different languages. To most people, grammar is a set ef rules for speaking and writing a language correctly. Usually, before you can speak any language well – even your own language which you have gradually been learning since you were little – you have to know something about its grammar. Small children start to pick up the grammar of their own language almost by instinct, by hearing how their parents talk and by seeing how words were put together in sentences in the books they read. Before long, they learn that some expressions sound wrong, or are ‘bad grammar’, such as ‘they looked at he’ instead of what it should be, they looked at him? or ‘the boys fighted for a hour’ instead of the boys fought for an hour’. By being exposed to the language over a period of time, they eventually know how to say the right things and avoid? saying the wrong things in order to be understood.

2. However, when children begin to learn a foreign language, they find that they have to set about deliberately3 learning its grammar rules by heart. It is not simple enough to know the words of a new language, or a person could learn it just by reading a dictionary. The words by themselves do not mean very much until they are fitted together to form sentences, and it is grammar that shows how to do this.

Question 1.
To be able to learn a language well, we have to learn something about its ………
(a) pronunciation
(b) rules
(c) diction
(d) grammar.

Question 2.
The words do not mean very much until they are arranged in order to form ………
(a) tenses
(b) narration
(c) sentences
(d) voice.

Question 3.
How are even small children able to distinguish a wrong expression from a correct one ?

Question 4.
How does grammar help in learning a foreign language ?

Question 5.
………. can be a way of comparing different languages.

Question 6.
Match the words in column A with their antonyms in column B.

A	B
different	carelessly
deliberately	similar
	intentionally

Passage 7

Gandhiji does not reject machinery as such. He observes “How can I be against all machinery, when I know that even this body is the most delicatel piece of machinery ? The spinning wheel is a machine, a little toothpick is a machine. What I object to is the craze? for machinery, not machinery as such. The craze is for what they call labour-saving machinery. Men go on ‘saving labour’ till thousands are without work and thrown on the open streets to die of starvation.

I want to save time and labour, not for a fraction of mankind, but for all. I want the collection of wealth, not in the hands of a few, but in the hands of all. Today, machinery merely helps a few to ride on the back of millions. The impetus behind it all is not philanthropys to save labour, but greed. It is against this constitution of things that I am fighting with all my might. The machine should not tend to atrophy the limbs of man. Factories run by power-driven machinery should be nationalized, state-controlled. The supreme consideration is man.”

Question 1.
Gandhiji objects to the ………. for machinery, not machinery itself.
(a) craze
(b) interest
(c) intention
(d) want.

Question 2.
The phrase ‘thrown on the open streets’ means ………..
(a) employed people
(b) enslaved people
(c) unemployed people
(d) freed people.

Question 3.
What is it about machinery that Gandhiji really objects to ?

Question 4.
Machines save time and labour, not for the poor worker, but for the rich factory owner. Explain how it happens.

Question 5.
Gandhiji does not want factories run by ……… machinery to be ……….

Question 6.
Match the words in column A with their meanings in column B.

A	B
craze	credit
impetus	stimulus
	fad

Punjab State Board PSEB 11th Class English Book Solutions English Grammar Translation Exercise Questions and Answers, Notes.

PSEB 11th Class English Grammar Translation

1. Present Indefinite Tense (V₁ + s / es)

1. मैं गीत गाता हूं। – I sing a song.
2. हम परिश्रम करते हैं। – We work hard.
3. आप झूठ नहीं बोलते हैं। – You do not tell lies.
4. में कक्षा में शोर नहीं मचाता हूं। – I do not make a noise in the class.
5. क्या गुरमीत आपकी सहायता करता है ? – Does Gurmeet help you ?
6. क्या बच्चे आंख-मिचौली खेलते हैं ? – Do the children play hide and seek ?
7. आप क्या चाहते हैं ? – What do you want ?
8. क्या आप अपना समय बर्बाद नहीं करते हैं ? – Do you not waste your time ?

Exercise

Translate into English:
1. राम सदा सवेरे उठता है।
2. आजकल उसका मुश्किल से गुज़ारा होता है।
3. पक्षी हवा में उड़ते हैं।
4. मेरी घड़ी ठीक समय नहीं देती है।
5. हम अपने देश की सेवा करते हैं।
6. क्या बच्चे का आधा टिकट नहीं लगता है ?
7. वह दुकानदार कम तोलता है।
8. यह सड़क अमृतसर को जाती है।
9. मैं तुम्हें नहीं जानता हूं।
10. क्या वह लिखना जानता है ?
Hints:
1. gets up early;
2. hardly makes both ends meet;
4. correct time;
5. serve;
6. travel half fare;
7. gives a short measure;
8. leads to.

2. Past Indefinite Tense (V₂)

1. ज़रूरत के समय उसने मेरी मदद की। – He helped me in need.
2. आपने डॉक्टर को बुलाया। – You sent for the doctor.
3. हमने आपको धोखा नहीं दिया। – We did not deceive you.
4. लड़कियों ने गीत नहीं गाया। – The girls did not sing a song.
5. क्या अध्यापक ने नया पाठ पढ़ाया ? – Did the teacher teach a new lesson ?
6. क्या आप कल झील पर नहीं गए ? – Did you not go to the lake yesterday ?
7. क्या गुरदीप ने हरजीत को कोई सूचना नहीं दी ? – Did Gurdeep not give any information to Harjeet ?
8. तुमने झूठ क्यों बोला ? – Why did you tell a lie ?

Exercise

Translate into English:
1. क्या विद्यार्थियों ने परीक्षा दी ?
2. वे मैच हार गए।
3. मेरे भाई ने मुझे दस रुपये का नोट दिया।
4. मैंने उसे पांच रुपए उधार दिएं।
5. क्या सुमन ने छात्रवृत्ति प्राप्त नहीं की ?
6. सिपाही को देखकर चोर सिर पर पांव रखकर भागा।
7. क्या उसने मेरी स्लेट तोड़ी ?
8. मैंने उसे चिट्ठी लिखी।
9. हम समय पर स्टेशन पहुंच गए।
10. वह मुझे प्रतिदिन मिला करता था।
Hints:
1. take the test;
2. lost;
3. a ten-rupee note;
4. lent;
5. scholarship;
6. took to heels;
7. break.

3. Future Indefinite Tense (will / shall + V₁)

1. आप अच्छे लड़कों से मित्रता करोगे। – You will make friends with good boys.
2. हम मन लगा कर पढ़ेंगे। – We shall study whole-heartedly.
3. आप यह काम समाप्त नहीं करोगे। – You will not finish this work.
4. मैं स्वार्थी मित्रों का विश्वास नहीं करूंगा। – I shall not trust the selfish friends.
5. क्या हम अपने देश की सेवा करेंगे? – Shall we serve our country ?
6. वह आपकी फीस कब देगा? – When will he pay your fees ?
7. क्या आपके पिता जी बैठक की अध्यक्षता नहीं करेंगे? – Will your father not preside over the meeting?
8. क्या राम वहां तीन दिन नहीं ठहरेगा? – Will Ram not stay there for three days ?

Exercise

Translate into English:
1. मैं नई घड़ी खरीदूंगा।
2. वहां मैं दिल लगाकर पढूंगा।
3. नाव डूब जाएगी।
4. राम अच्छे अंक लेकर पास होगा।
5. मैं उसे बीस रुपए उधार दूंगा।
6. वह अपनी प्रतिज्ञा पूरी करेगा।
7. स्कूल दीवाली के कारण बन्द रहेगा।
8. क्या रोगी कुछ दिनों में अच्छा हो जाएगा ?
Hints:
2. whole-heartedly;
3. sink;
6. keep word;
7. on account of.

4. Present Continuous Tense (is / are / am + V_1+ing)

1. मेरा सिर चकरा रहा है। – I am feeling giddy.
2. बूंदा-बांदी हो रही है। – It is drizzling.
3. आकाश में तारे नहीं चमक रहे हैं। – The stars are not shining in the sky.
4. सीता पूरा प्रयत्न नहीं कर रही है। – Sita is not doing her best.
5. क्या वह अपने बाल संवार रही है? – Is she combing her hair ?
6. क्या सारे बच्चे तालियां बजा रहे हैं? – Are all children clapping ?
7. क्या वह मुझे धोखा नहीं दे रहा है? – Is he not deceiving me ?
8. तुम चिल्ला क्यों रहे हो? – Why are you shouting ?

Exercise

Translate into English:
1. क्या आप यह मकान छोड़ रहे हैं ?
2. बाग में फ़व्वारे चल रहे हैं ?
3. मैं आपकी ही प्रतीक्षा कर रहा हूं।
4. आज ठण्डी-ठण्डी हवा चल रही है।
5. मज़दूर कुआं खोद रहे हैं।
6. बाहर बूंदाबांदी हो रही है।
7. क्या बच्चे भिखारी की हंसी उड़ा रहे हैं ?
8. शीला हारमोनियम बजा रही है।
Hints:
1. leaving;
2. playing;
3. waiting for;
6. drizzling;
7. making fun of;
8. playing on.

5. Past Continuous Tense (was / were + V_1+ing)

1. मैं अपना जूता पालिश कर रहा था। – I was polishing my shoe.
2. वह ज़ोर-ज़ोर से बोल रही थी। – She was talking loudly.
3. कुम्हार नए बर्तन नहीं बना रहा था। – The potter was not making new pots.
4. आकाश में बादल नहीं गरज रहे थे। – The clouds were not thundering in the sky.
5. क्या वह अपना भोजन नहीं कर रही थी? – Was she not taking her meals ?
6. क्या वे पागल कुत्ते के पीछे नहीं दौड़ रहे थे? – Were they not running after the mad dog ?
7. क्या माली पौधों को पानी दे रहा था? – Was the gardener watering the plants ?
8. नौकर कमरे में झाडू क्यों नहीं लगा रहा था? – Why was the servant not sweeping the room ?

Exercise

Translate into English:
1. हवा तेजी से चल रही थी।
2. वे समाचार-पत्र पढ़ रहे थे।
3. आकाश में बादल गरज रहे थे।
4. क्या वह झूठा बहाना बना रहा था।
5. लोग उसे बधाई पर बधाई दे रहे थे।
6. लड़के भिखारी का मज़ाक उड़ा रहे थे।
7. क्या तुम उसे तंग कर रहे थे ?
8. मोहन शतरंज खेल रहा था।
Hints:
1. blowing hard;
3. thundering;
4. lame excuse;
5. congratulations;
6. laughing at;
7. teasing;
8. playing at chess.

6. Future Continuous Tense (will / shall + be + V_1+ing)

1. मैं उसका विरोध कर रहा हूंगा। – I shall be opposing him.
2. मदारी अपना खेल दिखा रहा होगा। – The juggler will be showing his tricks.
3. हम हॉकी का मैच देखने नहीं जा रहे होंगे। – We shall not be going to see the hockey match.
4. आपका नौकर बिस्तर नहीं लगा रहा होगा। – Your servant will not be making the bed.
5. क्या वह भाषण दे रहा होगा? – Will he be making a speech ?
6. क्या मैं उसे व्यर्थ के बहाने बना कर टाल रहा हूँगा? – Shall I be putting him off with lame excuses ?
7. क्या बच्चा सिसकियां नहीं भर रहा होगा? – Will the child not be sobbing ?
8. तुम्हारे मित्र तुम्हारी भलाई क्यों नहीं चाह रहे होंगे? – Why will your friends not be wishing you well ?

Exercise

Translate into English:
1. कमला इस समय सो रही होगी।
2. मेरा भाई आपकी प्रतीक्षा कर रहा होगा।
3. दादी बच्चों को कहानी सुना रही होगी।
4. गड़ेरिया भेड़ें नहीं चरा रहा होगा।
5. वे गाड़ी में यात्रा कर रहे होंगे।
6. वह कक्षा में ऊँघ रहा होगा।
7. श्याम अपने कमरे में होगा।
8. नल चल रहा होगा।
9. लड़का पशु चरा रहा होगा।
10. आप भाषण दे रहे होंगे।
Hints:
3. grandmother;
4. shepherd;
6. doze;
8. tap will be running.

7. Present Perfect Tense (has / have + V₃)

1. मेरे भाई ने अपना काम समाप्त कर लिया है। – My brother has finished his work.
2. किसानों ने फसलें काट ली हैं। – The farmers have reaped the crops.
3. मैंने आपकी सलाह नहीं मानी है। – I have not acted upon your advice.
4. आपने अपना वचन पूरा नहीं किया है। – You have not kept your word.
5. क्या आपको जुकाम नहीं हो गया है ? – Have you not caught cold ?
6. क्या उसने सिगरेट पीना नहीं छोड़ दिया है? – Has he not given up smoking ?
7. क्या गाड़ी आ गई है? – Has the train steamed in ?
8. अंग्रेज़ी में कौन प्रथम आया है? – Who has stood first in English ?

Exercise

Translate into English:
1. मैंने जीवन में बड़े उतार-चढ़ाव देखे हैं।
2. धन-दौलत ने उसका सिर फेर दिया है।
3. बरसात का मौसम शुरू हो गया है।
4. उन्होंने नगर छोड़ दिया है।
5. क्या तुमने कभी शेर देखा है ?
6. क्या आपकी घड़ी बन्द नहीं हो गई है ?
7. आकाश में सितारे निकल आए हैं।
8. उसके टखने में मोच आ गई है।
9. उसने काम नहीं किया है।
10. क्या हमने आपकी सलाह नहीं मानी है ?
Hints:
1. ups and downs;
2. riches, turned;
3. set in;
7. appeared;
8. sprained.

8. Past Perfect Tense (had + V₃)

1. मैं पहले ही अपना काम समाप्त कर चुका था। – I had already finished my work.
2. उसके स्कूल पहुंचने से पहले ही घण्टी बज चुकी थी। – The bell had gone before he reached the school.
3. जब मैं स्टेशन पर पहुंचा तो गाड़ी जा चुकी थी। – The train had left when I reached the station.
4. मोहन ने कल तक अपना पाठ याद नहीं किया था। – Mohan had not learnt his lesson till yesterday.
5. सारे सदस्यों के आने से पहले प्रस्ताव पास नहीं – The resolution had not been passed before all the members came.
6. रोगी अभी मरा नहीं था। – The patient had not died yet.
7. क्या आप पहले कभी आगरा गए थे ? – Had you ever been to Agra before ?
8. क्या तुम्हारे बस स्टैंड पर पहुँचने से। – Had the bus not left before you.
9. पहले बस जा नहीं चुकी थी ? – reached the bus stand ?

Exercise

Translate into English:
1. डॉक्टर के आने से पहले रोगी मर चुका था।
2. मैंने कभी सूअर नहीं देखा था।
3. उसने पिछले वर्ष मैट्रिक की परीक्षा नहीं दी थी।
4. मैं पहले ही खाना खा चुका था।
5. हम उसे उसकी शादी के बाद नहीं मिले थे।
6. पुलिस के पहुंचने से पहले चोर भाग चुके थे।
7. मैं तो पहले ही इस काम से निपट चुका था।
8. आपके आने से पहले सारी तैयारी कर ली गई थी।
Hints:
4. had had the meal;
7. had finished;
8. all preparations had been made.

9. Future Perfect Tense (will / shall + have + V₂)

1. आप सूर्य अस्त होने तक लौट चुके होंगे। – You will have returned by sunset.
2. गाड़ी अब तक आ चुकी होगी। – The train will have arrived by now.
3. रात होने से पहले वे शहर पहुंच गए होंगे। – They will have reached the city before the night falls.
4. सुबह होने तक मैं सवाल हल कर चुका हूंगा। – I shall have solved the sums before the day breaks.
5. आपके आने से पहले मैं तस्वीर पूरी नहीं कर चुका हूँगा। – I shall not have completed the picture before you come.
6. चार बजे तक हम सौ दौड़ें नहीं बना चुके होंगे। – We shall not have scored one hundred runs by four o’clock.
7. क्या दवाई लेने से पहले रोगी मर चुका होगा? – Will the patient have died before taking चुका होगा? the medicine ?
8. क्या अगले महीने तक मुझे आराम आ चुका होगा? – Shall I have recovered by next month ?

Exercise

Translate into English:
1. प्रातः होने से पूर्व माली पौधों को पानी दे चुका होगा।
2. छ: बजने से पहले मैं डॉक्टर को टेलीफोन कर चुका हूंगा।
3. वह अक्तूबर तक नौकरी छोड़ चुका होगा।
4. वह अब तक बर्तन साफ कर चुकी होगी।
5. क्या आपके सभा में पहुंचने से पहले वक्ता भाषण नहीं दे चुके होंगे ?
6. लड़ाई खत्म होने से पहले हज़ारों लोग मर चुके होंगे।
7. तुम्हारे उच्च न्यायालय में पहुंचने से पहले न्यायाधीश अपना फैसला सुना चुका होगा।
8. उसने अभी तक पाठ्यक्रम खत्म नहीं किया होगा।
Hints:
2. shall have rung up;
8. syllabus.

10. Present Perfect Continuous Tense (has / have / been + V_1+ing)

1. दो दिन से लगातार वर्षा हो रही है। – It has been raining continuously for two days.
2. लड़के सुबह से तैर रहे हैं। – The boys have been swimming since morning.
3. वे 1990 से यहां नहीं रह रहे हैं। – They have not been living here since 1990.
4. रजनी कई दिनों से स्कूल नहीं आ रही है।। – Rajni has not been coming to school for many days.
5. क्या वह पिछले दो सालों से गुम है? – Has he been missing for the last two years ?
6. क्या आप अगस्त से हमारी प्रतीक्षा नहीं कर रहे हैं? – Have you not been waiting for us since August ?
7. पांच मिनट से दरवाज़ा कौन खटखटा रहा है? Who has been knocking at the door for five minutes ?
8. वह इतने दिनों से यहां क्यों ठहरा हुआ है ? – Why has he been staying here for so many days ?

Exercise

Translate into English:
1. दो घण्टे से छत का पंखा चल रहा है।
2. किसान प्रातः चार बजे से खेत में बीज बो रहा है।
3. शाम कई दिनों से बीमार है।
4. नल कल से चल रहा है।
5. प्रात: चार बजे से बूंदाबांदी हो रही है।
6. तेजिन्द्र दस बजे से हारमोनियम बजा रही है।
7. हम 1995 से होशियारपुर में रह रहे हैं।
8. तीन घण्टे से वर्षा हो रही है।
Hints:
1. ceiling fan;
2. sowing seeds;
4. running;
5. drizzling;
6. playing upon harmonium;
7. since 1995.

11. Past Perfect Continuous Tense (had + beon + V_1+ing)

1. वह एक घण्टे से फूल तोड़ रहा था। – He had been plucking flowers for an hour.
2. उसकी चाची बचपन से ही उसकी देखभाल कर रही थी। – Her aunt had been looking after her since childhood.
3. बच्चा तीन घण्टे से रो नहीं रहा था। – The child had not been crying since three o’clock.
4. रोगी पिछले सप्ताह से कुछ नहीं खा रहा था। – The patient had been taking nothing since last week.
5. क्या अध्यापक चार वर्षों से आपको पढ़ा रहे थे ? – Had the teachers been teaching you for four years?
6. क्या विद्यार्थी दो दिन से वृक्ष नहीं लगा रहे थे ? – Had the students not been planting trees for two days ?
7. क्या उसके पिता जी 1999 से शिमला में नहीं रह रहे थे? – Had his father not been living in Shimla since 1999 ?
8. वह सुबह से क्या पढ़ रहा था? – What had he been reading since morning ?

Exercise

Translate into English:
1. मैं प्रातः सात बजे से अपनी पुस्तक पढ़ रहा था।
2. मोहन दो वर्ष से नौकरी की खोज कर रहा था।
3. महात्मा गांधी जी दो साल से अन्याय के विरुद्ध लड़ रहे थे।
4. क्या हम एक घण्टे से कुएं से पानी खींच रहे थे ?
5. वह तीन घण्टे से प्रश्न हल कर रहा था।
6. धोबी प्रभात से कपड़े धो रहा था।
7. क्या वह काफ़ी देर से चिल्ला रहा था ?
8. कुत्ता आधी रात से भौंक रहा था।
Hints:
2. searching for a job;
3. fighting against injustice;
4. drawing; 8. barking.

12. Future Perfect Continuous Tense
(will / shall / have + been + V_1+ing)

1. शीला सुबह से गा रही होगी। – Sheela will have been singing since morning.
2. वह एक घण्टे से सितार बजा रही होगी। – She will have been playing on sitar for an hour.
3. हम सोमवार से परीक्षा नहीं दे रहे होंगे। – We shall not have been taking the examination since Monday.
4. सुन्दर सायं से अफसर की खुशामद नहीं कर रहा होगा। – Sunder will not have been flattering his officer since evening.
5. क्या वह प्रातः से गीता पढ़ रही होगी? – Will she have been reading the Gita since morning ?
6. क्या हम दो घण्टे से भजन नहीं गा रहे होंगे? – Shall we not have been chanting hymns for two hours ?
7. एक सप्ताह से वह कौन-सा पाठ दोहरा रही होगी? – Which lesson will she have been revising for a week ?
8. क्या डाकिया दो घण्टे से पत्र नहीं छांट रहा होगा? – Will the postman not have been sorting the letters for two hours ?

Exercise

Translate into English:
1. हम दो दिन से मैच खेल रहे होंगे।
2. वह चण्डीगढ़ में पन्द्रह दिन से ठहरा हुआ होगा।
3. पक्षी दो घण्टे से चहचहा रहे होंगे।
4. क्या रोगी चार दिन से बिस्तर पर नहीं पड़ा रहा होगा।
5. लोग प्रातः से मेले को जा रहे होंगे।
6. नल कब से बेकार चल रहा होगा?
7. बच्चे दो घण्टे से अपना पाठ याद कर रहे होंगे।
8. क्या वह पिछले सप्ताह से नौकरी की तलाश कर रहा होगा?
Hints:
2. staying;
3. chirping;
4. confined to bed;
6. running;
8. searching for.

13. Imperative Sentences
(वाक्य के आरम्भ में Bare Infinitive का प्रयोग)

1. बुरी संगत में मत बैठो। – Avoid bad company.
2. मुझ पर तरस खाओ। – Have pity on me.
3. फर्श पर मत थूको। – Do not spit on the floor.
4. किसी की चुगली मत करो। – Do not backbite anyone.
5. बकवास मत करो। – Do not talk nonsense.
6. निर्धनों की सहायता अवश्य कीजिए। – Do help the poor.
7. तुम्हें जो आदेश देता हूं, वह करो। – Do as I command you.
8. मोमबत्ती को फूंक मार कर बुझा दो। – Blow out the candle.
9. डॉक्टर को फोन करो। – Ring up the doctor.
10. नाखून काट लो। – Pare your nails.
11. कृप्या मुझे एक रुपए की रेज़गारी दो। – Please give me change for a rupee.
12. अतिथियों को अन्दर लाओ । – Show the guests in.
13. इधर-उधर की मत हांको, मतलब की बात करो। – Do not beat about the bush, come to the point.
14. मोटर गाड़ियां यहां मत खड़ी करो। – Do not park vehicles here.
15. तौलिया निचोड़ दो। – Wring out the towel.
16. संतरा छील लो। – Peel off the orange.
17. गड़े मुर्दे मत उखाड़ो। – Don’t rip up old sores.
18. परमात्मा पर विश्वास रखो और नेक काम करो। – Trust in God and do the right.
19. तकल्लुफ न करो। – Do not stand on ceremony.
20. अपनी आय से अधिक खर्च न करो। – Do not spend beyond your means.

Exercise

Translate into English:
1. काम से जी न चुराएं।
2. निर्धनों को घृणा की दृष्टि से न देखें।
3. ईमानदार बनो।
4. जेबकतरों से सावधान रहो।
5. अपने पर्चे नत्थी करो।
6. तुम्हें जो आदेश देता हूं, वह करो।
7. किसी की चुगली मत करो।
8. उसे परेशान मत करो।
9. आओ ताश खेलें।
10. चलो कुछ अच्छा करें।

14. Exclamatory And Optative Sentences

1. वे कितने बहादुर हैं ! – How brave they are !
2. कितना सुन्दर चित्र है ! – What a beautiful picture !
3. अहा ! हमने मैच जीत लिया है। – Hurrah ! We have won the match.
4. काश ! मै लखपति होता। – Would that I were a millionaire !
5. कितनी सुन्दर साड़ी है ! – What a beautiful sari !
6. कितना मूर्खतापूर्ण विचार है ! – What a foolish idea !
7. कितना प्यारा फूल है ! – What a lovely flower !
8. अफसोस ! वृद्धा स्त्री का पुत्र मर गया है। – Alas ! The old woman’s son is dead.

Exercise

Translate into English:
1. तुम कितने मूर्ख हो !
2. तुम कितने बुज़दिल हो !
3. परमात्मा करे कि आप परीक्षा में सफल हों !
4. बेटी, तुम्हारी आयु लम्बी हो !
5. ईश्वर आपको संतान दे !
6. अफसोस ! मैं लुट गया।
7. शाबाश, लड़कों ! तुमने कमाल कर दिया।
8. अहा ! मेरा भाई प्रथम आया है।
9. ईश्वर इस पापी को क्षमा करे !
10. काश ! मैं मंत्री होता।

15. Causative Sentences

1. उसने मुझे हंसाया। – He made me laugh.
2. गाय को पानी पिलाओ। – Water the cow.
3. मेरे जूते पालिश करवाओ। – Have my shoes polished.
4. उसने हमसे बहुत प्रतीक्षा करवाई। – He kept us waiting for a long time.
5. मैंने अपने मकान को सफ़ेदी करवाई। – I got my house whitewashed.
6. मेरे पिता जी ने मुझे बाहर नहीं जाने दिया। – My father did not let me go out.
7. मैंने सीटें आरक्षित करवा ली हैं। – I have got the seats reserved.
8. मेरे शत्रु ने मेरे भाई को मरवा दिया। – My enemy got my brother murdered.

Exercise

Translate into English:
1. रुपए से सब काम चलता है।
2. उसने मुझे रुलाया।
3. यह कोट धुलवा दो।
4. उसने अपना मकान खाली करवाया।
5. क्या तुमने टायफाइड का टीका लगवा लिया है ?
6. अपना जीवन बीमा करवा लो।
7. क्या आपने अपने मकान के दरवाजों को रंग करवा लिया है?
8. भिखारी ने सबको रुला दिया है।
9. मैंने बाल कटवाए।
10. चैक भुना लो।

16. Conditional Sentences

1. यदि आप अच्छा भोजन नही खाएंगे, तो आप बीमार हो जाएंगे। – If you do not eat good food, you will fall sick.
2. यदि अपने उसका अपमान किया तो आपने बहुत घटिया बात की। – If you insulted him, you did something very mean.
3. यदि मैंने आपका विरोध किया, तो मैंने अपना बदला लिया है। – If I have opposed you, I have taken my revenge.
4. यदि उसने परिश्रम किया होता, तो उसने छात्रवृति| प्राप्त कर ली होती। – If he had worked hard, he would have won a scholarship.
5. यदि आप दिल्ली गए, तो मेरे लिए कैमरा लाना। – If you go to Delhi, bring a camera for rne.
6. यदि पास होना है, तो परिश्रम करो। – If you want to pass, work hard.
7. यदि आप अमीर हैं, तो आपको ग़रीबों की सहायता| करनी चाहिए। – If you are rich, you should help the poor.
8. यदि मैं आपके स्थान पर होता, तो उसे क्षमा कर देता। – If I were you, I would forgive him.

Exercise

Translate into English:
1. यदि आप अमृतसर गए, तो मेरे भाई के पास ठहरना।
2. यदि मैं परिश्रम करूंगा, तो पास हो जाऊंगा।
3. यदि मैं आपकी जगह होता, तो उसे मज़ा चखा देता।
4. यदि आप धनी होते, तो ग़रीबों की सहायता अवश्य करते।
5. यदि आज वर्षा हुई, तो मैं स्कूल नहीं जाऊंगा।
6. यदि वह चाहता, तो तुम्हें नीचा दिखा सकता था।
7. यदि वह आए, तो उसे भगा देना।
8. यदि वह ग़रीब न होता, तो कोई उसका अपमान न करता।

17. Use Of Introductory ‘It’ AND ‘There’

1. आज आसमान साफ़ है। – It is clear today.
2. आपकी बड़ी कृपा है। – It is very kind of you.
3. कार से दिल्ली जाने में सात घण्टे लगेंगे। – It will take seven hours to reach Delhi by car.
4. इस डिब्बे में कोई खाली जगह नहीं। – There is no room in this compartment.
5. कल बैठक नहीं होगी। – There will be no meeting tomorrow.
6. युद्ध नहीं होगा। – There will be no war.
7. कल तूफ़ानी दिन होगा। – It will be a stormy day tomorrow.
8. अब चाय पीने का समय है। . – It is time to take tea now.

Exercise

Translate into English:
1. हमारे देश में बहुत से डॉक्टर नहीं हैं।
2. सड़क के दोनों ओर छायादार वृक्ष नहीं हैं।
3. हमारे देश में बहुतसी समस्याएं हैं।
4. स्कूल जाने का समय है।
5. आपकी ईमानदारी में कोई सन्देह नहीं है।
6. आज बहुत सुहावना मौसम है।
7. लो, छुट्टी की घंटी बज गई।
8. उसे उपदेश देने का कोई लाभ नहीं।
9. आज हवा बंद है।
10. सवा दस बजे हैं।

18. Use of : ‘Can’, ‘Could’, ‘Might’, ‘Should’,’Would’, ‘Must’ & ‘Ought’

1. क्या तुम इस खेत का क्षेत्रफल निकाल सकते हो? – Can you find out the area of this field ?
2. वह हंसे बिना न रह सका। – He could not help laughing.
3. शायद उसने आत्महत्या की हो। – He might have committed suicide.
4. आपको नए विचार अवश्य स्वीकार करने चाहिएं।| – You must accept the new ideas.
5. अमीरों को निर्धनों की सहायता करनी चाहिए। – The rich ought to help the poor.
6. मनुष्य नदियों के बहाव को भी रोक सकता है। – Man can change the course of rivers even.
7. वह मेरी सहायता कर सकता था। – He could have helped me.
8. हमें अपना वचन पूरा करना चहिए। – We should keep our word.

Exercise

Translate into English:
1. क्या वह आज समय पर स्कूल नहीं पहुंच सका?
2. शायद आज वर्षा हो।
3. क्या मैं अन्दर आ सकता हूं ?
4. मैं सितार बजा सकता हूं।
5. हमें अपने अध्यापकों का सम्मान करना चाहिए।
6. मैं यहां आया ताकि आपको मिल लूं।
7. हमें अपने देश से प्यार करना चाहिए।
8. वह हंसे बिना न रह सका।
9. शायद आज ओले पड़ें।
10. हमें ज़रूरतमंदों की सहायता करनी चाहिए।

19. Sequence of Tenses

1. वह कहता है कि इस वर्ष वह पहाड पर जाएगा।। – He says that he will go to hills this year.
2. मैं कहूंगा कि तुम बेईमान हो। – I shall say that you are dishonest.
3. उपदेशक ने कहा कि ईश्वर सर्व-व्यापक है। – The preacher said that God is omnipresent.
4. पिता जी ने कहा कि दो और दो चार होते हैं। – Father said that two and two make four.
5. हम नहीं जानते कि हमारी किस्मत में क्या लिखा है। – e do not know what is in store for us.
6. वह जानता है कि मैं उसका दोस्त हूं। – He knows that I am his friend.
7. मैं कहता हूं कि आप ग़लती पर हैं। – I say that you are in the wrong.
8. मैंने देखा कि मकान को आग लगी हुई है। – I saw that the house was on fire.

Exercise

Translate into English:
1. वह कहता है कि आज बारिश होगी।
2. मैं कहूँगा कि तुम बहुत चालाक हो।
3. उसने कहा कि ईश्वर बहुत दयालु है।
4. अध्यापक ने शिक्षा दी कि हमें परिश्रम करना चाहिए।
5. उसने कहा कि सत्य की हमेशा जीत होती है।
6. शाम परिश्रम करेगा ताकि पास हो जाए।
7. उसने कहा कि उसने एक पत्र लिखा है।
8. पिता जी ने मुझसे पूछा कि क्या मैं बाज़ार गया था।

20. Some Idiomatic Sentences

1. मुझे भूख नहीं है। – I have no appetite.
2. सच्चाई कड़वी होती है। – Truth tastes bitter.
3. झूठ के पांव नहीं होते हैं। – A lie has no legs to stand on.
4. मेरी बातों का बुरा न मानना। – Do not take offence at my words.
5. इस मामले को दबा दो। – Hush up this matter.
6. हमने उसे उल्लू बनाया। – We made a fool of him.
7. तुम मुंह क्यों चिढ़ाते हो? – Why do you make faces ?
8. तुम सारे काम अधूरे करते हो। – You do things by halves.
9. वह उसके झांसे में आ गया। – He fell into his trap.
10. यह सुनी सुनाई बात है। – It is a hearsay.
11. टोनी ने मेरे नाक में दम कर रखा है। – Tony has got on my nerves.
12. उसने मेरे ज़ख्मों पर नमक छिड़क दिया है। – He has added insult to my injury.
13. यह बात तुम्हारे और मेरे मध्य है। – It is between you and me.
14. उसके मस्तिष्क में गड़बड़ है। – He has a screw loose in his brain.
15. बहती गंगा में हाथ धो लो। – Make hay while the sun shines.
16. यह बूढ़ा कुछ दिन का मेहमान है। – This old man’s days are numbered.
17. उसकी आशाओं पर पानी फिर गया। – His hopes were dashed to the ground.
18. तुम मेरे पीछे क्यों पड़े हो? – Why are you after me ?
19. वह तुम्हारी मुट्ठी में है। – He is a puppet in your hands.
20. उन्होंने लज्जा बेच खाई है। – They have no sense of shame.
21. इधर-उधर की मत हांको। – Do not beat about the bush.
22. समय सारे घाव भर देता है। – Time is a great healer.
23. वह पूरा बदमाश है। – He is a rogue of the first water.
24. दूसरों के दोष मत निकालो। – Do not find fault with others.
25. बस खचाखच भरी हुई थी। – The bus was packed to capacity.

Exercise

Translate into English:
1. गले पड़ा ढोल बजाना ही पड़ता है।
2. फौज ने पूरे जोर से शत्रु का मुकाबला किया।
3. मेरे कामों में अपनी टांग मत अड़ाओ।
4. उसने मेरा सुख-दुःख में साथ दिया।
5. वह खुशी से फूला नहीं समाया।
6. मेरा समय काटे नहीं कटता।
7. आओ हम अपने मतभेद मिटा दें।
8. दाल में कुछ काला है।
9. बस खचाखच भरी हुई थी।
10. सच्चाई कड़वी होती है।

21. Some Proverbs

1. अन्त भले का भला। – All is well that ends well.
2. लोहे को लोहा काटता है। – Diamond cuts diamond.
3. अपना-अपना, पराया-पराया। – Blood is thicker than water.
4. ईंट का जवाब पत्थर। – Tit for tat.
5. आगा दौड़, पीछा चौड़। – Haste makes waste.
6. आज का काम कल पर मत छोड़ो। – Do not put off till tomorrow what you can do today.
7. तेल देखो तेल की धार देखो। – Let us see which way the wind blows.
8. वह हरफनमौला है। – He is a jack of all trades.
9. अपनी गली में कुत्ता भी शेर होता है। – Every cock fights best at his own dunghill.
10. आप मरे जग प्रलय।- Death’s day is doomsday.
11. उलटे बांस बरेली को। – To carry coals to Newcastle.
12. जैसा करोगे, वैसा भरोगे। – As you sow, so shall you reap.
13. होनहार बिरवान के होत चीकने पात। – Coming events cast their shadows before.
14. नीम-हकीम, खतरा-ए-जान। – A little knowledge is a dangerous thing.
15. घमंडी का सिर नीचा। – Pride hath a fall.
16. खाली दिमाग़ शैतान का घर है। – An idle brain is the devil’s workshop.
17. अपनी चादर देख कर पैर पसारो। – Cut your coat according to your cloth.
18. भागते चोर की लंगोटी ही सही। – Something is better than nothing.“
19. बात का बतंगड़ मत बनाओ। – Do not make a mountain of a mole hill.
20. कुत्ते का कुत्ता बैरी। – Two of a trade seldom agree.
21. एकता में बल है। – Union is strength.
22. सेवा बिन मेवा नहीं। – No pains, no gains.
23. जिसकी लाठी उसकी भैंस। – Might is right.
24. एक पंथ, दो काज। – To kill two birds with one stone.
25. जहां चाह, वहां राह। – Where there is a will, there is a way.

Exercise

Translate into English:

1. आंख से दूर, दिल से दूर।
2. खोदा पहाड़ निकला चूहा।
3. दिल को दिल से राह।
4. नीम हकीम खतराए-जान।
5. लोहे को लोहा काटता है।
6. अपनी चादर देख कर पैर पसारो।
7. बुरी संगत से आदमी अकेला भला।
8. भूख में चने बादाम।
9. बात का बतगंड़ मत बनाओ
10. दरिया में रह कर मगरमच्छ से बैर।

Sentences From Grammar Book

A. Affirmative Sentences
1. वह मेरा दोस्त है। – He is my friend.
2. मैं एक खिलाड़ी हूं। – I am a player.
3. आप ठीक हो। – You are right.
4. वे चालाक थे। – They were clever.
5. अमित मेरा विद्यार्थी था। – Amit was my student.

B. Negative Sentences

1. वह लेखक नहीं है। – He is not a writer.
2. हमें जल्दी नहीं हैं। – We are not in a hurry.
3. मैं नेता नहीं हूँ। – I am not a leader.
4. मैं बेईमान नहीं था। – I was not dishonest.
5. बच्चे दुःखी नहीं थे। – Children were not unhappy.

C. Interrogative Sentences

1. क्या वह आपका दोस्त है? – Is he your friend ?
2. क्या शमां कंजूस है? – Is Shama a miser ?
3. क्या मनजीत ईमानदार है? – Is Manjit honest?
4. क्या आप सही थे? – Were you right?
5. क्या लड़के खुश थे? – Were the boys happy ?

Exercise

1. वह भूखा है। – He is hungry.
2. ईमानदारी सबसे उत्तम नीति है। – Honesty is the best policy.
3. मैं कवि हूँ। – I am a poet.
4. हमें रोज़ सैर करनी चाहिए। – We should go for a walk daily.
5. वे भ्रष्ट नहीं थे। – They were not corrupt.
6. यह मकान बिकाऊ नहीं है। – This house is not for sale.
7. राज किसान नहीं था। – Raj was not a farmer.
8. क्या दस बजे हैं? – Is it ten o’clock ?
9. क्या आपके पिता जी बहुत अमीर थे? – Was your father very rich ?
10. क्या यह आम रास्ता है? – Is it a thoroughfare ?

I. Present Indefinite Tense

A. Affirmative Sentences

1. विद्यार्थी हर रोज़ मैदान में खेलते हैं। – Students play in the ground every day.
2. मैं अपने माता-पिता का दिल से सम्मान करता हूं। – I respect my parents from the core of my heart.
3. सतजोत डी० ए० वी० कॉलेज, जालन्धर में पढ़ता है। – Satjot studies in D.A.V. College, Jalandhar.
4. प्रिंसिपल आधी छुट्टी के समय स्कूल का चक्कर लगाता है। – The Principal takes a round of the school in the recess.
5. हम सड़क के नियमों का पालन करते हैं। – We obey the traffic rules.

B. Negative Sentences

1. सीता अपने भाई के साथ बाज़ार नहीं जाती है। – Sita does not go to the market with her brother.
2. तुम मुझे पसंद नहीं करते हो। – You don’t like me.
3. आजकल विद्यार्थी पढ़ाई से जी नहीं चुराते हैं। – The students do not shirk studies these days.
4. वह मेरी ओर बिल्कुल भी ध्यान नहीं देती है। – She does not pay any attention to me.
5. वह आटा गूंधना नहीं जानता। – He does not know how to knead flour.

C. Interrogative Sentonces

1. क्या आप किराए के मकान में रहते हैं? – Do you live in a rented house ?
2. क्या अध्यापक विद्यार्थी को सज़ा देता है? – Does the teacher punish the students ?
3. क्या वह स्वैटर नहीं बुनती है ? – Does she not knit sweater ?
4. क्या हम शाम को खेलने जाते हैं ? – Do we go to play in the evening ?
5. क्या दादा जी रोज़ मंदिर जाते हैं ? – Does grandfather go to temple everyday ?

II. Past Indefinite Tense

A. Affirmative Sentences

1. मैंने परसों नई कार खरीदी। – I bought a new car the day before yesterday.
2. वह अपना पर्स घर भूल गई। – She forgot her purse at home.
3. तुमने मेरे दोस्तों के सामने मुझे अपमानित किया। – You insulted me in the presence of my friends.
4. राजीव ने बहुत सुंदर तसवीर खींची। – Rajiv clicked a beautiful photograph.
5. जासूस ने अपने देश के साथ गद्दारी की। – The spy betrayed his country.
6. उसने मुझे रसीद दे दी। – She/he handed over the receipt to me.

B. Negative Sentences

1. चौकीदार ने दरवाज़े की कुंडी नहीं लगाई। – The watchman did not bolt the door.
2. अध्यापक ने टैस्ट नहीं लिया। – The teacher did not give the test.
3. हमें गर्मजोशी से स्वागत नही मिला। – We did not receive a warm welcome.
4. वह अदरक छीलना नहीं जानती थी। – She did not know how to peel ginger.
5. माता जी ने चादर तह नहीं की। – The mother did not fold the bed sheet.

C. Interrogative Sontences

1. क्या उसने मेरा जिक्र किया ? – Did he talk about me ?
2. क्या आप कल वहाँ गए ? – Did you go there yesterday?
3. क्या मुख्यमंत्री ने झंडा लहराया ? – Did the Chief Minister hoist the flag ?
4. क्या आपने उससे जुर्माना नहीं लिया ? – Did you not collect fine from him ?
5. क्या बावर्ची ने खाना परोसा ? – Did the chef serve the food ?

III. Future Indefinite Tense

A. Affirmative Sentences

1. अतिथि रोटी घर ही खायेंगे। – The guests will take food at home only.
2. वह मंगलवार तक कार्य समाप्त कर लेगा। – He will complete the work by Tuesday.
3. मेरे पिता जी मेरा कर्ज चुकाएंगे। – My father will pay my debt.
4. वह अवश्य तुम्हें धोखा देगी। – She will definitely deceive you.
5. पहलवान कुश्ती जीत लेगा। – The wrestler will win the fight/bout.

B. Negative Sentences

1. मैं आपको निराश नहीं करूंगा। – I will not disappoint you.
2. हम आपकी शिकायत नहीं करेंगे। – We won’t complain against you.
3. नौकरानी बर्तन साफ़ नहीं करेगी। – The maid will not wash the utensils.
4. हम अपना आस-पास साफ़ रखेंगे। – We shall keep our surroundings clean.
5. विद्यार्थी अपनी कक्षा में कूड़ा-कर्कट नहीं डालेंगे। – The students will not litter the class.

C. Interrogative Sentences

1. क्या आप कल साक्षात्कार में शामिल होंगे? – Will you appear in the interview tomorrow?
2. क्या हम इस साल पहाड़ों पर जाएंगे? – Shall we go to mountains this year?
3. क्या आप आज बिल बना लोगे? – Will you prepare the bill today?
4. क्या उसे कभी बोलने का तरीका आएगा? – Will he ever learn how to speak ?
5. क्या वह हमारे लिए चाय बनाएगी? – Will she prepare tea for us?

IV. Present Continuous Tense

A. Affirmative Sentences

1. नेता जी इधर-उधर की बातें कर रहे हैं। – The leader is beating about the bush.
2. वह तौलिया निचोड़ रही है। – She is wringing out the towel.
3. रमेश अपनी कल होने वाली परीक्षा की तैयारी कर रहा है। – Ramesh is preparing for his next day’s exam.
4. अध्यापक हमें अच्छे कार्य करने हेतु प्रेरित कर रहा है। – The teacher is motivating us to do good deeds.
5. पिता जी कपड़ों को इस्तरी कर रहे हैं। – The father is ironing the clothes.

B. Negative Sentences

1. वह मेरी बात नहीं सुन रही है। – She is not listening to me.
2. बच्चे पंक्ति में नहीं चल रहे हैं। – Children are not walking in a queue.
3. मै आपकी योग्यता पर शक नहीं कर रहा हूँ। – I am not doubting your competence.
4. न्यायाधीश सही निर्णय नहीं कर रहा है। – The judge is not delivering the right verdict.
5. राजनेता हमें मूर्ख नहीं बना रहे हैं। – The politicians are not befooling us.

C. Interrogative Sentences

1. क्या तुम मेरी उपेक्षा कर रहे हो? – Are you avoiding me?
2. क्या अधिकारी कर्मचारियों का शोषण कर रहा है? – Is the officer exploiting the employees?
3. क्या वह मेरा फ़ोन नहीं उठा रहा है? – Is he not receiving my call ?
4. क्या तुम धूप में बैठे हो? – Are you sitting in the sun ?
5. क्या वह अपना सामान अपने साथ ला रहा है? – Is he bringing his luggage along with him ?

V. Past Continuous Tense

A. Affirmative Sentences

1. वह मेरा मज़ाक उड़ा रहा था। – He was making fun of me.
2. वह शीशे के सामने अपने बालों को कंघी कर रही थी। – She was combing her hair in front of the mirror.
3. जब आपने फ़ोन किया था, तब मेरी माता जी मुझे सलाह दे रही थीं। – My mother was giving me a piece of advice when you called me up.
4. बच्चा पिता जी को मूर्ख बनाने की कोशिश कर रहा था। – The child was trying to befool his father.

B. Negative Sentences

1. भोली अपने पिता जी के साथ बहस नहीं कर रही थी।। – Bholi was not arguing with her father.
2. संगीतकार एक नई धुन की रचना नहीं कर रहा था। – The musician was not composing a new tune.
3. राम लाल अपनी बेटी की चिंता नहीं कर रहा था।| – Ram Lal was not worrying about his daughter.
4. बच्चा रो नहीं रहा था। – The child was not crying.
5. मेज़बान मेहमान को खाना नहीं परोस रहा था।| – The host was not serving food to the guest.

C. Interrogative Sentences

1. क्या आप उसके बचाव की कोशिश कर रहे थे? – Were you trying to defend him ?
2. क्या प्रिंसिपल उस समय कागज़ तसदीक कर रहा था? – Was the principal attesting the papers at that time ?
3. क्या रमेश उस समय अपनी गलती के लिए माफी मांग रहा था? – Was Ramesh apologizing for his mistake at that time ?
4. जब मैंने उसको बुलाया था, क्या तब बच्चा भोजन का आनन्द ले रहा था? – Was the child relishing the food when I called him ?

VI. Future Continuous Tense

A. Affirmative Sentences

1. वह अगले साल से बैंक में नौकरी कर रहा होगा। – He will be working in a bank next year.
2. हम अपनी परीक्षा की तैयारी कर रहे होंगे। – We shall be preparing for our examination.
3. उस समय माली पौधों को पानी दे रहा होगा। – The gardener will be watering the plants at that time.
4. जब मैं घर पहुंचूंगा, सूर्य अस्त हो रहा होगा। – The sun will be setting when I reach home.

B. Negative Sentences

1. जब आप उसके घर जाओगे, वह रात का खाना नहीं खा रहा होगा। – He will not be taking dinner when you go to his house.
2. जब आप हमें मिलने आओगे, हम आराम नहीं कर रहे होंगे। – We shall not be resting when you visit us.
3. हम एक सप्ताह तक वहां नहीं रहेंगे। – We shall not be staying there for a week.
4. तब वर्षा नहीं हो रही होगी। – It will not be raining then.

C. Interrogative Sentencos

1. क्या तब वहां वर्षा नहीं हो रही होगी? – Will it not be raining there then ?
2. जब आप खेल के मैदान में पहुँचोगे, क्या विद्यार्थी पंक्तियों में खड़े होंगे? – Will the students be standing in queues when you reach the playground ?
3. क्या सितम्बर में वृक्ष के पत्ते झड़ रहे होंगे? – Will the tree be shedding off its leaves in September ?
4. क्या कल सुबह सात बजे पुलिस चोर का पीछा कर रही होगी? – Will the police be chasing the thief at 7 a.m. tomorrow ?

VII. Present Perfect Tense

A. Affirmative Sentences

1. उसने चाय पी ली है। – He has taken tea.
2. तकनीक ने बहुत उन्नति कर ली है। – Technology has developed a lot.
3. हमने अपने स्कूल में विज्ञान मेले का आयोजन किया है। – We have organized a science fair in our school.
4. महंगाई ने हमारे जीवन को बहुत प्रभावित किया है। – Inflation has affected our lives a lot.
5. वे काफी समय पहले इस स्थान को छोड़ चुके हैं। – They have left this place long ago.

B. Negative Sentences

1. उसने रिश्ते से मना नहीं किया है। – She has not refused the marriage proposal.
2. मैंने उसे मनाने की कोशिश नहीं की है। – I have not tried to convince her.
3. उसने नया संगीत नहीं सुना है। – He has not listened to the latest music.
4. आपके लड़के ने मेरे साथ अच्छा व्यवहार नहीं किया है। – Your son has not behaved well with me.
5. हमने अपनी गलती का अहसास नहीं किया है।| – We have not realized our mistake.

C. Interrogative Sentences

1. क्या उसने स्वयं को मुसीबत में डाल लिया है? – Has he got himself into trouble ?
2. क्या उसने अपनी स्कूल की शिक्षा पूरी कर ली है? – Has he completed his schooling ?
3. क्या आपने बारहवीं में विज्ञान का चयन नहीं – Haven’t you opted for Science stream in grade 12 ?
4. क्या मुख्य अतिथि ने विद्यार्थियों के प्रदर्शन किया है? – Has the Chief Guest applauded the performance of the students ?
5. क्या उसने वोट डाल दी है? – Has he cast his vote?

VIII. Past Perfect Tense

A. Affirmative Sentences

1. वह नई कार 2011 तक खरीद चुका था। – He had bought a new car by 2011.
2. अपने पिता जी की मौत के बाद उसने अपना पारिवारिक व्यवसाय ही चाल रखा था। – He had continued his family business after his father’s death.
3. मैं पहले ही इस कारखाने में दो साल नौकरी कर चुका था। – I had already worked for two years in this factory.
4. हड़ताल के बाद कंपनी ने पिछले साल कई कर्मचारी नौकरी से निकाल दिये थे। – The company had fired many employees after the strike last year.

B. Negative Sentences

1. दो बजे तक अध्यापक ने सारे उत्तर चैक नहीं किये थे। – The teacher had not checked all the answers by 2 p.m.
2. मई 2016 तक सरकार ने कोई नोटिस जारी नहीं किया था। – The government had not issued any notice till May 2016.
3. जब वह आया, तब तक मैं अपना कार्य समाप्त नहीं कर चुका था। – I had not finished my work when he came.
4. वे वर्षा रुकने के बाद ही गए। – They left after the rain had stopped.

C. Interrogative Sentences

1. क्या उपभोक्ता ने धोखा खाने के बाद कम्पनी के खिलाफ केस दायर कर दिया था? – Had the consumer filed a case against the company after he was cheated ?
2. जब आप हवाई अड्डा पहुंचे, क्या हवाई जहाज़ उड़ान भर चुका था? – Had the flight taken off when you reached the airport?
3. जब आप स्टेशन पहुंचे, क्या रेलगाड़ी आ गयी थी? – Had the train arrived when you reached the station ?
4. क्या उसने 2017 तक काफी धन इकट्ठा कर लिया था? – Had he accumulated a lot of wealth by 2017 ?

IX. Future Perfect Tense

A. Affirmative Sentences

1. उसने अपना गृहकार्य शाम छ: बजे तक समाप्त कर लिया होगा। – She will have finished her homework by 6 o’clock.
2. मैं जब विद्यालय पहुंचूंगा, विद्यार्थी मैदान में एकत्रित हो चुके होंगे। – The students will have gathered in the ground when I reach school.
3. आपके तैयार होने तक मैं पगड़ी बांध चुका हूँगा। – I shall have tied the turban by the time you get ready.
4. हम सूरज छिपने तक विरोधी पक्ष को हरा चके होंगे। – We will have defeated the opponents by sunset.
5. हम सभी मिलकर नौ बजे तक जन्मदिन मना चुके होंगे। – We all shall have celebrated the birthday by 9 p.m.

B. Negative Sentences

1. पिता जी पाँच बजे तक घर नहीं पहुंच चुके होंगे। – Father will not have reached home by 5 p.m.
2. गाड़ी के आने तक कुली सारा सामान नहीं ले गया होगा। – The porter will not have carried the entire luggage by the time the train arrives.
3. अगले पांच साल तक हम नई खोज नहीं कर चुके होंगे। – We shall not have made a new discovery in the next five years.
4. अगले सप्ताह तक आप सारे तरीके नहीं आजमा चुके होंगे। – You will not have tried all the ways by I the next week.

C. Interrogative Sentences

1. क्या आप सोमवार तक हवाई यात्रा कर चुके होंगे? – Will you have travelled by air by Monday ?
2. क्या मंत्री जी ने अन्त में चुनाव भारी अंतर से जीत लिये होंगे? – Will the minister have won the election by a big margin in the end ?
3. क्या हवाई जहाज़ दोपहर दो बजे तक उतर चुका होगा? – Will the plane have landed by 2 p.m. ?
4. क्या मज़दूर संघ ने अगले महीने हड़ताल खत्म कर दी होगी? – Shall the workers’ union have called off the strike by the next month ?
5. क्या पीड़ित ने दिसम्बर तक अदालत में अपील लगाई होगी? – Will the victim have appealed in the court by December ?

X. Present Perfect Continuous Tense

A. Affirmative Sentences

1. मैं पिछले 20 सालों से इस मकान में रह रहा हूँ। – I have been living in this house for last twenty years.
2. दोनों सहेलियाँ सुबह से ही गप्पें मार रही हैं। – Both the friends have been gossiping since morning.
3. दोनों परिवार काफी समय से एक-दूसरे से लड़ रहे हैं। – Both the families have been quarrelling with each other since long.
4. वह काफी समय से इस दिन की तैयारी (प्रतीक्षा)कर रहा है। – He has been waiting (preparing) for this day since ages (long).
5. मैं 2:00 बजे से फिल्म देख रहा हूँ। – I have been watching the movie since 2 o’clock.

B. Negative Sentences

1. रमेश कई दिन से स्कूल नहीं आ रहा है। – Ramesh has not been coming to school for many days.
2. उम्मीदवार पिछले सप्ताह से चुनाव प्रचार नहीं कर रहा है। – The candidate has not been canvassing since last week.
3. आप कई दिनों से मुझे मिलने नहीं आए हो। You have not come to meet me for many days.
4. उसकी दादी जी सुबह से खाना नहीं खा रही हैं। – Her grandmother has not been eating since morning.
5. बच्चा शाम से अपने माता-पिता की बात नहीं सुन रहा है। – The child has not been listening to his parents since evening.

C. Interrogative Sentences

1. क्या आप 1990 से यहां काम कर रहे हो? – Have you been working here since 1990?
2. क्या मैकेनिक सुबह से मोटर ठीक कर रहा है? – Has the mechanic been repairing the motor since morning ?
3. क्या आप कई महीनों से परीक्षा की तैयारी कर रहे हो? – Have you been preparing for exam for many months ?
4. क्या किराएदार पिछले छः महीनों से किराया नहीं दे रहा है? – Has the tenant not been paying the rent for the last six months ?
5. क्या अध्यापक सुबह से पढ़ा रहा है? – Has the teacher been teaching since morning ?

XI. Past Perfect Continuous Tense

A. Affirmative Sentonces

1. जब उसके पिता जी की मृत्यु हो गयी, वह पिछले दस सालों से मेहनत कर रहा था। – He had been working hard for the last ten years when his father died.
2. जब मैं उससे मिलने गई, बच्चा सुबह से ही रो रहा था। – The child had been crying since morning when I visited her.
3. जब मैं घर पहुंचा तो पिछले दो घण्टे से वह मेरी प्रतीक्षा कर रहे थे। – They had been waiting for me for the last two hours when I reached home.
4. मैं पिछले कई दिनों से इस पुस्तक को पढ़ रहा था। – I had been reading this book for läst many days.
5. प्रकाश पिछले कई सालों से टैनिस खेल रहा था। – Prakash had been playing tennis for last many years.

B. Negative Sentences

1. माली सोमवार से पौधों को पानी नहीं दे रहा था। – The gardener had not been watering the plants since Monday.
2. सचिन दो सालों से क्रिकेट नहीं खेल रहा था। – Sachin had not been playing cricket for two years.
3. रवि पिछले दस दिनों से स्कूल नहीं आ रहा था। – Ravi had not been coming to school for the last ten days.

C. Interogative Sentences

1. क्या आप यहां पिछले पांच सालों से काम। कर रहे थे? – Had you been working here for the last five years ?
2. क्या बच्चा पिछले पन्द्रह दिनों से बीमार था? – Had the child been sick for the last fifteen days ?
3. जब वह घर आए तो क्या उनकी बेटी दो घंटे से काम कर रही थी? – Had his daughter been working for two hours when he came home ?

XII. Future Perfect Continuous Tense

A. Affirmative Sentences

1. उसके पिता जी सुबह दो घण्टे से बगीचे में वृक्ष लगा रहे होंगे। – His father will. have been planting trees in the garden for two hours in the morning.
2. वह चार घंटों से दो बजे तक मेरी प्रतीक्षा कर रहा होगा। – He will have been waiting for me for four hours by 2 o’clock
3. वह अगले महीने कार चलाना सीख रहा होगा। – He will have been learning how to drive a car next month.

B. Negative Sentences

1. कल इस समय नेता जी लम्बे-लम्बे भाषण नहीं दे रहे होंगे। – The leader will not have been delivering long speeches by this time tomorrow.
2. जब मैं स्कूल जाऊंगा, लड़के फुटबाल नहीं खेल रहे होंगे। – When I go to school, the boys will not have been playing football.
3. मैं जब घर पहुंचूंगा, माता जी खाना नहीं बना रही होंगी। – The mother will not have been cooking when I reach home.

C. Interrogative Sentences

1. क्या ड्राइवर लगातार 15 दिन से लगातार कार चला रहा होगा? – Will the driver have been continuously driving the car for fifteen days?
2. क्या हम मई महीने में हर रोज़ दो घंटे पैसे इकट्ठे कर रहे होंगे? – Shall we have been collecting the money for two hours every day in May ?
3. क्या जब मैं घर वापिस आऊँगा, बच्चे सुबह से पतंग उड़ा रहे होंगे? – Will the children have been flying the kites since morning when I return home?

Imperative Sentences

1. अपने अध्यापकों की आज्ञा का पालन करो। – Obey your teachers.
2. गरीबों की सहायता करो। – Help the poor.
3. इस पत्र को डाक द्वारा भेजो। – Post this letter.
4. पशुओं की हत्या न करो। – Don’t kill animals.
5. आग से मत खेलो। – Never play with fire.

Affirmative Sentences

1. परमात्मा पर विश्वास रखो। – Trust in God.
2. बड़ों का सत्कार करें। – Obey your elders.
3. जेब कतरों से बचें। – Beware of the pickpockets.
4. उसे जाने दो। – Let him go.
5. जल्दी करो। – Hurry up.

Negative Sentences

1. मुझे तंग न करो। – Don’t disturb me.
2. गरीब का मज़ाक न उड़ाओ। – Don’t make fun of the poor.
3. अफवाह न फैलाओ। – Don’t spread rumours.
4. किसी की चुगली न करो। – Don’t backbite anyone.
5. बकवास न करो। – Don’t talk nonsense.

Miscellaneous Exercises from Grammar Book (Fully Solved)

Exercise 1

1. नंगे पांव न चलो। – Don’t walk barefoot.
2. अपने स्वास्थ्य का ध्यान रखो। – Take care of your health.
3. समय बर्बाद न करें। – Don’t waste time.
4. समय का सदुपयोग करें। – Make a proper use of time.
5. बाएं हाथ चलें। – Keep to the left.
6. पुलिस को बुलाओ। – Call the police.
7. अभ्यास करो। – Do the practice.
8. अपना कार्य स्वयं करें। – Do your work yourself.
9. अपना ध्यान रखें। – Take care of yourself.
10. परिश्रम करें। – Work hard.

Exercise 2

1. सूरज अस्त हो गया है। – The sun has set.
2. मन्द मन्द हवा चलनी शुरू हो गई है। – Light breeze has started blowing.
3. बहुत सारे लोग स्टेशन के बाहर एकत्रित हो गए थे। – Many people had gathered outside the station.
4. मुझे यह पुस्तक चाहिए। – I need this book.
5. भूगोल मेरा मनपसंद विषय है। – Geography is my favourite subject.
6. मुझे स्कूल पहुंचने में देर हो गई है। – I am late for school.
7. जब तक आप कहोगे, वह जागती रहेगी। – She will keep awake till you want
8. माली पौधों को पानी दे रहा था। – The gardener was watering the plants.
9. यह बहुत ही मनोरंजक कहानी है। – This is a very interesting story.
10. वह शायद आज आ जाए। – He/She might come today.

Exercise 3

1. वह कई घंटे लगातार कार्य कर सकता है। – He can work for many hours at a stretch.
2. रेखा मेरी मनपसंद अभिनेत्री है। – Rekha is my favourite actress.
3. सिगरेट पीना स्वास्थ्य के लिए हानिकारक है। – Smoking is injurious to health.
4. वह अनाथ था। – He was an orphan.
5. दहेज प्रथा समाज पर एक कलंक है। – Dowry system is a blot on the society.
6. वह उम्र में मुझसे बड़ी है। – She is older than I.
7. दोनों भाइयों की आपस में नहीं बनती। – Both the brothers dont see each other eye to eye.
8. हरिद्वार भारत का बहुत प्रसिद्ध तीर्थस्थान है। – Haridwar is the most famous pilgrimage of India.
9. डॉ० ए० पी० जे० अब्दुल कलाम एक विश्व प्रसिद्ध वक्ता थे। – Dr. A.P.J. Abdul Kalam was a world famous speaker.
10. जनसंख्या में भारत विश्व में दूसरे स्थान पर है। – As regards population, India occupies second place in the world.

Exercise 4

1. वह एक अच्छा कलाकार है। – He is a very good artist.
2. आज धूप बहुत तेज़ है। – The sun is very sharp today.
3. क्या आपको मुझ पर भरोसा नहीं? – Don’t you trust me ?
4. जज ने उसको जमानत दे दी। – The judge has granted him bail.
5. जी गरजते हैं; वे बरसते नहीं। – Barking dogs seldom bite.
6. वह आपंको उल्लू बना रहा है। – He is befooling you.
7. गरीब का शाप कभी न लो।। – Never have curses of the poor.
8. सड़क की मरम्मत नहीं की गई। – The road was not repaired.
9. जैसा करोगे, वैसा भरोगे। – As you sow, so shall you reap.
10. क्या मैं आपकी प्रतीक्षा करूं? – Shall I wait for you?

Exercise 5

1. शहर में डेंग फैल रहा है। – Dengue is spreading in the city.
2. वहां क्या हो रहा है? – What is happening there ?
3. एकता में बल है। – Unity is strength.
4. जीवन में संतुष्टि भी एक वरदान है। – Contentment in life is also a bliss.
5. उसे सारा निबंध मौखिक याद था। – He had learnt the whole essay by heart.
6. साफ़-सफ़ाई करना अच्छी आदत है। – Cleanliness is a good habit.
7. बच्चा क्यों रो रहा है? – Why is the child crying ?
8. वातावरण साफ रखना हर नागरिक का कर्तव्य है। – It is every citizen’s duty to keep the environment clean.
9. रोगी की हालत ठीक हो रही है। – The patient’s condition is improving.
10. विद्या व्यक्ति को सूझवान बनाती है। – Education makes man wise.

Exercise 6

1. वह टेढ़ी खीर है। – He is a hard nut to crack.
2. पढ़ाई में अपना मन लगाओ। – Concentrate on your studies.
3. क्या आप गरीबों की सहायता करते हो? – Do you help the poor?
4. मैं यह अपमान सहन नहीं कर सकूँगा। – I will not be able to bear this insult.
5. क्या हवाई यात्रा सुरक्षित है? – Is the air travel safe ?
6. मैंने उसे गले लगा लिया। – I embraced him.
7. उसकी जुबान इतनी क्यों चलती है? – Why does he talk so much ?
8. वह रात बहुत ठंडी थी।। – That night was very cold.
9. आप अपना भविष्य खतरे में क्यों डाल रहे हो? – Why are you endangering your future ?
10. बाहर घनघोर अंधेरा था। – It was pitch dark outside.

Punjab State Board PSEB 11th Class English Book Solutions English Composition Notice Writing Exercise Questions and Answers, Notes.

PSEB 11th Class English Composition Note-Making

Note-making means presenting big chunks of knowledge in a neat and brief form. The characteristics of good notes are:

1. They are brief.
2. Only relevant facts are given.
3. Only words or phrases are used. Full sentences are often not necessary. In other words, the language used in making or taking notes may not be grammatically very correct.
4. Information is presented in a tabular form. It is divided and sub-divided. The division may be : main sections : 1, 2, 3, etc; sub-sections : a, b, c, etc.
5. Abbreviations and symbols are used instead of complete words.

Common abbreviations are given in dictionaries. In addition, every individual can use his or her own set of abbreviations and symbols. For example, ‘Parliament may be reduced to ‘parlť or ‘par’ or ‘Po. But care should be taken that one abbreviation does not stand for more than one word.

A Solved Example
Passage

About 2,500 million years ago (according to present theories), the earth on which we live was a ball of gas which, on cooling, grew smaller and became liquid. The liquid continued to cool, giving off some of its heat by radiation and when liquids grew colder they solidified. The crust of the earth is the solid matter that resulted from this cooling, but probably inside the earth there is still a mass of molten material which has not cooled as much as the outer crust.

The crust on which we live and from which we obtain almost everything that we need is not quite rigid. The volcanoes of Etna and Stromboli in the Italian islands remind us from time to time of the force and heat below the ground. Various places on the earth are subject to earthquakes, the country that has suffered most from these being Japan. The study of earthquakes, the science of seismology, has shown that the origin of many of them is under the bottom of the sea and near the coast of a continent or large island. In an earthquake, the surface of the land may move suddenly in any direction, bringing houses down, changing a straight railway-track into a useless zigzag, altering the course of rivers, changing the shape of hills, and sometimes causing an immense sea wave to rush on to the nearest shore and leading to immense destruction. This tidal wave, as it is called, is wrongly named because it has nothing to do with the tide.

Title : THE EARTH’S CRUST
Notes : 1. Formation of the earth
(a) 2,500 million yrs. ago – earth – a ball of gas;
(b) gradually gas grew cooler – became liquid;
(c) liquid cooled and solidified – this became earth’s crust.

2. Volcanoes and earthquakes
(a) Vol. indicate force and heat below earth’s crust.
(b) Ea. qs. – origin and effects :
(i) origin of many
1. under bottom of the sea
2. near the coast of continents.

(ii) effects-
1. bring widespread destruction, e.g., of houses, railways
2. alter-courses of rivers-shape of hills
3. cause tidal waves.

Examination-Style Fully Solved Passages

Passage 1

1 Men who have studied the ways of ants tell strange things about their social life. Just as in ancient days, men kept slaves to work for them, so also some ants raid the homes of other ants, and making captives, bring them back to their own nests and compel them to work for their captors’. Ants, indeed, are by no means the only inhabitants? of ants’ nests. We are told that several hundreds of other small creatures such as beetles and crickets make their home with ants. Some of these creatures are useful to the ants and clean up the nest and do other duties, while others seem to be mere boarders”, and do nothing for their keep“. Why the ants allow them to stay no one knows. Is it sheer good nature ?

2 Ants, we are told, play games. They nurse their sick, bury their dead and see that everyone does its share of the work, and that no one is allowed to be lazy. All this seems to show that ants have some form of reasonable government. A celebrated naturalist? tells how he captured an ant and covered it with a piece of clay so that only its head showed. For some time, the little captive was not discovered, then one of its fellow ants found out what had happened and tried to set it free. But this task proved too much for one ant, and very soon it hurried away, to return in a few minutes with a dozen companions who at once set to work and in a very short time set the captive free.

Word-meanings :

Questions
(a) On the basis of your reading of the passage, make notes on it. Use recognizable abbreviations wherever necessary.
(b) Give a suitable title to the passage.
Model Answers
Abbreviations used:
1. str. – strange
2. soc. – social
3. sl. – slaves
4. act. – activities

(A) Notes on the Passage:
1. Ants:
(a) str., soc. insects
(b) keep ant sl. to work for them
(c) have many others in their nests.
→ clean the nests
→ do other duties

2. Ant act-
(a) play games
(b) nurse their sick
(c) bury their dead
(d) keep everyone busy
(e) help those in need.

(B) Title : The Social Life of Ants

Passage 2

1 Why don’t I have a telephone ? Not because I pretend to be wise or pose as unusual. There are two chief reasons : because I don’t really like the telephone, and because I find I can still work and play, eat, breathe, and sleep without it. Why don’t I like the telephone ? Because I think it is a pest’ and a time-waster. It may create unnecessary suspense and anxiety, as when you wait for an expected call that doesn’t come; or irritating delay, as when you keep ringing a number that is always engaged. As for speaking in a public telephone box, that seems to me really horrible. You would not use it unless you were in a hurry, and because you are in a hurry, you will find other people waiting before you. When you do get into the box, you are half asphyxiated? by stale, unventilated air, flavoured with cheap face powder and chain-smoking; and by the time you have begun your conversation, your back is chilled by the cold looks of somebody who is fidgeting to take your place.

2 If you have a telephone in your house, you will admit that it tends to ring when you least want it – when you are asleep, or in the middle of a meal or a conversation, or when you are just going out, or when you are in your bath. Are you strong-minded enough to ignore it, to say to yourself, ‘Ah well, it will be the same in a hundred years time.’ ? You are not. You think there may be some important news or message for you. Have you never rushed dripping from the bath, or chewing from the table, or dazed4 from bed, only to be told that you are wrong number ? You were told the truth. In my opinion, all telephone numbers are wrong numbers. If, of course, your telephone rings and you decide not to answer it, then you will have to listen to an idiotic bell ringing and ringing in what is supposed to be the privacy of your own home. You might as well buy a bicycle bell and ring it yourself.

Word-meanings:

Questions
(a) Make notes on the basis of your reading of the above passage, using headings and sub headings. Use recognizable abbreviations wherever necessary.
(b) Give a suitable title to the passage.
Model Answers
Abbreviations used:
1. wr. – writer
2. tlph. – telephone
3. ppl. – people anxiety.
4. unwtd. – unwanted

(A) Notes on the Passage:
1. Why the wr. dislikes having a tlph.
(a) can do without it in all ways
(b) thinks it a pest and a time-waster
(c) creates unnecessary suspense and anxiety

2. What he thinks of using a tlph. box
(a) ppl. waiting before you
(b) can’t use when in hurry
(c) smelly, stinking boxes
(d) cold looks of ppl. waiting behind you.

3. The nuisance of having a tlph.
(a) unwtd. disturbance during sleep, meals, conversation, etc.
(b) can’t be ignored in any case
(c) even if ignored, the idiotic bell is most jarring.

(B) Title : Telephone — An Avoidable Nuisance

Passage 3

1. Gandhiji, the greatest political genius’ of our time, indicated the path to be taken. He gave living testimony to the fact that man’s will, sustained by an indomitable* conviction”, is more powerful than material forces that seem insurmountable’. On the whole, I believe that Gandhiji held the most enlightened? views of all the political men in our times. We should strives to do things in his spirit : not to use violence in fighting for our cause and to refrain’ from taking part in anything we believe as evil. Revolution without the use of violence was the method by which Gandhiji brought about the liberation of India. It is my belief that the problem of bringing peace to the world on a supranational basis will be solved only by employing Gandhiji’s method on a large scale.

2 The veneration1l in which Gandhiji has been held throughout the world rests on the recognition2, for the most part unconscious, that in our age of moral decay, he was the only statesman who represented that higher conception of human relation in the political sphere to which we must aspire 3 with all our powers. We must learn the difficult lesson that the future of mankind will only be tolerable when our course in world affairs as in all other matters, is based upon justice and law rather than the threat of naked” power, as has been true so far.

3 Gandhiji was a victim of his own principle, the principle of non-violence. He died because he did not take any personal armed protection in the times of disorder and general unrest in the country. He fully believed that the use of force is an evil in itself to be shunned?s by those who strive for absolute justice. He adopted this faith throughout his life and led the nation to the path of liberation.

Word-meanings:

Questions

(a) On the basis of your reading of the above passage, make notes on it. Use recognizable abbreviations wherever necessary.
(b) Give a suitable title to the passage.
Model Answers
Abbreviations used:
1. pol. = political
2. rev. – revolution
3. conc. – conception
4. pers. – personal
5. prot. – protection

(A) Notes on the Passage:
1. Gandhiji
(a) the greatest pol. genius of our time
(b) followed the path of non-violence
(c) believed in the power of the will
(d) believed in the rev. without the use of violence.

2. For tolerable future of mankind
(a) Gandhiji’s higher conc. of human relations
(b) application of justice and law instead of naked power.

3. Gandhiji, victim of his own principle of non-violence
(a) neglected pers. prot.
(b) called the use of force an evil

(B) Title : Gandhiji and Non-violence

Passage 4

1 Two determining factors pertaining to the nation’s health and well-being are family planning and nutrition. While talking about our country and its problems, let us remember that many of these problems are the result of our success and development. Our population problem is an example. As an eminent doctor has rightly remarked, doctors themselves are the creators of the population explosion. They must also find a way out. A child brings happiness. As Kabir, the saint-poet, has said, “A child comes crying into the world but the people around are swathed insmiles.” But such is the economic lot of the bulk of our people that even the birth of a child adds to their anxiety.

2 The aim of family planning is to restore4 joy to motherhood and to make every child a wanted child, with a fair chance of health, education and employment. Until now, our major difficulty was to create motivation and psychological climate. Our campaign has been successful in certain pockets. It is not unlikely that soon a nationwide clamour? for doctors and appliances for family planning will build up. And delay or failure in the family planning campaign will cause a setback to all our programmes. The other important health problem is that of nutrition. However good our harvest may be, let us not forget that a large part of our people are chronicallyundernourished in terms of calories as well as life-building proteins. If nothing is done about this protein scarcity’, these masses will remain condemned 10 to an incomplete and lustrelessl life.

Word-meanings :

Questions

(a) On the basis of your reading of the above passage, make notes on it in points only using abbreviations wherever necessary.
(b) Give a suitable title to the passage.
Model Answers
Abbreviations used:
1. nut. – nutrition
2. dev. — development
3. drs. – doctors
4. pop. – population
5. prob. – problem
6. ch. – child
7. ed. – education
8. emp. – employment
9. diff. – difficulties
10. mot. – motivation
11. psy. – psychological
12. camp. – campaign
13. prog. – programme

(A) Notes on the Passage:
1. Factors belonging to the nation’s health:
(a) Family planning
(b) Nut.

2. Our country’s problems:
(a) results of success and dev. e.g.
(i) Drs. responsible for pop. prob.
(ii) Birth of ch. brings anxiety.

3. Aim of family planning
(a) restore joy to motherhood
(b) provide every child health, ed. and emp.

4. Our major diff.:
(a) to create mot. and psy. climate
(b) camp. successful only in certain areas.

5. Delay or failure in family planning prog. :
(a) cause setback to all other progs.

6. Prob. of nut.:
(a) people undernourished
(b) condemned to incomplete and lustreless life.

(B) Title : Family Planning and Nutrition

Passage 5

Conversation is indeed the most easily teachable of all arts. All you need to do in order to become a good conversationalist’ is to find a subject that interests you and your listeners. There are, for example, numberless hobbies to talk about. But the important thing is that you must talk about the other fellow’s hobby rather than your own. Therein lies the secret of your popularity?. Talk to your friends about the things that interest them, and you will get a reputation for good fellowship*, charming wito and a brilliant mind. There is nothing that pleases people so much as your interest in their interests.

2 It is just as important to know what subjects to avoid as what subject to select for good conversation. If you don’t want to be set down as a wet blanket? or a bore, be careful to avoid certain unpleasant topics. Avoid talking about yourself, unless you are asked to do so. People are interested in their own problems, not in yours. Sickness or death bores everybody. The only one who willingly listens to such talk is the doctor, but he gets paid for it.

3 To be a good conversationalist, you must know not only what to say, but how to say it. Be civil and modest. Don’t overemphasizeyour own importance. Be mentally quick and witty. But don’t hurt others with your wit. Finally, try to avoid mannerism in your conversation.

Word-meanings :

Questions
(a) On the basis of your reading of the above passage, make notes on it. Use recognizable abbreviations wherever necessary.
(b) Give a suitable title to the passage.
Model Answers
Abbreviations used:
1. con. – conversationalist
2. sub. – subject
3. imp. – importance
4. mann. — mannerism

(A) Notes on the Passage :
1. To become a good con. :
(a) find sub. that interests listeners
(b) be civil and modest
(c) be mentally quick and witty

2. Avoid :
(a) talking about yourself
(b) talking about sickness and death
(c) overemphasizing your own imp.
(d) hurting others with your wit
(e) mann.

(B) Title : How to Become a Good Conversationalist

Passage 6

1 There is a big difference between the natural slavery of man and the unnatural slavery of man to man. Nature is kind to her slaves. If she forces you to eat and drink, she makes eating and drinking so pleasant that when we can afford it, we eat and drink too much. We must sleep or go mad : but then sleep is so pleasant that we have great difficulty in getting up in the morning. Thus, instead of resenting’ our natural wants as slavery, we take the greatest pleasure in their satisfaction. We write sentimental songs in praise of them.

2 The slavery of man to man is the very opposite of this. It is hateful to the body and to the soul. Our poets do not praise it; they proclaim that no man is good enough to be another man’s master. The latest of the great Jewish prophets, a gentleman named Mars, spent his life in proving that there is no extremity+ of selfish cruelty at which the slavery of man to man will stop if it is not stopped by law. You can see for yourself that it produces a continual civil war called the class war between the slaves and their masters, organised as trade unions on one side and employer’s federations on the other. We shall never have a peaceful and stable society until this struggle is ended by the abolition of slavery altogether and the compulsions of everyone to do his share of the world’s work with his own hands and brain, and not to attempt to put it on anyone else.

Word-meanings :

Questions
(a) On the basis of your reading of the above passage, make notes on it. Use recognizable abbreviations wherever necessary.
(b) Give a suitable title to the passage.
Model Answers
Abbreviations used:
1. slav. – slavery
2. nat. – natural
3. res. – resent
4. sent. — sentimental
5. soc. – society
6. imp. – impossible

(A) Notes on the Passage:
1. Slav. of nat. kind
(a) forces to eat, drink and sleep
(b) makes them pleasant.

2. Results of natural slav.
(a) we do not res.
(b) take pleasure
(c) write sent. songs.

3. Slav. of man to man
(a) hateful
(b) no poets praise
(c) no man good enough to be another man’s master.

4. Results of slav. of man to man
(a) continuous civil war
(b) peaceful and stable soc. imp.

(B) Title : Natural Slavery v/s Slavery of Man to Man

Passage 7

1. Much before medical science discovered it, Reader’s Digest’ came out with the prescription? – Laughter is the Best Medicine. Newspapers and magazines which regularly run humour columns are, therefore, doing their bit to keep the readers in good health. Reading light articles, whether they be satirical, comic or just humorous, relieves the tedium of work a-days world. Some pieces may even tickleó one’s grey matter?

2. It is said that if you laugh for ten minutes, you will be in a better position to put up with pain for two hours. According to US researchers, laughter is a good antidote8 to stress that tones up the system. Facial laugh muscles instruct the brain to ‘feel good? regardless of how you feel.

3. According to a French doctor, laughter deepens breathing, improves blood circulation, speeds up the process of tissue healing and stabilises many body functions. In short, it acts as a power drug with no side effects. Researchers state that laughter stimulates production of beta-endorphins, natural painkillers in the body and improves digestion. Those who laugh are less prone to digestive disorders and ulcers.

4. Some people in France have made it a career. You can hire a “jovialist who cracks jokes and laughs and promises to make you dissolve your worries in helpless laughter.

5 A word of caution. Although laughing is a good exercise for toning up the facial muscles, laughing at others’ expense, particularly at their disabilities, is in bad taste and is to be avoided. Secondly, laughing with food in the mouth is dangerous as the foodstuff can get into the windpipe and may choke the respiratory system.

Word-meanings :

Questions
(a) On the basis of your reading of the above passage, make notes on it. Use recognizable abbreviations wherever necessary.
(b) Give a suitable title to the passage.
Model Answers
Abbreviations used :
1. mdcn. – medicine
2. facl. – facial
3. hlth. – health
4. tdm – tedium
5. efts. – effects
6. p. killers – painkillers

(A) Notes on the Passage:
1. Laughter :
(a) the best mdcn.
(b) antidote to stress
(c) tones up facl. muscles.

2. Humorous articles in newspapers, magazines:
(a) keep readers in good hlth.
(b) relieve the tdm. of everyday life
(c) tickle one’s grey matter (intelligence).

3. A power drug with no side efts.:
(a) deepens breathing
(b) improves blood circulation
(c) speeds up the process of tissue healing
(d) stimulates natural p. killers in the body.

4. Never laugh:
(a) at the expense of others
(b) while eating.

(B) Title : Laughter, the Best Medicine

Passage 8

1. Every year, a large number of Siberian cranes come to the bird sanctuary’ at Bharatpur. Year after year, in the beginning of winter these birds cover thousands of miles from Siberia in the north of Russia to come to Bharatpur, and then, as winter ends, they once again return to their original habitat?, without making any mistake in the direction they have to take in their long flights. It is surprising how these birds find their way over such long distances, especially when vast stretches of land happen to be covered with clouds so that familiar landmarks”, which could have guided them, are not even visible. The same phenomenon“ has been observed in other parts of the world as well. By way of experiment, light metal rings with specific information are put on the legs of some migratory birds, and it is observed that they keep returning to the winter sanctuary year after year without fail.

2. Pigeons are well known for finding their way back home in spite of all odds. At one time, it was believed that they could find their way with the help of familiar landmarks which they had seen and remembered. In an experiment, some pigeons were carried to a considerable distance in cages covered with black cloth so that they could not see anything on the way. However, when they were released, they still managed to come back to the place from where they had been taken away in covered cages. To check whether the pigeons remembered the direction of movement even when they could not see anything, another experiment was undertaken. To confuse their sense of direction, some pigeons were carried in an aeroplane and then released. Many of them still managed to return home on their own. One possibility is that these birds somehow sense the direction of the magnetic poles of earth and use that to find their direction.

Word-meanings:

Questions
(a) On the basis of your reading of the above passage, make notes on it. Use recognizable abbreviations wherever necessary.
(b) Give a suitable title to the passage.
Model Answers
Abbreviations used:
1. sanc. – sanctuary
2. mis. – mistake of each winter.
3. exp. – experiments
4. yr. – year
5. dir. – direction
6. mag. – magnetic

(A) Notes on the Passage:
1. Siberian cranes:
(a) come to Bharatpur sanc. in the beginning
(b) go back at the end of winter.
(c) cover thousands of miles without making any mis.

2. Exp. prove the same birds return yr. after yr.
(light metal rings put on the legs of some birds).

3. Pigeons are known for their sense of dir. Exp.:
(a) carried to considerable distances in cages covered with black cloth.
(b) carried in an aeroplane.
(c) managed to return home on their own. 4. One possibility :
Birds somehow sense the dir. of mag. poles of the earth and use it to find dir.

(B) Title : Migratory Birds

Passage 9

1. Few animals are as useful or as unpopular as the goat. From ancient times, it has supplied people with milk and meat. Its skin has been made into leather and the wool of some breeds2 woven into soft, warm cloth. Goats are hardy creatures, and can live on the green remains of a thorny bush or a poor grassland. Nevertheless, they have always had a bad reputation. Perhaps this is because the billy goats (males) often have a bad temper and a strong, unpleasant smell. Goats also do serious damage to young trees and other plants,
and can quickly reduce a lush grazing land to a barren wasteland.

2. For its size, the goat provides man with more useful things than almost any other animal, yet it often does not receive the food and care given to other animals. The goat will try to eat anything and will put up with the most uncomfortable surroundings. But if it is well fed and carefully housed, the goat will produce much better milk, flesh and wool.

3. The goat is very closely related to the sheep. In fact, it looks very much like a sheep except for three things. It has a shorter tail which turns up instead of hanging down. Goats (both males and females) have beards and backward-slanting4 horns, whereas male sheep (rams) have curly horns. Goats have a hairy coat whereas sheep have a wolley one.

woolly one. Word-meanings :

Questions
(a) On the basis of your reading of the above passage, make notes on it. Use recognizable abbreviations wherever necessary.
(b) Give a suitable title to the passage.
Model Answers
Abbreviations used:
1. unpop. – unpopular
2. bly. – billy
3. difrs. – differs

(A) Notes on the Passage:
1. Goat-one of the most useful, yet one of the most unpop. animals.

2. Uses-meat, milk, wool.

3. Reasons of being hated:
(a) unpleasant smell of bly. goats
(b) bad temper
(c) damage to crops.

4. Positive Points:
(a) Needs least care
(b) Proper care can give better milk, wool and flesh.

5. How it difrs. from the sheep:
(a) tail
(b) horns
(c) coat.

(B) Title : The Goat and the Sheep

Passage 10

1. One question that often troubles today’s youth is the relevance of the education they are receiving. They feel disheartened by the thought that the degrees they are pursuing may not be of much help to them in earning their livelihood. So they lose interest in studies and let themselves drift with time. They do not realize that they are frittering away the golden phase of life when their mind is free from the routine worries of a householder and when they can enrich their mind with knowledge. This is also the time when they can develop their intellect and moral vision as they look at the society and the world around them with an open mind.

2 Relevance of any system of education has to be seen from three angles : (1) How far does it nurture the values that are highly respected by society ? (2) How far does it develop the individuals’ capabilities and give them confidence in themselves so that they can think and decide what is right, and can act according to that instead of always being influenced by propaganda and persuasion ? (3) How far does the system of education equip them with such skills and knowledge as would constructively help them in finding some occupation which would enable them to earn their living and satisfy their emotional needs as human beings ?

3 Our system of education may have some limitations as far as these purposes of education are concerned; however, a great deal can be achieved even from a somewhat defective system of education, if the students have an eager and open mind and are keen to learn and grow.

Word-meanings:

Questions
(a) On the basis of your reading of the above passage, make notes on it. Use recognizable abbreviations wherever necessary.
(b) Give a suitable title to the passage.
Model Answers
Abbreviations used:
1. ed. – education
2. rel. – relevant
3. att. – attitude
4. int. – intellect
5. soc. social

(A) Notes on the Passage :
1. Youth feel troubled:
(a) ed. they receive not rel.
(b) degree they pursue won’t help to earn their livelihood.

2. Harms of such an att.:
(a) waste away the golden phase of life
(b) fail to develop their int. and moral vision.

3. Relevance of ed. has to be judged by :
(a) how far it nurtures soc. values
(b) how far it develops the individuals capacities to think and decide independently
(c) How far it enables the youth to earn their living and satisfies their emotional needs.

4. Our system of ed.:
(a) may be somewhat defective
(b) if pursued with an eager and open mind, a great deal can be achieved.

(B) Title : Relevance of Education

Passages From Grammar Book (Fully Solved)

Passage 1

Social media has impacted the world in many ways. Millions of people are using Facebook, Twitter and Instagram worldwide. Such websites have proved to be the best way to connect and communicate with friends and family. These have also become the best tool to keep oneself abreast with latest buzz in every field. These are so convenient that one tends to forget that such tools are to be used very judiciously?. One needs to learn to be a responsible social media user. Especially young children must be taught to use social media very carefully. It is the responsibility of the parents and teachers to teach them proper use of social media.

Facebook started by Mark Zukerberg is the most used social media tool. Its business depends on persuading* people into sharing their personal experiences with public or their known people. Posting pictures, updating status has become an important part of their daily routine. No one is immune to its addictive effect. Periodically looking at their phones for any updates has become a norm for people. Users must be made aware of the fact that sharing personal details and photos can be misused by criminals or antisocial elements.

One must understand that every post on Facebook or such sites is not always backed by facts or truth. One must not make friends with unknown persons. Time and effort spent on social media can be used productively. Some people may use social media to spread religious fanaticism? or hatred. Social media should be used to help connect with people, not to disconnect or disintegrate from mainstream. It should be used to bring harmonys and love only.

Word-meanings:

Questions
(a) On the basis of your reading of the passage, make notes on it. Use recognizable abbreviations wherever necessary.
(b) Give a suitable title to the passage.
Model Answers
Abbreviations used :
1. wldwd.- worldwide
2. effve. – effective
3. jdes. – judicious with near ones
4. respl. – responsible

(A) Notes on the Passage:
1. Social media like Facebook, Twitter, etc.:
(a) popular wldwd.
(b) effve. means to remain in contact
(c) help us to keep abreast of current events.

2. Some cautions while using them :
(a) shouldn’t forget to make a jdcs. use of them
(b) should be respl. social media users
(c) young children to be taught their correct use.

3. The advantages of social media:
(a) sharing personal experiences
(b) can be shared with general public also.

4. Some grave possibilities:
(a) misuse by criminals
(b) not based on facts
(c) unknown / suspicious persons can become friends.

5. Useful advice:
(a) shouldn’t spend much time on the social media
(b) must not be influenced by fanaticism or hatred
(c) should be used to spread harmony and love.

(B) Title : Social Media — a Boon or a Bane?

Passage 2

Windows give the character to a house, just as final features reflect the personality in a face. Small changes in style, finish or proportions of windows can destroy the spririt of home. Consider carefully the effect that changes will make to the outside as well as the inside of the building. Until the seventeenth century, most windows were of the four-panel casement type. They opened outwards (or inside) like a door on butt hinges. The particular irregularity of the glass is important, and such windows are ruined by insensitive and mechanical metal casements and phoney lead lights.

Thereafter, sash window became more common in Britain and America; usually they open vertically, but horizontal-sliding sash windows have a peculiar charm and work well in wide, low frames. Today, we see a great use of glass, but originally windows were busily divided by mullions. The fine art of fenestration seems to have fallen by the wayside. Proper leaded lights, decorative Geogrian fanlights, and the dignified proportions conveyed by a satisfying geometry of wooden mullions seem to be a thing of the past. Replacing old windows with modern versions is almost always to the detrimento of house. Unfortunately, almost all double-glazing is hideous and metal-frames windows are heinous; terrible crimes are committed in the name of fuss-free windows. And blocking in a window or a glazed door can have a quite disproportionate effect on the light within – look closely at the path of light shed by the glazing in question before you decide to remove it.

Word-meanings:

Questions
(a) On the basis of your reading of the passage, make notes on it. Use recognizable abbreviations wherever necessary.
(b) Give a suitable title to the passage.
Model Answers
Abbreviations use:
1. chrct. – character
2. dest. — destroy
3. cfly. – carefully
4. otwds. – outwards
5. inwd. – inward
6. fnstn. – fenestration

(A) Notes on the Passage:
1. Importance of windows:
(a) give chrct. to house
(b) changes in finish & proper, can dest. spirit of house.
(c) effect of changes must be cfly. considered.

2. The windows in the seventeenth country:
(a) opened otwd. or inwd. like a door.
(b) such windows common in Britain & America.

3. Scenario today:
(a) people used to glass grates
(b) fine art of fnstn. has given way.

(B) Title : Significance of the Arrangement of Windows in a Building

Passage 3

In negotiation’, tactics and strategy are many a time mixed up. In simple words, tactics means negotiating small-scale and short-term interim? issues whereas strategy is about large-scale, longterm and final outcomes. For example, the strategic decisions about a journey will be about where the journey is from and to, the mode of travel – car, train, plane or boat – and when the journey takes place. On the other hand, from a tactical point of view, you would think of route and the terrain, the kind of traffic and other details regarding the nature of interaction with different people enroute this journey. So in negotiations, strategically you are more focused on the outcomes of your journey and tactically you think how to react to a situation or an action.

To be a successful negotiator, you need to take into account both these factors. We must choose them with care and with due regard for the outcome that we yearn for. Also they must be very effectively used. Having common interests with the negotiator will be an added advantage while making a negotiation. Such strategy leads to mutually beneficial agreements that can result in great satisfying needs of all parties. Such a strategy will require a high level of trust and a willingness to share ideas and make adjustments.

Word-meanings:

Questions
(a) On the basis of your reading of the passage, make notes on it. Use recognizable abbreviations wherever necessary.
(b) Give a suitable title to the passage.
Model Answers
Abbreviations used:
1. strgy. – strategy
2. otcm. – outcome interim issues
3. undtk. – undertake
4. ptcl. – particular

(A) Notes on the Passage:
1. In any negotiation :
(a) a mixture of tactics and strgy.
(b) tactics pertain to short-term
(c) strategy – the name given to long-term otcms.

2. Strategic decisions explained with journey as an example:
(a) will pertain to starting and finishing points
(b) will be about the mode of travel
(c) may be about the best time to undtk. the journey.

3. From the tactical point of view :
(a) one would think of route and terrain
(b) one would take into account the traffic, etc.
(c) the nature of interaction with people enroute will have to be considered.

4. Essentials to be a successful negotiator :
(a) need to be focused on the otcms. of the journey
(b) must know how to react in a ptcl. situation
(c) must choose them with care for a desired otcm.

5. Added advantages can be had by :
(a) an effective use of both – strgy. and tactics
(b) having common interests with the negotiator
(c) such strgy. will bring satisfaction to all parties.

6. Requirements of such a strgy.:
(a) a high level of mutual trust
(b) a ready willingness to make adjustments.

(B) Title : Negotiation – How it is Akin to a Journey

Passage 4

Swami Vivekanand wrote: We say, “Newton discovered Gravitaion. Was it sitting anywhere in a corner waiting for him?” It was in his mind. The time came and he found it out. All knowledge that the world has ever received comes from the mind. The infinite library of the universe is in your own mind. The external world is only the suggestion, the occasion’ which sets you to study your own mind. “No one was never really taught by anyone. Each of us has to teach himself. The external teacher offers only the suggestion which rouses the internal teacher to work to understand things. You cannot teach a child any more than you can grow a plant. The plant develops its own nature’. The child also teaches itself. But you can help him to go forward in his own way. Parents are constantly taxing their children to read and work, telling them that they will never learn anything and calling them fools and so forth”; the latter do actually turn out to be so in many cases. If you speak kind words to them and encourage them, they are bound to improve in time.

Word-meanings :

Questions
(a) On the basis of your reading of the passage, make notes on it. Use recognizable abbreviations wherever necessary.
(b) Give a suitable title to the passage.
Model Answers
Abbreviations used :
1. grvtn.- gravitaion
2. opprn.- opportune
3. knlge. — knowledge
4. lib. – library
5. extl. – external

(A) Notes on the Passage:
1. Swami Vivakanand said about gravtn.
(a) was always there
(b) was not waiting in a corner
(c) in fact, was in Newton’s mind
(d) he found it at the opprn. moment.

2. What he said about the mind:
(a) all knlge. comes from the mind
(b) it is the infinite lib. of the universe
(c) extł. world only a suggestion, an occasion.

3. About teaching:
(a) no one can teach another
(b) everyone of us has to teach himself
(c) an extl. teacher can only offer a useful help.

4. Similarity between a child and a plant:
(a) a plant develops its own nature
(b) a child teaches itself.

5. What can we do to help a child ?
(a) should not tax our children to read and work.
(b) should not always call them witless or fools.
(c) If done so, they may actually turn into fools.
(d) should treat them kindly and always encourage them.
(e) This done, they are bound to improve in time.

(B) Title : The Mind – its Boundless Potential

Passage 5

The energy conservation’ in street lighting is a new concept. But various technologies applied to achieve the goals are not new. It has been adopted and tested in various Industrial and Medical applications from decades. So these technologies are trustworthy’, when adopted at street lighting energy conservation. This will yield results in conserving the electrical energy. However, when such technologies are applied in public domain”, the measurement and verification procedures should be strong and should be in place before implementing such projects. In case of energy efficiency projects in Karnataka, there was no baseline of energy consumption.

As a result, it has become very difficult to realize the savings on records. So without baseline reference, crystalline realization of Energy Savings is impossible. These projects were implemented for a portion of the entire town / city. The maximum area covered under the project is about 20% of the total city lamp population. Under such circumstances, it is difficult to bifurcate and analyse the bills. Moreover, billing pattern from electricity companies to the municipalities is very erratic and needs lots of modificaitions.

Word-meanings:

Questions
(a) On the basis of your reading of the passage, make notes on it. Use recognizable abbreviations wherever necessary.
(b) Give a suitable title to the passage.
Model Answers
(A) Notes on the Passage:
1. Energy cons. in street lighting:
(a) a new concept
(b) various techs. applied
(c) techs. are t.worthy.

2. When applied in public domain:
(a) should be in place before impmtg.
(b) Karnataka – no baseline in e.s.
(c) without baseline no e.s. possible

3. Project implementation in city / town:
(a) only 20% area covered
(b) not easy to bifct. & analyse bills
(c) bill patn. very erratic.

(B) Title : Energy Conservation in Street Lighting

Abbreviations used:
1. cons. – conservation
2. techs. – technologies
3. t.worthy. – trustworthy
4. impmtg. – implementing
5. e.s. – energy saving
6. bifct. – bifurcate
7. patn. – pattern

Passages For Practice

Passage 1

1. “Reading comprehension’ is the ability to know and understand what has been read. For example, when you read a passage about trains, you learn certain facts about this form of transport. Once you have finished reading, your teacher may ask you questions to find out how well you understood the passage. The meaning of a passage lies within the material being read, and you may understand or comprehend the meaning when you read a given passage. This can be termed as local comprehension.

2. Recently another idea about reading comprehension has become more common. Instead of simply asking the reader what meaning he or she has obtained from a given passage, a teacher may stress the importance of what ideas the passage stimulates in the reader’s mind. These ideas or these feelings are influenced by the reader’s own experience of what he or she is reading about. So when you read about trains, you may remember some of your own experiences when you were once travelling on or looking at trains. Words such as ‘engine’, ‘station’, or ‘passenger’ have their own special meaning for you.

For one person, an article about trains might bring memories of the time when a train he was in was three hours late because of a terrible storm. Another person might remember a train journey to a hill station during a holiday. A third person might recall4 a story she read about an exciting railway trip in a foreign land. Each reader ‘comprehends’ in a different manner. This can be termed as ‘global comprehension.

Word-meanings:

Questions
(a) On the basis of your reading of the above passage, make notes on it. Use recognizable abbreviations wherever necessary.
(b) Give a suitable title to the passage.

Passage 2

1. Many people enjoy collecting things as a hobby. If you already have several costume dolls (i.e. dolls with dresses of particular period), or a pile of old comics, or a scrapbook containing picture cards, then you are already a collector. Collecting need not take up a lot of time, or cost a lot of money, but it can be rewarding and informative pastime. Everywhere you go, you may come across something new to add to your collection.

2. It does not really matter? what you collect. You can collect model aircraft, badges, postcards, glassware, pottery, matchbox labels, sports programmes, autographs, rocks, fossils”, and practically anything small enough to bring home and keep in a box or drawer. As your collection grows4, you will become more expert in the subject that most interests you. Yet, the more you know, the more there will be still to discover. You will make friends with other collectors, and perhaps join a collectors’ club or society.

3 A collection need not be of costly things. A scrapbook filled with newspaper cuttings and holiday photogaphs will grow in interest and value with the passing years. If it is kept safely, it will be a treasure store of interest, not just for you when you are older, but perhaps for your children and grandchildren in years to come. 4 Simple everyday things (food cans, magazines, buttons, and toys are examples) can become collector’s items in future. Some may become valuable. Greeting cards made in the 19th century, for instance, are now carefully collected. In a hundred years’ time, someone may be keenly interested in your last year’s birthday cards !

Word-meanings:

Questions
(a) On the basis of your reading of the above passage, make notes on it. Use recognizable abbreviations wherever necessary.
(b) Give a suitable title to the passage.

Passage 3
1. How does television affect our lives ? It can be very helpful to people who carefully choose the shows that they watch. Television can increase our knowledge of the outside world; there are high-quality programmes that help us understand many fields of study; science, medicine, the arts and so on. Moreover, television benefits very old people, who can’t often leave the house as well as patients in hospitals. It also offers non-native speakers the advantages of daily informal language practice. They can increase their vocabulary and practise listening.

2. On the other hand, there are several serious disadvantages of television. Of course, it provides us with a pleasant way to relax? and spend our free time, but in some countries, people watch the ‘boob-tube’ for an average of six hours or more a day. Many children stare at a television screen for more hours each day than they do anything else, including studying and sleeping. It is clear that the tube has a powerful influence on their Yives and that its influence is often negative.

3. Recent studies show that after only thirty seconds of television, a person’s brain ‘relaxes’ the same way that it does just before the person falls asleep. Another effect of television on the human brain is that it seems to cause poor concentration4. Children who view a lot of television can often concentrate on a subject for only fifteen to twenty minutes. They can pay attention only for the amount of time between commercials.

Word-meanings :

Questions
(a) On the basis of your reading of the above passage, make notes on it. Use recognizable abbreviations wherever necessary.
(b) Give a suitable title to the passage.

Passage 4

1. Tourism has emerged as the world’s largest industry. Growing rapidly in the last two
decades’, today it accounts for 6% of world output? and employs some 100 million people around the globe. Since the end of the Second World War, it has developed immense revenue and development potential* and stands today as a unique natural renewables
resource industry.

2. Tourism – the travel-based recreation – provides people with a change of place and a break from the monotony? of daily life. It brings people of different nations together, allowing them to come into close contact with each other’s customs and other aspects of life. It reveals the scenic beauty8 and past heritage of a country of people belonging to other nations. The knowledge and experience gained in the process can lead to greater understanding and tolerancelo, and can even foster world peace.

3. The contribution of tourism can be seen nowhere more clearly than on the economic front. A study conducted by the United Nations has shown that developing countries, in particular, can reap handsome benefits out of tourism which greatly boosts national income. 4 Tourism generates employment, and adds to the entrepreneurial wealth of a nation. While tourism’s advantages are many, its undesirable side effects have raised fresh problems.

Word-meanings:

Questions
(a) On the basis of your reading of the above passage, make notes on it. Use recognizable abbreviations wherever necessary.
(b) Give a suitable title to the passage.

Passage 5
1. It’s headache having a headache. Almost all of us have suffered from a headache at some time or the other. For some, a headache is a constant companion and life can be a painful hell of wasted time.

2. The most important step to cope with headaches is to identify the type of headache one is suffering from. In tension headaches (two-hand headache), a feeling of a tight band around the head exists along with pain in the neck and shoulders. It usually follows activities such as long stretches of driving, typing or sitting on the desk. They are usually short-lived but can also last for days or weeks.

3. A headache is usually caused due to spinal misalignment of the head and due to poor posture. Sleeping on the stomach with the head turned to one side and bending over positions for a long time make it worse.

4. In migraine headaches (one-handed headaches), the pain is usually on one side of the head and may be accompanied by nausea”, vomiting, irritability and bright spots of flashes of light. This headache is made worse by activity, especially bending. The throbbing pain in the head gets worse by noise and light. Certain triggers for migraines may be chocolate, caffeine”, smoking or MSU in certain food items. The pain may last from 8 to 24 hours and there may be a hangover’ for two-three days.

5. Migraines are often preceded by an ‘aura’ – changes in sight and sensation. There is usually a family history of migraine.

Word-meanings:

Questions
(a) On the basis of your reading of the above passage, make notes on it. Use recognizable abbreviations wherever necessary.
(b) Give a suitable title to the passage.

Passage 6

1. Properly planned and executed’ holidays can tone up2 both mind and body. But many of us don’t know how to take a holiday. Good planning is all-important. A leading clinical psychologist reports that many people seek his help after poorly planned holidays that have led to family quarrels. Instead of making their holiday a period of rest, development of understanding and love for their families, people try to block everything out with ‘funpacked’ activity. When the ‘fun’ is over, they return in worse shape, mentally and physically,
than when it began.

2. Here are other common holiday errors which, as a doctor, I caution against : some of us delude ourselves into thinking that a quick holiday will give instanto relaxation and get us back on the track. Most people need a full three weeks or longer. To get any lasting good out of your holiday, you must have a change of pace and scene. That is one reason why travel can be so helpful. Thus, spending your entire holiday at home may be a mistake. Another common error that tourists often make is trying to see and do far too much. To be able to live in peace and quiet for a time is a mark of maturity. It gives you a chance to think about your family and yourself.

3. A holiday’s success is measurable by the results it produces. A good one is often marked by relaxed muscles, more satisfying sleep, slower heart rate and reduction in blood pressure.

Word-meanings:

Questions
(a) On the basis of your reading of the above passage, make notes on it. Use recognizable abbreviations wherever necessary.
(b) Give a suitable title to the passage.

Passage 7
1. Contrary to popular belief, the camel does not store water in its hump?. The hump is a food reserve composed mainly of fat. Although water is produced when the fat is broken down, the oxygen used in the process causes an additional loss of water through the lungs. This more or less cancels the amount of water gained from the hump. The main advantage of the hump is that the camel’s fat is concentrated in a single place – allowing the camel to lose heat freely from the rest of its body.

2. Another way in which camels conserve water is by retaining the urea which most mammals excrete in their urine. The urea is built up into proteins by bacteria in the camel’s stomach.

3. In addition, camels avoid sweating by allowing their body temperature to vary over a greater range than that of any other mammal. Camels do not begin to sweat until their body temperature reaches 46°C. After a lengthy period without water, camels will drink up to 180 litres at a time. They swell visibly in the process, and dilute their blood and tissue fluids to an extent that would kill most other mammals. Part of the explanation for the camel’s prodigiouss drinking ability may be the unusual shape of its blood corpuscles. They are oval rather than round. When it drinks, its blood as well as its tissues can absorb large amounts of water because the oval corpuscles can swell into spheres without any danger of the cell-walls bursting.

Word-meanings:

Questions
(a) On the basis of your reading of the above passage, make notes on it. Use recognizable abbreviations wherever necessary.
(b) Give a suitable title to the passage.

Punjab State Board PSEB 11th Class English Book Solutions English Notice Writing Exercise Questions and Answers, Notes.

PSEB 11th Class English Notice Writing Speech

A notice is a publicly displayed written or printed information of something about to happen or that has happened. It is a kind of information meant for others to know and follow.

Always remember-

1. The language of a notice should be impersonal. It should be written in the third person.
2. First and second person Pronouns like T and you should never be used.
3. The notice must be put in a square box.
4. It must contain complete information.
5. The purpose of the notice must be made very clear.
6. Date of writing the notice should also be mentioned.
7. It should carry all necessary information.
8. It must have a signature and the designation.

A Specimen of Notice-writing

You are the Secretary, Cultural Club of your school. The school is celebrating its Annual Day on the 25th of February. Draft a notice to be put up on the noticeboard of your school, informing the badge holders and house captains of a meeting you are going to conduct to take decisions on some important matters regarding the conduct of the programme. The notice should not exceed 50 words.

Examination-Style Examples of Notices

1. A debate on the uses and abuses of mobile phones is being organised in your school on 18 April 20 ………. The Education Minister will be the chief guest. All the students of classes 8th to 12th are invited to participate. The Principal of the school has asked you, the student secretary of the cultural committee, to inform the students about the programme and to ask the interested participants to give their names to Mr Singh, the English teacher.

Write a notice for the students’ noticeboard, inviting the students to give their names within four days. Put the notice in a box.
Answer:

Notice

10 April 20 …….

A debate on the uses and abuses of mobile phones is being organised in our school on 18 April 20……. . The Education Minister will be the chief guest. All the students of classes 8th to 12th are invited to participate. Those interested to take part in the debate should give their names to Mr Singh, the English teacher, within four days.

Avinash Kapoor
Student Secretary
Cultural Society

2. Samson of class X has just passed his annual examination. Two of his books are in a fairly good condition and he wants to sell them at reduced prices. He puts up a notice on the school noticeboard, giving all the necessary details. Write the notice, using not more than 50 words. Put the notice in a box.
Answer:

Notice

18 April 20 …….

Second-Hand Books For Sale
High School English Grammar
Tulip English Reader, Book 10.

The above two books are in a very good condition and can be had at only 50% of their printed price. Those interested may please contact the undersigned.

Samson
Class XI-A
(Last year in X-A)

3. A boy is missing. The police are trying to find him. They ask his parents about him. The police have to draft a notice to he published in a newspaper about the missing boy. Draft the notice in not more than 50 words. Put the notice in a box.
Answer:

Notice

18 April 20 …….

The Missing Boy

A boy, aged twelve, of fair complexion and tall thin body, has been missing from the Krishna Nagar locality of the town since Monday evening. The boy was last seen in the Nehru Park and was wearing a striped shirt with blue jeans. Anyone who can provide a clue in tracing the missing boy will be suitably rewarded. Such persons can inform the nearest police station or ring up 100.

4. Mr Vivek Kumar, a child psychologist, wants to open a nursery school. He asks you to draft a notice on his behalf to be published in a newspaper. Give necessary details without any extra information.
Answer:
25 January 20………

Lovedale Nursery School
Admission Notice

No one can train your child better than a child psychologist. And here at Lovedale Nursery School, your child will learn and grow under the direct guidance of a child psychologist in a healthy child-friendly environment. Admission starts from the 15 th of February. Admission forms can be had from the school office between 10.00 a.m. and 2.00 p.m. on ail working days.

5. Meenakshi Goel is the Sports Captain of Navodava School. The sports day is to be held on March 21st which is just two weeks away. She has to inform House Captains that they have to submit the names of the participants to her in a week’s time. A student cannot take part in more than three events excluding the relay. Any delay or wrong information will lead to disqualification of the student.
Write the notice for Meenakshi, using not more than 50 words. Put the notice in a box.
Answer:

Notice

7 March 20 …….

Navodaya School, Rampur Participants For Sports Day Events

For the Sports Day to be held on 21st March, House Captains should submit the names of the participants in a week’s time. No student can take part in more than three events, excluding the relay. Any delay or wrong information will lead to disqualification.

Meenakshi (Sports Captain)

6. You are Anupam, the secretary of the school quiz club. You want to hold an inter¬class competition to decide on the entries for the Inter-school Quiz Competition to be held 2 weeks from now. Draft a notice for the students’ noticeboard, inviting the intending participants. Mention all details required like entry dates, prizes, etc. Give your notice an appealing heading. Put the notice in a box.
Answer:

Notice

7 March 20 …….

Prizes Through Quizzes

The Inter-school Quiz Competition is going to be held two weeks from now. In. order to decide on the entries for the same, we have decided to hold an inter¬class competition on Monday, the 15th. All those who desire to participate should give their names to the undersigned by tomorrow. The best three participants shall be awarded free school blazers.

Anupam
(Secretary, School Quiz Club)

7. You are Rubina, the Head Girl of City Public School, Moga. You were given the following letter and told to put up a notice on the school noticeboard. Including the details from the letter, write the notice in not more than 50 words. Do not give any extra information. Put the notice in a box.

Arpana Clinic
Rajaji Street
Moga
20 February 20 ………
The Principal
City Public School
Moga
Sir

I acknowledge the receipt of your letter and accept your invitation to speak to the children on dental care. Your efforts to make children aware of dental hygiene should be appreciated.

The 25th of this month would be a convenient day for me. I will speak to them for an hour and make it interesting through demonstrations and slides. I hope you will inform them of the same.

Thank you very much
Yours faithfully
Dr Celine
Answer:

Notice

21 February 20 ………..

City Public School, Moga Lecture On Dental Care & Hygiene

Dr Celine, the eminent dentist of the city, has very kindly agreed to give a lecture on dental care and hygiene. The lecture will be held on the 25th of this month in the school hall, at 11.30 a.m. It will be an interesting and useful lecture through demonstrations and slides. All are requested to attend.

Rubina
(Head Girl)

8. You are Sharat, the President of the Interact Club of your school. You wish to organize a snack bar on the Sports Day of your school. The proceeds of the sale will go for charity. You have to put up a notice on the noticeboard of your school, calling for a meeting to discuss the same. Draft the notice, giving all the details in not more than 50 words. Put the notice in a box.
Answer:

Students’ Noticeboard

23 March 20 ………..

It has been desired by some members of the Interact Club that a snack bar should be organised on the Sports Day of the school. The Principal has given his consent. The proceeds of the sale will go for charity to the local orphanage.

In order to discuss about the same, a meeting will be held in the club office on the 27th of this month in the recess period. All the members are requested to attend the meeting.

Sharat
(President)
Interact Club

9. You are the Student Secretary of your school. You are asked by the Principal of the school to inform students of classes 8th to 12th about an Inter-school Debate Competition to be held in your school on 5th April 20 Draft a suitable notice for the same.
Answer:

Notice

25 March 20 ……….

Blossoms Public School, Ludhiana

An Inter-school Debate Competition for the students of classes 8th to 12th will be held in our school on 5th April 20 The topic of the debate will be ‘Caste-Based Reservation : a Bane or a Boon. Those who want to take part in this debate should give their names to the undersigned within three days.

Munish Verma
(Student Secretary)

10. You are Akhil / Akanksha, the Head Boy / Girl of Modern Public School, Raikot. As part of social service, your school has decided to clean the surroundings. Write a notice, in not more than 50 words, for your school noticeboard, asking for the names of the students of senior wing who wish to participate wholeheartedly.
Answer:

Notice

20 April 20………..

Modern Public School, Raikot

The school has decided to clean its surroundings as part of social service on 30 April 20 ……. The students of senior wing who wish to participate in it are requested to give names to the undersigned by the 25th of this month. The participants must be willing to work wholeheartedly in this social cause.

Akhil
(Head Boy)

11. You are Salman / Salma of St. Joseph s School, Ramnagar. Your school is organizing a charity show in aid of the victims of recent floods. Write a notice to be put up on the school noticeboard, inviting names of the students from classes IX-XII interested in participating. Also urge them to contribute generously to the cause. Write the notice in not more than 50 words.
Answer:

Notice

20 April 20……….

St. Joseph’s School, Ramnagar

The recent floods in the stare have caused a great havoc. The school has decided to organize a charity show to collect funds for the victims of these floods. The students of Classes IX to XII who are interested in participating should give thqpr’ names to the undersigned by the 25th of this month. Ail students are also requested to donate generously for this noble cause.

Salman
(Head Boy)

Exercise From Grammar Book (Fully Solved)

1. You are Amrinder, the Secretary of the Eco Club of your school. Write a notice for the students about the celebration of ‘Ban Plastics Day’ to create awareness regarding the dangers of using plastic products. Put the notice in a box.
Answer:

N. M. Oswal Public School, Jagraon
Notice
Eco Club

24 December 20 ……….

To create awareness regarding the dangers of using plastic products, the Eco Club of the school is going to hold the celebration of ‘Ban Plastics Day’ on 30 December 20 …….. on the school grounds, at 11.00 a.m. Eminent environmentalists will deliver lectures on the dangers posed by plastics. The students of all classes have to be present there. They can bring with them posters, articles and placards against the use of plastics.

Amrinder
Secretary

2. You are Krishna, the Head Boy of Guru Nanak Public School, Nabha. You found a tiffin box in the playground. Draft a notice for the school noticeboard. Put the notice in a box.
Answer:

Guru Nanak Public School, Nabha
Notice
Lost And Found

30 March 20 …………

A tiffin box has been found in the school playground. It is of brown colour and is of Milton make. The owner can collect it from the school office after proving his / her ownership of the item.

Krishna
(Head Boy)

3. You are Priya, the Head Girl of S.D. Public School, Moga. Your school is organizing its Annual Day Function. Your school Activities In-charge has asked you to call a meeting of all the members of the School Students’ Council to allocate different duties for the Annual Day Function. Draft a notice for all members to attend the meeting. Put the notice in a box.
Answer:

S. D. Public School, Moga
Notice
Annual Day Function

4 Februaiy 20 ………..

Our school is organizing its Annual Day Function on the 25th of February. For this purpose, a meeting of all the members of the School Students’ Council has been called for allocating different duties for the Function tomorrow at 10.00 a.m. in the office of the Activities In-charge. All the members must attend the meeting.

Priya
(Head Girl)

4. Suppose you are Akshay. You are the Secretary of Arya Model Senior Secondary School, Ludhiana. Write a notice for students, informing them about the details of an Inter¬class Science Quiz to be organized in your school.
Answer:

Arya Model Sen. Sec. School, Ludhiana
Notice
Inter-Class Science Quiz

5 September 20………..

This is to inform all the students that an Inter-class Science Quiz is going to be held on the 20th of this month. Dr Narendra Gautam, the famous scientist, will act as the judge. Those who wish to take part in the Quiz should give their names to the undersigned by the 15th of this month.

Akshay
(School Secretary)

5. Suppose you are Navneet, the President of the Readers’ Club of the your school. ‘Penguin Books’ is organizing a book fair in your school to promote the habit of reading among students. Draft a notice, inviting the students to visit this fair and get the best books. Put the notice in a box.
Answer:

A. B. Sen. Sec. School, Kapurthala
Notice
A Book Fair

14 May 20 ……….

With a view to promoting the habit of reading among students, the famous publishers, ‘Penguin Books’, are organizing a book fair in our school from the 20th to 23rd of this month. Books on all possible subjects will be on display. Books will be available for purchase on maximum discount. Therefore, the students are advised to visit this book fair in maximum numbers and buy the books of their choice.

Navneet
President
Readers’ Club

6. You are Muskan. You are the Secretary of ‘Literacy Club’ of Government Senior Secondary School, Bhim Nagar, Moga. Your school is organising a lecture by famous English author, Ruskin Bond, during the ‘Literacy Week’ being organized in your school. Draft a notice, informing the students about the lecture.
Answer:

Govt. Sen. Sec. School, Bhim Nagar, Moga
Notice
Lecture By Ruskin Bond

25 November 20 ………

Our school is going to organize a lecture by the famous English author, Ruskin Bond, during the ‘Literacy Week’ which will be celebrated by the school from 30 November 20…….. to 5 December 20 …….. Students will do well to attend this lecture in maximum numbers. This is bound to be a very educative lecture. Don’t miss it.

Muskan
Secretary
Literacy Club

7. You are Mohan, Sports Secretary of R.S.D. College, Firozpur. Your school is organizing a T-20 match with a nearby college. Draft a notice for the students, giving them details about the time and venue, etc.
Answer:

R.S.D. College, Firozpur
Notice
T-20 Cricket Match

27 October 20 ……….

Our college is organizing a T-20 cricket match against the local Khalsa College. The match will be played on the 30th of October on the college grounds. This will be a very interesting match. Timing of the match will be from 3.00 p.m. onwards. Students are requested to come and watch this match in maximum numbers.

Mohan
(Sports Secretary)

8. Your District Transport Officer has issued an advisory for a week-long Anti-Pollution Drive in your district. Draft a notice about it for the general public.
Answer:

District Transport Officer, Moga
Public Notice
Anti-Pollution Drive

16 June 20 ………

This is to inform the general public that a week-long Anti-Pollution Drive will be launched from the 20th of June to the 25th of june. During this drive, the pollution control certificate of every vehicle will be checked.

Narottam Purohit
(D.T.O, Moga)

9. The Northern Railways, Ambala, has issued a public notice for some special trains for the comfort of passengers arranged during the celebration of the ‘Kumbh Mela’ at Kurukshetra. Draft the notice, giving the details of the special trains and their schedule.
Answer:

Northern Railways, Ambala
Public Notice
Special Trains For Kumbh Mela

17 June 20 ………

Northern Railways have decided to run special trains to cope up with the passengers’ rush during the Kumbh Mela that will be held in Kurukshetra. These are the names and numbers of the special trains : 1006 Up Kurukshetra Express, 3006 Up Pilgrim Express and 0211 Down Shalimar Express. The dates and timings of these trains can be obtained from the Railway enquiry number 139.

Sd/ ……..
(Commercial Superintendent)

10. As the Head Girl of your school, you are organizing a Career Counselling Seminar for the students of classes XI and XII. Draft a notice for the students, giving details about the timing and venue.
Answer:

S.D. Vidya Manoir, Jalandhar
Notice
Career Counselling Seminar

18 July 20 ………..

A Career Counselling Seminar is being organized in the school on 20 July 20 ……… Famous Career Counseller, Vidya Devi, has graciously consented to preside over the seminar. This is a great opportunity for the students seeking career in different fields. The students of XI and XII classes should particularly make it a point to attend the seminar. Timing of the seminar will be from 3.00 p.m. to 5.00 p.m.

Paramjit Kaur
(Head Girl)

11. You are Aarti, the Secretary of the Social Welfare Club of Saraswati Vidya Mandir, Khanna. Your school is organizing a special cultural show in aid of Children with Special Needs of your city. Draft a notice, informing the schoolchildren about the details of this programme.
Answer:

Saraswati Vidya Mandir, Khanna
Notice
Special Cultural Benefit Show

20 July 20 ……….

Our school is organizing a special cultural benefit show in the aid of Children with Special Needs (CWSN) of our city on 25 July 20 . In this show, skits, songs, jokes and folk dance will be presented by the children of our school. Tickets for this show are available with the school office. The money collected through the show will be sent to the Composite Rehabilitaion Centre of our city. All people are invited to this show. Venue for the show is the auditorium of our school. Timing of the show is 4.00 p.in. to 7.00 p.m.

Aarti
Secretary
Social Welfare Club

12. You are Yogesh, the Head Boy of Jain Public School, Bamala. Draft a notice regarding a bicycle found in the school campus.
Answer:

Jain Public School, Barnala
Notice
Lost And Found

20 August 20………

A bicycle was found in the school campus yesterday. It is an Avon cycle of black colour with red seat cover. Whosoever is the owner of the bicycle should collect it from the school office during working hours.

Yogesh
(Head Boy)

13. You are Lovepreet, President of Guru Gobind Singh Apartments Society, Mohali. There is a serious problem of frequent power failure in your society. You want to organize a meeting to find a solution to this problem. Draft a notice for all residents, inviting them to attend the meeting.
Answer:

Guru Gobind Singh Apartments Society, Mohali
Notice

26 October 20 ……….

The residents of Guru Gobind Singh Apartments Society are nowadays facing the problem of frequent power failure. To find a solution to this problem, a meeting of the residents is being organized tomorrow at 6.00 p.m. in the Community Centre. All residents should make it a point to attend the meeting.

Lovepreet
President

14. You are the President of Eco Club of your school. Draft a notice, requesting the students to keep the school campus clean and green.
Answer:

Arya Sen. Sec. School, Hoshiarpur
Notice

17 November 20 ……..

It has been found that some students are indulging in making the school look dir|y by throwing paper scraps and other rubbish here and there and destroying the plants. It tarnishes the image of the school. The students should think it their duty to keep the campus clean and green. They should treat the school as their second home. If they don’t mend their ways, strict action will be taken against such students.

Raman
President
Eco Club

15. You are Sujan Singh, Executive Engineer with State Highways Authority, Punjab. Draft a tender notice for the widening of the road between Chandigarh and Ludhiana.
Answer:

State Highways Authority, Punjab
Tender Notice

15 March 20……….

The road between Chandigarh and Ludhiana is to be widened. For this purpose, State Highways Authority, Punjab, invites tenders from reputed road-construction firms. Sealed tenders should reach the headquarters of State Highways Authority, Punjab, at Chandigarh within 15 days from the publication of this notice.

Sujan Singh
(Executive Engineer)

16. Suppose you are the Principal of Government Senior Secondary School, Dhaliwal. Your school needs some new desktop computers. Draft a tender notice for the supply of the desktop computers..
Answer:

Govt. Sen. Secondary School, Dhaliwal
Tender Notice

20 April 20……….

Government Senior Secondary School, Dhaliwal, requires 20 desktop computers for its new computer laboratory which is under construction. For this purpose, tenders are invited from reputed computer firms. Sealed tenders should reach the Administrative Officer of the School by 5 May 20………

Sdl-
(Principal)

17. Suppose you are the Principal of Swami Vivekanand School, Jalandhar. Your school wants to dispose of some of the old desktop computers and printers. Draft a tender notice for the sale of the old desktop computers and printers.
Answer:

Swami Vivekanand School, Jalandhar
Tender Notice
Sale Of Old Computers

27 August 20 ……..

The school has many old desktop computers and printers. The school intends to dispose of them. For that purpose, tenders are invited from the firms that deal with second-hand things. Sealed tenders should reach the office of Swami Vivekanand School, Jalandhar, within ten days from.the publication of the tender notice.

Sd/…….
(Principal)

18. You are the Property Incharge of your school. Your school is constructing a new classroom. Draft a tender notice for the supply of construction material for the room.
Answer:

Nyaan International School, Rajpura
Tender Notice

6 February 20………..

A new classroom is to be constructed for the new Senior Secondary Wing of the school. For that purpose, tenders are invited from the construction material suppliers. Sealed tenders should reach the office of the school by the 10th of this month.

Gurdarshan Singh
(Property Incharge)

19. Your father has left an old Fiat car for you. You don’t need it. So draft a notice for its auction.
Answer:

Auction Notice
Old Car For Sale

25 September 20………

As I am going abroad soon, I wish to dispose of my Maruti Swift car as soon as possible. It is a 2011 model of white colour. It has done 7000 kms and is accident- free, fully insured and in a very good running condition. Its auction is going to be held at my house – 113, Ashiana Enclave, Rajpura Road, Ludhiana – on 5 October 20….. at 4.00 p.m. Interested bidders may come on the given date, time and place.

Bhanu Pratap

20. Your school has some old furniture and almirahs lying in the stores. Draft an auction notice for their disposal.
Answer:

Prabh Dayaal Public School, Hoshiarpur
Auction Notice

16 July 20……….

Some old furniture and almirahs are lying in the stores of the school. The school wants to dispose of them. For this purpose, an auction of these items will be held in the school campus on 28 July 20 at 11.00 a.nr. Interested bidders should reach the auction venue on the given date and time.

Rajinder Vaswani
(Property Incharge)

Notice-Writing Tasks For Practice

1. Rahul of X-A wants to start an Art Club in school. He has spoken to a well-known artist, who has agreed to conduct art workshops after school. His Principal has given permission for the workshops and for an Art Exhibition to be held to celebrate the Independence Day of India. Rahul decides to write a notice to get those interested in art to take part in the programme. Write out the notice in not more than 50 words. Give the notice an appealing heading. Put the notice in a box.

2. Write a notice for the school noticeboard, informing students about the school inspection to be held on 2.3.20 by the District Education Officer. The notice should be of maximum 50 words, stressing on punctuality, cleanliness and discipline. Put the notice in a box.

3. A notice for the students’ noticeboard is to be prepared regarding a tree plantation ceremony to be held on 7th July, 20 in the school premises, inviting students to participate in it by contributing at least five plants from each class. The notice should not have more than 50 words. Put the notice in a box.

4. The school has decided to hold a fete in the school playground. The Principal has asked you to write, as the Head Boy / Head Girl of the school, a notice about this fete, inviting the students and the teachers to participate in it. The notice should include all necessary details. Write out the notice in not more than 50 words excluding the heading. Put the notice in a box.

5. You are the Head Girl of St. Clare’s High School. The office-bearers and the Principal have had a meeting on raising funds for the slum-dwellers in the neighbourhood. A certificate and an award will be given for the one who donates most. Write the notice for your school noticeboard, requesting the students to donate generously. The notice should not be in more than 50 words. Put the notice in a box.

6. You are the Student Editor of your school magazine (Gandhi Memorial School, Ludhiana). Write out a notice in not more than 50 words, inviting the names of those who would like to give articles, stories, cartoons, etc. for the school magazine. Put the notice in a box.

7. Ramesh Kaushal of Class XI-A has lost his bus pass somewhere in the school compound. The pass bears his name as well as photograph. He puts up a notice on the school noticeboard, giving all the necessary details. Write the notice, using not more than 50 words. Try to make this notice catch people’s attention. Put the notice in a box.

8. Your school has decided to choreograph a dance drama for the school Annual Day. You are the Cultural Secretary of the school. Write a notice in not more than 50 words for your school noticeboard, inviting talented students on 12th Nov. in the auditorium. They will be selected by the famous dancer, Sarala Nagpal. Put the notice in a box.