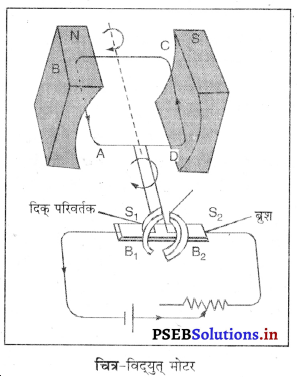
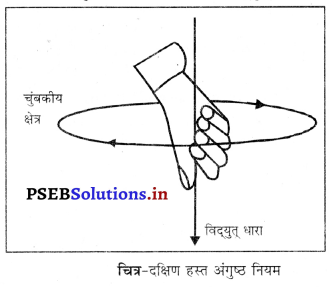
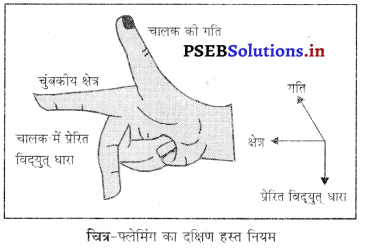
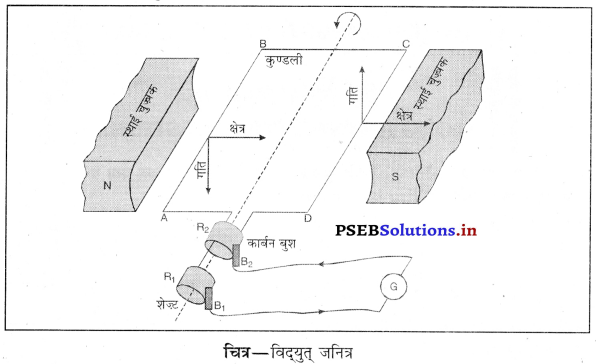
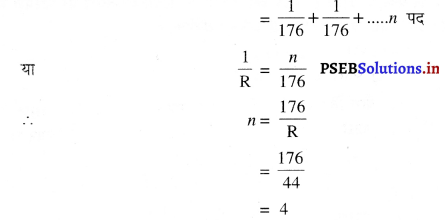Punjab State Board PSEB 10th Class Science Book Solutions Chapter 12 विद्युत Textbook Exercise Questions, and Answers.
PSEB Solutions for Class 10 Science Chapter 12 विद्युत
PSEB 10th Class Science Guide विद्युतTextbook Questions and Answers
प्रश्न 1.
प्रतिरोध R के किसी तार के टुकड़े को पाँच बराबर भागों में काटा गया है। इन टुकड़ों को फिर पार्श्वक्रम में संयोजित कर देते हैं। यदि संयोजन का तुल्य प्रतिरोध R’ है तो R/R’ अनुपात का मान क्या है?
(a) 1/25
(b) 1/5
(c) 5
(d) 25.
हल-प्रत्येक कटे हुए भाग का प्रतिरोध R/5 होगा।
∴ R1 = R2 = R3 = R4 = R5 = \(\frac{\mathrm{R}}{5}\)
∴ पाँच कटे हुए टुकड़ों को पार्यक्रम में संयोजित करने पर
img
∴ R’ = \(\frac{\mathrm{R}}{25}\)
या \(\frac{\mathrm{R}}{\mathrm{R}^{\prime}}\) = 25
उत्तर : (d) 25
प्रश्न 2.
निम्नलिखित में से कौन-सा पद, विद्युत् परिपथ में विद्युत् शक्ति को निरूपित नहीं करता है –
(a) I2R
(b) IR2
(c) VI
(d) V2/R.
हल –
विद्युत् शक्ति P =V × I
= VxI
= (IR)xI
= I2R
= \(\frac{\mathrm{V}^{2}}{\mathrm{R}^{2}}\) x R
= \(\frac{\mathrm{V}^{2}}{\mathrm{R}^{2}} \times h\)
= \(\frac{\mathrm{V}^{2}}{\mathrm{R}}\)
अतः केवल IR2 विद्युत् परिपथ में विद्युत् शक्ति को निरूपित नहीं करता है।
उत्तर-
(b) IR2
प्रश्न 3.
किसी विद्युत् बल्ब का अनुमतांक 220V; 110W है। जब इसे 110V पर प्रचालित करते हैं तब इसके द्वारा उपभुक्त शक्ति कितनी होती है ?
(a) 100W
(b) 75W
(c) 50W
(d) 25W.
हल-सूत्र
P = \(\frac{\mathrm{V}^{2}}{\mathrm{R}}\)
बल्ब का प्रतिरोध R = \(\frac{\mathrm{v}^{2}}{\mathrm{P}}\)
= \(\frac{(220)^{2}}{100}\)
= \(\frac{220 \times 220}{100}\) = 484 Ω
∴ दूसरी दशा में 110V पर प्रचालित करने पर बल्ब द्वारा उपयुक्त शक्ति
P1 = \(\frac{\mathrm{V}_{1}^{2}}{\mathrm{R}}\)
= \(\frac{110 \times 110}{484}\)
= 25W
उत्तर-
(d) 25W.
प्रश्न 4.
दो चालक तार जिनके पदार्थ, लंबाई तथा व्यास समान हैं किसी विद्युत् परिपथ में पहले श्रेणीक्रम में और फिर पार्यक्रम में संयोजित किए जाते हैं। श्रेणीक्रम तथा पार्श्वक्रम संयोजन में उत्पन्न ऊष्माओं का अनुपात क्या होगा?
(a) 1: 2
(b) 2 : 1
(c) 1:4
(d) 4 : 1.
हल–
क्योंकि सभी तार एक ही प्रकार के पदार्थ, लंबाई व व्यास के हैं, इसलिए सभी का प्रतिरोध समान होगा। मान लो यह R है। दोनों को पार्यक्रम में जोड़ने पर प्रतिरोध
Rs = R + R = 2R
दोनों को श्रेणी क्रम में जोड़ने पर प्रतिरोध \(\frac{1}{\mathrm{R}_{p}}=\frac{1}{\mathrm{R}}+\frac{1}{\mathrm{R}}\)
= \(\frac{2}{\mathrm{R}}\)
श्रेणी क्रम में उत्पन्न ऊष्मा H1 = Ps = \(\frac{\mathrm{V}^{2}}{\mathrm{R}_{s}}\)
पार्श्वक्रम में उत्पन्न ऊष्मा H2 = Pp = \(\frac{\mathrm{V}^{2}}{\mathrm{R}_{p}} \)
यदि विभवांतर V है तो ऊष्माओं का अनुपात
\(\frac{\mathrm{H}_{1}}{\mathrm{H}_{2}}=\frac{\mathrm{V}^{2} / \mathrm{R}_{s}}{\mathrm{~V}^{2} / \mathrm{R}_{p}}\)
= \(\frac{\mathrm{R}_{p}}{\mathrm{R}_{s}}=\frac{\mathrm{R} / 2}{2 \mathrm{R}}\)
= \(\frac{1}{4}\)
उत्तर-(C) 1:4
प्रश्न 5.
किसी विद्युत् परिपथ में दो बिंदुओं के बीच विभवांतर मापने के लिए वोल्टमीटर को किस प्रकार संयोजित किया जाता है ?
उत्तर-
दो बिंदुओं के बीच का विभवांतर मापने के लिए वोल्टमीटर को दोनों बिंदुओं के बीच पार्श्वक्रम में संयोजित किया जाता है।
प्रश्न 6.
किसी ताँबे की तार का व्यास 0.5 mm तथा प्रतिरोधकता 1.8 x 10-8 2m है। 10Ω प्रतिरोध का प्रतिरोधक बनाने के लिए कितने लंबे तार की आवश्यकता होगी? यदि इससे दोगुने व्यास का तार लें तो प्रतिरोध में क्या अंतर आएगा?
उत्तर-
दिया है, प्रतिरोधकता (p) = 1.6 x 10-8 Ωm,
प्रतिरोध (R) = 10Ω
व्यास (2r) = 0.5 mm = 5 x 10-4 m
∴ त्रिज्या (r) = 2.5 x 10-4 m
अब तार का अनुप्रस्थ क्षेत्रफल A = πr²
= 3.14 x (2.5 x 10-4)2 m2
= 19.625 x 10-8 m2
∴ सूत्र R = P\(\frac{l}{\mathrm{~A}}\) से,
तार को लंबाई (l) = \(\frac{\mathrm{R} \times \mathrm{A}}{\rho}\)
= \(\frac{10 \Omega \times 19.625 \times 10^{-8} \mathrm{~m}^{2}}{1.6 \times 10^{-8} \Omega \mathrm{m}}\)
= 12.26 × 103m
= 122.6 m उत्तर
व्यास दोगुना करने पर त्रिज्या दोगुनी तथा अनुप्रस्थ क्षेत्रफल (A = πr²) चार गुना हो जाएगा।
∵ R ∝ \(\frac{1}{\mathrm{~A}}\)
∴ क्षेत्रफल चार गुना होने पर प्रतिरोध एक-चौथाई रह जाएगा।
अर्थात् तथा प्रतिरोध R’ = \(\frac{1}{4}\) R
= \(\frac{1}{4}\) × R
= \(\frac{1}{4}\) × 10
= 2.5Ω उत्तर
प्रश्न 7.
किसी प्रतिरोधक के सिरों के बीच विभवांतर V के विभिन्न मानों के लिए उससे प्रवाहित विद्युत् धाराओं I के संगत मान आगे दिए गए हैं
I(ऐंपियर) : 0.5 1.0 2.0 3.0 4.0
v (वोल्ट) : 1.6 3.4 6.7 10.2 13.2
V और I के बीच ग्राफ खींचकर इस प्रतिरोधक का प्रतिरोध ज्ञात कीजिए।
हल- अभीष्ट ग्राफ के लिए देखिए संलग्न चित्र-
प्रतिरोधक का प्रतिरोध = ग्राफ की ढाल
अर्थात् R= \(\frac{\Delta \mathrm{V}}{\Delta \mathrm{I}}\)
दिए गए आँकड़ों से,
V1 = 3.4 V, V2 = 10.2V
तथा संगत विद्युत् धाराएँ I1 = 1.0A, I2 = 3.0A .
ΔV = V2 – V1
= 10.2 – 3.4 = 6.8V
ΔI = I2 – I1
= 3.0 – 1.0
= 2.0A

∴ प्रतिरोध R= \(\frac{\Delta \mathrm{V}}{\Delta \mathrm{I}}\)
= \(\frac{6.8 \mathrm{~V}}{2.0 \mathrm{~A}}\)
R = 3.4Ω उत्तर
प्रश्न 8.
किसी अज्ञात प्रतिरोध के प्रतिरोधक के सिरों से 12V की बैटरी को संयोजित करने पर परिपथ में 2.5 mA विद्युत् धारा प्रवाहित होती है। प्रतिरोधक का प्रतिरोध परिकलित कीजिए।
हल-
दिया है, विभवांतर V= 12V प्रवाहित धारा I = 2.5 mA = 2.5 x 10-3 A
∴ प्रतिरोधक का प्रतिरोध R= \(\frac{\mathrm{V}}{\mathrm{I}}\)
= \(\frac{12 \mathrm{~V}}{2.5 \times 10^{-3} \mathrm{~A}}\)
= 4.8 × 103 Ω
= 4.8 kΩ उत्तर
प्रश्न 9.
9V की किसी बैटरी को 0.2Ω, 0.3Ω, 0.4Ω, 0.5Ω तथा 12Ω के प्रतिरोधकों के साथ श्रेणीक्रम में संयोजित किया गया है। 12Ω के प्रतिरोधक से कितनी विद्युत् धारा प्रवाहित होगी?
हल-
दिया है R1 = 0. 2Ω, R2 = 0.3Ω, R3 = 0.4Ω, R4 = 0.5Ω, तथा R5 = 12Ω
श्रेणी संयोजन का कुल प्रतिरोध R = R1 + R2 + R3 + R4 + R5
= 0.2 + 0.3 + 0.4 + 0.5 + 12
= 13.4Ω
∴ परिपथ में प्रवाहित कुल धारा I = \(\frac{\mathrm{V}}{\mathrm{R}}\)
= \(\frac{9 \mathrm{~V}}{13.4 \Omega}\)
= 0.67A
श्रेणी संयोजन में प्रत्येक प्रतिरोध से 0.67A की धारा प्रवाहित होगी।
श्रेणी क्रम में संयोजित सभी चालकों (प्रतिरोधकों) में से समान विद्युत धारा प्रवाहित होगी, इसलिए 120 के प्रतिरोधक में से प्रवाहित धारा = 0.67A उत्तर
प्रश्न 10.
176Ω प्रतिरोध के कितने प्रतिरोधकों को पार्यक्रम में संयोजित करें कि 220V के विद्युत् स्रोत से संयोजन से 5A विद्युत् धारा प्रवाहित हो?
हल-
दिया है,
V= 220V,
I = 5A
∴ संयोजन का तुल्य प्रतिरोध R = \(\frac{\mathrm{V}}{\mathrm{I}}\)
= \(\frac{220 \mathrm{~V}}{5 \mathrm{~A}}\)
या R = 44Ω
R1 = R2 =……..= Rn = 176Ω
मान लो ऐसे n प्रतिरोधक पार्श्वक्रम में जोड़े गए हैं, तब
∴ \(\frac{1}{\mathrm{R}}=\frac{1}{\mathrm{R}_{1}}+\frac{1}{\mathrm{R}_{2}}+\ldots \ldots+\frac{1}{\mathrm{R}_{n}}\)
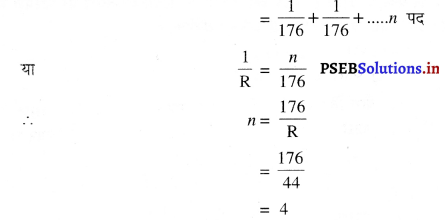
अतः 4 प्रतिरोधक पार्श्वक्रम में जोड़ने होंगे। उत्तर
प्रश्न 11.
यह दर्शाइए कि आप 6Ω प्रतिरोध के तीन प्रतिरोधकों को किस प्रकार संयोजित करेंगे कि प्राप्त संयोजन का प्रतिरोध
(i) 9Ω,
(ii) 4Ω हो।
हल-
(i) 9Ω का प्रतिरोध पाने के लिए, पहले दो प्रतिरोधकों को समांतर क्रम में तथा तीसरे प्रतिरोध को श्रेणी क्रम में जोड़ना होगा।

मान लो पार्श्व संयोजन का प्रतिरोध R है।
∴ \(\frac{1}{R}=\frac{1}{6}+\frac{1}{6}\) = \(\frac{2}{6}\)
∴ R = \(\frac{6}{2}\) = 3Ω होगा।
यह 3Ω का तुल्य प्रतिरोध, 6Ω के तीसरे प्रतिरोध के साथ श्रेणी क्रम में जुड़कर प्रतिरोध 3Ω + 6Ω = 90 का होगा। उत्तर
(ii) 4Ω का प्रतिरोध पाने के लिए पहले 6Ω के दो प्रतिरोधकों को श्रेणीक्रम में जोड़ना होगा तत्पश्चात् तीसरा प्रतिरोधक इनके पार्श्वक्रम में जोड़ना होगा। 6Ω तथा 6Ω के दो प्रतिरोधों के श्रेणी संयोजन का तुल्य प्रतिरोध 6Ω + 6Ω = 12Ω होगा। यह 12Ω का प्रतिरोध 6Ω के साथ पार्श्वक्रम में जोड़ने से 4Ω का प्रतिरोध देगा।
∵ \(\frac{1}{R}=\frac{1}{6}+\frac{1}{12}\)
= \(\frac{2+1}{12}\)
= \(\frac{3}{12}\)

∴ R = \(\frac{12}{3}\)
= 4 Ω उत्तर
प्रश्न 12.
220 V की विद्युत् लाइन पर उपयोग किए जाने वाले बहुत से बल्बों का अनुमतांक 10w है। यदि 220V लाइन से अनुमत अधिकतम विद्युत् धारा 5A है तो इस लाइन के दो तारों के बीच कितने बल्ब पार्श्वक्रम में संयोजित किए जा सकते हैं?
हल-
माना n बल्बों को पार्यक्रम में जोड़ा है। तब परिपथ में कुल शक्ति P= n x एक बल्ब की शक्ति
= n x 10 W
= 10n W
दिया है, V= 220V,
I = 5A
P = V × I से,
10Ω = 220V × 5A
n = 220V× 5A
∴ n = \(\frac{220 \mathrm{~V} \times 5 \mathrm{~A}}{10 \Omega}\)
= 110
अर्थात् 110 बल्बों को पार्श्वक्रम में जोड़ा जा सकता है।
वैकल्पिक विधि
प्रत्येक बल्ब का प्रतिरोध (r) = \(\frac{\mathrm{V}^{2}}{\mathrm{P}}\)
= \(\frac{(220)^{2}}{10}\)
= \(\frac{220 \times 220}{10}\)
= 4840Ω
परिपथ की कुल प्रतिरोधकता (R) = \(\frac{220 \mathrm{~V}}{5 \mathrm{~V}}\) = 44 Ω
मान लो बल्बों की कुल संख्या n है।
तो प्रतिरोधकता (R) = \(\frac{r}{n}\)
⇒ n= \(\frac{r}{n}=\frac{4840}{44}\) =110 उत्तर
प्रश्न 13.
किसी विद्युत् भट्टी की तप्त प्लेट दो प्रतिरोधक कुंडलियों A तथा B की बनी हैं जिनमें प्रत्येक का प्रतिरोध 242 है तथा इन्हें पृथक्-पृथक् श्रेणीक्रम में अथवा पार्श्वक्रम में संयोजित करके उपयोग किया जा सकता है। यदि यह भट्टी 220V विद्युत् स्रोत से संयोजित की जाती है तो तीनों प्रकरणों में प्रवाहित विद्युत् धाराएँ क्या हैं?
हल-
दिया है, V = 220V, कुंडलियों का प्रतिरोध R1 = R2 = 24Ω
प्रथम दशा में, जब किसी एक कुंडली को में प्रयोग किया जाता है तो कुल प्रतिरोध R = R1 = 24Ω
∴ प्रवाहित धारा I = \(\frac{\mathrm{V}}{\mathrm{R}}=\frac{220 \mathrm{~V}}{24 \Omega}\) 9.17 A
दूसरी दशा में, जब दोनों कुंडलियों को श्रेणीक्रम में प्रयोग किया जाता है, तब
कुल प्रतिरोध R= R1 + R2
= 24Ω + 24Ω
श्रेणीकृत कुंडलियों में प्रवाहित धारा I = \(\frac{\mathrm{V}}{\mathrm{R}}=\frac{220 \mathrm{~V}}{48 \Omega}\)
= 4.58 A
तीसरी दशा में, जब कुंडलियों को पार्श्वक्रम में प्रयोग किया जाता है, तब
\(\frac{1}{\mathrm{R}}=\frac{1}{\mathrm{R}_{1}}+\frac{1}{\mathrm{R}_{2}}\)
= \(\frac{1}{24}+\frac{1}{24}\)
= \(\frac{2}{24}\)
∴ R = \(\frac{24}{2}\)
= 12 Ω
प्रवाहित विद्युत् धारा I = \(\frac{\mathrm{V}}{\mathrm{R}}\)
∴ I = \(\frac{220 \mathrm{~V}}{12 \Omega}\)
I = 18.3A उत्तर
प्रश्न 14.
निम्नलिखित परिपथों में प्रत्येक में 20 प्रतिरोधक द्वारा उपयुक्त शक्तियों की तुलना कीजिए :
(i) 6V की बैटरी से संयोजित 12 तथा 22 श्रेणीक्रम संयोजन,
(ii) 4V बैटरी से संयोजित 120 तथा 22 का पार्श्वक्रम संयोजन।
हल-
(i) दिया है, V = 6V
1Ω व 2Ω के श्रेणी-संयोजन का प्रतिरोध
R = 1Ω + 2Ω = 3Ω
∴ परिपथ में प्रवाहित विद्युत् धारा I = \(\frac{\mathrm{V}}{\mathrm{R}}\)
= \(\frac{6 \mathrm{~V}}{3 \Omega}\)
= 2A
श्रेणीक्रम में प्रत्येक प्रतिरोध में से 2A की धारा प्रवाहित होगी।
∴ 2Ω के प्रतिरोधक द्वारा उपयुक्त शक्ति
P1 = I2R
= (2A)2 x 20
= 8w
(ii) ∵ दोनों प्रतिरोध पार्श्वक्रम में जुड़े हैं; इसलिए प्रत्येक प्रतिरोध के सिरों के बीच एक ही विभवांतर, 4V होगा।
∴ 2Ω के प्रतिरोध द्वारा उपयुक्त शक्ति
P2 = \(\frac{\mathrm{V}^{2}}{\mathrm{R}}\)
= \(\frac{(4 \mathrm{~V})^{2}}{2 \Omega}\)
∴ = \(\frac{16}{2}\)
∴ P2 = 8W
अतः दोनों दशाओं में 2Ω प्रतिरोधक में समान शक्ति व्यय होगी। उत्तर
प्रश्न 15.
दो विद्युत् लैंप, जिनमें से एक का अनुमतांक 100W; 220V तथा दूसरे का 60 W; 220V है, विद्युत् मेंस के साथ पार्श्वक्रम में संयोजित हैं। यदि विद्युत् आपूर्ति की वोल्टता 220V है तो मेंस से कितनी धारा ली जाती है?
हल-
प्रथम लैंप के लिए, विभवांतर V1 = 220V
लैंप की शक्ति P1 = 100W माना इसका प्रतिरोध R1 है तो
P= \(\frac{\mathrm{V}^{2}}{\mathrm{R}}\) से,
प्रथम लैंप का प्रतिरोध, R1 = \(\frac{v_{1}^{2}}{P_{1}}\) = \(\frac{(220 \mathrm{~V})^{2}}{100 \mathrm{~W}}\)
= 484Ω
दूसरे लैंप के लिए, विभवांतर V2 = 220 V,
लैंप की शक्ति P2 = 60W
∴ दूसरे लैंप का प्रतिरोध R2 = \(\frac{\mathrm{V}_{2}^{2}}{\mathrm{P}_{2}}\)
= \(\frac{(220 \mathrm{~V})^{2}}{60 \mathrm{~W}}\)
= \(\frac{2420}{3}\) Ω
दोनों लैंप को पार्यक्रम में जोड़ने पर संयोजन का प्रतिरोध R हो तो

∵ लाइन वोल्टेज V = 220V
∴ लाइन से ली जाने वाली धारा I = \(\frac{\mathrm{V}}{\mathrm{R}}\)
= \(\frac{220 \mathrm{~V}}{302.5 \Omega}\)
= 0.73 A उत्तर
प्रश्न 16.
किसमें अधिक विद्युत् ऊर्जा उपभुक्त होती है : 250w का टी० वी० सेट जो एक घंटे तक चलाया जाता है अथवा 120W का विद्युत् हीटर जो 10 मिनट के लिए चलाया जाता है ?
हल-
T.V. सेट के लिए, P1 = 250w
= 250 Js-1
t1 = 1h = 60 x 60s
∴ T.V. सेट द्वारा उपभुक्त विद्युत् ऊर्जा = P1 × t1,
= 250 Js-1 x (60 x 60s)
= 900000J
= 9x 105 J …………………….(i)
विद्युत् हीटर के लिए,
P2 = 120W
= 120 Js-1 तथा
t2 = 10 min.
= 10 x 60s
∴ विद्युत् हीटर द्वारा उपभुक्त विद्युत् ऊर्जा = P2 x t2
= 120 Js-1 x (10 x 60s)
= 72000J = 7.2 x 104J ………………………(ii)
समीकरण (i) तथा (ii) से स्पष्ट है कि T.V. सेट द्वारा उपभुक्त ऊर्जा अधिक है।
प्रश्न 17.
82 प्रतिरोध का कोई विद्युत् हीटर विद्युत् मेंस से 2 घंटे तक 15A विद्युत् धारा लेता है। हीटर में उत्पन्न ऊष्मा की दर परिकलित कीजिए।
हल-
दिया है, R = 8Ω,
I = 15A विद्युत् हीटर में ऊष्मा उत्पन्न होने की दर अर्थात् विद्युत् हीटर की शक्ति P = I2R
= (15A)2 x 8Ω
= 225×8
= 1800 W
प्रश्न 18.
निम्नलिखित को स्पष्ट कीजिए-
(a) विद्युत् लैम्पों के तंतुओं के निर्माण में प्रायः एकमात्र टंगस्टन का ही उपयोग क्यों किया जाता है?
(b) विद्युत् तापन युक्तियों जैसे ब्रेड-टोस्टर तथा विद्युत् इस्तरी के चालक शुद्ध धातुओं के स्थान पर मिश्रधातुओं के क्यों बनाए जाते हैं ?
(c) घरेलू विद्युत् परिपथों में श्रेणीक्रम संयोजन का उपयोग क्यों नहीं किया जाता है।
(d) किसी तार का प्रतिरोध उसकी अनुप्रस्थ काट के क्षेत्रफल में परिवर्तन के साथ किस प्रकार परिवर्तित होता है?
(e) विद्युत् संचारण के लिए प्राय: कॉपर तथा ऐलुमिनियम के तारों का उपयोग क्यों किया जाता है ?
उत्तर-
(a) क्योंकि टंगस्टन की उच्च प्रतिरोधकता (5.2 x 10-8 ओम-मीटर) है तथा टंगस्टन का गलनांक भी अन्य धातुओं की तुलना में बहुत ऊंचा (3400°C) होता है, इसलिए विद्युत् लैंपों के तंतुओं के निर्माण में प्रायः एकमात्र टंगस्टन ही उपयोग किया जाता है।
(b) मिश्रधातुओं की प्रतिरोधकता, शुद्ध धातुओं की तुलना से अधिक होती है तथा ताप वृद्धि के साथ इनकी प्रतिरोधकता से नगण्य परिवर्तन होता है। इसके अतिरिक्त मिश्रधातुओं का ऑक्सीकरण भी कम होता है जिसके फलस्वरूप मिश्रधातुओं में बने चालकों की आयु शुद्ध धात्विक चालकों की तुलना में अधिक होती है। इसलिए ब्रेड-टोस्टर, विद्युत् इस्तरी आदि के चालक मिश्रधातुओं के बनाए जाते हैं।
(c) श्रेणीक्रम संयोजन में जैसे-जैसे नये प्रतिरोधक जुड़ते जाते हैं, परिपथ का कुल प्रतिरोध बढ़ता जाता है और अलग-अलग प्रतिरोधकों के सिरों के बीच उपलब्ध विभवांतर घटता जाता है। अतः परिपथ में धारा भी कम हो जाती है। यदि घरों में प्रकाश करने के लिए श्रेणीबद्ध व्यवस्था प्रयोग की जाए तो परिपथ में जितने अधिक लैंप जुड़े होंगे, उनका प्रकाश उतना ही कम हो जाएगा। इसके अतिरिक्त श्रेणीबद्ध व्यवस्था प्रयोग करने पर स्विच ऑन करने पर सभी लैम्प एक साथ प्रदीप्त होंगे तथा स्विच ऑफ करने पर सभी लैंप एक साथ बुझ जाएँगे, जबकि पार्श्वक्रम व्यवस्था करने पर इच्छानुसार स्वतंत्र रूप से किसी भी लैंप को प्रकाशित या अप्रकाशित किया जा सकता है।
(d) तार का प्रतिरोध उसकी अनुप्रस्थ काट के क्षेत्रफल के व्युत्क्रमानुपाती होता है। अर्थात् मोटी तार का अनुप्रस्थ काट क्षेत्रफल अधिक होगा और उसका प्रतिरोध कम होगा। \(\left(R \propto \frac{1}{A}\right)\)
(e) विद्युत् के सर्वश्रेष्ठ चालकों में प्रथम स्थान पर चाँदी, दूसरे पर ताँबा तथा तीसरे स्थान पर एल्यूमिनियम है। चाँदी एक कीमती धातु है तथा अपेक्षाकृत कम मात्रा में उपलब्ध है। इसलिए विद्युत् संचारण व्यवस्था में चाँदी के स्थान पर सस्ती तथा पर्याप्त मात्रा में उपलब्ध धातुएं ताँबा तथा एल्यूमिनियम प्रयोग की जाती है।
Science Guide for Class 10 PSEB विद्युत InText Questions and Answers
प्रश्न 1.
विद्युत् परिपथ का क्या अर्थ है ?
उत्तर-
विद्युत् परिपथ- विद्युत् स्रोत से विभिन्न घटकों में से होकर विद्युत् धारा बहने के सतत् तथा बंद पथ को विद्युत् परिपथ कहा जाता है। विद्युत्धारा के घटक-विद्युत्धारा के निम्नलिखित प्रमुख घटक हैं-
- विद्युत् स्रोत (बैटरी अथवा सेल)
- चालक
- स्विच (कुंजी)
- कोई अन्य उपकरण जो परिपथ में जोडा गया हो।
प्रश्न 2.
विद्युत्धारा के मात्रक की परिभाषा लिखिए।
अथवा
विद्युत्धारा की इकाई का नाम लिखें। इसकी परिभाषा भी लिखें।
उत्तर-
विद्युत्धारा का S.I. मात्रक ‘ऐंपियर’ है जिसे ‘A’ से व्यक्त किया जाता है।
ऐंपियर-जब किसी चालक में 1 सेकंड में 1 कूलॉम आवेश का प्रवाह होता है तो प्रयुक्त विद्युत्धारा की मात्रा को 1 ऐंपियर कहा जाता है।
∴ 1A = \(\frac{1 \mathrm{C}}{1 \mathrm{~s}}\)
प्रश्न 3.
एक कूलॉम आवेश की रचना करने वाले इलेक्ट्रानों की संख्या परिकलित कीजिए।
उत्तर-
हम जानते हैं कि 1 इलेक्ट्रॉन पर आवेश = 1.6 x 10-19C
माना 1 कूलॉम की रचना करने वाले इलेक्ट्रॉनों की संख्या = n
∴ n x 1.6 x 10-19C = 1C
या n = \(\frac{1}{1.6 \times 10^{-19}}\)
= \(\frac{1 \times 10^{19}}{1.6}\)
= \(\frac{10}{16} \times 10^{9}\)
= 0.625 x 1019
. = 6.25 x 1018
अतः 1 कूलॉम (c) = 6.25 x 1018 इलेक्ट्रॉन उत्तर
प्रश्न 4.
उस युक्ति का नाम लिखिए जो किसी चालक के सिरों पर विभवांतर बनाए रखने में सहायता करती है।
उत्तर-
सेल एक ऐसी युक्ति है जो किसी चालक के सिरों पर विभवांतर बनाए रखने में सहायता करती है।
प्रश्न 5.
यह कहने का क्या तात्पर्य है कि दो बिंदुओं के बीच विभवांतर 17 है?
उत्तर-
दो बिंदुओं के बीच विभवांतर 1V से तात्पर्य है कि दो बिंदुओं के बीच 1 कूलॉम आवेश ले जाने में 1 जूल कार्य होता है।
प्रश्न 6.
6V बैटरी से गुजरने वाले हर एक कूलॉम आवेश को कितनी ऊर्जा दी जाती है?
हल : यहाँ
विभवांतर (V) = 6V
आवेश की मात्रा (Q) = 1 कूलॉम
दी गई ऊर्जा (किया गया कार्य) (W) = ?
हम जानते हैं, विभवांतर = 
V= \(\frac{W}{Q}\)
∴ दी गई ऊर्जा W = V × Q
= 6V × 1C
= 6 जूल (J) उत्तर
प्रश्न 7.
किसी चालक का प्रतिरोध किन कारकों पर निर्भर करता है?
उत्तर-
चालक के प्रतिरोध की निर्भरता-किसी चालक का प्रतिरोध निम्नलिखित कारकों पर निर्भर करता है –
(i) चालक की लंबाई (l)-किसी चालक का प्रतिरोध चालक की लंबाई (l) के अनुक्रमानुपाती होता है।
अर्थात् R ∝ l
(ii) चालक की अनुप्रस्थ काट का क्षेत्रफल (A)-किसी चालक का प्रतिरोध उसकी अनुप्रस्थ काट (A) के क्षेत्रफल के व्युत्क्रमानुपाती होता है।
अर्थात्
R ∝ \(\frac{1}{\mathrm{~A}}\)
(iii) चालक के पदार्थ की प्रकृति-किसी चालक का प्रतिरोध उस चालक के पदार्थ की प्रकृति पर निर्भर करता है।
R ∝ \(\frac{l}{\mathrm{~A}}\)
अथवा R = ρ × \(\frac{l}{\mathrm{~A}}\)
जहाँ ρ अनुपातिकता स्थिराँक है।
प्रश्न 8.
समान पदार्थ के दो तारों में यदि एक पतला तथा दूसरा मोटा हो तो इनमें से किसमें विद्युत् धारा आसानी से प्रवाहित होगी जबकि उन्हें समान विद्युत् स्रोत से संयोजित किया जाता है ? क्यों?
उत्तर-
हम जानते हैं कि चालक तार का प्रतिरोध तार के अनुप्रस्थ काट (Area of Cross-Section) के व्युत्क्रमानुपाती होता है। अर्थात् मोटी तार का प्रतिरोध कम और पतली तार का प्रतिरोध अधिक होगा।
अब R ∝ \(\frac{1}{A}\)
इसलिए मोटी तार का प्रतिरोध पतली तार की अपेक्षा कम होने के कारण उसमें से विद्युत्धारा का प्रवाह अधिक तथा सुगमता से होता है।
प्रश्न 9.
मान लीजिए किसी वैद्युत अवयव के दो सिरों के बीच विभवांतर को उसके पूर्व के विभवांतर की तुलना में घटाकर आधा कर देने पर भी उसका प्रतिरोध नियत रहता है। तब उस अवयव से प्रवाहित होने वाली विद्युत्धारा में क्या परिवर्तन होगा?
उत्तर-
मान लो पूर्व अवस्था में विद्युत् अवयव के दो सिरों के बीच विभवांतर V, प्रतिरोध R तथा प्रवाहित होने वाली विद्युत्धारा I है, तो ओम नियम के अनुसार, I = \( \frac{\mathrm{V}}{\mathrm{R}}\) …………………………….(1)
यदि वैद्युत् अवयव के दोनों सिरों के बीच विभवांतर पूर्व अवस्था का आधा करें (अर्थात \(\frac{\mathrm{V}}{2}\) करें) जबकि प्रतिरोध नियत रहे तो विद्युत्धारा की मात्रा बदलकर I’ हो जायेगी।
∴ I’ = \(\frac{\frac{\mathrm{V}}{2}}{\mathrm{R}}\)
⇒ I’ = \(\frac{V}{2 R}\) …………………………….(2)
(2) को (1) से भाग देने पर

∴ I’ =\(\frac{1}{2}\) x I अर्थात् विद्युत्धारा पूर्व अवस्था की तुलना में आधी रह जायेगी।
प्रश्न 10.
विद्युत् टोस्टरों तथा विद्युत् इस्तरियों के तापन अवयव शुद्ध धातु के न बनाकर किसी मिश्रधातु के क्यों बनाए जाते हैं ?
उत्तर-
विद्युत् टोस्टरों तथा विद्युत् इस्तरियों के तापन अवयव शुद्ध धातु के न बनाकर किसी मिश्रधातु के बनाए जाते हैं। इसके निम्नलिखित कारण हैं:
- मिश्र धातुओं की प्रतिरोधकता अवयवी धातुओं की अपेक्षा अधिक होती है।
- मिश्र धातुओं का उच्चताप पर शीघ्र ही दहन (उपचयन) नहीं होता है।
- मिश्रधातुओं का गलनाँक अधिक होता है।
प्रश्न 11.
निम्नलिखित प्रश्नों के उत्तर पाठ्य-पुस्तक की तालिका 12.2 में दिए गए आँकड़ों के आधार पर दीजिए:
(a) आयरन (Fe) तथा मर्करी (Hg) में कौन अच्छा विद्युत् चालक है?
(b) कौन-सा पदार्थ सर्वश्रेष्ठ चालक है।
उत्तर-
(a) आयरन (Fe) मर्करी (Hg) की अपेक्षा अच्छा चालक है क्योंकि आयरन की प्रतिरोधकता मर्करी की अपेक्षा अधिक होती है।
(b) चाँदी सर्वश्रेष्ठ चालक है क्योंकि इसका प्रतिरोध (1.60 x 10-8Ωm) न्यूनतम है।
प्रश्न 12.
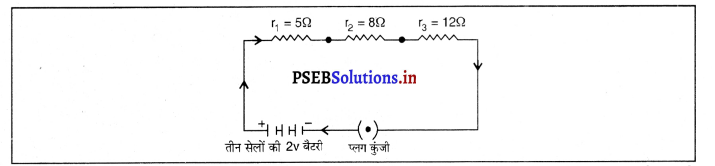
किसी विद्युत् परिपथ का व्यवस्था आरेख खींचिए जिसमें 27 के तीन सेलों की बैटरी, एक 5Ω प्रतिरोधक, एक 8Ω प्रतिरोधक, एक 12Ω प्रतिरोधक तथा एक प्लग कुंजी सभी श्रेणीक्रम में संयोजित हों।
उत्तर-
विद्युत् परिपथ व्यवस्था आरेख
प्रश्न 13.
प्रश्न 1 का परिपथ चित्र दोबारा खींचिए तथा इसमें प्रतिरोधकों से प्रवाहित विद्युत्धारा को मापने के लिए ऐमीटर तथा 12Ω के प्रतिरोधक के सिरों के बीच विभवांतर मापने के लिए वोल्टमीटर लगाइए। ऐमीटर तथा वोल्टमीटर के क्या पाठ्याँक होंगे?
हल-
यहाँ r1 = 5Ω
r2 = 8Ω
r3 = 12 Ω
परिपथ का कुल प्रतिरोध (R) = ?
हम जानते हैं प्रतिरोधकों को श्रेणी क्रम में संयोजित करने पर परिपथ का कुल प्रतिरोध R = r1 + r2+ r3
R = 5Ω + 8Ω + 120 Ω
R = 25Ω.
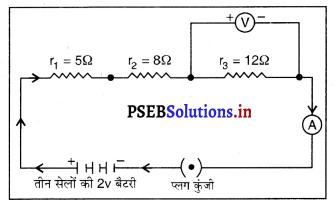
बैटरी के टर्मीनलों के बीच कुल विभवांतर A = 2 V
अब, ओम के नियम अनुसार I = \(\frac{\mathrm{V}}{\mathrm{R}}\)
I = \( \frac{2 \mathrm{~V}}{25 \Omega}\)
I = 0.08A
12Ω के प्रतिरोधक के सिरों के बीच विभवांतर = I x r3
= 0.08 x 12
= 0.96 वोल्ट (V)
∴ ऐमीटर तथा वोल्टमीटर के पाठ्याँक क्रमश: 0.08A तथा 0.96V होंगे।
प्रश्न 14.
जब (a) 12 तथा 106Ω
(b) 1Ω तथा 103Ω तथा 106Ω के प्रतिरोध पार्श्वक्रम में संयोजित किए जाते हैं तो इनके तुल्य प्रतिरोध के संबंध में आप क्या निर्णय करेंगे?
हल-
(a) दिया है r1 = 1Ω
तथा r2 = 106Ω
माना R तुल्य प्रतिरोध है, तो पार्श्वक्रम में संयोजित करने पर \(\frac{1}{\mathrm{R}}=\frac{1}{r_{1}}=\frac{1}{r_{2}}\)
= \(\frac{1}{1}+\frac{1}{10^{6}}\)
= \(\frac{10^{6}+1}{10^{6}}\)
= \(\frac{1000000+1}{1000000}\)
= \(\frac{1000001}{1000000}\)
R = \( \frac{1000000}{1000001}\) = 0.9Ω (लगभग) उत्तर
(b) यहाँ
r1 = 1Ω
r2 = 103 Ω
r = 106 Ω
माना R तुल्य प्रतिरोध है तो पार्यक्रम में संयोजित किए जाने पर

∴ R = \(\frac{10^{6}}{10^{6}+10^{3}+1}\)
= \(\frac{1000000}{1001001} \)
= 0.9Ω (लगभग) उत्तर
प्रश्न 15.
100Ω का एक विद्युत् लैंप, 50Ω का एक विद्युत् टोस्टर तथा 5002 का एक जल फिल्टर 200V के विद्युत् स्रोत से पार्श्वक्रम में संयोजित है। उस विद्युत् इस्तरी का प्रतिरोध क्या है जिसे यदि समान स्त्रोत के साथ संयोजित कर दें तो वह उतनी ही विद्युत् धारा लेती है जितनी तीनों युक्तियाँ लेती हैं। यह भी ज्ञात कीजिए कि इस विद्युत् इस्तरी से कितनी विद्युत् धारा प्रवाहित होती है ?
हल-
100Ω का विद्युत् लैंप, 502 का विद्युत् टोस्टर तथा 500Ω का फिल्टर पार्श्वक्रम में संयोजित किया गया है और R इनका तुल्य प्रतिरोध है तो ओम नियमानुसार
\(\frac{1}{R}=\frac{1}{100}+\frac{1}{50}+\frac{1}{500}\)
= \(\frac{5+10+1}{500}\)
= \(\frac{16}{500}\)
∴ R = \(\frac{500}{16}\) = 31.25 Ω
अतः विद्युत् इस्तरी का तुल्य प्रतिरोध = R = 31.250Ω
विभवांतर V = 220V विद्युत्धारा की मात्रा
(I) = ?
हम जानते हैं I = \(\frac{\mathrm{V}}{\mathrm{R}}\)

∴ I = 7.04A उत्तर
प्रश्न 16.
श्रेणीक्रम में संयोजित करने के स्थान पर वैद्युत् युक्तियों को पार्यक्रम में संयोजित करने के क्या लाभ हैं?
उत्तर-
वैद्युत् युक्तियों को श्रेणीक्रम की अपेक्षा पार्श्वक्रम में संयोजित करने के लाभ-
1. किसी श्रेणी बद्ध विद्युत् परिपथ में शुरू से अंत तक विद्युत् धारा नियत रहती है जोकि व्यावहारिक नहीं है। यदि हम किसी विद्युत् परिपथ में विद्युत् बल्ब तथा विद्युत् हीटर को श्रेणीक्रम में संयोजित करें तो यह उचित प्रकार से कार्य नहीं कर पायेंगे क्योंकि इन्हें भिन्न मानों की विद्युत् धाराओं की आवश्यकता होगी। इसके विपरीत पार्श्वक्रम परिपथ में विद्युत् धारा विभिन्न वैद्युत् युक्तियों में विभाजित हो जाती है।
2. श्रेणीबद्ध परिपथ की यदि एक विद्युत् युक्ति कार्य करना बंद कर देती है तो परिपथ टूट जाता है तथा अन्य युक्तियाँ कार्य करना बंद कर देती हैं। इसके विपरीत पार्यक्रम परिपथ में विद्युत् धारा विभिन्न विद्युत् युक्तियों में विभाजित होने पर अन्य युक्तियाँ कार्य करती रहती हैं।
3. प्रतिरोधों को पार्श्वक्रम में जोड़ने से किसी भी चालक में स्विच की सहायता से विद्युत्धारा स्वतंत्रतापूर्वक प्रवाहित या रोकी जा सकती है जिससे विद्युत् यक्तियों को स्वतंत्रतापूर्वक प्रयोग में लाया जा सकता है।
प्रश्न 17.
2Ω, 3Ω तथा 6Ω के तीन प्रतिरोधकों को किस प्रकार संयोजित करेंगे कि संयोजन का कुल प्रतिरोध
(a) 4Ω
(b) 1Ω हो?
हल-
(a) कुल प्रतिरोध 4Ω प्राप्त करने के लिए-ऐसा करने के लिए 3Ω तथा 6Ω के प्रतिरोधकों को समानांतर क्रम करके 2Ω के प्रतिरोधक के साथ श्रेणीक्रम में संयोजित किया जाता है।
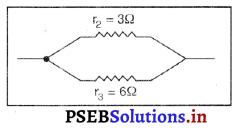
मान लो r2 = 3Ω और r3 = 6Ω को समानांतर क्रम में संयोजित करने पर कुल प्रतिरोध । है, तो
\(\frac{1}{r}=\frac{1}{r_{2}}+\frac{1}{r_{3}}\)
= \(\frac{1}{3}+\frac{1}{6}\)
या \(\frac{1}{r}=\frac{2+1}{6}\)
\( \frac{1}{r}=\frac{3}{6}\)
\(\frac{1}{r}=\frac{1}{2}\)
∴ r = 2Ω
अब r1 = 2Ω, तथा r = 2Ω को श्रेणी क्रम में संयोजित करने पर मान लो कुल प्रतिरोध है, तो R = r1 +r
= 2Ω + 2Ω = 4Ω.
(b) कुल प्रतिरोध 1Ω प्राप्त करने के लिए संयोजन-1Ω का कुल प्रतिरोध प्राप्त करने के लिए r1 = 2Ω, r2 = 3Ω तथा r3 = 6Ωको समानांतर क्रम में जोड़ना होगा।
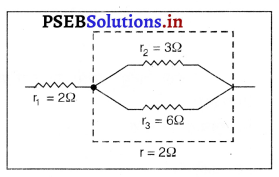
मान लो कुल प्रतिरोध R है तो समानांतर क्रम के सूत्र से,
\(\frac{1}{R}=\frac{1}{r_{1}}+\frac{1}{r_{2}}+\frac{1}{r_{3}}\)
= \(\frac{1}{2}+\frac{1}{3}+\frac{1}{6}\)
= \(\frac{3+2+1}{6}\)
= \(\frac{6}{6}\)
R = 1Ω
प्रश्न 18.
4Ω, 8Ω, 12Ω तथा 24Ω प्रतिरोध की चार कुंडलियों को किस प्रकार संयोजित करें कि संयोजन से
(a) अधिकतम
(b) निम्नतम प्रतिरोध प्राप्त हो सके ?
हल-
(a) अधिकतम प्रतिरोध प्राप्त करने हेतु संयोजन-यदि इन चारों प्रतिरोधों को श्रेणीक्रम में रखा जाए तो अधिकतम प्रतिरोध प्राप्त होगा।
Rs = r1+r2+r3+r4
= 4Ω + 8Ω + 12Ω + 24Ω
= 480 उत्तर
(b) न्यूनतम प्रतिरोध प्राप्त करने हेतु–यदि दिए गए चारों प्रतिरोधों को पार्यक्रम में जोड़ा जाए तो न्यूनतम प्रतिरोध प्राप्त होगा।
∴ \(\frac{1}{\mathrm{R}_{p}}=\frac{1}{r_{1}}+\frac{1}{r_{2}}+\frac{1}{r_{3}}+\frac{1}{r_{4}}\)
= \(\frac{1}{4}+\frac{1}{8}+\frac{1}{12}+\frac{1}{24}\)
= \(\frac{6+3+2+1}{24}\)
= \(\frac{12}{24}\)
\(\frac{1}{\mathrm{R}_{p}}=\frac{1}{2}\)
∴RP = 2Ω. उत्तर
प्रश्न 19.
किसी विद्युत् हीटर की डोरी क्यों उत्तप्त नहीं होती जबकि उसका तापन अवयव उत्तप्त हो जाता है?
उत्तर-
हम जानते हैं
H = I2Rt
⇒ H ∝ R
तापन अवयव का उच्च प्रतिरोध होता है जिस कारण अधिक विद्युत् ऊर्जा, ताप ऊर्जा में परिवर्तित होती है जिससे तापन अवयव उत्तप्त हो जाता है। दूसरी तरफ विद्युत् हीटर की डोरी का प्रतिरोध बहुत कम होता है जिससे वह उत्तप्त नहीं होती है।
प्रश्न 20.
एक घंटे में 50V विभवांतर से 96000 कूलॉम आवेश को स्थानांतरित करने में उत्पन्न ऊष्मा परिकलित कीजिए?
हल-
दिया है, स्थानांतरित आवेश Q = 96000 कूलॉम, 1 = 1 घंटा समय = 60 x 60 सेकंड,
विभवांतर V = 50 वोल्ट
⇒ उत्पन्न उष्मा ऊर्जा, H = Q x V
= 96000 x 50
= 48250000
= 4.825 x 10 जूल
∴ H = 4.825 x 103 किलो जूल उत्तर
प्रश्न 21.
20Ω प्रतिरोध की कोई विद्युत् इस्तरी 5A विद्युत् धारा लेती है। 30s में उत्पन्न उष्मा परिकलित कीजिए।
हल –
दिया है
प्रतिरोध (R) = 20Ω
विद्युत्धारा (I) = 5A
समय (1) = 30s
उत्पन्न उष्मा (H) = ?
हम जानते हैं, उत्पन्न हुई उष्मा ऊर्जा (H) = I2Rt
= (5)2 x 20 x 30 J
= 25 x 20 x 30 J
= 15000 J (जूल)
= 1.5 x 104J उत्तर
प्रश्न 22.
विद्युत् धारा द्वारा प्रदत्त ऊर्जा की दर का निर्धारण कैसे किया जाता है ?
उत्तर-
विद्युत् धारा द्वारा प्रदत्त ऊर्जा की दर का निर्धारण विद्युत् शक्ति द्वारा किया जाता है।
प्रश्न 23.
कोई विद्युत् मोटर 220V के विद्युत् स्रोत से 5.0A विद्युत् धारा लेता है। मोटर की शक्ति निर्धारित कीजिए तथा 2 घंटे में मोटर द्वारा उपभुक्त ऊर्जा परिकलित कीजिए।
हल–
दिया है, विद्युत् धारा (I) = 5.0A
विद्युत् विभवांतर (V) = 220V
समय (t) = 2 घंटे ज्ञात करना है,
मोटर की शक्ति (P) = ?
1 घंटे में उपभुक्त ऊर्जा (E) = ?
हम जानते हैं, शक्ति (P) = Vx I
= 220×5
= 1100 W (वाट) उत्तर
मोटर द्वारा 2 घंटे में उपभुक्त ऊर्जा (E) = Pxt
= 1100 वाट x 2 घंटे
= 2200 वाट-घंटे (Wh)
= 2.2 किलोवाट-घंटा (KWh) उत्तर