Bhagya

Punjab State Board PSEB 6th Class Maths Book Solutions Chapter 8 Basic Geometrical Concepts MCQ Questions with Answers.

PSEB 6th Class Maths Chapter 8 Basic Geometrical Concepts MCQ Questions

Multiple Choice Questions

Question 1.
How many lines can pass through a point?
(a) 1
(b) 2
(c) 4
(d) Infinite.
Answer:
(d) Infinite.

Question 2.
The number of points lie on a line are
(a) 2
(b) 4
(c) 1
(d) Infinite.
Answer:
(d) Infinite.

Question 3.
The number of lines passes through two points are ……………… .
(a) 1
(b) 2
(c) 3
(d) Infinite.
Answer:
(a) 1

Question 4.
In how many parts, a closed curve divides the plane?
(a) 1
(b) 2
(c) 3
(d) 4.
Answer:
(c) 3

Question 5.
A quadrilateral has……………. diagonals.
(a) 1
(b) 2
(c) 3
(d) 4.
Answer:
(b) 2

Question 6.
Which of the following is not a polygon?
(a) Triangle
(b) Pentagon
(c) Circle
(d) Quadrilateral.
Answer:
(c) Circle

Question 7.
A triangle has…………… parts.
(a) 3
(b) 6
(c) 9
(d) 2.
Answer:
(b) 6

Question 8.
Which of file following is not a quadrilateral?

Answer:

Question 9.
A line segment joining the opposite vertices of a quadrilateral is called its …………. .
(a) Diagonal
(b) Side
(c) Angle
(d) Region.
Answer:
(a) Diagonal

Question 10.
The radius of a circle is 4 cm then the diameter is …………….. .
(a) 8 cm
(b) 2 cm
(c) 6 cm
(d) 12 cm.
Answer:
(a) 8 cm

Question 11.
The diameter of a circle is 12 cm then the radius is …………….. .
(a) 24 cm
(b) 6 cm
(c) 18 cm
(d) 4 cm.
Answer:
(b) 6 cm

Question 12.
The longest chord of a circle is…………….
(a) Arc
(b) Perimeter
(c) Diameter
(d) Radius.
Answer:
(c) Diameter

Question 13.
Which of the following figure is not a polygon?

Answer:

Question 14.
Which of the following figure is a polygon?

Answer:

Question 15.
Which of the following is a closed curve?

Answer:

Question 16.
Which of the following is an open curve?

Answer:

Question 17.
Two different line when intersect each other at some point, they are called:
(a) Intersecting lines
(b) Parallel lines
(c) Concurrent lines
(d) None of these.
Answer:
(a) Intersecting lines

Fill in the blanks:

Question (i)
In the environment, a railway track is an example of ……………. .
Answer:
parallel lines

Question (ii)
In the environment, a nail fixed in the wall is an example of ……………….. .
Answer:
a point

Question (iii)
The intersection of the three walls of a room, is an example of …………… .
Answer:
concurrent lines

Question (iv)
……………….. is the longest chord of a circle.
Answer:
Diameter

Question (v)
A triangle has ……………… part.
Answer:
six

Write True/False:

Question (i)
Three lines can pass through a point. (True/False)
Answer:
False

Question (ii)
The number of lines passing through two points is one. (True/False)
Answer:
True

Question (iii)
Every circle has a centre. (True/False)
Answer:
True

Question (iv)
The diameter is twice the radius. (True/False)
Answer:
True

Question (v)
Every chord of a circle is also a diameter. (True/False)
Answer:
False

Punjab State Board PSEB 7th Class Maths Book Solutions Chapter 14 Symmetry Ex 14.1 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 7 Maths Chapter 14 Symmetry Ex 14.1

1. Which of the following figures are asymmetrical ?


Solution:
Figure (a) and (c) are asymmetrical.

2. Draw the lines of symmetry in the following figures.


Solution:

3. Draw all lines of symmetry if any in each of the following figures.


Solution:

4. Copy the figures with punched holes and find the axes of symmetry for the following.

Solution:

5. In the following figures mark the missing holes in order to make them symmetrical about the dotted line.

Solution:
The missing holes are marked by dark punches (small circles) in each of following figures

6. In each of the following figures, the mirror line (i.e. the line of symmetry) is given as dotted line complete each figure performing reflection in the dotted (mirror) line. (You might perhaps place a mirror along the dotted line and look into the mirror for the image). Are you able to recall the name of figure you complete.

Solution:

7. Draw the reflection of the following letter in the given mirror line.

Solution:

8. Copy each diagram on a squared paper and complete each shape to be symmetrical about the mirror lines shown dotted.

Solution:

9. State the number of lines of symmetry for the following figures :

(a) A scalene triangle
(b) A rectangle
(c) A rhombus
(d) A parallelogram
(e) A regular hexagon
(f) A circle
Answer:
(a) 0
(b) 2
(c) 2
(d) 0
(e) 6
(f) Infinite.

10. Multiple Choice Questions :

Question (i).
Which of the following triangles have no line of symmetry ?
(a) An equilateral triangle
(b) An Isosceles triangle
(c) A scalene triangle
(d) All of above
Answer:
(a) An equilateral triangle

Question (ii).
What is the other name for a line of symmetry of a circle ?
(a) An arc
(b) A sector
(c) A diameter
(d) A radius
Answer:
(c) A diameter

Question (iii).
How many lines of symmetry does a regular polygon have ?
(a) Infinitely many
(b) As many as its sides
(c) One
(d) Zero
Answer:
(b) As many as its sides

Question (iv).
In the given figure, the dotted line is the line of symmetry which figure is formed if the given figure is reflected in the dotted line fig.

(a) Square
(b) Rhombus
(c) Triangle
(d) Pentagon
Answer:
(b) Rhombus

Question (v).
What is other name for a line of symmetry of an Isosceles triangle ?
(a) Side
(b) Median
(c) Radius
(d) Angle
Answer:
(b) Median

Question (vi).
Which of the following alphabets has a vertical line of symmetry ?
(a) M
(b) Q
(c) E
(d) B
Answer:
(a) M

Question (vii).
Which of the following alphabets has a horizontal line of symmetry ?
(a) C
(b) D
(c) K
(d) All the above
Answer:
(d) All the above

Question (viii).
Which of the following alphabets has no line of symmetry ?
(a) A
(b) B
(c) P
(d) O
Answer:
(c) P

Punjab State Board PSEB 7th Class Maths Book Solutions Chapter 13 Exponents and Powers MCQ Questions with Answers.

PSEB 7th Class Maths Chapter 13 Exponents and Powers MCQ Questions

Multiple Choice Questions :

Question 1.
The value of (-1)¹⁰¹
(a) 1
(b) -1
(c) 101
(d) -101
Answer:
(b) -1

Question 2.
The value of (-1)¹⁰⁰ is:
(a) 100
(b) -100
(c) 1
(d) -1
Answer:
(c) 1

Question 3.
Find the value of 2⁶ will be :
(a) 32
(b) 64
(c) 16
(d) 8
Answer:
(b) 64

Question 4.
The exponential form of 6 × 6 × 6 × 6 is :
(a) 6²
(b) 6°
(c) 6⁴
(d) 6⁵
Answer:
(c) 6⁴

Question 5.
The exponential notation of 512 is :
(a) 2⁶
(b) 2⁷
(c) 2⁸
(d) 2⁹
Answer:
(d) 2⁹

Question 6.
The exponential form of a × a × a × c × c × c × c × d is :
(a) a³c⁴d
(b) a⁸cd
(c) a³c⁵d
(d) ab⁵d³
Answer:
(a) a³c⁴d

Question 7.
Simplify: (-3)² × (-5)².
(a) 45
(b) 75
(c) 15
(d) 225
Answer:
(d) 225

Question 8.
Choose correct expanded form of 47051 out of the following :
(a) 4 × 10⁶ + 7 × 10⁵ + 5 × 10³ + 1 × 10²
(b) 4 × 10⁵ + 7 × 10⁴ + 5 × 10 + 1
(c) 4 × 10⁴ + 7 × 10³ + 5 × 10 + 1
(d) 4 × 10⁴ + 7 × 10³ + 5 × 10² + 1
Answer:
(c) 4 × 10⁴ + 7 × 10³ + 5 × 10 + 1

Question 9.
Find the number for the following form :
3 × 10⁴ + 7 × 10² + 5 × 10°
(a) 3075
(b) 30705
(c) 375
(d) 3750
Answer:
(b) 30705

Question 10.
The value of (2° + 3° + 4°) will be :
(a) 9
(b) 3
(c) 5
(d) 24
Answer:
(b) 3

Fill in the blanks :

Question 1.
The value of (1000)° is ………………
Answer:
1

Question 2.
The value of (1)¹⁰⁰⁰ is ………………
Answer:
1

Question 3.
The value of 2⁵ is ………………
Answer:
32

Question 4.
The exponential notation of 512 is ………………
Answer:
29

Question 5.
The exponential form of 5 × 5 × 5 × 5 × 5 × 5 is ………………
Answer:
56

Write True or False :

Question 1.
The value of a⁰ is 1. (True/False)
Answer:
True

Question 2.
The value of 2⁰ × 3⁰ × 4⁰ will be 24. (True/False)
Answer:
False

Question 3.
The value of (3⁰ + 5⁰ × 2⁰ will be 2. (True/False)
Answer:
True

Question 4.
a^m ÷ aⁿ = a^mn (True/False)
Answer:
False

Question 5.
(a^m)ⁿ = a^mn (True/False)
Answer:
True

Punjab State Board PSEB 7th Class Maths Book Solutions Chapter 9 Rational Numbers Ex 9.1 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 7 Maths Chapter 9 Rational Numbers Ex 9.1

1. Write two equivalent rational numbers of the following :

Question (i).
\(\frac {4}{5}\)
Solution:
\(\frac {4}{5}\) = \(\frac {4}{5}\) × \(\frac {2}{2}\)
= \(\frac {8}{10}\)
\(\frac {4}{5}\) = \(\frac {4}{5}\) × \(\frac {3}{3}\)
= \(\frac {12}{15}\)
∴ Equivalent rational numbers of \(\frac {4}{5}\) are \(\frac {8}{10}\) and \(\frac {12}{15}\)

Question (ii).
\(\frac {-5}{9}\)
Solution:
\(\frac {-5}{9}\) = \(\frac {-5}{9}\) × \(\frac {2}{2}\)
= \(\frac {-10}{18}\)
\(\frac {-5}{9}\) = \(\frac {-5}{9}\) × \(\frac {3}{3}\)
= \(\frac {-15}{27}\)
∴ Equivalent rational numbers of \(\frac {-5}{9}\) are \(\frac {-10}{18}\) and \(\frac {-15}{27}\)

Question (iii).
\(\frac {3}{-11}\)
Solution:
\(\frac {3}{-11}\) = \(\frac {3}{-11}\) × \(\frac {2}{2}\)
= \(\frac {6}{-22}\)
\(\frac {3}{-11}\) = \(\frac {3}{-11}\) × \(\frac {3}{3}\)
= \(\frac {9}{-33}\)
∴ Equivalent rational numbers of \(\frac {3}{-11}\) are \(\frac {6}{-22}\) and \(\frac {9}{-33}\)

2. Find the standard form of the following rational numbers :

Question (i).
\(\frac {35}{49}\)
Solution:
\(\frac {35}{49}\)
∵ H.C.F. of 35 and 49 is 7

So dividing both the numerator and denominator by 7 we get.
\(\frac {35}{49}\) = \(\frac{35 \div 7}{49 \div 7}\) = \(\frac {5}{7}\)
∴ Standard form of \(\frac {35}{49}\) is \(\frac {5}{7}\)

Question (ii).
\(\frac {-42}{56}\)
Solution:
\(\frac {-42}{56}\)
∵ H.C.F. of -42 and 56 is 14

So dividing both the numerator and denominator by 14 we get.
\(\frac {-42}{56}\) = \(\frac{-42 \div 14}{56 \div 14}\) = \(\frac{-3}{4}\)
∴ Standard form of \(\frac {-42}{56}\) is \(\frac{-3}{4}\)

Question (iii).
\(\frac {19}{-57}\)
Solution:
\(\frac {19}{-57}\)
∵ H.C.F. of 59 and 57 is 19

So dividing both the numerator and denominator by 19 we get.
\(\frac {19}{-57}\) = \(\frac{-19 \div 19}{-57 \div 19}\) = \(\frac{1}{-3}\)
∴ Standard form of \(\frac {19}{-57}\) is \(\frac{1}{-3}\)

Question (iv).
\(\frac{-12}{-36}\)
Solution:
\(\frac{-12}{-36}\)
∵ H.C.F. of 12 and 36 is 12.

So dividing both the numerator and denominator by 12 we get.
\(\frac{-12}{-36}\) = \(\frac{-12 \div 12}{-36 \div 12}\) = \(\frac{1}{3}\)
Standard form of \(\frac{-12}{-36}\) is \(\frac{1}{3}\)

3. Which of the following pairs represent same rational number ?

Question (i).
\(\frac{-15}{25}\) and \(\frac{18}{-30}\)
Solution:
\(\frac{-15}{25}\) = \(\frac{-15 \div 5}{25 \div 5}\)
= \(\frac{-3}{5}\)
\(\frac{18}{-30}\) = \(\frac{18 \div-6}{-30 \div-6}\)
= \(\frac{-3}{5}\)
∴ \(\frac{-15}{25}\) and \(\frac{18}{-30}\) represents the same number.

Question (ii).
\(\frac{2}{3}\) and \(\frac{-4}{6}\)
Solution:
\(\frac{2}{3}\) = \(\frac{2}{3}\) × \(\frac{1}{1}\)
= \(\frac{2}{3}\)
\(\frac{-4}{6}\) = \(\frac{-4 \div 2}{6 \div 2}\)
= \(\frac{-2}{3}\)
∴ \(\frac{-2}{3}\) and \(\frac{-4}{6}\) doesnot represents the same rational numbers.

Question (iii).
\(\frac{-3}{4}\) and \(\frac{-12}{16}\)
Solution:
\(\frac{-3}{4}\) = \(\frac{-3}{4}\) × \(\frac{4}{4}\)
= \(\frac{-12}{16}\)
\(\frac{-12}{16}\) = \(\frac{-12}{16}\)
∴ \(\frac{-3}{4}\) and \(\frac{-12}{16}\) represents the same rational number.

Question (iv).
\(\frac{-3}{-7}\) and \(\frac{3}{7}\)
Solution:
\(\frac{-3}{4}\) = \(\frac{-3 \div-1}{-7 \div-1}\)
= \(\frac{-3}{4}\)
∴ \(\frac{-3}{-7}\) and \(\frac{3}{7}\) represents the same rational number.

4. Which is greater in each of the following ?

Question (i).
\(\frac{3}{7}\), \(\frac{4}{5}\)
Solution:
Given rational nrnnbere are \(\frac{3}{7}\) and \(\frac{4}{5}\)
L.C.M. of 7 and 5 is 35
∴ \(\frac{3}{7}\) = \(\frac{3 \times 5}{7 \times 5}\)
= \(\frac{15}{35}\)
and \(\frac{4}{5}\) = \(\frac{4 \times 7}{5 \times 7}\)
= \(\frac{28}{35}\)
∵ Numerator of second is greater than first i.e. 28 > 15
So \(\frac{4}{5}\) > \(\frac{3}{7}\)

Question (ii).
\(\frac{-4}{12}\), \(\frac{-8}{12}\)
Solution:
Given rational numbere are \(\frac{-4}{12}\) and \(\frac{-8}{12}\)
∵ Numerator of first is greater than second i.e. -4 > – 8
∴ \(\frac{-4}{12}\) > \(\frac{-8}{12}\)

Question (iii).
\(\frac{-3}{9}\), \(\frac{4}{-18}\)
Solution:
Given rational numbers are \(\frac{-3}{9}\), \(\frac{4}{-18}\)
\(\frac{-3}{9}\) = \(\frac{-3 \times 2}{9 \times 2}\)
= \(\frac{-6}{18}\)
\(\frac{4}{-18}\) = \(\frac{4 \times-1}{-18 \times-1}\)
\(\frac{-4}{18}\)
Since -4 > – 6.
\(\frac{4}{-18}\) > \(\frac{-3}{9}\)

Question (iv).
-2\(\frac{3}{5}\), -3\(\frac{5}{8}\)
Solution:
-2\(\frac{3}{5}\) = \(\frac{-13}{5} \times \frac{8}{8}\)
= \(\frac{-104}{40}\)
-3\(\frac{5}{8}\) = \(\frac{-29}{8} \times \frac{5}{5}\)
= \(\frac{-135}{40}\)
∵ -104 > -135
∴ \(\frac{-13}{5}\) > \(\frac{-29}{8}\)
Thus, -2\(\frac{3}{5}\) > -3\(\frac{5}{8}\)

5. Write the following rational numbers in ascending order.

Question (i).
\(\frac{-5}{7}, \frac{-3}{7}, \frac{-1}{7}\)
Solution:
\(\frac{-5}{7}, \frac{-3}{7}, \frac{-1}{7}\)
Here -5 < -3 < -1
i.e. \(\frac{-5}{7}, \frac{-3}{7}, \frac{-1}{7}\)
Therefore, the ascending order is:
\(\frac{-5}{7}, \frac{-3}{7}, \frac{-1}{7}\)

Question (ii).
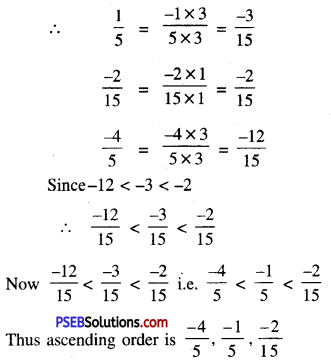
\(\frac{-1}{5}, \frac{-2}{15}, \frac{-4}{5}\)
Solution:
\(\frac{-1}{5}, \frac{-2}{15}, \frac{-4}{5}\)
L.C.M of 5, 15, 5 is 15

Question (iii).
\(\frac{-3}{8}, \frac{-2}{4}, \frac{-3}{2}\)
Solution:
\(\frac{-3}{8}, \frac{-2}{4}, \frac{-3}{2}\)
L.C.M of 8, 4, 2 is 8
∴ \(\frac{-3}{8}=\frac{-3}{8} \times \frac{1}{1}=\frac{-3}{8}\)
\(\frac{-2}{4}=\frac{-2 \times 2}{4 \times 2}=\frac{-4}{8}\)
\(\frac{-3}{2}=\frac{-3 \times 4}{2 \times 4}=\frac{-12}{8}\)
∴ -12 < -4 < -3
or \(\frac {-12}{8}\) < \(\frac {-4}{8}\) < \(\frac {-3}{8}\)
Hence assending order is \(\frac{-3}{2}, \frac{-2}{4}, \frac{-3}{8}\)

6. Write five rational numbers between following rational numbers.

Question (i).
-2 and -1
Solution:
Given rational numbers are -2 and -1
Let us write -2 and -1 as rational numbers with 5 + 1 = 6 as denominator.
We have -2 = -2 × \(\frac {6}{6}\)
= \(\frac {-6}{6}\)
\(\frac {-12}{6}\) < \(\frac {-11}{6}\) < \(\frac {-10}{6}\) < \(\frac {-9}{6}\) < \(\frac {-8}{6}\) < \(\frac {-7}{6}\) < \(\frac {-6}{6}\)
Hence five rational numbers between -2 and -1 are :
\(\frac {-11}{6}\),\(\frac {-10}{6}\),\(\frac {-9}{6}\),\(\frac {-8}{6}\),\(\frac {-7}{6}\)
i.e. \(\frac {-11}{6}\),\(\frac {-5}{3}\),\(\frac {-3}{2}\),\(\frac {-4}{3}\),\(\frac {-7}{6}\)

Question (ii).
\(\frac {-4}{5}\) and \(\frac {-2}{3}\)
Solution:
Given rational numbers are \(\frac {-4}{5}\) and \(\frac {-2}{3}\)
First we find equivalent rational numbers having same denominator
Thus \(\frac {-4}{5}\) = \(\frac{-4 \times 9}{5 \times 9}\)
= \(\frac {-36}{45}\)
and \(\frac {-2}{3}\) = \(\frac{-2 \times 15}{3 \times 15}\)
= \(\frac {-30}{45}\)
Now, we choose any five integers -35, -34, -33, -32, -31 between the numerators -36 and -30
Then the five rational numbers between \(\frac {-36}{45}\) and \(\frac {-30}{45}\) are:
\(\frac{-35}{45}, \frac{-34}{45}, \frac{-33}{45}, \frac{-32}{45}, \frac{-31}{45}\)
Hence, five rational numbers between \(\frac {-4}{5}\) and \(\frac {-2}{3}\) are
\(\frac{-35}{45}, \frac{-34}{45}, \frac{-33}{45}, \frac{-32}{45}, \frac{-31}{45}\)
i.e. \(\frac{-7}{9}, \frac{-34}{45}, \frac{-11}{15}, \frac{-32}{45}, \frac{-31}{45}\)

Question (iii).
\(\frac {1}{3}\) and \(\frac {5}{7}\)
Solution:
Given rational numbers are \(\frac {1}{3}\) and \(\frac {5}{7}\)
First we find equivalent rational numbers having same denominator

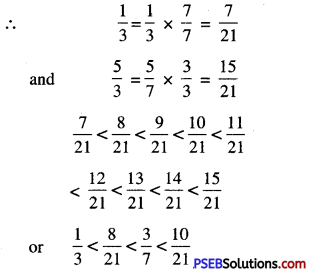
\(<\frac{4}{7}<\frac{13}{21}<\frac{2}{3}<\frac{5}{7}\)
Hence, five rational numbers between \(\frac {1}{3}\) and \(\frac {5}{7}\) are
\(\frac{8}{21}, \frac{3}{7}, \frac{10}{21}, \frac{4}{7}, \frac{13}{21}\).

7. Write four more rational numbers in each of the following.

Question (i).
\(\frac{-1}{5}, \frac{-2}{10}, \frac{-3}{15}, \frac{-4}{20}, \ldots\)
Solution:
The given rational numbers are :
\(\frac{-1}{5}, \frac{-2}{10}, \frac{-3}{15}, \frac{-4}{20}, \ldots\)
\(\frac {-1}{5}\) is the rational number in its lowest form
Now, we can write
\(\frac{-2}{10}=\frac{-1}{-5} \times \frac{2}{2}\),
\(\frac{-3}{15}=\frac{-1}{5} \times \frac{3}{3}\) and \(\frac{-1}{5}=\frac{-1}{5} \times \frac{4}{4}\)
Thus, we observe a pattern in these numbers.
The next four rational numbers would be
\(\frac{-1}{5} \times \frac{5}{5}=\frac{-5}{25}\),
\(\frac{-1}{5} \times \frac{6}{6}=\frac{-6}{30}\),
\(\frac{-1}{5} \times \frac{7}{7}=\frac{-7}{35}\)
\(\frac{-1}{5} \times \frac{8}{8}=\frac{-8}{40}\)
Hence required four more rational numbers are :
\(\frac{-5}{25}, \frac{-6}{30}, \frac{-7}{35}, \frac{-8}{40}\)

Question (ii).
\(\frac{-1}{7}, \frac{2}{-14}, \frac{3}{-21}, \frac{4}{-28}, \ldots\)
Solution:
The given rational numbers are
\(\frac{-1}{7}, \frac{2}{-14}, \frac{3}{-21}, \frac{4}{-28}, \ldots\)
\(\frac {-1}{7}\) is the rational number in its lowest form
Now, we can write
\(\frac{2}{-14}=\frac{-1}{7} \times \frac{-2}{-2}=\frac{2}{-14}, \frac{3}{-21}\)
= \(\frac{-1}{7} \times \frac{-3}{-3}\) and \(\frac{4}{-28}=\frac{-1}{7} \times \frac{-4}{-4}\)
Thus, we observe a pattern in these numbers.
The next four rational numbers would be :
\(-\frac{1}{7} \times \frac{-5}{-5}=\frac{5}{-35}\), \(\frac{-1}{7} \times \frac{-6}{-6}=\frac{6}{-42}\),
\(\frac{-1}{7} \times \frac{-7}{-7}=\frac{7}{-49}\), \(\frac{-1}{7} \times \frac{-8}{-8}=\frac{8}{-56}\)
Hence required four more rational numbers are :
\(\frac{5}{-35}, \frac{6}{-42}, \frac{7}{-49}, \frac{8}{-56}\)

8. Draw a number line and represent the following rational number on it.

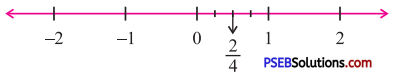
Question (i).
\(\frac {2}{4}\)
Solution:
Draw a line and choose a point O on it to represent the rational number zero (0). We choose a point A to the right of 0 to represent 1.
Divide the segment OA into four equal parts. Second part from O to the right represents the rational number \(\frac {2}{4}\) as shown in the figure.

Question (ii).
\(\frac {-3}{4}\)
Solution:
Draw a line and choose a point O on it to represent the rational number zero (0). We choose a point A to the right of 0 to represent -1.
Divide the segment OA into four equal parts. Third part from O to the left represents the rational number \(\frac {-3}{4}\) as shown in the figure.

Question (iii).
\(\frac {5}{8}\)
Solution:
Draw a line and choose a point O on it to represent the rational number zero (0).
We choose a point A to the right of 0 to represent 1.
Divide the segment OA into eight equal parts. Fifth part from O to the right represents the rational number as shown in the figure.

Question (iv).
\(\frac {-6}{4}\)
Solution:
Draw a line and choose a point O on it to represent the rational number zero (0).
We choose a point A to the left of O to represent -2.
Divide the segment OA into eight equal parts. Sixth part from O to the left represents the rational number \(\frac {-6}{4}\) as shown in the figure.

9. Multiple Choice Questions :

Question (i).
\(\frac{3}{4}=\frac{?}{12}\) then ? =
(a) 3
(b) 6
(c) 9
(d) 12.
Answer:
(c) 9

Question (ii).
\(\frac{-4}{7}=\frac{?}{14}\) then ? =
(a) -4
(b) -8
(c) 4
(d) 8
Answer:
(b) -8

Question (iii).
The standard form of rational number \(\frac {-21}{28}\) is
(a) \(\frac {-3}{4}\)
(b) \(\frac {3}{4}\)
(c) \(\frac {3}{7}\)
(d) \(\frac {-3}{7}\)
Answer:
(a) \(\frac {-3}{4}\)

Question (iv).
Which of the following rational number is not equal to \(\frac {7}{-4}\) ?
(a) \(\frac {14}{-8}\)
(b) \(\frac {21}{-12}\)
(c) \(\frac {28}{-16}\)
(d) \(\frac {7}{-8}\)
Answer:
(d) \(\frac {7}{-8}\)

Question (v).
Which of the following is correct ?
(a) 0 > \(\frac {-4}{9}\)
(b) 0 < \(\frac {-4}{9}\)
(c) 0 = \(\frac {4}{9}\)
(d) None
Answer:
(a) 0 > \(\frac {-4}{9}\)

Question (vi).
Which of the following is correct ?
(a) \(\frac{-4}{5}<\frac{-3}{10}\)
(b) \(\frac{-4}{5}>\frac{3}{-10}\)
(c) \(\frac{-4}{5}=\frac{3}{-10}\)
(d) None
Answer:
(a) \(\frac{-4}{5}<\frac{-3}{10}\)

Punjab State Board PSEB 6th Class Maths Book Solutions Chapter 3 Playing with Numbers Ex 3.3 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 6 Maths Chapter 3 Playing with Numbers Ex 3.3

1. Find prime factors of the following numbers by factor tree method:

Question (i)
96
Solution:

∴ Prime factorisation of 96 = 2 × 2 × 2 × 2 × 2 × 3

Question (ii)
120
Solution:

∴ Prime factorisation of 120 = 2 × 2 × 2 × 3 × 5

Question (iii)
180.
Solution:

∴ Prime factorisation of 180 = 2 × 2 × 3 × 3 × 5

2. Complete each factor tree:

Question (i)

Solution:

Question (ii)

Solution:

Question (iii)

Solution:

3. Find the prime factors of the following numbers by division method:

Question (i)
420
Solution:

∴ 420 = 2 × 2 × 3 × 5 × 7

Question (ii)
980
Solution:

∴ 980 = 2 × 2 × 5 × 7 × 7

Question (iii)
225
Solution:

∴ 225 = 3 × 3 × 5 × 5

Question (iv)
150
Solution:

∴ 150 = 2 × 3 × 5 × 5

Question (v)
324
Solution:

∴ 324 = 2 × 2 × 3 × 3 × 3 × 3