PSEB 8th Class Maths Solutions Chapter 11 Mensuration Ex 11.1

Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 11 Mensuration Ex 11.1 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 8 Maths Chapter 11 Mensuration Ex 11.1

1. A square and a rectangular field with measurements as given in the figure have the same perimeter. Which field has a larger area?

Question (a)

Solution:
Side of a square field = 60 m
∴ Perimeter of a square field = 4 × side
= 4 × 60 = 240 m
Area of a square field = (side)²
= (60)²
= 60 × 60
= 3600m²

Question (b)

Solution:
Perimeter of a rectangular field = Perimeter of square field
∴ Perimeter of a rectangular held = 240
∴ 2 (length + breadth) = 240
∴ 2 (80 + breadth) = 240
∴ 80 + breadth = \(\frac {240}{2}\)
∴ 80 + breadth =120
∴ breadth = 120 – 80
∴ breadth = 40
Breadth of rectangular field = 40 m
∴ Area of rectangular field = length × breadth
= (80 × 40)
= 3200 m²
Area of square field > Area of rectangular field
Thus, area of square field (a) is larger.

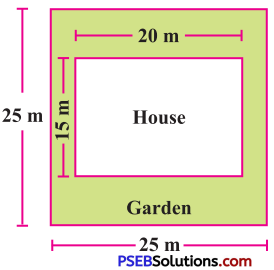
2. Mrs. Kaushik has a square plot with the measurement as shown g in the figure. She wants to construct a house in the middle of the plot. A garden is developed around the house. Find the total cost of developing a garden around the house at the rate of ₹ 55 per m².

Solution:
Side of the square plot = 25 m
∴ Area of the square plot = (side)²
= (25 × 25) m²
= 625 m²
In square plot, a rectangular-shaped house is to be constructed.
∴ Area of the constructed house
= length × breadth
= (20 × 15) m²
= 300 m²
∴ Area of the garden = Area of square plot – Area of constructed house
= 625 – 300 = 325 m²
Cost of developing garden of 1 m² is ₹ 55
∴ Cost of developing garden of 325 m²
= ₹ (55 × 325)
= ₹ 17,875
Thus, total cost of developing garden is ₹ 17,875.

3. The shape of a garden is rectangular in the middle and semicircular at the ends as shown in the diagram. Find the area and the perimeter of this garden [Length of rectangle is 20 – (3.5+ 3.5) metres].

Solution:
[Note: Here 2 semicircles at the ends of a rectangular garden makes a whole circle. So first find area of a circle and then area of a rectangle. Sum of these two areas is total area. Follow same pattern to find perimeter too. For perimeter of a garden, take only length as rectangle is between two semicircles. Diameter of a circle = Breadth of a rectangle = 7 m]
For semicircle:
∴ Radius = \(\frac{\text { diameter }}{\text { 2 }}\) = \(\frac {7}{2}\)m
Area of circle = πr²
Area of a semicircle = \(\frac {1}{2}\)πr²
∴ Area of 2 semicircles = 2(\(\frac {1}{2}\)πr²)
= \(\frac{22}{7} \times \frac{7}{2} \times \frac{7}{2}\)m²
= 38.5 m²
Circumference of two semicircles = 2πr
= 2 × \(\frac {22}{7}\) × \(\frac {7}{2}\)
= 22 m

For rectangle:
length = 20 – (3.5 + 3.5) = 20 – 7 = 13 m
breadth = 7 m
Area of the rectangle = length × breadth
= 13 × 7 = 91 m²
Perimeter of the rectangle
= 2 (length × breadth)
= 2 (13 + 0)
= 2 × 13 = 26 m
∴ Total area of the garden = (38.5 + 91) m²
= 129.5 m²
∴ Perimeter of the garden = (22 + 26) m
= 48 m
Thus, area of the garden is 129.5 m² and the perimeter is 48 m.

4. A flooring tile has the shape of a parallelogram whose base is 24 cm and the corresponding height is 10 cm. How many such tiles are required to cover a floor of area 1080 m²? (If required you can split the tiles in whatever way you want to fill up the corners.)
Solution:
[Note : To find number of tiles, divide the area of the floor by area of a tile. Let us do it in a simple way. Unit of floor area and tile area should be same.] Here, tile is parallelogram shaped.
So it’s area = base × corresponding height
Area of a floor = 1080 m²
Base of a tile = 24 cm = \(\frac {24}{100}\) m
Corresponding height of a tile = 10 cm = \(\frac {10}{100}\) m
Number of tiles = \(\frac{\text { Area of a floor }}{\text { Area of a title }}\)
= \(\frac{1080}{\frac{24}{100} \times \frac{10}{100}}\)
= \(\frac{1080 \times 100 \times 100}{24 \times 10}\)
= 45,000
Thus, 45,000 tiles are required to cover the given floor.

5. An ant is moving around a few food pieces of different shapes scattered on the floor. For which food piece would the ant have to take a longer round? Remember, circumference of a circle can be obtained by using the expression c = 2 πr, where r is the radius of the circle.

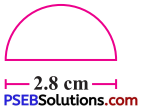
Question (a)

Solution:
Here, the shape is semi-circular.
Diameter = 2.8 cm
Radius = \(\frac{\text { Diameter }}{2}=\frac{2.8}{2}\) = 1.4 cm
Circumference of a semicircle = πr
Perimeter of the given figure
= πr + diameter
= (\(\frac {22}{7}\) × 1.4) + 2.8
= 4.4 + 2.8
= 7.2 cm

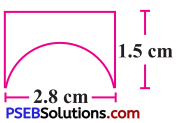
Question (b)

Solution:
Here, given shape is semicircular at one side, (radius = \(\frac {2.8}{2}\) = 1.4 cm)
So perimeter of semicircular region (circumference) = πr
= \(\frac {22}{7}\) × 1.4
= \(\frac {22}{7}\) × \(\frac {14}{10}\)
= 4.4 cm … (i)
Perimeter of the other portion
= breadth + length + breadth
= (1.5 + 2.8 + 1.5) cm
= 5.8 cm … (ii)
∴ Perimeter of the given figure
= (4.4 + 5.8) cm [from (i) and (ii)]
= 10.2 cm

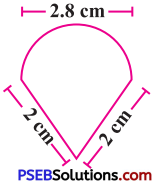
Question (c)

Solution:
Perimeter of a given part
(semi circular circumference) = πr
= \(\frac {22}{7}\) × 1.4
= 4.4 cm
∴ Perimeter of the given figure
= (4.4 + 2 + 2) cm
= 8.4 cm
Thus, 7.2 cm < 8.4 cm < 10.2 cm.
Thus, the ant would has to take a longer round for food piece (b), as it has a larger perimeter.