Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 8 Comparing Quantities InText Questions and Answers.
PSEB 8th Class Maths Solutions Chapter 8 Comparing Quantities InText Questions
Try These (Textbook Page No. 119)
In a primary school, the parents were asked about the number of hours they spend per day in helping their children to do homework. There were 90 parents who helped for \(\frac {1}{2}\) hour to 1\(\frac {1}{2}\) hours. The distribution of parents according to the time for which, they said they helped is given in the figure;
20 % helped for more than 1\(\frac {1}{2}\) hours per day;
30 % helped for \(\frac {1}{2}\) hour to 1\(\frac {1}{2}\) hours;
50 % did not help at all.
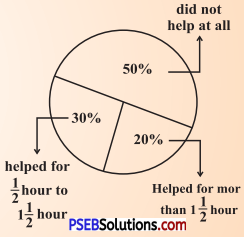
Using this, answer the following:
Question (i).
How many parents were surveyed ?
Solution:
90 parents helped their children for \(\frac {1}{2}\) h to 1\(\frac {1}{2}\) h.
Given, percentage in pie chart = 30 %
Let x parents be surveyed.
30 % of x helped for \(\frac {1}{2}\) h to 1\(\frac {1}{2}\) h = 90
∴ 30 % of x = 90
∴ \(\frac {30}{100}\) × x = 90
∴ x = \(\frac{90 \times 100}{30}\)
∴ x = 300
Thus, 300 parents were surveyed.
Question (ii).
How many said that they did not help ?
Solution:
50% parents did not help.
So the number of parents who did not help :
= 50 % of 300
= \(\frac {1}{2}\) × 300
= 50 × 3
= 150
Thus, 150 parents did not help.
Question (iii).
How many said that they helped for more than 1\(\frac {1}{2}\) hours ?
Solution:
20% parents helped for more than 1\(\frac {1}{2}\)h.
So the number of parents who helped for more than 1 \(\frac {1}{2}\) h
= 20% of 300
= \(\frac {20}{100}\) × 300
= 20 × 3
= 60
Thus, 60 parents helped for more than 1\(\frac {1}{2}\) h.
![]()
Try These (Textbook Page No. 121)
1. A shop gives 20% discount. What would the sale price of each of these be?
Question (a).
A dress marked at ₹ 120
Solution:
Marked price of the dress = ₹ 120
Discount offered = 20 % )
∴ Discount = 20% of MP
= \(\frac {20}{100}\) × 120 = ₹ 24
∴ Sale price of the dress = MP – Discount
= ₹ (120 – 24)
= ₹ 96
Thus, sale price of the dress is ₹ 96.
Question (b).
A pair of shoes marked at ₹ 750
Solution:
Marked price of the pair of shoes = ₹ 750
Discount offered = 20 %
∴ Discount = 20 % of MP
= \(\frac {20}{100}\) × 750 = ₹ 150
∴ Sale price of the pair of shoes
= MP – Discount s
= ₹ (750 – 150) = ₹ 600
Thus, sale price of the pair of shoes is ₹ 600.
Question (c).
A bag marked at ₹ 250
Solution:
Marked price of the bag = ₹ 250
Discount offered = 20 %
∴ Discount = 20 % of MP
= \(\frac {20}{100}\) × 250 = ₹ 50
∴ Sale price of the bag = MP – Discount
= ₹ (250 – 50)
= ₹ 200
Thus, sale price of the bag is ₹ 200.
2. A table marked at ₹ 15,000 is available for ₹ 14,400. Find the discount given and the discount per cent.
Solution:
Marked price of the table = ₹ 15,000
Sale price of the table = ₹ 14,400
Discount = MP – SP
= ₹ (15000 – 14400)
= ₹ 600
Discount per cent = \(\frac{\text { Discount }}{\text { MP }}\) × 100
= \(\frac{600}{15000}\) × 100
= \(\frac {60}{15}\)
= 4 %
Thus, the discount = ₹ 600 and discount per cent = 4%.
3. An almirah is sold at ₹ 5225 after allowing a discount of 5%. Find its marked price.
Solution:
Sale price of the almirah = ₹ 5225
Discount per cent = 5 %
Let marked price of the almirah be ₹ x.
∴ Discount = 5 % of x
= \(\frac {5}{100}\) × x
= \(\frac{5 x}{100}\)
Sale price = MP – Discount
= x – \(\frac{5 x}{100}\)
=\(\frac{100 x-5 x}{100}\)
= \(\frac{95 x}{100}\)
Sale price of the almirah = ₹ 5225 (Given)
∴ \(\frac{95 x}{100}\) = 5225
∴ x = \(\frac{5225 \times 100}{95}\)
= 5500
Thus, the marked price of the almirah is ₹ 5500.
![]()
Try These (Textbook Page No. 123)
1. Find selling price (SP) if a profit of 5 % is made on:
Question (a).
A cycle of ₹ 700 with ₹ 50 as overhead charges.
Solution:
Cost price of the cycle = ₹ 700
Overhead charges = ₹ 50
Total cost price = Cost price + Overhead charges
= ₹ (700 + 50)
= ₹ 750
Profit per cent = 5 %
Profit amount = 5% of total cost of the cycle
= ₹ \(\left(\frac{5}{100} \times 750\right)\)
= ₹ \(\frac{3750}{100}\)
= ₹ 37.50
∴ Selling price of the cycle = Total cost of the cycle + Profit
= ₹ (750 + 37.50)
= ₹ 787.50
Thus, the selling price of the cycle is ₹ 787.50.
Question (b).
A lawn mower bought at ₹ 1150 with ₹ 50 as transportation charges.
Solution:
Cost price of the lawn mower = ₹ 1150
Transportation (overhead) charges = ₹ 50
Total cost price = Cost price + Overhead charges
= ₹ (1150 + 50)
= ₹ 1200
Profit per cent = 5 %
Profit amount = 5 % of total cost price of the lawn mower
= \(\frac {5}{100}\) × 1200
= ₹ \(\left(\frac{5}{100} \times 1200\right)\)
= ₹ 60
∴ Selling price of the lawn mower = Total cost price of the lawn mower + Profit
= ₹ (1200 + 60)
= ₹ 1260
Thus, the selling price of the lawn mower is ₹ 1260.
Question (c).
A fan bought for ₹ 560 and expenses of ₹ 40 made on its repairs.
Solution:
Cost price of the fan = ₹ 560
Repair (overhead) charges = ₹ 40
Total cost price = Cost price + Overhead charges
= ₹ (560 + 40)
= ₹ 600
Profit per cent = 5 %
Profit amount = 5% of total cost of the fan
= ₹ \(\left(\frac{5}{100} \times 600\right)\)
= ₹ 30
∴ Selling price of the fan = Total cost price of the fan + Profit
= ₹ (600 + 30)
= ₹ 630
Thus, the selling price of the fan is ₹ 630.
![]()
Try These (Textbook Page No. 123)
1. A shopkeeper bought two TV sets at ₹ 10,000 each. He sold one at a profit of 10% and the other at a loss of 10%. Find whether he made an overall profit or loss.
Solution:
Cost price of each TV set = ₹ 10,000
Cost price of two TV sets = 2 × ₹ 10000
= ₹ 20,000
For TV set sold at a profit:
Profit per cent =10% |
∴ Profit amount =10% of a cost price
= \(\left(\frac{10}{100} \times 10000\right)\)
= ₹ 1000
Selling price = Cost price + Profit
= ₹ (10000 + 1000)
= ₹ 11,000
For TV set sold at a loss:
Loss per cent = 10%
∴ Loss amount = 10% of a cost price
= \(\left(\frac{10}{100} \times 10000\right)\)
= ₹ 1000
Selling price = Cost price – Loss
= ₹ (10000 – 1000)
= ₹ 9000
Total selling price of 2 TV sets
= ₹ (11000 + 9000)
= ₹ 20,000
∴ Cost price of 2 TV sets = Selling price of 2 TV sets
Thus, there is neither profit nor loss.
Think, Discuss and Write (Textbook Page No. 125)
1. Two times a number is a 100% increase in the number. If we take half the number what would be the decrease in per cent?
Solution:
Let the number be x
∴ Half the number = \(\frac{x}{2}\)
Now, decrease in number = x – \(\frac{x}{2}\)
= \(\frac{2 x-x}{2}\)
= \(\frac{x}{2}\)
Decrease per cent = \(\left(\frac{\text { Decrease }}{\text { Original value }} \times 100\right) \%\)
= \(\left(\frac{\frac{x}{2}}{x} \times 100\right) \%\)
= \(\left(\frac{x}{2} \div \frac{x}{1} \times 100\right) \%\)
= \(\left(\frac{x}{2} \times \frac{1}{x} \times 100\right) \%\)
= 50%
Thus, 50% would be the decrease in number, if we take half the number.
2. By what per cent is ₹ 2000 less than ₹ 2400? Is it the same as the per cent by which ₹ 2400 is more than ₹ 2000?
Solution:
(a) ₹ (2400 – 2000) = ₹ 400
i.e., ₹ 2000 is less than ₹ 2400 by ₹ 400.
∴ Percentage decrease = \(\left(\frac{\text { Decrease in value }}{\text { Original value }} \times 100\right) \%\)
= \(\left(\frac{400}{2400} \times 100\right) \%\)
= \(\left(\frac{400}{24}\right) \%\)
= 6\(\frac {1}{2}\)%
Thus, percentage decrease is 16\(\frac {2}{3}\)%%
(b) ₹ (2400 – 2000) = ₹ 400
i.e., ₹ 2400 is more than ₹ 2000 by ₹ 400.
∴ Percentage increase = \(\left(\frac{\text { Increase in value }}{\text { Original value }} \times 100\right) \%\)
= \(\left(\frac{400}{2000} \times 100\right) \%\)
= (4 × 5) %
= 20%
∴ Percentage increase is 20 %.
Thus, percentage increase and percentage decrease are not the same.
![]()
Try These (Textbook Page No. 126)
Find interest and amount to be paid on f 15,000 at 5% per annum after 2 years.
Solution:
Here, P = ₹ 15,000; R = 5%, T = 2 years
SI = \(\frac{P \times R \times T}{100}\)
= \(\frac{15000 \times 5 \times 2}{100}\)
= ₹ 1500
Amount = Principal + Interest
= ₹ (15000 + 1500)
= ₹ 16,500
OR
∴ interest on ₹ 15,000 for 1 year
= ₹ \(\frac {15000}{100}\) × 5
= ₹ 750
∴ interest on ₹ 15,000 for 2 years
= ₹ 750 × 2
= 1500
Amount = Principal + Interest
= ₹ (15000 + 1500)
= ₹ 16500
Thus, interest ₹ 1500 and amount ₹ 16,500.
Try These (Textbook Page No. 129)
1. Find CI on a sum of ₹ 8000 for 2 years at 5% per annum compounded annually.
Solution:
Here, P = ₹ 8000, R = 5 %, n = 2 years
A = P\(\left(1+\frac{\mathrm{R}}{100}\right)^{n}\)
= 8000\(\left(1+\frac{5}{100}\right)^{2}\)
= 8000\(\left(\frac{100+5}{100}\right)^{2}\)
= 8000\(\left(\frac{105}{100}\right)^{2}\)
= 8000 × \(\frac {21}{20}\) × \(\frac {21}{20}\) = 8820
Thus, amount = ₹ 8820
Compound interest = Amount – Interest
= ₹ (8820 – 8000)
= ₹ 820
Thus, compound interest = ₹ 820
![]()
Try These (Textbook Page No. 130)
Find the time period and rate for each:
1. A sum taken for 1\(\frac {1}{2}\) years at 8% per annum is compounded half yearly.
Solution:
[Note : If interest is compounded half yearly, then the rate of interest (R) will be half i.e., \(\frac{\mathrm{R}}{2}\)% and time (n) will be double i.e., 2n years.]
n (time period) = 1\(\frac {1}{2}\) years
= \(\frac {3}{2}\) years
For half yearly, time period = \(\frac {3}{2}\) × 2 = 3
R (rate of interest) = 8%
For half yearly, rate of interest = \(\frac {8}{2}\)% = 4%
Thus, here n = 3 and R = 4%
2. A sum taken for 2 years at 4% per annum compounded half yearly.
Solution:
n (time period) = 2 years
For half yearly period = 2 × 2 = 4
R (rate of interest) = 4%
For half yearly, rate of interest = \(\frac {2}{2}\) % = 2 %
Think, Discuss and Write (Textbook Page No. 130)
A sum is taken for one year at 16% p.a. If interest is compounded after every three months, how many times will interest be charged in one year?
Solution:
Here, the interest is compounded after every three months (quarterly).
For quarterly,
R = 16% p.a.
= \(\frac {16}{4}\)% = 4%.
n = 1 year
= 1 × 4
= 4
Thus, interest will be charged 4 times in one year.
Try These (Textbook Page No. 131)
Find the amount to be paid:
1. At the end of 2 years on ₹ 2400 at 5 % per annum compounded annually.
Solution:
Here, P = ₹ 2400, n = 2 years, R = 5%
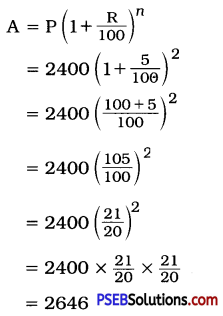
Thus, amount to be paid = ₹ 2646
2. At the end of 1 year on ₹ 1800 at 8% per annum compounded quarterly.
Solution:
Here, the interest is compounded quarterly.
P = ₹ 1800, R = \(\frac {8}{4}\) = 2 %, T = 1 year
n = 4 × 1 = 4

Thus, amount to be paid = ₹ 1948.38
![]()
Try These (Textbook Page No. 133)
1. A machinery worth ₹ 10,500 depreciated by 5%. Find its value after one year.
Solution:
Here, P = ₹ 10,500; R = – 5 %; T = 1 year
∴ n = 1
A = \(\mathrm{P}\left(1-\frac{\mathrm{R}}{100}\right)^{n}\)
(∵ R = -5 – means depreceation)
∴ A = 10500\(\left(1-\frac{5}{100}\right)^{1}\)
∴ A = 10500\(\left(\frac{100-5}{100}\right)\)
= 10500 × \(\frac {95}{100}\)
= 9975
Thus, the valu of a machinery after one year will be ₹ 9975.
2. Find the population of a city after 2 years, which is at present 12 lakh, if the rate of increase is 4%.
Solution:
Here, P = 12,00,000; Rate of increase R = 4 %; T = 2 years; n = 2
Population after 2 years = \(\mathrm{P}\left(1+\frac{\mathrm{R}}{100}\right)^{n}\)
= 120000\(\left(1+\frac{4}{100}\right)^{2}\)
= 120000\(\left(\frac{100+4}{100}\right)^{2}\)
= 120000\(\left(\frac{104}{100}\right)^{2}\)
= 1200000 x \(\frac {104}{100}\) × \(\frac {104}{100}\)
= 120 × 104 × 104
= 12,97,920
Thus, the population of a city after 2 years will be 12,97,920.