PSEB 8th Class Maths Solutions Chapter 6 Squares and Square Roots Ex 6.4

Punjab State Board PSEB 8th Class Maths Book Solutions Chapter 6 Squares and Square Roots Ex 6.4 Textbook Exercise Questions and Answers.

PSEB Solutions for Class 8 Maths Chapter 6 Squares and Square Roots Ex 6.4

1. Find the square root of each of the following numbers by Division method.

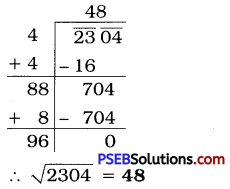
Question (i).
2304
Solution:

Question (ii).
4489
Solution:

Question (iii).
3481
Solution:

Question (iv).
529
Solution:

Question (v).
3249
Solution:

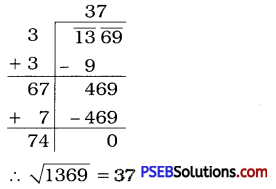
Question (vi).
1369
Solution:

Question (vii).
5776
Solution:

Question (viii).
7921
Solution:

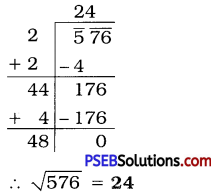
Question (ix).
576
Solution:

Question (x).
1024
Solution:

Question (xi).
3136
Solution:

Question (xii).
900
Solution:

2. Find the number of digits in the square root of each of the following numbers (without any calculation).

Question (i).
64
Solution:
Here, number of digits, n = 2
(Which is an even number.)
∴ Number of digits in the square root of 64 = \(\frac{n}{2}=\frac{2}{2}\) = 1

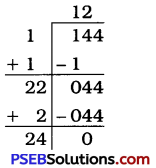
Question (ii).
144
Solution:
Here, number of digits, n = 3
(Which is an odd number.)
∴ Number of digits in the square root of 144 = \(\frac{n+1}{2}=\frac{3+1}{2}\)
= \(\frac {4}{2}\)
= 2

Question (iii).
4489
Solution:
Here, number of digits, n = 4
(Which is an even number.)
∴ Number of digits in the square root of 4489 = \(\frac{n}{2}=\frac{4}{2}\)
= 2

Question (iv).
27225
Solution:
Here, number of digits, n = 5
(Which is an odd number.)
∴ Number of digits in the square root of 27225 = \(\frac{n+1}{2}=\frac{5+1}{2}\)
= \(\frac {6}{2}\)
= 3

Question (v).
390625
Solution:
Here, number of digits, n = 6
(Which is an even number.)
∴ Number of digits in the square root of 390625 = \(\frac{n}{2}=\frac{6}{2}\)
= 3

3. Find the square root of the following decimal numbers.

Question (i).
2.56
Solution:

Here, number of decimal places are two.
∴ The number of decimal places in square root should be one.

Question (ii).
7.29
Solution:

Here, number of decimal places are two.
∴ The number of decimal places in square root should be one.

Question (iii).
51.84
Solution:

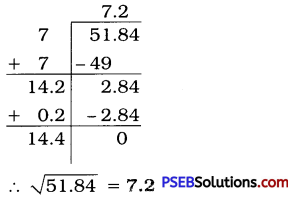
Here, number of 51.84 decimal places are two.
∴ The number of decimal places in square root should be one.

Question (iv).
42.25
Solution:

Question (v).
31.36
Solution:

4. Find the least number which must be subtracted from each of the following numbers so as to get a perfect square. Also find the square root of the perfect square so obtained.

Question (i).
402
Solution:

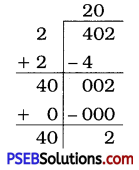
Here, the remainder is 2. It shows that 20² is less than 402 by 2.
So, to get a perfect square, 2 must be subtracted from given number.
∴ Required perfect square number = 402 – 2 = 400
\(\sqrt{400}\) = 20

Question (ii).
1989
Solution:

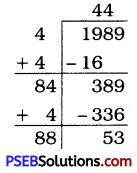
Here, the remainder is 53. It shows that 44² is less than 1989 by 53.
So, to get a perfect square, 53 must be subtracted from the given number.
∴ Required perfect square number = 1989 – 53 = 1936
\(\sqrt{1936}\) = 44

Question (iii).
3250
Solution:

Here, the remainder is 1. It shows that 57² is less them 3250 by 1.
So, to get a perfect square, 1 must be subtracted from the given number.
∴ Required perfect square number = 3250 – 1 = 3249
\(\sqrt{3249}\) = 57

Question (iv).
825
Solution:

Here, the remainder is 41. It shows that 28² is less than 825 by 41.
So, to get a perfect square, 41 must be subtracted from the given number.
∴ Required perfect square number = 825 – 41 = 784
\(\sqrt{784}\) = 28

Question (v).
4000
Solution:

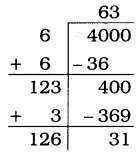
Here, the remainder is 31. It shows that 63² is less than 4000 by 31.
So, to get a perfect square, 31 must be subtracted from the given number.
∴ Required perfect square number = 4000 – 31 = 3969
\(\sqrt{3969}\) = 63

5. Find the least number which must be added to each of the following numbers so as to get a perfect square. Also find the square root of the perfect square so obtained.

Question (i).
525
Solution:

Here, the remainder is 41.
525 > 22²,
and the number next to 22 is 23.
23² = 529.
∴ The required number to be added
= 23² – 525
= 529 – 525 = 4
Now, 525 + 4 = 529
\(\sqrt{529}\) = 23
Thus, 4 is the least number which must be added to 525 to get a perfect square.

Question (ii).
1750
Solution:

Here, the remainder is 69.
1750 > 41²,
and the number next to 41 is 42.
42² = 1764.
∴ The required number to be added = 42² – 1750
= 1764 – 1750
= 14
Now, 1750 + 14 = 1764
\(\sqrt{1764}\) = 42
Thus, 14 is the least number which must be added to 1750 to get a perfect square.

Question (iii).
252
Solution:

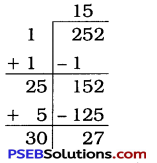
Here, the remainder is 27.
252 > 15²,
and the number next to 15 is 16.
16² = 256.
∴ The required number to be added = 16² – 252
= 256 – 252
= 4
Now, 252 + 4 = 256
\(\sqrt{256}\) = 16
Thus, 4 is the least number which must be added to 252 to get a perfect square.

Question (iv).
1825
Solution:

Here, the remainder is 61.
1825 > 42²,
and the number next to 42 is 43.
43² = 1849.
∴ The required number to be added 43² – 1825
= 1849 – 1825
= 24
Now, 1825 + 24 = 1849
\(\sqrt{1849}\) = 43
Thus, 24 is the least number which must be added to 1825 to get a perfect square.

Question (v).
6412
Solution:

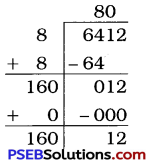
Here, the remainder is 12.
6412 > 80²,
and the number next to 80 is 81.
81² = 6561.
∴ The required number to be added = 81² – 6412
= 6561 – 6412
= 149
Now, 6412 + 149 = 6561
\(\sqrt{6561}\) = 81
Thus, 149 is the least number which must be added to 6412 to get a perfect square.

6. Find the length of the side of a square whose area is 441 m².
Solution:
Let the side of a square be x m.
Area of a square = x × x = x²
Area of a square = 441 (given)

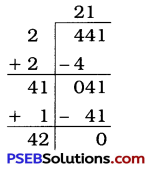
∴x² = 441
∴ x = \(\sqrt{441}\)
∴ x = 21
Thus, the length of the side of the square is 21 m.

7. In a right triangle ABC, ∠B = 90° :
(a) If AB = 6 cm, BC = 8 cm, find AC.
(b) If AC = 13 cm, BC = 5 cm, find AB.
Solution:
[Note: In a right triangle, the longest side is called the hypotenuse. The square of the hypotenuse is equal to the sum of the squares of the remaining two sides.]
Let us use this theorem here.
(a) Here, ∠B = 90°, AB = 6 cm, BC = 8 cm
In ΔABC, AC is hypotenuse.

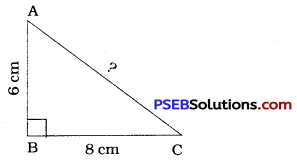
AC² = AB² + BC²
= (6)² + (8)²
= 36 + 64
=100
∴ AC = \(\sqrt{100}\)
= 10cm

(b) Here, ∠B= 90°, AC = 13cm, BC = 5cm
In ΔABC, AC Is hypotenuse.


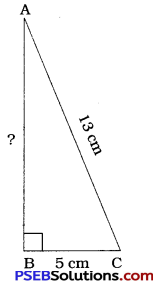
AC² = AB² + BC²
∴ (13)² = AB² + (5)²
∴ 169 = AB² + 25
∴ AB² = 169 – 25
= 144
∴ AB = \(\sqrt{144}\)
= 12 cm

8. A gardener has 1000 plants. He wants to plant these in such a way that the number of rows and the number of columns remain same. Find the minimum number of plants he needs more for this.
Solution:
Total number of plants = 1000
The number of plants in a row = The number of plants in a column
Let the number of plants planted in a row be x.
So, the number of plants planted in a column is x.
∴ Total plants = x × x = x²
∴ x² > 1000
∴ x > \(\sqrt{1000}\)
Here, the remainder is 39.
(31)² < 1000
The next square number would be 32.
32² = 1024
∴ The number of plants required to be added = 1024 – 1000
= 24
Thus, 24 more plants needed.

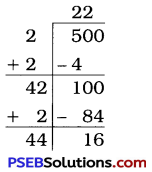
9. There are 500 children in a school. For a P.T. drill they have to stand in such a manner that the number of rows is equal to number of columns. How many children would be left out in this arrangement?
Solution:
Total number of children in a school = 500
The number of rows = The number of columns
Let the number of children in a row be x.
So, the number of children in a column is x.
Total number of children = x × x = x².
∴ x² < 500
∴ x < \(\sqrt{500}\)
Here, the remainder is 16.
500 > 22²
∴ 500 > 484
500 – 484 = 16
Thus, 16 children would be left out in this arrangement.