Punjab State Board PSEB 12th Class Maths Book Solutions Chapter 2 Inverse Trigonometric Functions Miscellaneous Exercise Textbook Exercise Questions and Answers.
PSEB Solutions for Class 12 Maths Chapter 2 Inverse Trigonometric Functions Miscellaneous Exercise
Find the value of the following :
Question 1.
cos-1 (cos \(\frac{13 \pi}{6}\)).
Solution.
We know that cos-1(cos x) = x if x ∈ [0, π], which is the principal value of cos-1 x.
Here, \(\frac{13 \pi}{6}\) ∉ [0, π].
Now, cos-1 (cos \(\frac{13 \pi}{6}\)) can be written as
cos-1 (cos \(\frac{13 \pi}{6}\)) = cos-1 [cos (2π + \(\frac{pi}{6}\))]
= cos-1 [cos (\(\frac{pi}{6}\))],
where \(\frac{pi}{6}\) ∈ [0, π]
[∵ cos(2π + x) = cos x]
∴ cos-1 (cos \(\frac{13 \pi}{6}\)) = cos-1 [cos (\(\frac{pi}{6}\))]
= \(\frac{pi}{6}\).
![]()
Question 2.
tan-1 (tan \(\frac{7 \pi}{6}\))
Solution.
We know that tan-1(tan x) = x if x ∈ (\(-\frac{\pi}{2}, \frac{\pi}{2}\)) which is the principal value of cos-1 x.
Here, \(\frac{7 \pi}{6}\) ∉ (\(-\frac{\pi}{2}, \frac{\pi}{2}\))
Now, tan-1 (tan \(\frac{7 \pi}{6}\)) can be written as
tan-1 (tan \(\frac{7 \pi}{6}\)) = tan-1 (tan (π + \(\frac{\pi}{6}\)))
= tan-1 [tan (\(\frac{\pi}{6}\))]
where \(\frac{pi}{6}\) ∈ (\(-\frac{\pi}{2}, \frac{\pi}{2}\))
[∵ tan(π + x) = tan x]
∴ tan-1 (tan \(\frac{7 \pi}{6}\)) = tan-1 [tan (\(\frac{\pi}{6}\))]
= \(\frac{pi}{6}\)
Prove that
Question 3.
2 sin-1 \(\frac{3}{5}\) = tan-1 \(\frac{24}{7}\).
Solution.
Let sin-1 \(\frac{3}{5}\) = x.
Then, sin x = \(\frac{3}{5}\)
⇒ cos x = \(\sqrt{1-\left(\frac{3}{5}\right)^{2}}=\sqrt{1-\frac{9}{25}}=\sqrt{\frac{25-9}{25}}=\sqrt{\frac{16}{25}}=\frac{4}{5}\)
∴ tan x = \(\frac{3 / 5}{4 / 5}=\frac{3}{4}\)
∴ x = tan-1 \(\frac{3}{4}\)
⇒ sin-1 \(\frac{3}{5}\) = tan-1 \(\frac{3}{4}\)
Now, we have
L.H.S = 2 sin-1 \(\frac{3}{5}\) = 2 tan-1 \(\frac{3}{4}\)
= tan-1 \(\left(\frac{2 \times \frac{3}{4}}{1-\left(\frac{3}{4}\right)^{2}}\right)\)
[∵ 2 tan-1 x = tan-1 \(\frac{2 x}{1-x^{2}}\)]
= tan-1 \(\left(\frac{\frac{3}{2}}{\frac{16-9}{16}}\right)\)
= tan-1 \(\left(\frac{3}{2} \times \frac{16}{7}\right)\)
= tan-1 \(\frac{24}{7}\)
= R.H.S.
Hence proved.
![]()
Question 4.
sin-1 \(\frac{8}{17}\) + sin-1 \(\frac{3}{5}\) = tan-1 \(\frac{77}{36}\)
solution.
Let sin-1 \(\frac{8}{17}\) = x.
Then, sin x = \(\frac{8}{17}\)
⇒ cos x = \(\sqrt{1-\left(\frac{8}{17}\right)^{2}}=\sqrt{\frac{225}{289}}=\frac{15}{17}\)
∴ tan x = \(\frac{8 / 17}{15 / 17}=\frac{8}{15}\)
⇒ x = tan-1 \(\frac{8}{15}\)
∴ sin-1 \(\frac{8}{17}\) = tan-1 \(\frac{8}{15}\) …………..(i)
Now, let sin-1 \(\frac{3}{5}\) = y.
Then, sin y = \(\frac{3}{5}\)
⇒ cos y = \(\sqrt{1-\left(\frac{3}{5}\right)^{2}}=\sqrt{\frac{16}{25}}=\frac{4}{5}\)
∴ tan y = \(\frac{3 / 5}{4 / 5}=\frac{3}{4}\)
⇒ y = tan-1 \(\frac{3}{4}\)
∴ sin-1 \(\frac{3}{5}\) = tan-1 \(\frac{3}{4}\) …………..(ii)
Now, we have
L.H.S = sin-1 \(\frac{8}{17}\) + sin-1 \(\frac{3}{5}\)
[Using Eqs. (i) and (ii)]
= tan-1 \(\frac{8}{15}\) + tan-1 \(\frac{3}{4}\)
= tan-1 \(\frac{\frac{8}{15}+\frac{3}{4}}{1-\frac{8}{15} \times \frac{3}{4}}\)
= tan-1 \(\left(\frac{32+45}{60-24}\right)\)
[tan-1 x + tan-1 y = tan-1 \(\left(\frac{x+y}{1-x y}\right)\)]
= tan-1 \(\frac{77}{36}\)
= R.H.S
Hence proved.
![]()
Question 5.
cos-1 \(\frac{4}{5}\) + cos-1 \(\frac{12}{13}\) = cos-1 \(\frac{33}{65}\)
Solution.
Let cos-1 \(\frac{4}{5}\) = x
Then, cos x = \(\frac{4}{5}\)
⇒ sin x = \(\sqrt{1-\left(\frac{4}{5}\right)^{2}}=\sqrt{\frac{9}{25}}=\frac{3}{5}\)
∴ tan x = \(\frac{3 / 5}{4 / 5}=\frac{3}{4}\) …………(i)
⇒ x = tan-1 \(\frac{3}{4}\)
Now, let cos-1 \(\frac{12}{13}\) = y.
Then cos y = \(\frac{12}{13}\)
⇒ sin y = \(\sqrt{1-\left(\frac{12}{13}\right)^{2}}=\sqrt{\frac{25}{169}}=\frac{5}{13}\)
∴ tan y = \(\frac{5 / 13}{12 / 13}=\frac{5}{12}\)
⇒ y = tan-1 \(\frac{5}{12}\)
∴ cos-1 \(\frac{12}{13}\) = tan-1 \(\frac{5}{12}\) ……………(ii)
Let cos-1 \(\frac{33}{65}\) = z.
Then, cos z = \(\frac{33}{65}\)
⇒ sin z = \(\sqrt{1-\left(\frac{33}{65}\right)^{2}}=\sqrt{\frac{3136}{4225}}=\frac{56}{65}\)
∴ tan z = \(\frac{56 / 65}{33 / 65}=\frac{56}{33}\)
⇒ z = tan-1 \(\frac{56}{33}\)
∴ cos-1 \(\frac{33}{65}\) = tan-1 \(\frac{56}{33}\) …………..(iii)
Now, we have
L.H.S = cos-1 \(\frac{4}{5}\) + cos-1 \(\frac{12}{13}\)
= \(\frac{3 / 5}{4 / 5}=\frac{3}{4}\) + tan-1 \(\frac{5}{12}\)
[∵ Usin Eqs. (i) and (ii)]
= tan-1 \(\frac{\frac{3}{4}+\frac{5}{12}}{1-\frac{3}{4} \cdot \frac{5}{12}}\)
[∵ tan-1 x + tan-1 y = tan-1 \(\left(\frac{x+y}{1-x y}\right)\)]
= tan-1 \(\frac{36+20}{48-15}\)
= tan-1 \(\frac{56}{33}\)
= cos-1 \(\frac{33}{65}\) = R.H.S.
Hence proved.
![]()
Question 6.
cos-1 \(\frac{12}{13}\) + sin-1 \(\frac{3}{5}\) = sin-1 \(\frac{56}{65}\)
Solution.
Let sin-1 \(\frac{3}{5}\) = x.
Then, sin x = \(\frac{3}{5}\)
⇒ cos x= \(\sqrt{1-\left(\frac{3}{5}\right)^{2}}=\sqrt{\frac{16}{25}}=\frac{4}{5}\)
∴ tan x = \(\frac{3 / 5}{4 / 5}=\frac{3}{4}\)
⇒ x = tan-1 \(\frac{3}{4}\)
∴ sin-1 \(\frac{3}{5}\) = tan-1 \(\frac{3}{4}\) …………(i)
Now, let cos-1 \(\frac{12}{13}\) = y.
Then, cos y = \(\frac{12}{13}\)
⇒ sin y = \(\sqrt{1-\left(\frac{12}{13}\right)^{2}}=\sqrt{\frac{25}{169}}=\frac{5}{13}\)
∴ tan y = \(\frac{5 / 13}{12 / 13}=\frac{5}{12}\)
⇒ y = tan-1 \(\frac{5}{12}\)
∴ cos-1 \(\frac{12}{13}\) = tan-1 \(\frac{5}{12}\) ………………(ii)
Let sin-1 \(\frac{56}{65}\) = z.
Then, sin z = \(\frac{56}{65}\)
⇒ cos z = \(\sqrt{1-\left(\frac{56}{65}\right)^{2}}=\sqrt{\frac{1089}{4225}}=\frac{33}{65}\)
∴ tan z = \(\frac{56 / 65}{33 / 65}=\frac{56}{33}\)
⇒ z = tan-1 \(\frac{56}{33}\)
∴ sin-1 \(\frac{56}{65}\) = tan-1 \(\frac{56}{33}\) …………….(iii)
Now, we have
L.H.S. = cos-1 \(\frac{12}{13}\) + sin-1 \(\frac{3}{5}\)
= tan-1 \(\frac{3}{4}\) + tan-1 \(\frac{5}{12}\)
[Using Eqs. (i) and (ii)]
= tan-1 \(\frac{\frac{5}{12}+\frac{3}{4}}{1-\frac{5}{12} \cdot \frac{3}{4}}\)
[∵ tan-1 x + tan-1 y = tan-1 \(\left(\frac{x+y}{1-x y}\right)\)]
= tan-1 \(\frac{20+36}{48-15}\)
= tan-1 \(\frac{56}{33}\)
= sin-1 \(\frac{56}{65}\).
Hence proved.
![]()
Question 7.
tan-1 \(\frac{63}{16}\) = sin-1 \(\frac{5}{13}\) + cos -1 \(\frac{3}{5}\)
Solution.
Let sin-1 \(\frac{5}{13}\) = x
Then, sin x = \(\frac{5}{13}\)
⇒ cos x = \(\sqrt{1-\left(\frac{5}{13}\right)^{2}}=\sqrt{\frac{144}{169}}=\frac{12}{13}\)
∴ tan x = \(\frac{5 / 13}{12 / 13}=\frac{5}{12}\)
⇒ x = tan-1 \(\frac{5}{12}\)
∴ sin-1 \(\frac{5}{13}\) = tan-1 \(\frac{5}{12}\) ………….(i)
Let cos-1 \(\frac{3}{5}\) = y.
Then, cos y = \(\frac{3}{5}\)
⇒ sin y = \(\sqrt{1-\left(\frac{3}{5}\right)^{2}}=\sqrt{\frac{16}{25}}=\frac{4}{5}\)
∴ tan y = \(\sqrt{1-\left(\frac{3}{5}\right)^{2}}=\sqrt{\frac{16}{25}}=\frac{4}{5}\)
⇒ y = tan-1 \(\frac{4}{5}\)
∴ cos-1 \(\frac{3}{5}\) = tan-1 \(\frac{4}{5}\) ………………(ii)
Using Eqs. (i) and (ii), we have
R.H.S = sin-1 \(\frac{5}{13}\) + cos-1 \(\frac{3}{5}\)
= tan-1 \(\frac{5}{12}\) + tan-1 \(\frac{4}{5}\)
[∵ tan-1 x + tan-1 y = tan-1 \(\left(\frac{x+y}{1-x y}\right)\)]
= tan-1 \(\left(\frac{\frac{5}{12}+\frac{4}{3}}{1-\frac{5}{12} \times \frac{4}{3}}\right)\)
= tan-1 \(\left(\frac{15+48}{36-20}\right)\)
= tan-1 \(\frac{63}{16}\) = L.H.S.
Hence proved.
![]()
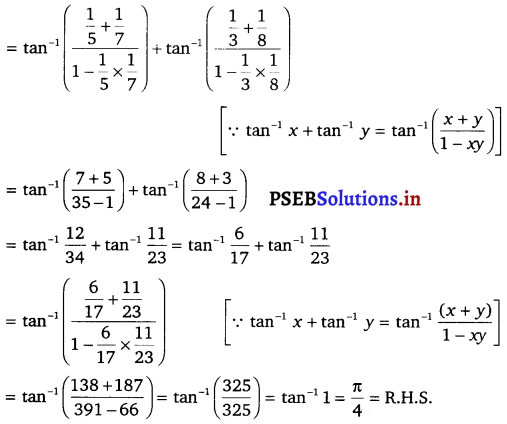
Question 8.
tan-1 \(\frac{1}{5}\) + tan-1 \(\frac{1}{7}\) + tan-1 \(\frac{1}{3}\) + tan-1 \(\frac{1}{8}\) = \(\frac{\pi}{4}\)
Solution.
tan-1 \(\frac{1}{5}\) + tan-1 \(\frac{1}{7}\) + tan-1 \(\frac{1}{3}\) + tan-1 \(\frac{1}{8}\)
![]()
Question 9.
tan-1 √x = \(\frac{1}{2}\) cos-1 \(\left(\frac{1-x}{1+x}\right)\), x ∈ [0, 1]
Solution.
Let x = tan2 θ.
Then, √x = tan θ
⇒ θ = tan-1 √x
∴ \(\frac{1-x}{1+x}=\frac{1-\tan ^{2} \theta}{1+\tan ^{2} \theta}\) = cos 2θ
[∵ cos 2θ = \(\frac{1-\tan ^{2} \theta}{1+\tan ^{2} \theta}\)]
Now, we have
R.H.S = \(\frac{1}{2}\) cos-1 \(\left(\frac{1-x}{1+x}\right)\)
= \(\frac{1}{2}\) cos-1 2θ = θ
= tan-1 √x = L.H.S
Hence Proved.
Question 10.
cot-1 \(\left(\frac{\sqrt{1+\sin x}+\sqrt{1-\sin x}}{\sqrt{1+\sin x}-\sqrt{1-\sin x}}\right)=\frac{x}{2}\), x ∈ (0, \(\frac{\pi}{4}\))
Solution.
Consider, \(\left(\frac{\sqrt{1+\sin x}+\sqrt{1-\sin x}}{\sqrt{1+\sin x}-\sqrt{1-\sin x}}\right)\)
= \(\frac{(\sqrt{1+\sin x}+\sqrt{1-\sin x})^{2}}{(\sqrt{1+\sin x})^{2}-(\sqrt{1-\sin x})^{2}}\) (By rationalising)
= \(\frac{(1+\sin x)+(1-\sin x)+2 \sqrt{(1+\sin x)(1-\sin x)}}{1+\sin x-1+\sin x}\)
= \(\frac{2\left(1+\sqrt{\left.1-\sin ^{2} x\right)}\right.}{2 \sin x}=\frac{1+\cos x}{\sin x}=\frac{2 \cos ^{2} \frac{x}{2}}{2 \sin \frac{x}{2} \cos \frac{x}{2}}\)
= cot \(\frac{x}{2}\)
L.H.S = cot-1 \(\left(\frac{\sqrt{1+\sin x}+\sqrt{1-\sin x}}{\sqrt{1+\sin x}-\sqrt{1-\sin x}}\right)\)
= cot-1 (cot \(\frac{x}{2}\))
= \(\frac{x}{2}\) = R.H.S
![]()
Question 11.
tan-1 \(\left(\frac{\sqrt{1+x}-\sqrt{1-x}}{\sqrt{1+x}+\sqrt{1-x}}\right)\) = \(\frac{\pi}{4}-\frac{1}{2} \cos ^{-1} x\), \(-\frac{1}{\sqrt{2}}\) ≤ x ≤ 1.
[Hint: put x = cos 2θ]
Solution.
Put x = cos 2θ, so that θ = \(\frac{1}{2}\) cos-1 x.
Then, we have
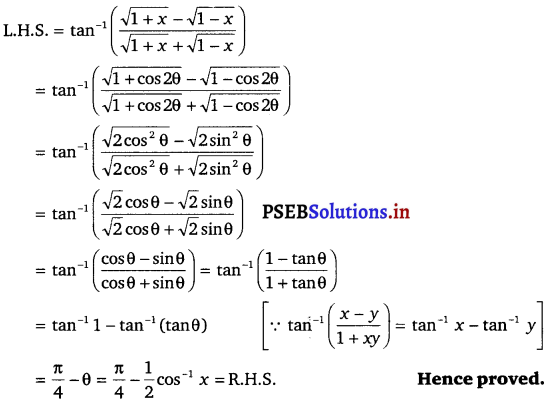
Question 12.
\(\frac{9 \pi}{8}-\frac{9}{4} \sin ^{-1} \frac{1}{3}=\frac{9}{4} \sin ^{-1} \frac{2 \sqrt{2}}{3}\)
Solution.
L.H.S = \(\frac{9 \pi}{8}-\frac{9}{4} \sin ^{-1} \frac{1}{3}\)
= \(\frac{9}{4}\left(\frac{\pi}{2}-\sin ^{-1} \frac{1}{3}\right)\)
= \(=\frac{9}{4}\left(\cos ^{-1} \frac{1}{3}\right)\) [∵ sin-1 x + cos-1 x = \(\frac{\pi}{2}\)]
= \(\frac{9}{4}\left(\sin ^{-1} \sqrt{1-\left(\frac{1}{3}\right)^{2}}\right)\)
[∵ cos-1 x = sin-1 \(\sqrt{1-x^{2}}\)]
= \(\frac{9}{4} \sin ^{-1} \sqrt{\frac{8}{9}}\)
= \(\frac{9}{4} \sin ^{-1} \frac{2 \sqrt{2}}{3}\)
= R.H.S
Hence proved.
![]()
Direction (13 – 17): Solve the following equations.
Question 13.
2 tan-1 (cos x) = tan-1 (2cosec x)
Solution.
We have, 2 tan-1 (cos x) = tan-1 (2 cosec x)
⇒ tan-1 \(\left(\frac{2 \cos x}{1-\cos ^{2} x}\right)\) = tan-1 (2 cosec x)
[∵ 2 tan-1 x = tan-1 \(\frac{(2 x)}{1-x^{2}}\)]
⇒ \(\left(\frac{2 \cos x}{1-\cos ^{2} x}\right)\) = 2 cosec x
⇒ \(\frac{2 \cos x}{\sin ^{2} x}=\frac{2}{\sin x}\)
⇒cos x = sin x
⇒ tan x = 1 = tan \(\frac{\pi}{4}\).
![]()
Question 14.
tan-1 \(\frac{1-x}{1+x}\) = \(\frac{1}{2}\) tan-1 x, (x > 0)
Solution.
We have, sin-1 (1 – x) – 2 sin-1 x = \(\frac{\pi}{2}\)
⇒ – 2 sin-1 x = \(\frac{\pi}{2}\) – sin-1 (1 – x)
⇒ – 2 sin-1 x = cos-1 (1 – x) ………….(i)
Let sin-1 x = θ
⇒ sin θ = x
⇒ cos θ = \(\sqrt{1-x^{2}}\)
∴ θ = cos-1 \(\sqrt{1-x^{2}}\)
∴ sin-1 x = cos-1 \(\sqrt{1-x^{2}}\)
Therefore, from Eq. (i), we have
– 2 cos-1 (\(\sqrt{1-x^{2}}\) ) = cos-1 (1 – x)
Put x = sin y. Then, we have
– 2 cos-1 (\(\)) = cos-1 (1 – sin y)
⇒ – 2 cos-1 (cos y) = cos-1 (1 – sin y)
⇒ – 2y = cos-1 (1 – sin y)
⇒ 1 – sin y = cos(- 2y) = cos 2y
⇒ 1 – sin y = 1 – 2 sin2 y
⇒ 2 sin2 y – sin y = 0
⇒ sin y(2 sin y – 1) = 0
sin y = 0 or \(\frac{1}{2}\)
∴ x = 0 or x = \(\frac{1}{2}\)
But, when x = \(\frac{1}{2}\), it can be observed that
We have, tan-1 \(\frac{1-x}{1+x}\) = \(\frac{1}{2}\) tan-1 x
⇒ tan-1 1 – tan-1 x = \(\frac{1}{2}\) tan-1 x
[∵ tan-1 x – tan-1 y = tan-1 \(\frac{(x-y)}{1+x y}\)]
[∵ tan-1 (1) = \(\frac{\pi}{4}\)]
⇒ \(\frac{\pi}{4}\) = \(\frac{3}{2}\) tan-1 x
⇒ tan-1 x = \(\frac{\pi}{6}\)
⇒ x = tan \(\frac{\pi}{6}\)
∴ x = \(\frac{1}{\sqrt{3}}\).
![]()
Question 15.
sin(tan-1 x), |x| < 1 is equal to
(A) \(\frac{x}{\sqrt{1-x^{2}}}\)
(B) \(\frac{1}{\sqrt{1-x^{2}}}\)
(C) \(\frac{1}{\sqrt{1+x^{2}}}\)
(D) \(\frac{x}{\sqrt{1+x^{2}}}\)
Solution.
Let tan-1 x = y.
Then, tan y = x
⇒ sin y = \(\frac{x}{\sqrt{1+x^{2}}}\)
∴ y = sin-1 (\(\frac{x}{\sqrt{1+x^{2}}}\))
⇒ tan-1 x = sin-1 (\(\frac{x}{\sqrt{1+x^{2}}}\))
Now, sin(tan-1 x) = sin(sin-1 (\(\frac{x}{\sqrt{1+x^{2}}}\)))
= \(\frac{x}{\sqrt{1+x^{2}}}\)
The correct answer is (D).
Question 16.
sin-1 (1 – x) – 2 sin-1 x = \(\frac{\pi}{2}\), then x is equal to
(A) 0, \(\frac{1}{2}\)
(B) 1, \(\frac{1}{2}\)
(C) 0
(D) \(\frac{1}{2}\)
Solution.
Given, sin-1 (1 – x) – 2 sin-1 x = \(\frac{\pi}{2}\)
putting \(\frac{\pi}{2}\) = sin-1 (1 – x) + cos-1 (1 – x)
or sin-1 (1 – x) – 2 sin-1 (1 – x) = sin-1 (1 – x) + cos-1 (1 – x)
⇒ – 2 sin-1 x = cos-1 (1 – x)
Let sin-1 x = α
∴ sin α = x
∴ – 2 sin-1 x = – 2 α = cos-1 (1 – x)
or cos 2α = 1 – x [∵ cos(- θ) = cos θ]
∴ 1 – 2 sin2 α = (1 – x)
Putting sin α = x
⇒ 1 – 2x2 = 1 – x
or 2x2 – x = 0
x(2x – 1) = 0
∴ x = 0, \(\frac{1}{2}\)
But x = \(\frac{1}{2}\) does not satisfy the equation.
∴ x = 0
Hence, the correct answer is (C).
![]()
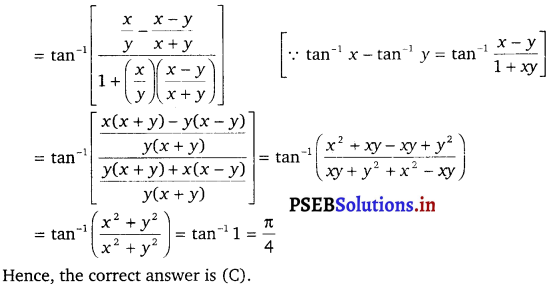
Question 17.
tan-1 \(\left(\frac{x}{y}\right)\) – tan-1 \(\frac{x-y}{x+y}\) is equal to
(A) \(\frac{\pi}{2}\)
(B) \(\frac{\pi}{3}\)
(C) \(\frac{\pi}{4}\)
(D) \(\frac{3 \pi}{4}\)
Solution.
We have tan-1 \(\left(\frac{x}{y}\right)\) – tan-1 \(\frac{x-y}{x+y}\)